The basic proportionality theorem was found by a famous greek mathematician Thales. It is also called the Thales theorem. According to the basic proportionality theorem, if a line is drawn parallel to one side of a triangle and intersect the other two sides, then the line divides the two sides in the same ratio. The application of BPT is given here for the sake of the students.
Application of Basic Proportionality Theorem
The application of the basic proportionality theorem states that the internal bisector of an angle of a triangle divides the opposite side in the ratio of the sides containing the angle.
The proof for the statement is mentioned here:
Given: XP is the internal bisector of ∠YXZ, intersecting YZ at P.
To prove: \(\frac { YP }{ PZ } \) = \(\frac { XY }{ XZ } \)
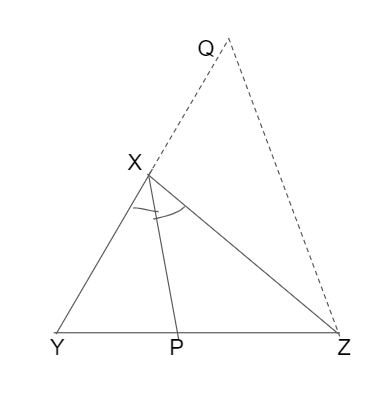
Construction: Draw ZQ ∥ XP such that ZQ meets YX produced at Q.
Proof:
| Statement | Reason |
|---|---|
| ∠YXP = ∠XQZ | XP ∥ QZ and YQ is a transversal |
| ∠PXZ = ∠XZQ | XP ∥ QZ and XZ is a transversal |
| ∠XQZ = ∠XZQ | ∠YXP = ∠PXZ |
| XQ = XZ | ∠XQZ = ∠XZQ |
| \(\frac { YX }{ XQ } \) = \(\frac { YP }{ PZ } \) | XP ∥ QZ |
| \(\frac { YX }{ XZ } \) = \(\frac { YP }{ PZ } \) | From Statement 4 |
Hence proved.
Also, See
Important Points:
- The above statement is true for the external division also. So, \(\frac { YP }{ ZP } \) = \(\frac { XY }{ XZ } \)
- Converse of the above proposition is also true. If P is the point on YZ such that \(\frac { YP }{ PZ } \) = \(\frac { XY }{ XZ } \), then XP bisects ∠YXZ internally or externally.
Solved Questions on Thales Theorem
Question 1:
In triangle ABC, DE || BC. If AD = x cm, DB = x-2 cm, AE = x +2, and EC = x-1 cm, then find the value of x.
Solution:
Given that,
In the ∆ABC, DE || BC
According to the basic proportionality theorem, \(\frac { AD }{ DB } \) = \(\frac { AE }{ EC } \)
Substitute the values in the above equation.
\(\frac { x }{ x-2 } \) = \(\frac { x+2 }{ x-1 } \)
x² – x = x² – 4
-x = -4
x = 4
Therefore, the value of x = 4.
Question 2:
In a given ∆XYZ, the line PQ meeting the sides XY and XZ at P and Q respectively is parallel to YZ. If XP = 6 cm, XQ = 7.5 cm, PY = 2 cm, then find QZ.
Solution:
In ∆XYZ,
\(\frac { XP }{ PY } \) = \(\frac { XQ }{ QZ } \) (as per basic proportionality theorem)
\(\frac { 6 }{ 2 } \) = \(\frac { 7.5 }{ QZ } \)
3 = \(\frac { 7.5 }{ QZ } \)
QZ = \(\frac { 7.5 }{ 3 } \)
QZ = 2.5 cm
Question 3:
ABCD is a trapezium in which AB || DC and P,Q are points on AD and BC respectively, such that PQ || DC if PD = 18 cm, BQ = 35 cm and QC = 15 cm, find AD?
Solution:
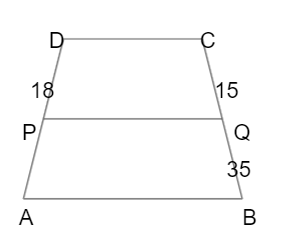
Join D, B
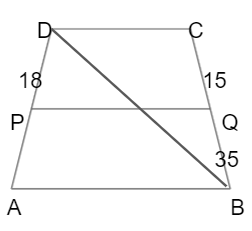
In △DAB,
\(\frac { PD }{ PA } \) = \(\frac { DT }{ TB } \) —– (i)
In △DBC
\(\frac { BT }{ TD } \) = \(\frac { BQ }{ QC } \)
By taking the reciprocal on both sides
\(\frac { TD }{ BT } \) = \(\frac { QC }{ BQ } \) —- (ii)
Equating both statements
\(\frac { PD }{ PA } \) = \(\frac { QC }{ BQ } \)
\(\frac { 18 }{ PA } \) = \(\frac { 15 }{ 35 } \)
PA = 42
AD = 42 + 18
AD = 60 cm
FAQ’s on Application of BPT
1. What is the converse of the basic proportionality theorem?
The converse of basic proportionality theorem states that if a line divides any two sides of a triangle in the same ratio, then the line must be parallel to the third side.
2. How to use the BPT theorem?
According to Thales theorem, if a line is drawn parallel to one side of a triangle to intersect the other two sides in two different points then the other two sides are divided in the same ratio.
3. What is the application of the basic proportionality theorem?
One of the applications of BPT is the internal bisector of an angle of a triangle divides the opposite side in the ratio of the sides containing the angle.