Big Ideas Math Answers Grade 4 Chapter 8 Add and Subtract Fractions

Big Ideas Math Answers Grade 4 Chapter 8 Add and Subtract Fractions: Free step by step solutions to Big Ideas Math Grade 4 Chapter 8 Add and Subtract Fractions is available here. The Big Ideas Math Answers Grade 4 Chapter 8 Add and Subtract Fractions are given in the pdf format. So Download Big ideas Math Answers Grade 4 Chapter 8 Add and Subtract Fractions pdf for free. By referring to the BIM 4th Grade Chapter 8 Add & Subtract Fractions you can finish your assignment and homework in time.

Big Ideas Math Book 4th Grade Answer Key Chapter 8 Add and Subtract Fractions

Practice Big Ideas Math Chapter 8 Add and Subtract Fractions Questions you can score good marks in the exams. We have provided the solutions according to the topics. This chapter includes Decompose Fractions, Add Fractions with Like Denominators, Subtract Fractions with Like Denominators, Add Mixed Numbers, Subtract Mixed Numbers, etc. You can get the solutions for all the topics in an easy manner in different methods. Tap the links and start practicing the problems and improve your math skills.

Lesson 1: Use Models to Add Fractions

• Lesson 8.1 Use Models to Add Fractions
• Use Models to Add Fractions Homework & Practice 8.1

Lesson 2: Decompose Fractions

• Lesson 8.2 Decompose Fractions
• Decompose Fractions Homework & Practice 8.2

Lesson 3: Add Fractions with Like Denominators

• Lesson 8.3 Add Fractions with Like Denominators
• Add Fractions with Like Denominators Homework & Practice 8.3

Lesson 4: Use Models to subtract Fractions

• Lesson 8.4 Use Models to subtract Fractions
• Use Models to subtract Fractions Homework & Practice 8.4

Lesson 5: Subtract Fractions with Like Denominators

• Lesson 8.5 Subtract Fractions with Like Denominators
• Subtract Fractions with Like Denominators Homework & Practice 8.5

Lesson 6: Model Fractions and Mixed Numbers

• Lesson 8.6 Model Fractions and Mixed Numbers
• Model Fractions and Mixed Numbers Homework & Practice 8.6

Lesson 7: Add Mixed Numbers

• Lesson 8.7 Add Mixed Numbers
• Add Mixed Numbers Homework & Practice 8.7

Lesson 8: Subtract Mixed Numbers

• Lesson 8.8 Subtract Mixed Numbers
• Subtract Mixed Numbers Homework & Practice 8.8

Lesson 9: Problem Solving: Fractions

• Lesson 8.9 Problem Solving: Fractions
• Problem Solving: Fractions Homework & Practice 8.9

Performance Task

• Add and Subtract Fractions Performance Task 8
• Add and Subtract Fractions Activity
• Add and Subtract Fractions Chapter Practice 8

Lesson 8.1 Use Models to Add Fractions

Explore and Grow

Draw models to show \(\frac{2}{8}\) and \(\frac{5}{8}\).

Answer:
You can add fractions by joining parts that refer to the same whole.

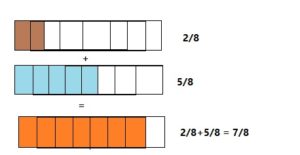
Use your models to find \(\frac{2}{8}\) + \(\frac{5}{8}\). Explain your method.

Answer:
Combine the like terms
\(\frac{2}{8}\) + \(\frac{5}{8}\) = (2 + 5)/8 = 7/8

Repeated Reasoning
Write two fractions that have a sum of \(\frac{6}{8}\). Explain your reasoning.

Think and Grow: Use Models to Add Fractions

You can add fractions by joining parts that refer to the same whole.

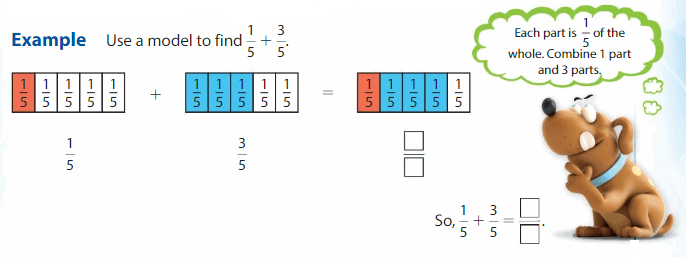
Answer:
The denominators of the fraction are the same so you have to add numerators.
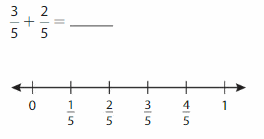
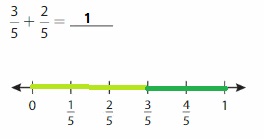
\(\frac{1}{5}\) + \(\frac{3}{5}\) = \(\frac{4}{5}\)

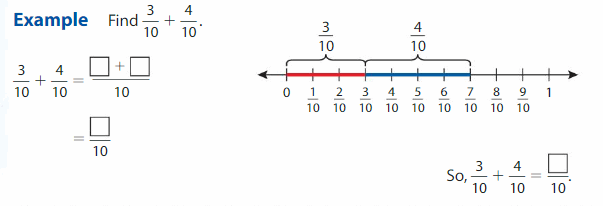
Example
Use a number line to find \(\frac{5}{4}\) + \(\frac{2}{4}\).

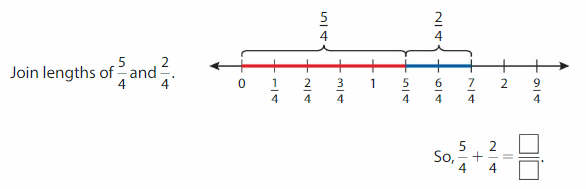
Answer:
The denominators of the fraction are the same so you have to add numerators.
\(\frac{5}{4}\) + \(\frac{2}{4}\) = \(\frac{7}{4}\)

Show and Grow

Find the sum. Explain how you used the model to add.

Question 1.

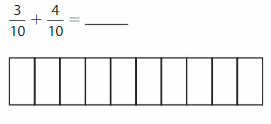
Answer:
The denominators of the fraction are the same so you have to add numerators.

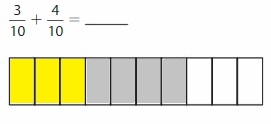
(3+4)/10 = 7/10

Question 2.

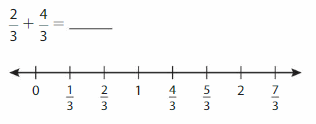
Answer:
The denominators of the fraction are the same so you have to add numerators.

Apply and Grow: Practice

Find the sum. Use a model or a number line to help.

Question 3.

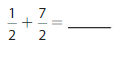
Answer:
The denominators of the fraction are the same so you have to add numerators.

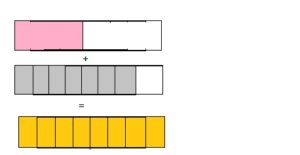
Question 4.

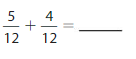
Answer:
The denominators of the fraction are the same so you have to add numerators.

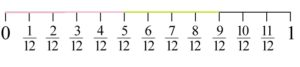
\(\frac{5}{12}\) + \(\frac{4}{12}\) = \(\frac{9}{12}\)

Question 5.

Answer:
The denominators of the fraction are the same so you have to add numerators.

Question 6.

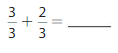
Answer:
The denominators of the fraction are the same so you have to add numerators.

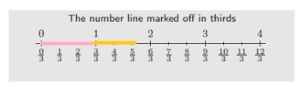
Question 7.

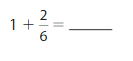
Answer:
The denominators of the fraction are the same so you have to add numerators.

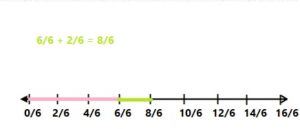
Question 8.

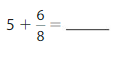
Answer:
Add a fraction to the whole number.

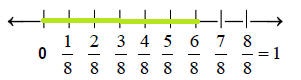
5 + \(\frac{6}{8}\) = \(\frac{46}{8}\)

Question 9.

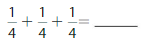
Answer:
The denominators of the fraction are the same so you have to add numerators.

Question 10.

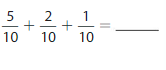
Answer:
The denominators of the fraction are the same so you have to add numerators.

Question 11.

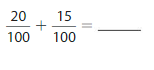
Answer:
The denominators of the fraction are the same so you have to add numerators.

Question 12.
Structure
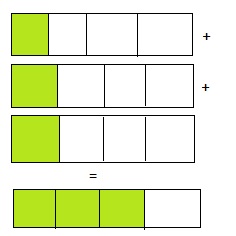
Write the addition equation represented by the models.

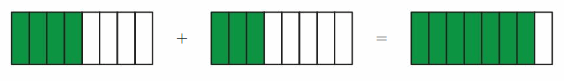
Answer:
By seeing the above model we can find the addition equation.
\(\frac{4}{8}\) + \(\frac{3}{8}\) = \(\frac{7}{8}\)

Question 13.
Open-Ended
Write three fractions with different numerators that have a sum of 1.

Answer:
\(\frac{2}{8}\) + \(\frac{5}{8}\) + \(\frac{1}{8}\) = \(\frac{8}{8}\) = 1

Question 14.
Writing
Explain why \(\frac{1}{8}\) + \(\frac{4}{8}\) does not equal \(\frac{5}{16}\).

Answer:
In the above expressions, the denominators are the same but the numerators are different.
So, you have to add the numerators not denominators.
\(\frac{1}{8}\) + \(\frac{4}{8}\) = \(\frac{5}{8}\)

Think and Grow: Modeling Real Life

Example
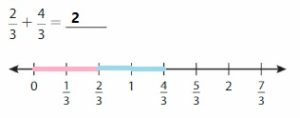
You need \(\frac{2}{3}\) cup of hot water and \(\frac{4}{3}\) cups of cold water for a science experiment. How many cups of water do you need in all?

Because each fraction represents a part of the same whole you can join the parts.
Use a model to find \(\frac{2}{3}\) + \(\frac{4}{3}\).

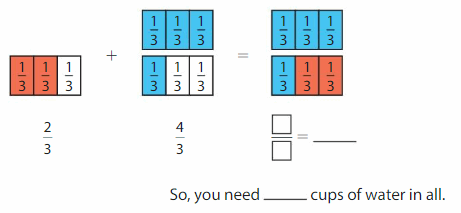
Answer:
Given that,
You need \(\frac{2}{3}\) cup of hot water and \(\frac{4}{3}\) cups of cold water for a science experiment.

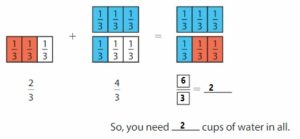
Thus you need 2 cups of water in all.

Show and Grow

Question 15.
You cut a foam noodle for a craft. You use \(\frac{2}{4}\) of the noodle for one part of the craft and \(\frac{1}{4}\) of the noodle for another part. What fraction of the foam noodle do you use altogether?

Answer:
Given that,
You cut a foam noodle for a craft. You use \(\frac{2}{4}\) of the noodle for one part of the craft and \(\frac{1}{4}\) of the noodle for another part.
\(\frac{2}{4}\) + \(\frac{1}{4}\) = \(\frac{3}{4}\)
Thus \(\frac{3}{4}\) of the foam noodle is used.

Question 16.
You make a fruit drink using \(\frac{4}{8}\) gallon of orange juice, \(\frac{2}{8}\) gallon of mango juice, and \(\frac{4}{8}\) gallon of pineapple juice. How much juice do you use in all?

Answer:
Given that,
You make a fruit drink using \(\frac{4}{8}\) gallon of orange juice, \(\frac{2}{8}\) gallon of mango juice, and \(\frac{4}{8}\) gallon of pineapple juice.
\(\frac{4}{8}\) + \(\frac{2}{8}\) = \(\frac{6}{8}\)
\(\frac{6}{8}\) + \(\frac{4}{8}\) = \(\frac{10}{8}\)
Thus you used \(\frac{10}{8}\) fraction of juice.

Question 17.
DIG DEEPER!
A community plants cucumbers in \(\frac{5}{12}\) of a garden, broccoli in \(\frac{3}{12}\) of the garden, and carrots in \(\frac{4}{12}\) of the garden. What fraction of the garden is planted with green vegetables?

Answer:
Given that,
A community plants cucumbers in \(\frac{5}{12}\) of a garden, broccoli in \(\frac{3}{12}\) of the garden, and carrots in \(\frac{4}{12}\) of the garden.
\(\frac{5}{12}\) + \(\frac{3}{12}\) + \(\frac{4}{12}\) = \(\frac{12}{12}\) = 1
\(\frac{12}{12}\) fraction of the garden is planted with green vegetables.

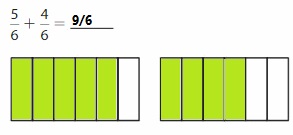
Use Models to Add Fractions Homework & Practice 8.1

Find the sum. Explain how you used the model to add.

Question 1.

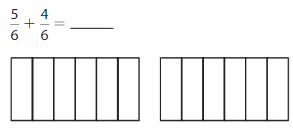
Answer: \(\frac{9}{6}\)

Question 2.

Answer: 1

Find the sum. Use a model or a number line to help.

Question 3.

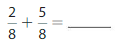
Answer: \(\frac{7}{8}\)
You can add fractions by joining parts that refer to the same whole.

Question 4.

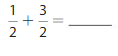
Answer: 2

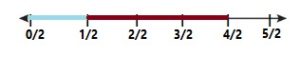
Question 5.

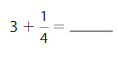
Answer: 3 \(\frac{1}{4}\)

Explanation:
Add fraction to the whole number.
\(\frac{1}{4}\) + 3
\(\frac{1}{4}\) + 3 × \(\frac{4}{4}\)
\(\frac{1}{4}\) + \(\frac{13}{4}\) = \(\frac{13}{4}\)

Question 6.

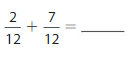
Answer: \(\frac{9}{12}\)

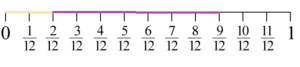
Question 7.

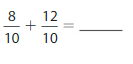
Answer: 2

\(\frac{12}{10}\) = \(\frac{10}{10}\) + \(\frac{2}{10}\)
\(\frac{10}{10}\) + \(\frac{2}{10}\) + \(\frac{8}{10}\) = \(\frac{20}{10}\) = 2

Question 8.

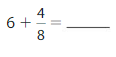
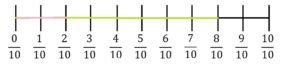
Answer:
4/8 = 1/2
6 + 1/2 = (12 + 1)/2 = 13/2

Find the sum. Use a model or a number line to help.

Question 9.

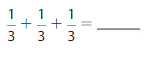
Answer:
Add all the three unit fractions.

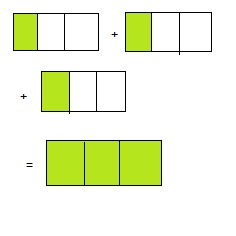
Question 10.

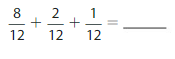
Answer:

Question 11.

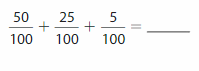
Answer:
The denominators of all the fractions are the same. So you have to add the numerators of the fraction.
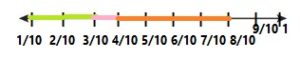
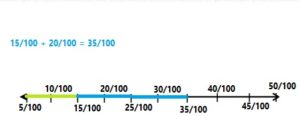
\(\frac{50}{100}\) + \(\frac{25}{100}\) + \(\frac{5}{100}\) = \(\frac{80}{100}\)

Question 12.
YOU BE THE TEACHER
Newton says \(\frac{3}{5}\) + \(\frac{1}{5}\) = \(\frac{4}{10}\). Descartes says the sum is \(\frac{4}{5}\). Who is correct? Explain.

Answer:
Newton says \(\frac{3}{5}\) + \(\frac{1}{5}\) = \(\frac{4}{10}\). Descartes says the sum is \(\frac{4}{5}\).
Descartes is correct.
\(\frac{3}{5}\) + \(\frac{1}{5}\) = \(\frac{4}{5}\)
You have to add numerators, not denominators.
So, Newton’s equation is not correct.

Question 13.
Make each statement true by writing two fractions whose denominators are the same and whose numerators are 3 and 2.
The sum of ___ and __ is greater than 1.
___________________________
The sum of ___ and ___ is less than 1.
___________________________
The sum of ___ and ___ is equal to 1.

Answer:
The sum of 3/2 and 2/2 is greater than 1.
3/2 + 2/2 = 5/2
5/2 > 1
The sum of 3/6 and 2/6 is less than 1.
3/6 + 2/6 = 5/6
5/6 < 1
The sum of 3/5 and 2/5 is equal to 1.
3/5 + 2/5 = 5/5 = 1

Question 14.
Modeling Real Life
Your teacher assigns 5 pages to read. You read \(\frac{3}{5}\) of the pages in class and \(\frac{1}{5}\) of the pages at home. What fraction of the reading assignment is complete?

Answer:
Given that,
Your teacher assigns 5 pages to read. You read \(\frac{3}{5}\) of the pages in class and \(\frac{1}{5}\) of the pages at home.
The denominators of all the fractions are the same. So you have to add the numerators of the fraction.
\(\frac{3}{5}\) + \(\frac{1}{5}\) = \(\frac{4}{5}\)

Question 15.
Modeling Real Life
In the Sahara Desert, it rains \(\frac{2}{10}\) inch in September, \(\frac{3}{10}\) inch in October, and \(\frac{5}{10}\) inch in November. How much does it rain in the 3 months?

Answer:
Given that,
In the Sahara Desert, it rains \(\frac{2}{10}\) inch in September, \(\frac{3}{10}\) inch in October, and \(\frac{5}{10}\) inch in November.
\(\frac{2}{10}\) + \(\frac{3}{10}\) + \(\frac{5}{10}\)
The denominators of all the fractions are the same. So you have to add the numerators of the fraction.
\(\frac{2}{10}\) + \(\frac{3}{10}\) + \(\frac{5}{10}\) = (2 + 3 + 5)/10 = 10/10 = 1
It rains 10/10 in the 3 months.

Review & Refresh

Tell whether the number is prime or composite. Explain.

Question 16.
37

Answer: 37 is a prime number.
A prime number is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number.

Question 17.
21

Answer: 21 is a composite number.
A composite number is a positive integer that can be formed by multiplying two smaller positive integers. Equivalently, it is a positive integer that has at least one divisor other than 1 and itself.

Question 18.
99

Answer: 99 is a composite number.
A composite number is a positive integer that can be formed by multiplying two smaller positive integers. Equivalently, it is a positive integer that has at least one divisor other than 1 and itself.

Lesson 8.2 Decompose Fractions

Explore and Grow

Use a model to find .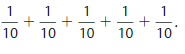

How can you write \(\frac{7}{10}\) as a sum of unit fractions? Explain your reasoning.

Answer: The sum of unit fraction of \(\frac{7}{10}\) is \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\)

Structure
Explain how you can write \(\frac{7}{10}\) as a sum of two fractions. Draw a model to support your answer.

Answer: You can write sum of \(\frac{7}{10}\) as \(\frac{2}{10}\) + \(\frac{5}{10}\)

Think and Grow: Decompose Fractions

A unit fraction represents one equal part of a whole. You can write a fraction as a sum of unit fractions.

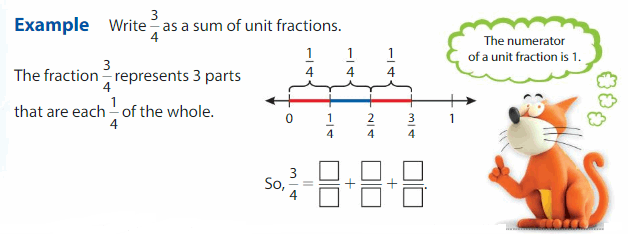
Answer:

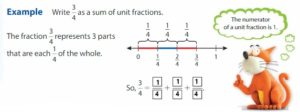
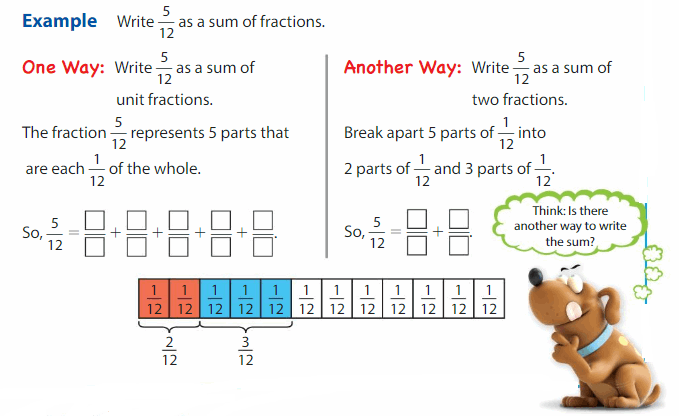
Answer:

Show and Grow

Question 1.
Write \(\frac{4}{5}\) as a sum of unit fractions.

Answer: The unit fraction of \(\frac{4}{5}\) is \(\frac{1}{5}\) + \(\frac{1}{5}\) + \(\frac{1}{5}\) + \(\frac{1}{5}\)
A unit fraction is a rational number written as a fraction where the numerator is one and the denominator is a positive integer.

Question 2.
Write \(\frac{5}{6}\) as a sum of fractions in two different ways.

Answer: The unit fraction of \(\frac{5}{6}\) is \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)
A unit fraction is a rational number written as a fraction where the numerator is one and the denominator is a positive integer.
You can also write \(\frac{5}{6}\) as \(\frac{2}{6}\) + \(\frac{3}{6}\)
That means \(\frac{5}{6}\) can be written as 2 parts of \(\frac{1}{6}\) and 3 parts of \(\frac{1}{6}\)

Apply and Grow: Practice

Question 3.
\(\frac{4}{7}\)

Answer: The unit fraction of \(\frac{4}{7}\) is \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\)
A unit fraction is a rational number written as a fraction where the numerator is one and the denominator is a positive integer.

Question 4.
\(\frac{7}{8}\)

Answer: The unit fraction of \(\frac{7}{8}\) is \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)
A unit fraction is a rational number written as a fraction where the numerator is one and the denominator is a positive integer.

Question 5.
\(\frac{3}{10}\)

Answer: The unit fraction of \(\frac{3}{10}\) is  \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\)
A unit fraction is a rational number written as a fraction where the numerator is one and the denominator is a positive integer.

Question 6.
\(\frac{10}{100}\)

Answer: The unit fraction of \(\frac{10}{100}\) is \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\)
A unit fraction is a rational number written as a fraction where the numerator is one and the denominator is a positive integer.

Question 7.
\(\frac{6}{2}\)

Answer: 3
The unit fraction of \(\frac{6}{2}\) is \(\frac{1}{2}\) + \(\frac{1}{2}\) + \(\frac{1}{2}\) + \(\frac{1}{2}\) + \(\frac{1}{2}\) + \(\frac{1}{2}\)
A unit fraction is a rational number written as a fraction where the numerator is one and the denominator is a positive integer.

Question 8.
\(\frac{9}{4}\)

Answer:
Break apart 9 parts of \(\frac{1}{4}\) into 5 parts of \(\frac{1}{4}\) and 4 parts of \(\frac{1}{4}\).

Question 9.
\(\frac{8}{12}\)

Answer: Break apart 8 parts of \(\frac{1}{12}\) into 5 parts of \(\frac{1}{12}\) and 3 parts of \(\frac{1}{12}\).

Question 10.
\(\frac{5}{3}\)

Answer: The unit fraction of \(\frac{5}{3}\) is \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\)
A unit fraction is a rational number written as a fraction where the numerator is one and the denominator is a positive integer.

Question 11.
Writing
You write \(\frac{4}{6}\) as a sum of unit fractions. Explain how the numerator of \(\frac{4}{6}\) is related to the number of addends.

Answer: The unit fraction of \(\frac{4}{6}\) is \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)
A unit fraction is a rational number written as a fraction where the numerator is one and the denominator is a positive integer.
Also, you can write \(\frac{4}{6}\) as 4 parts of \(\frac{1}{6}\), 2 equal parts of \(\frac{1}{6}\) and 2 equal parts of \(\frac{1}{6}\).

Question 12.
DIG DEEPER!
Why is it important to be able to write a fraction as a sum of fractions in different ways?

Answer:
Asking students to write a fraction as a sum of unit fractions, or as a sum of other fractions, encourages students to make sense of quantities and their relationships. Students further develop their understandings about fractions and decomposing numbers through this process.

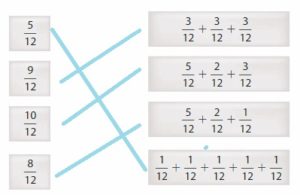
Question 13.
Precision
Match each fraction with an equivalent expression.

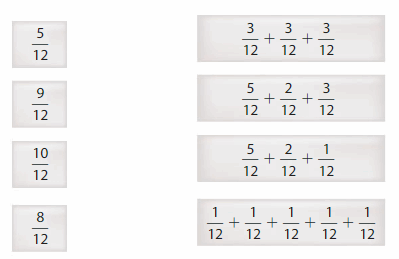
Answer:

Think and Grow: Modeling Real Life

Example
A chef has \(\frac{8}{10}\) liter of soup. How can the chef pour all of the soup into 2 bowls?

Break apart \(\frac{8}{10}\) into any two fractions that have a sum of \(\frac{8}{10}\).

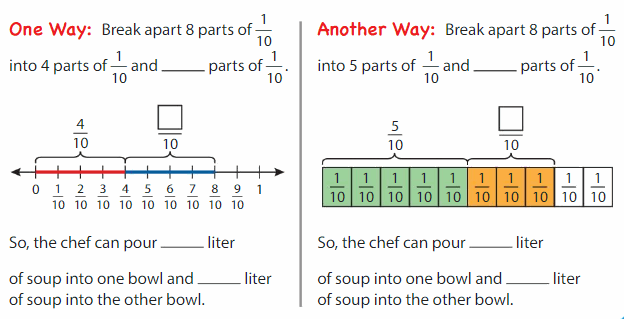
Answer:

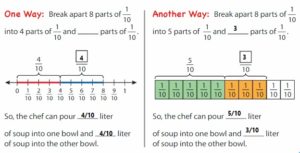
Show and Grow

Question 14.
You have \(\frac{7}{3}\) pounds of almonds. What are two different ways you can put all of the almonds into 2 bags?

Answer:
Given that,
You have \(\frac{7}{3}\) pounds of almonds.
Break apart 7 parts of \(\frac{1}{3}\) into 5 parts of \(\frac{1}{3}\) and 2 parts of \(\frac{1}{3}\)
Thus you can put 5 parts of \(\frac{1}{3}\) and 2 parts of \(\frac{1}{3}\) of the almonds into 2 bags.

Question 15.
A 3-person painting crew has \(\frac{10}{12}\) of a fence left to paint. What is one way the crew can finish painting the fence when each person paints a fraction of the fence?

Answer:
Given that,
A 3-person painting crew has \(\frac{10}{12}\) of a fence left to paint.
\(\frac{10}{12}\) can be written as \(\frac{3}{12}\) + \(\frac{3}{12}\) + \(\frac{4}{12}\)
Thus each person paints \(\frac{3}{12}\) + \(\frac{3}{12}\) + \(\frac{4}{12}\) fraction of the fence.

Question 16.
DIG DEEPER!
Three teammates have to run a total of miles for a relay race. Can each team member run the same fraction of a mile, in fourths, to complete the race? Explain.

Answer:
Three teammates have to run a total of miles for a relay race.
No three members cannot run the same fraction of a mile, in fourths, to complete the race
\(\frac{10}{12}\) can be written as \(\frac{3}{12}\) + \(\frac{3}{12}\) + \(\frac{4}{12}\)

Decompose Fractions Homework & Practice 8.2

write the fraction as a sum of unit fractions.

Question 1.
\(\frac{2}{2}\)

Answer: 1
The sum of unit fractions of \(\frac{2}{2}\) is \(\frac{1}{2}\) + \(\frac{1}{2}\)

Question 2.
\(\frac{3}{5}\)

Answer: The sum of unit fractions of \(\frac{3}{5}\) is \(\frac{1}{5}\) + \(\frac{1}{5}\) + \(\frac{1}{5}\)

Question 3.
\(\frac{4}{3}\)

Answer: The sum of unit fractions of \(\frac{4}{3}\) is \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\)

Question 4.
\(\frac{6}{4}\)

Answer: The sum of unit fractions of \(\frac{6}{4}\) is \(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\)

write the fraction as a sum of fractions in two different ways.

Question 5.
\(\frac{8}{12}\)

Answer: The sum of unit fractions of \(\frac{8}{12}\) is \(\frac{1}{12}\) + \(\frac{1}{12}\) + \(\frac{1}{12}\) + \(\frac{1}{12}\) + \(\frac{1}{12}\) + \(\frac{1}{12}\) + \(\frac{1}{12}\) + \(\frac{1}{12}\)

Another Way:
Break apart \(\frac{8}{12}\) as 4 parts of \(\frac{1}{12}\) and 4 parts of \(\frac{1}{12}\)

Question 6.
\(\frac{10}{6}\)

Answer: The sum of unit fractions of \(\frac{10}{6}\) is \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)

Another way:
Break apart \(\frac{10}{6}\) as 5 parts of \(\frac{1}{6}\) and 5 parts of \(\frac{11}{6}\)

Question 7.
\(\frac{11}{100}\)

Answer: The sum of unit fractions of \(\frac{11}{100}\) is \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\)

Another way:
Break apart \(\frac{11}{100}\) as 5 parts of \(\frac{1}{100}\), 4 parts of \(\frac{1}{100}\) and 2 parts of \(\frac{1}{100}\)

Question 8.
\(\frac{14}{8}\)

Answer: The sum of unit fractions of \(\frac{14}{8}\) is \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)

Another way:
Break apart \(\frac{14}{8}\) as 5 parts of \(\frac{1}{8}\), 9 parts of \(\frac{1}{8}\)

Question 9.
Which One Doesn’t Belong? Which expression does belong with the other three?

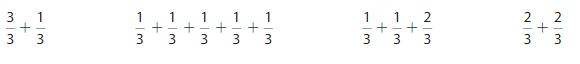
Answer: The expression \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) does not belong to the other three.

Question 10.

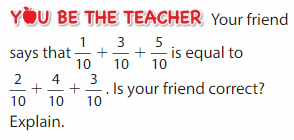
Answer: Yes your friend is correct.
\(\frac{1}{10}\) + \(\frac{3}{10}\)  + \(\frac{5}{10}\)
Here the denominators are the same so you have to add the numerators.
\(\frac{1}{10}\) + \(\frac{3}{10}\)  + \(\frac{5}{10}\) = \(\frac{9}{10}\)
\(\frac{2}{10}\) + \(\frac{4}{10}\) + \(\frac{3}{10}\)
Here the denominators are the same so you have to add the numerators.
\(\frac{2}{10}\) + \(\frac{4}{10}\) + \(\frac{3}{10}\) = \(\frac{9}{10}\)

Question 11.
Number Sense
Is it possible to write \(\frac{7}{12}\) as the sum of three fractions with three different numerators and the same denominator? Explain.

Answer: Yes it is possible to write \(\frac{7}{12}\) as the sum of three fractions with three different numerators and the same denominator.
\(\frac{7}{12}\) = \(\frac{3}{12}\) + \(\frac{3}{12}\) + \(\frac{1}{12}\)

Question 12.
You have \(\frac{8}{4}\) pounds of dried pineapple. What are two different ways you can put all of the pineapples into 2 bags?

Answer:
Given that,
You have \(\frac{8}{4}\) pounds of dried pineapple.
Break apart \(\frac{8}{4}\) as 4 parts of \(\frac{1}{4}\) and 4 parts of \(\frac{1}{4}\).
The two different ways you can put all of the pineapples into 2 bags are 4 parts of \(\frac{1}{4}\).

Question 13.
DIG DEEPER!
A carpenter has 3 planks of wood. Each plank has a different thickness. When stacked, the thickness of the 3 planks is \(\frac{6}{8}\) inch. What are the possible thickness of each plank?

Answer:
Given that,
A carpenter has 3 planks of wood. Each plank has a different thickness.
When stacked, the thickness of the 3 planks is \(\frac{6}{8}\) inch.
\(\frac{6}{8}\) = \(\frac{2}{8}\) + \(\frac{3}{8}\) + \(\frac{1}{8}\)
The possible thickness of each plank are \(\frac{2}{8}\), \(\frac{3}{8}\), \(\frac{1}{8}\)

Review & Refresh

Find the product. Check whether your answer is reasonable.

Question 14.
Estimate: ___
608 × 5 = ___

Answer:
600 × 5 = 3000
The number close to 608 is 600.
Step 2:
608 × 5 = 3040
3040 is close to 3000. So, the answer is reasonable.

Question 15.
Estimate: ___
7 × 5,394 = ___

Answer:
7 × 5400 = 37,800
The number close to 5394 is 5400.
Step 2:
7 × 5394 = 37,758
37,758 is close to 37,800. So, the answer is reasonable.

Question 16.
Estimate: ___
927 × 3 = ___

Answer:
900 × 3 = 2700
The number close to 927 is 900.
Step 2:
927 × 3 = 2781
2781 is close to 2700. So, the answer is reasonable.

Lesson 8.3 Add Fractions with Like Denominators

Explore and Grow

Write each fraction as a sum of unit fractions. Use models to help.

How many unit fractions did you use in all to rewrite the fractions above? How does this relate to the sum \(\frac{3}{6}+\frac{5}{6}\) ?

Answer:

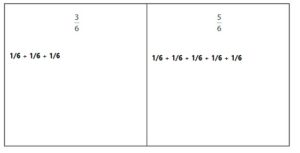
\(\frac{3}{6}+\frac{5}{6}\) = \(\frac{8}{6}\)

Construct Arguments
How can you use the numerators and the denominators to add fractions with like denominators? Explain why your method makes sense.

Answer:
To add fractions with like denominators, add the numerators and keep the same denominator. Then simplify the sum. You know how to do this with numeric fractions.
\(\frac{3}{6}+\frac{5}{6}\) = \(\frac{8}{6}\)

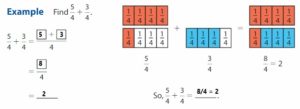
Think and Grow: Add Fractions

To add fractions with like denominators, add the numerators.

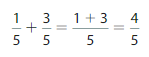
The denominator stays the same.

Answer:

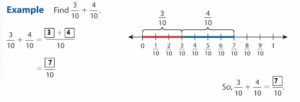
Add the numerators of the like denominators.

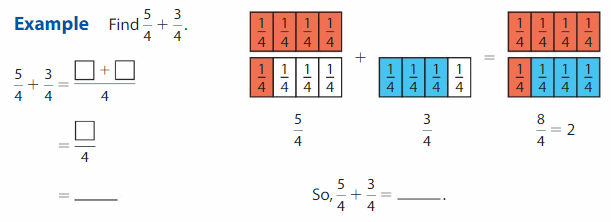
Answer:
Add the numerators of the like denominators.

Show and Grow

Add.

Question 1.

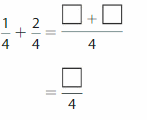
Answer:
Add the numerators of the like denominators.

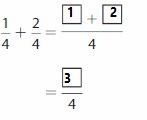
Question 2.

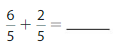
Answer:
Add the numerators of the like denominators.
6 + 2 = 8
\(\frac{6}{5}+\frac{2}{5}\) = \(\frac{8}{5}\)

Question 3.

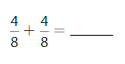
Answer:
Add the numerators of the like denominators.
4 + 4 = 8
\(\frac{4}{8}+\frac{4}{8}\) = \(\frac{8}{8\) = 1

Apply and Grow: Practice

Add.

Question 4.

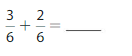
Answer:
Add the numerators of the like denominators.
3 + 2 = 5
\(\frac{3}{6}+\frac{2}{6}\) = \(\frac{5}{6}\)

Question 5.

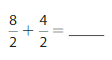
Answer:
Add the numerators of the like denominators.
8 + 4 = 12
\(\frac{8}{2}+\frac{4}{2}\) = \(\frac{12}{2}\) = 6

Question 6.

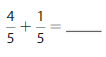
Answer:
Add the numerators of the like denominators.
4 + 1 = 5
\(\frac{4}{5}+\frac{1}{5}\) = \(\frac{5}{5}\) = 1

Question 7.

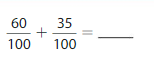
Answer:
Add the numerators of the like denominators.
60 + 35 = 95
\(\frac{60}{100}+\frac{35}{100}\) = \(\frac{95}{100}\)

Question 8.

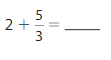
Answer:
The denominators are not the same. So first you have to make the common denominators and add the fraction with the number.
2 × 3/3 = 6/3
\(\frac{6}{3}+\frac{5}{3}\) = \(\frac{11}{3}\)

Question 9.

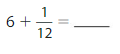
Answer:
The denominators are not the same. So first you have to make the common denominators and add the fraction with the number.
6 × 12/12 = 72/12
\(\frac{72}{12}+\frac{1}{12}\) = \(\frac{73}{12}\)

Question 10.

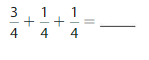
Answer:
Add the numerators of the like denominators.
3 + 1 + 1 = 5
3/4 + 1/4 + 1/4 = 5/4

Question 11.

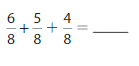
Answer:
Add the numerators of the like denominators.
\(\frac{6}{8}\) + \(\frac{5}{8}\) + \(\frac{4}{8}\)
6 + 5 + 4 = 15
\(\frac{6}{8}\) + \(\frac{5}{8}\) + \(\frac{4}{8}\) = \(\frac{15}{8}\)

Question 12.

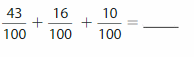
Answer:
Add the numerators of the like denominators.
43 + 16 + 10 = 69
\(\frac{43}{100}\) + \(\frac{16}{100}\) + \(\frac{10}{100}\) = \(\frac{69}{100}\)

Question 13.
You eat \(\frac{2}{10}\) of a vegetable pizza. Your friend eats \(\frac{3}{10}\) of the pizza. What fraction of the pizza do you and your friend eat together?

Answer:
Given that,
You eat \(\frac{2}{10}\) of a vegetable pizza. Your friend eats \(\frac{3}{10}\) of the pizza.
\(\frac{2}{10}\) + \(\frac{3}{10}\) = \(\frac{5}{10}\) = \(\frac{1}{2}\)
\(\frac{1}{2}\) fraction of the pizza do you and your friend eat together

Question 14.
Number Sense
A sum has 5 addends. Each addend is a unit fraction. The sum is 1. What are the addends?

Answer:
\(\frac{1}{5}\) + \(\frac{1}{5}\) + \(\frac{1}{5}\) + \(\frac{1}{5}\) + \(\frac{1}{5}\) = \(\frac{5}{5}\) = 1

Question 15.
Writing
Explain how to add \(\frac{3}{4}\) and \(\frac{1}{4}\). Use a model to support your answer.

Answer:

\(\frac{3}{4}\) + \(\frac{1}{4}\) = \(\frac{4}{4}\) = 1

Think and Grow: Modeling Real Life

Example
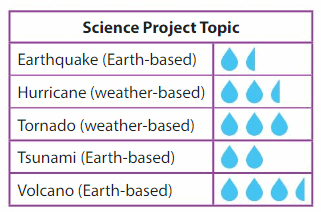
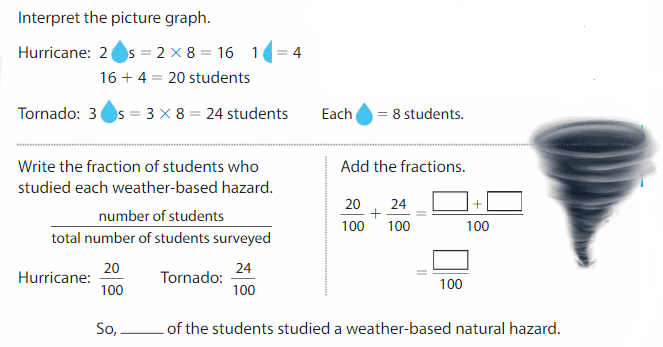
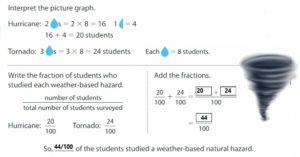
The table shows the natural hazards studied by 100 students for a science project. What fraction of the students studied a weather-based natural hazard?

Answer:

Show and Grow

Question 16.
Use the graph above to find what fraction of the students studied an Earth-based natural hazard.

Answer:
We can find the fraction of the students who studied an Earth-based natural hazard
1 × 8 = 8
Half drop = 4
8 + 4 = 12
=  Number of students/Total number of students surveyed
= 12/100
Thus \(\frac{12}{100}\) fraction of the students who studied an Earth-based natural hazard.

Question 17.
DIG DEEPER!
A caterer needs at least 2 pounds of lunch meat to make a sandwich platter. She has \(\frac{6}{4}\) pounds of turkey and \(\frac{3}{4}\) pound of ham. Does the caterer have enough lunch meat to make a sandwich platter? Explain.

Answer:
Given that,
A caterer needs at least 2 pounds of lunch meat to make a sandwich platter. She has \(\frac{6}{4}\) pounds of turkey and \(\frac{3}{4}\) pound of ham.
\(\frac{6}{4}\) + \(\frac{3}{4}\) = \(\frac{9}{4}\)
Convert it into mixed fraction
\(\frac{9}{4}\) = 1 \(\frac{3}{4}\)
Thus the caterer does not have enough lunch meat to make a sandwich platter.

Add Fractions with Like Denominators Homework & Practice 8.3

Add

Question 1.

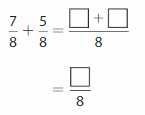
Answer:
Add the numerators of the like denominators.
Take the denominator as common and add the numerators.

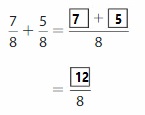
Question 2.

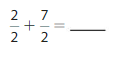
Answer:
Add the numerators of the like denominators.
Take the denominator as common and add the numerators.
\(\frac{2}{2}\) + \(\frac{7}{2}\) = (2 + 7)/2 = \(\frac{9}{2}\)

Question 3.

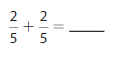
Answer:
Add the numerators of the like denominators.
Take the denominator as common and add the numerators.
\(\frac{2}{5}\) + \(\frac{2}{5}\) = (2 + 2)/5 = \(\frac{4}{5}\)

Question 4.

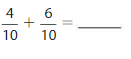
Answer:
Add the numerators of the like denominators.
Take the denominator as common and add the numerators.
\(\frac{4}{10}\) + \(\frac{6}{10}\) = (4 + 6)/10 = \(\frac{10}{10}\) = 1

Question 5.

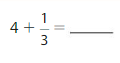
Answer:
The denominators are not the same. So first you have to make the common denominators and add the fraction with the number.
Take the denominator as common and add the numerators.
4 × 3/3 = 12/3
\(\frac{12}{3}\) + \(\frac{1}{3}\) = (12 + 1)/3 = \(\frac{13}{2}\)

Question 6.

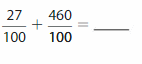
Answer:
Add the numerators of the like denominators.
Take the denominator as common and add the numerators.
\(\frac{27}{100}\) + \(\frac{460}{100}\) = (27 + 460)/100 = \(\frac{487}{100}\)

Question 7.

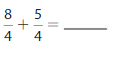
Answer:
Add the numerators of the like denominators.
Take the denominator as common and add the numerators.
\(\frac{8}{4}\) + \(\frac{5}{4}\) = (8 + 5)/4 = \(\frac{13}{4}\)

Question 8.

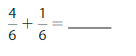
Answer:
Add the numerators of the like denominators.
Take the denominator as common and add the numerators.
\(\frac{4}{6}\) + \(\frac{1}{6}\) = (4 + 1)/6 = \(\frac{5}{6}\)

Question 9.

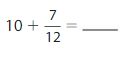
Answer:
The denominators are not the same. So first you have to make the common denominators and add the fraction with the number.
Take the denominator as common and add the numerators.
10 × 12/12 = 120/12
\(\frac{120}{12}\) + \(\frac{7}{12}\) = (120 + 7)/3 = \(\frac{127}{12}\)

Question 10.

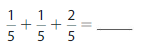
Answer:
Add the numerators of the like denominators.
Take the denominator as common and add the numerators.
\(\frac{1}{5}\) + \(\frac{1}{5}\) + \(\frac{2}{5}\) = (1 + 1 + 2)/5 = \(\frac{4}{5}\)

Question 11.

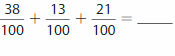
Answer:
Add the numerators of the like denominators.
Take the denominator as common and add the numerators.
\(\frac{38}{100}\) + \(\frac{13}{100}\) + \(\frac{21}{100}\) = (38+ 13 + 21)/100 = \(\frac{72}{100}\)

Question 12.

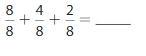
Answer:
Add the numerators of the like denominators.
Take the denominator as common and add the numerators.
\(\frac{8}{8}\) + \(\frac{4}{8}\) + \(\frac{2}{8}\) = (8 + 4 + 2)/8 = \(\frac{14 }{8}\)

Question 13.
You plant a sunflower seed. After 11 week, the plant is \(\frac{1}{2}\) inch tall. The next week your plant grows \(\frac{3}{2}\) inches. How tall is your plant after the second week?

Answer:
Given that,
You plant a sunflower seed. After 11 week, the plant is \(\frac{1}{2}\) inch tall. The next week your plant grows \(\frac{3}{2}\) inches.
\(\frac{1}{2}\) + \(\frac{3}{2}\) = \(\frac{4}{2}\) = 2
The plant is 2 inches tall after the second week.

Question 14.
Writing
Explain how to find the unknown addend.

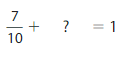
Answer:
1 can be written as \(\frac{10}{10}\)
\(\frac{7}{10}\) + ? = \(\frac{10}{10}\)
? = \(\frac{10}{10}\) – \(\frac{7}{10}\)
? = \(\frac{3}{10}\)
Thus the unknown addend is \(\frac{3}{10}\)

Question 15.
DIG DEEPER!
When you double me and add \(\frac{1}{6}\), you get \(\frac{5}{6}\). What fraction am I?

Answer: \(\frac{2}{6}\)

Explanation:
If you add \(\frac{2}{6}\) twice and add \(\frac{1}{6}\) to it you get \(\frac{5}{6}\).

Question 16.
Reasoning
You eat \(\frac{2}{8}\) of a large apple at lunch and another \(\frac{4}{8}\) of it as a snack. Your friend eats \(\frac{4}{8}\) of a small apple at lunch and another \(\frac{2}{8}\) of it as a snack. Do you each eat the same amount? Explain.

Answer:
Given that,
You eat \(\frac{2}{8}\) of a large apple at lunch and another \(\frac{4}{8}\) of it as a snack. Your friend eats \(\frac{4}{8}\) of a small apple at lunch and another \(\frac{2}{8}\) of it as a snack.
\(\frac{2}{8}\) + \(\frac{4}{8}\) = \(\frac{6}{8}\)
\(\frac{2}{8}\) + \(\frac{4}{8}\) = \(\frac{6}{8}\)
Yes you and your friend eat same amount of food.

Question 17.
Modeling Real Life
The graph shows the classification of 100 species of birds in North America according to their extinction rate. What fraction of the species are classified as near threatened or vulnerable?

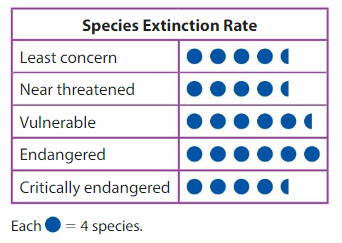
Answer:
• = 4 species
Near threatened = 4 × 4 = 16 species
half • = 2 species
16 + 2 = 18 species
Fraction of near threatened = number of species/total number of species of birds in North America
= 18/100
Vulnerable = 5 × 4 = 20 species
half • = 2 species
20 + 2 = 22 species
Fraction of near threatened = number of species/total number of species of birds in North America
= 22/100

Question 18.
Modeling Real Life
Use the graph above to find what fraction of not the species are critically endangered.

Answer:
• = 4 species
critically endangered = 4 × 4 = 16 species
half • = 2 species
16 + 2 = 18 species
Fraction of near threatened = number of species/total number of species of birds in North America
= 18/100

Review & Refresh

Question 19.
A pet store has 25 tanks with 32 fish in each tank. A customer buys 7 fish. How many fish does the pet store have now?

Answer:
Given,
A pet store has 25 tanks with 32 fish in each tank. A customer buys 7 fish.
\(\frac{25}{32}\) – \(\frac{7}{32}\) = \(\frac{18}{32}\)
Thus there are 18 fishes in the pet store.

Lesson 8.4 Use Models to subtract Fractions

Explore and Grow

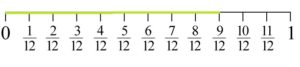
Draw a model to show \(\frac{9}{12}\).
Answer:

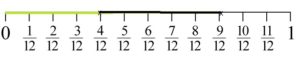
Use your model to find \(\frac{9}{12}\) – \(\frac{5}{12}\). Explain your method.
Answer:

Repeated Reasoning
Write two fractions that have a difference of \(\frac{7}{12}\). Explain your reasoning.

Answer:  \(\frac{9}{12}\) – \(\frac{2}{12}\) = \(\frac{7}{12}\)

Think and Grow: Use Models to Subtract Fractions

You can subtract fractions by taking away parts that refer to the same whole.

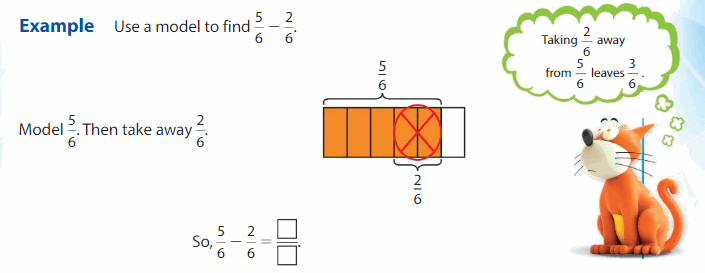
Answer:

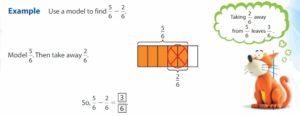
Answer:

Show and Grow

Find the difference. Explain how you used the model to subtract.

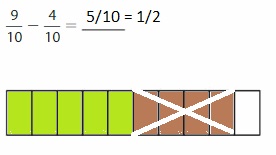
Question 1.

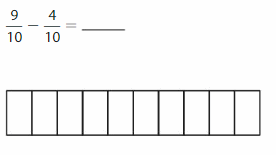
Answer: 5/10 = 1/2
Take away a length of 4/10 from the length of 9/10.

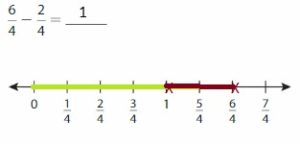
Question 2.

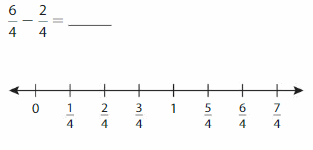
Answer:
Take away a length of 6/4 from the length of 2/4.

Apply and Grow: Practice

Find the difference. Use a model or a number line to help.

Question 3.

Answer: 4/8
Take away a length of 8/8 from the length of 4/8.

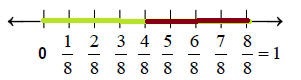
Question 4.

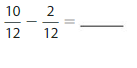
Answer: 8/12
Take away a length of 10/12 from the length of 2/12.

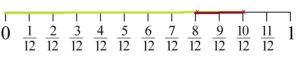
Question 5.

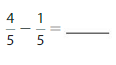
Answer: 3/5
Take away a length of 4/5 from the length of 1/5.

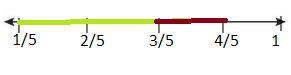
Question 6.

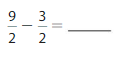
Answer: 6/2 = 3
Take away a length of 9/2 from the length of 3/2.

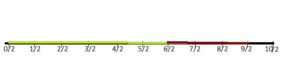
Question 7.

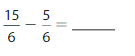
Answer: 10/6
Take away a length of 15/6 from the length of 5/6.

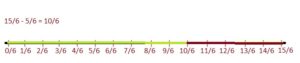
Question 8.

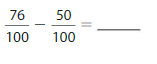
Answer: \(\frac{26}{100}\)
The denominators of both the fractions are the same. So subtract the numerators.
\(\frac{76}{100}\) – \(\frac{50}{100}\) = \(\frac{26}{100}\)

Question 9.
You need to walk \(\frac{3}{4}\) mile for your physical education class. So far, you have walked \(\frac{2}{4}\) mile. How much farther do you need to walk?

Answer:
Given that,
You need to walk \(\frac{3}{4}\) mile for your physical education class. So far, you have walked \(\frac{2}{4}\) mile.
\(\frac{3}{4}\) – \(\frac{2}{4}\) = \(\frac{1}{4}\)
You need to walk \(\frac{1}{4}\) miles more.

Question 10.
Number Sense
Which expressions have a difference of \(\frac{4}{5}\) ?

Answer:
5/5 – 1/5 = 4/5
10/5 – 6/5 = 4/5
6/5 – 3/5 = 3/5
9/5 – 5/5 = 4/5
i, ii, iv has the difference of \(\frac{4}{5}\)

Question 11.
Structure
Write the subtraction equation represented by the model.

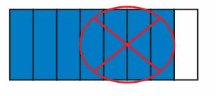
Answer: \(\frac{7}{8}\) – \(\frac{4}{8}\) = \(\frac{3}{8}\)

Question 12.
Writing
Explain why the numerator changes when you subtract fractions with like denominators, but the denominator stays the same.

Answer:
The most simple fraction subtraction problems are those that have two proper fractions with a common denominator. That is, each denominator is the same. The process is just as it is for the addition of fractions with like denominators, except you subtract! You subtract the second numerator from the first and keep the denominator the same.

Think and Grow: Modeling Real Life

Example
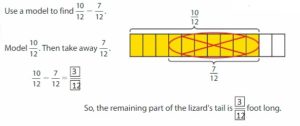
A lizard’s tail is \(\frac{10}{12}\) foot long. It sheds a \(\frac{7}{12}\) foot long part of its tail to escape a predator. How long is the remaining part of the lizard’s tail?

Because each fraction represents a part of the same whole, you can take away a part.

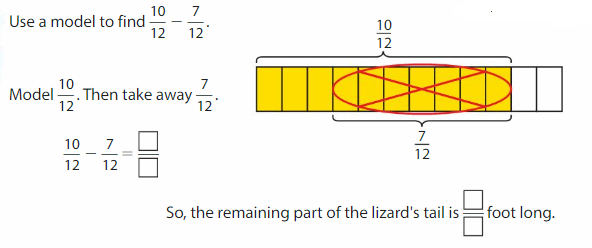
Answer:
Given that,
A lizard’s tail is \(\frac{10}{12}\) foot long. It sheds a \(\frac{7}{12}\) foot long part of its tail to escape a predator.

Show and Grow

Question 13.
You have \(\frac{9}{8}\) cups of raisins. You eat \(\frac{2}{8}\) cup. What fraction of a cup of raisins do you have left?

Answer:
Given that,
You have \(\frac{9}{8}\) cups of raisins. You eat \(\frac{2}{8}\) cup.
\(\frac{9}{8}\) – \(\frac{2}{8}\) = \(\frac{7}{8}\)
Thus \(\frac{7}{8}\) fraction of a cup of raisins is left.

Question 14.
A large bottle has \(\frac{7}{4}\) quarts of liquid soap. A small bottle has \(\frac{3}{4}\) quart of liquid soap. How much more soap is in the large bottle than in the small bottle?

Answer:
Given that,
A large bottle has \(\frac{7}{4}\) quarts of liquid soap. A small bottle has \(\frac{3}{4}\) quart of liquid soap.
\(\frac{7}{4}\) – \(\frac{3}{4}\) = \(\frac{4}{4}\) = 1
Thus 1 more soap is in the large bottle than in the small bottle.

Question 15.
DIG DEEPER!
You need 2 cups of milk for a recipe. You have cup of \(\frac{1}{3}\) milk. How much more milk do you need? Explain.

Answer:
Given,
You need 2 cups of milk for a recipe. You have cup of \(\frac{1}{3}\) milk.
2 × \(\frac{1}{3}\) = \(\frac{2}{3}\)
Thus \(\frac{2}{3}\) more milk you need.

Use Models to subtract Fractions Homework & Practice 8.4

Find the difference. Explain how you used the model to subtract.

Question 1.

Answer:

Question 2.

Answer:

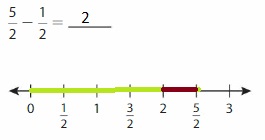
Find the difference. Use a model or a number line to help.

Question 3.

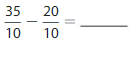
Answer: 15/10

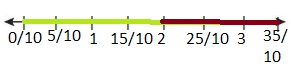
Question 4.

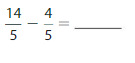
Answer: 10/5

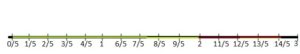
Question 5.

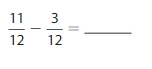
Answer: 8/12

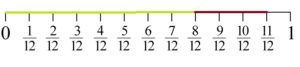
Question 6.

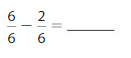
Answer:

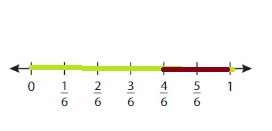
Question 7.

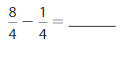
Answer: 7/4

Question 8.

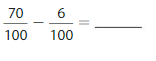
Answer:
The denominators of both the fractions are the same. So subtract the numerators.
\(\frac{70}{100}\) – \(\frac{6}{100}\) = \(\frac{64}{100}\)

Question 9.
You have \(\frac{2}{3}\) yard of ribbon. You cut off \(\frac{1}{3}\) yard of the ribbon. How much ribbon do you have left?

Answer:
Given that,
You have \(\frac{2}{3}\) yard of ribbon. You cut off \(\frac{1}{3}\) yard of the ribbon.
The denominators of both the fractions are the same. So subtract the numerators.
\(\frac{2}{3}\) – \(\frac{1}{3}\) = \(\frac{1}{3}\)
\(\frac{1}{3}\) ribbon has left.

Question 10.
Structure
When using circular models to find the difference of \(\frac{4}{2}\) and \(\frac{1}{2}\), why do you shade two circles to represent \(\frac{4}{2}\)?

Answer:
The denominators of both the fractions are the same. So subtract the numerators.
\(\frac{4}{2}\) – \(\frac{1}{2}\) = \(\frac{3}{2}\)

Question 11.
YOU BE THE TEACHER
In a box of pens, \(\frac{3}{4}\) of the pens are blue. Your friend takes \(\frac{1}{4}\) of the blue pens and says that now \(\frac{2}{4}\) of the pens in the box are blue. Is your friend correct? Explain.

Answer:
Given,
In a box of pens, \(\frac{3}{4}\) of the pens are blue. Your friend takes \(\frac{1}{4}\) of the blue pens and says that now \(\frac{2}{4}\) of the pens in the box are blue.
The denominators of both the fractions are the same. So subtract the numerators.
\(\frac{3}{4}\) – \(\frac{1}{4}\) = \(\frac{2}{4}\)
Yes, your friend is correct.

Question 12.
DIG DEEPER!
Using numerators that even number, write two different subtraction equations that each have a difference of 1.

Answer: \(\frac{6}{4}\) – \(\frac{2}{4}\) = \(\frac{4}{4}\) = 1

Question 13.
Modeling Real Life
In our solar system, \(\frac{6}{8}\) of the planets have moons, and \(\frac{4}{8}\) of the planets have moons and rings. What fraction of the planets in our solar system have moons, but do not have rings?

Answer:
Given,
In our solar system, \(\frac{6}{8}\) of the planets have moons, and \(\frac{4}{8}\) of the planets have moons and rings.
The denominators of both the fractions are the same. So subtract the numerators.
\(\frac{6}{8}\) – \(\frac{4}{8}\) = \(\frac{2}{8}\)

Question 14.
Modeling Real Life
A professional pumpkin carver carves a pumpkin that weighs \(\frac{7}{10}\) ton. He carves a second pumpkin that weighs \(\frac{6}{10}\) ton. How much heavier is the first pumpkin than the second pumpkin?

Answer:
Given that,
A professional pumpkin carver carves a pumpkin that weighs \(\frac{7}{10}\) ton. He carves a second pumpkin that weighs \(\frac{6}{10}\) ton.
The denominators of both the fractions are the same. So subtract the numerators.
\(\frac{7}{10}\) – \(\frac{6}{10}\) = \(\frac{1}{10}\)
The first pumpkin is \(\frac{1}{10}\) heavier than the second pumpkin.

Review & Refresh

Find an equivalent fraction.

Question 15.
\(\frac{7}{4}\)

Answer:
The equivalent fraction of \(\frac{7}{4}\) is given below,
\(\frac{7}{4}\) × \(\frac{2}{2}\) = \(\frac{14}{8}\)

Question 16.
\(\frac{3}{5}\)

Answer:
The equivalent fraction of \(\frac{3}{5}\) is given below,
\(\frac{3}{5}\) × \(\frac{3}{3}\) = \(\frac{9}{15}\)

Question 17.
\(\frac{2}{3}\)

Answer:
The equivalent fraction of \(\frac{2}{3}\) is given below,
\(\frac{2}{3}\) × \(\frac{2}{2}\) = \(\frac{4}{6}\)

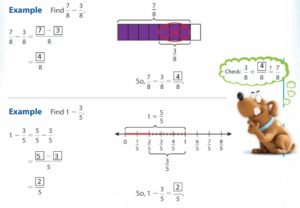
Lesson 8.5 Subtract Fractions with Like Denominators

Explore and Grow

Write each fraction as a sum of unit fractions. Use models to help.

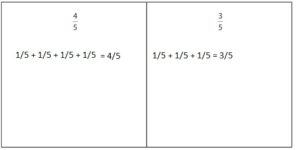
How many more unit fractions did you use to rewrite \(\frac{4}{5}\) than \(\frac{3}{5}\)?
How does this relate to the difference \(\frac{4}{5}\) – \(\frac{3}{5}\) ?

Answer:

\(\frac{4}{5}\) – \(\frac{3}{5}\) = \(\frac{1}{5}\)

Construct Arguments
How can you use the numerators and the denominators to subtract fractions with like denominators? Explain why your method makes sense.

Answer: Steps on How to Add and Subtract Fractions with the Same Denominator. To add fractions with like or the same denominator, simply add the numerators then copy the common denominator. Always reduce your final answer to its lowest term.

Think and Grow: Subtract Fractions

To subtract fractions with like denominators, subtract the numerators. The denominator stays the same.

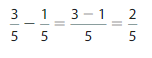
Answer:

Show and Grow

Subtract.

Question 1.

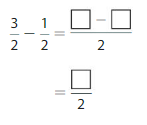
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.

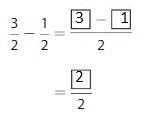
Question 2.

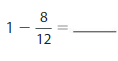
Answer:
First, make the denominators common and then subtract the numerators
1 can be written as \(\frac{12}{12}\)
\(\frac{12}{12}\) – \(\frac{8}{12}\) = (12 – 8)/12
= \(\frac{4}{12}\) or \(\frac{1}{3}\)

Question 3.

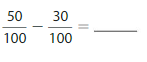
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{50}{100}\) – \(\frac{30}{100}\) = (50 – 30)/100
= \(\frac{20}{100}\) or \(\frac{1}{5}\)

Apply and Grow: Practice

Subtract.

Question 4.

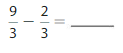
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.

Question 5.

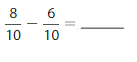
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.

Question 6.

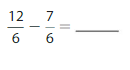
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{12}{6}\) – \(\frac{7}{6}\) = (12- 7)/6
\(\frac{5}{6}\)

Question 7.

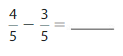
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{4}{5}\) – \(\frac{3}{5}\) = (4- 3)/5
\(\frac{1}{5}\)

Question 8.

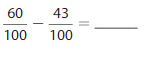
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{60}{100}\) – \(\frac{43}{100}\) = (60 – 43)/100
\(\frac{17}{100}\)

Question 9.

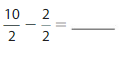
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{10}{2}\) – \(\frac{2}{2}\) = (10 – 2)/2
\(\frac{8}{4}\) = 2

Question 10.

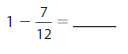
Answer:
First, make the denominators common and then subtract the numerators.
\(\frac{12}{12}\) – \(\frac{7}{12}\) = (12 – 7)/12
= \(\frac{5}{12}\)

Question 11.

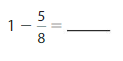
Answer:
First, make the denominators common and then subtract the numerators
1 can be written as \(\frac{8}{8}\)
\(\frac{8}{8}\) – \(\frac{5}{8}\) = \(\frac{3}{8}\)

Question 12.

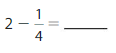
Answer:
First, make the denominators common and then subtract the numerators
2 can be written as \(\frac{8}{4}\)
\(\frac{8}{4}\) – \(\frac{1}{4}\) = \(\frac{7}{4}\)

Question 13.
You have 1 gallon of paint. You use \(\frac{2}{3}\) gallon to paint a wall. How much paint do you have left?

Answer:
Given that,
You have 1 gallon of paint. You use \(\frac{2}{3}\) gallon to paint a wall.
First, make the denominators common and then subtract the numerators
1 – \(\frac{2}{3}\)
1 can be written as \(\frac{3}{3}\)
\(\frac{3}{3}\) – \(\frac{2}{3}\) = \(\frac{1}{3}\)

Question 14.
Reasoning
Why is it unreasonable to get a difference of \(\frac{7}{8}\) when subtracting \(\frac{1}{8}\) from \(\frac{7}{8}\)? Use a model to support your answer.

Answer:
The difference of \(\frac{7}{8}\) when subtracting \(\frac{1}{8}\) from \(\frac{7}{8}\) is,

Question 15.
Your friend says each difference is \(\frac{3}{10}\). Is your friend correct? Explain.

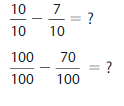
Answer:

Your friend is correct.
10/10 – 7/10 = 3/10
100/100 = 70/100 = 30/100 = 3/10

Think and Grow: Modeling Real Life

Example
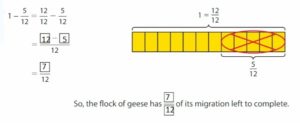
A flock of geese has completed \(\frac{5}{12}\) of its total migration. What fraction of its migration does the flock of geese have left to complete?

Because the total migration is 1 whole, find 1 − \(\frac{5}{12}\).

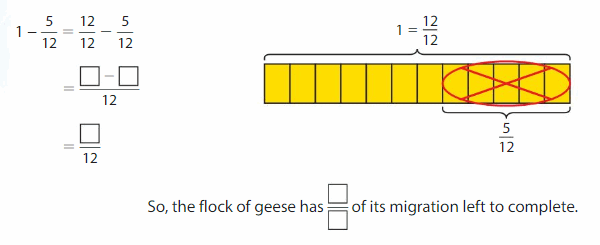
Answer:
Given,
A flock of geese has completed \(\frac{5}{12}\) of its total migration.
Because the total migration is 1 whole, find 1 − \(\frac{5}{12}\).
First, make the denominators common and then subtract the numerators.

Show and Grow

Question 16.
A runner has completed \(\frac{6}{10}\) of a race. What fraction of the race does the runner have left to complete?

Answer:
Given that,
A runner has completed \(\frac{6}{10}\) of a race.
1 – \(\frac{6}{10}\)
1 can be written as \(\frac{10}{10}\)
\(\frac{10}{10}\) – \(\frac{6}{10}\) = \(\frac{4}{10}\)
The runner has left \(\frac{4}{10}\) fraction of the race to complete.

Question 17.
A pizza buffet serves pizzas of the same size with different toppings. There is \(\frac{7}{8}\) of a vegetable pizza and \(\frac{2}{8}\) of a pineapple pizza left. How much more vegetable pizza is left than pineapple pizza?

Answer:
Given,
A pizza buffet serves pizzas of the same size with different toppings.
There is \(\frac{7}{8}\) of a vegetable pizza and \(\frac{2}{8}\) of a pineapple pizza left.
\(\frac{7}{8}\) – \(\frac{2}{8}\) = \(\frac{5}{8}\)
\(\frac{5}{8}\) more vegetable pizza is left than pineapple pizza.

Question 18.
DIG DEEPER!
Baseball practice is 1 hour long. You stretch for 7 minutes and play catch for 8 minutes. What fraction of an hour do you have left to practice?

Answer:
Given,
Baseball practice is 1 hour long. You stretch for 7 minutes and play catch for 8 minutes.
7 minutes + 8 minutes = 15 minutes
15 minutes = \(\frac{1}{4}\) hour
1 – \(\frac{1}{4}\) = \(\frac{3}{4}\)
Thus \(\frac{3}{4}\) fraction of an hour is left to practice.

Subtract Fractions with Like Denominators Homework & Practice 8.5

Subtract

Question 1.

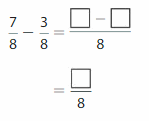
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{7}{8}\) – \(\frac{3}{8}\) = \(\frac{4}{8}\)

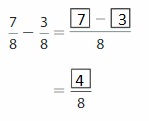
Question 2.

Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{5}{4}\) – \(\frac{3}{4}\) = \(\frac{2}{4}\)

Question 3.

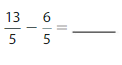
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{13}{5}\) – \(\frac{6}{5}\) = \(\frac{7}{6}\)

Question 4.

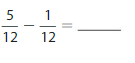
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{5}{12}\) – \(\frac{1}{12}\) = \(\frac{4}{12}\)

Question 5.

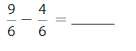
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{9}{6}\) – \(\frac{4}{6}\) = \(\frac{5}{6}\)

Question 6.

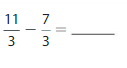
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{11}{3}\) – \(\frac{7}{3}\) = \(\frac{4}{3}\)

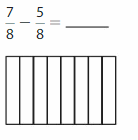
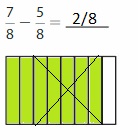
Question 7.

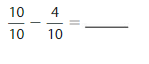
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{10}{10}\) – \(\frac{4}{10}\) = \(\frac{6}{10}\)

Question 8.

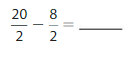
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{20}{2}\) – \(\frac{8}{2}\) = \(\frac{12}{2}\)

Question 9.

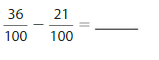
Answer:
The denominators of the above fraction are the same so you have to subtract the numerators.
\(\frac{36}{100}\) – \(\frac{21}{100}\) = \(\frac{15}{100}\)

Question 10.

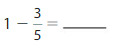
Answer:
First, make the denominators common and then subtract the numerators.
1 can be written as 5/5.
\(\frac{5}{5}\) – \(\frac{3}{5}\) = \(\frac{2}{5}\)

Question 11.

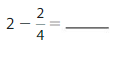
Answer:
First, make the denominators common and then subtract the numerators.
2 can be written as 8/4
\(\frac{8}{4}\) – \(\frac{2}{4}\) = \(\frac{6}{4}\)

Question 12.

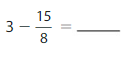
Answer:
First, make the denominators common and then subtract the numerators
3 can be written as 24/8.
\(\frac{24}{8}\) – \(\frac{15}{8}\) = \(\frac{9}{8}\)

Question 13.
A family eats \(\frac{2}{3}\) of a tray of lasagna. What fraction of the tray of lasagna is left?

Answer:
Given,
A family eats \(\frac{2}{3}\) of a tray of lasagna.
1 – \(\frac{2}{3}\)
1 can be written as \(\frac{3}{3}\)
\(\frac{3}{3}\) – \(\frac{2}{3}\) = \(\frac{1}{3}\)
Therefore \(\frac{1}{3}\) fraction of the tray of lasagna is left.

Question 14.
Writing
Explain how finding is \(\frac{7}{10}-\frac{4}{10}\) similar to finding 7 – 4.

Answer:
Yes \(\frac{7}{10}-\frac{4}{10}\) similar to finding 7 – 4. Because the denominators of the fractions are the same.
\(\frac{7}{10}-\frac{4}{10}\) = \(\frac{3}{10}\)

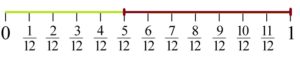
Question 15.
Open-Ended
The model shows equal parts of a 1 whole. Write a subtraction problem whose answer is shown.

Answer:
By seeing the above figure we can write the subtraction problem.
1 – \(\frac{3}{8}\)

Question 16.
Modeling Real Life
you fill \(\frac{2}{4}\) of your plate with vegetables. What fraction of your plate does not contain vegetables?

Answer:
Given,
you fill \(\frac{2}{4}\) of your plate with vegetables.
1 – \(\frac{2}{4}\)
1 can be written as \(\frac{4}{4}\)
\(\frac{4}{4}\) – \(\frac{2}{4}\) = \(\frac{2}{4}\)
Thus \(\frac{2}{4}\) fraction of your plate does not contain vegetables.

Question 17.
Modeling Real Life
A group of students designs a rectangular playground. They use \(\frac{2}{8}\) of the playground for a basketball court and \(\frac{3}{8}\) of the playground for a soccer field. How much space is left?

Answer:
Given,
A group of students designs a rectangular playground.
They use \(\frac{2}{8}\) of the playground for a basketball court and \(\frac{3}{8}\) of the playground for a soccer field.
\(\frac{2}{8}\) + \(\frac{3}{8}\) = \(\frac{5}{8}\)
1 – \(\frac{5}{8}\) = \(\frac{3}{8}\)
Thus \(\frac{3}{8}\) space is left.

Review & Refresh

Find the quotient and the remainder

Question 18.
34 ÷ 7 = ___R___

Answer: 4R6

Explanation:
34 ÷ 7 = \(\frac{34}{7}\)
\(\frac{34}{7}\) = 4R6
Thus the quotient is 4 and the remainder is 6.

Question 19.
28 ÷ 3 = ___R___

Answer: 9 R1

Explanation:
28 ÷ 3 = \(\frac{28}{3}\)
\(\frac{28}{3}\) = 9 R1
Thus the quotient is 9 and the remainder is 1.

Lesson 8.6 Model Fractions and Mixed Numbers

Explore and Grow

Draw a model to show 1 + 1 + \(\frac{2}{3}\).

Use your model to write the sum as a fraction.

Answer:

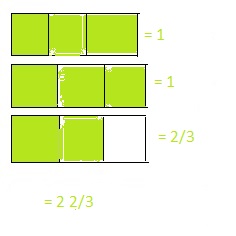
Repeated Reasoning
How can you write a fraction greater than 1 as the sum of a whole number and a fraction less than 1? Explain.

Answer:
\(\frac{3}{2}\)  = 1 \(\frac{1}{2}\)
The fraction 1 \(\frac{1}{2}\) the whole fraction is greater than 1 and the fraction is less than 1.

Think and Grow: Write Fractions and Mixed Numbers

A mixed number represents the sum of a whole number and a fraction less than 1.

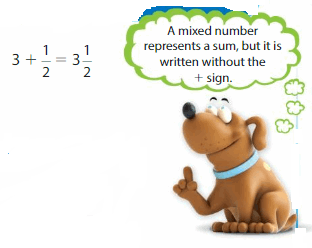
Answer:

Example
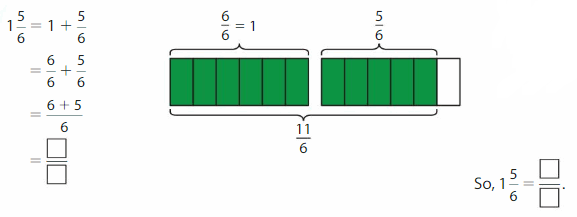
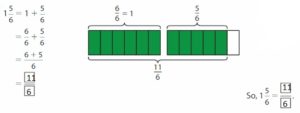
Write \(\frac{5}{2}\) as a mixed number.

Find how many wholes are in \(\frac{5}{2}\) and how many halves are left over.

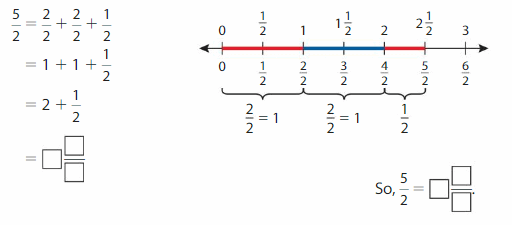
Answer:

Show and Grow

Question 1.
Write 3\(\frac{1}{4}\) as a fraction. Use a model or a number line to help.

Answer:

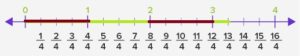
Question 2.
Write \(\frac{9}{6}\) as a mixed number. Use a model or a number line to help.

Answer:
\(\frac{9}{6}\) can be written as \(\frac{3}{2}\)
Now convert \(\frac{3}{2}\) into the mixed fraction
\(\frac{3}{2}\) = 1 \(\frac{1}{2}\)

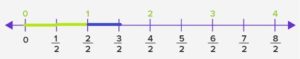
Apply and Grow: Practice

Write the mixed number as a fraction.

Question 3.
3\(\frac{4}{5}\)

Answer: \(\frac{19}{5}\)

Explanation:
Step 1
Multiply the denominator by the whole number
5 × 3 = 15
Step 2
Add the answer from Step 1 to the numerator
15 + 4 = 19
Step 3
Write an answer from Step 2 over the denominator
19/5

Question 4.
2\(\frac{1}{3}\)

Answer: \(\frac{7}{3}\)

Explanation:
Step 1
Multiply the denominator by the whole number
3 × 2 = 6
Step 2
Add the answer from Step 1 to the numerator
6 + 1 = 7
Step 3
Write an answer from Step 2 over the denominator
7/3

Question 5.
6\(\frac{7}{12}\)

Answer: \(\frac{79}{12}\)

Explanation:
Step 1
Multiply the denominator by the whole number
12 × 6 = 72
Step 2
Add the answer from Step 1 to the numerator
72 + 7 = 79
Step 3
Write an answer from Step 2 over the denominator
79/12

Question 6.
1\(\frac{82}{100}\)

Answer: \(\frac{182}{100}\)

Explanation:
Step 1
Multiply the denominator by the whole number
100 × 1 = 100
Step 2
Add the answer from Step 1 to the numerator
100 + 82 = 182
Step 3
Write an answer from Step 2 over the denominator
\(\frac{182}{100}\)

Question 7.
11\(\frac{3}{8}\)

Answer: \(\frac{91}{8}\)

Explanation:
Step 1
Multiply the denominator by the whole number
8 × 11 = 88
Step 2
Add the answer from Step 1 to the numerator
88 + 3 = 91
Step 3
Write an answer from Step 2 over the denominator
91/8

Question 8.
9\(\frac{5}{10}\)

Answer: \(\frac{95}{10}\)

Explanation:
Step 1
Multiply the denominator by the whole number
10 × 9 = 90
Step 2
Add the answer from Step 1 to the numerator
90 + 5 = 95
Step 3
Write an answer from Step 2 over the denominator
95/10

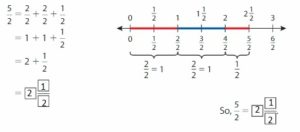
Write the fraction as a mixed number or a whole number.

Question 9.
\(\frac{9}{8}\)

Answer: 1 \(\frac{1}{8}\)

Explanation:
9÷8=1R1
\(\frac{9}{8}\) = 1 \(\frac{1}{8}\)

Question 10.
\(\frac{19}{3}\)

Answer: 6 \(\frac{1}{3}\)

Explanation:
19÷3=6R1
\(\frac{19}{3}\) = 6 \(\frac{1}{3}\)

Question 11.
\(\frac{38}{5}\)

Answer: 7 \(\frac{3}{5}\)

Explanation:
38÷5=7R3
\(\frac{38}{5}\) = 7 \(\frac{3}{5}\)

Question 12.
\(\frac{22}{10}\)

Answer: 2 \(\frac{1}{5}\)

Explanation:
11÷5=2R1
\(\frac{22}{10}\) = 2 \(\frac{1}{5}\)

Question 13.
\(\frac{460}{100}\)

Answer: 4 \(\frac{3}{5}\)

Explanation:
23÷5=4R3
\(\frac{460}{100}\) = 4 \(\frac{3}{5}\)

Question 14.
\(\frac{20}{4}\)

Answer: 5

Explanation:
4 divides 20 five times.
\(\frac{20}{4}\) = 5

Compare

Question 15.

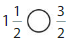
Answer: =

Explanation:
\(\frac{3}{2}\) can be written as 1 \(\frac{1}{2}\)
1 × 2 + 1 = 3
So, 1 \(\frac{1}{2}\) = \(\frac{3}{2}\)

Question 16.

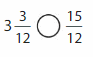
Answer: >

Explanation:
3\(\frac{3}{12}\) can be written as \(\frac{39}{12}\)
\(\frac{39}{12}\) > \(\frac{15}{12}\)
So, 3\(\frac{3}{12}\) > \(\frac{15}{12}\)

Question 17.

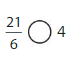
Answer: <

Explanation:
\(\frac{21}{6}\) can be written as 3 \(\frac{3}{6}\) or 3 \(\frac{1}{2}\)
So, 3 \(\frac{1}{2}\) < 4
\(\frac{21}{6}\) < 4

Question 18.
Which One Doesn’t Belong? Which expression does not Belong to the other three?

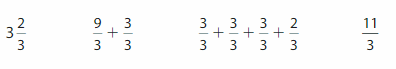
Answer:
3 \(\frac{2}{3}\) = \(\frac{11}{3}\)
\(\frac{9}{3}\) + \(\frac{3}{3}\) = \(\frac{12}{3}\)
\(\frac{3}{3}\) + \(\frac{3}{3}\) +\(\frac{3}{3}\) + \(\frac{2}{3}\) = \(\frac{11}{3}\)
\(\frac{11}{3}\)
So, the second expression does not belong to the other three expressions.

DIG DEEPER!
Find the unknown Number

Question 19.

Answer: 2

Explanation:
\(\frac{8}{6}\) is 4÷3=1R1
\(\frac{8}{6}\) = 1 \(\frac{2}{6}\)
So, the unknown number is 2.

Question 20.

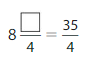
Answer: 3

Explanation:
\(\frac{35}{4}\) = 8 R 3
8 \(\frac{3}{4}\) = \(\frac{35}{4}\)
So, the unknown number is 3.

Question 21.

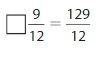
Answer: 10

Explanation:
12 × 10 + 9 = 129
\(\frac{129}{12}\) = 10 \(\frac{9}{12}\)
So, the unknown number is 10.

Think and Grow: Modeling Real Life

Example
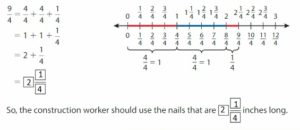
A construction worker needs nails that are \(\frac{9}{4}\) inches long. Which size of nails should the worker use?

Write \(\frac{9}{4}\) as a mixed number.

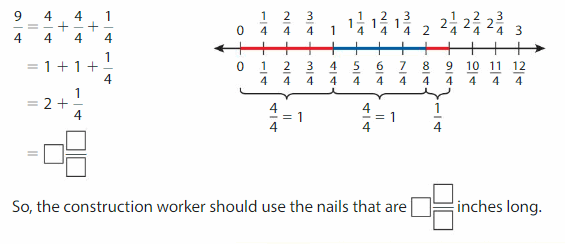
Answer:
Given,
A construction worker needs nails that are \(\frac{9}{4}\) inches long.
Convert from improper fraction to the mixed fraction.

Show and Grow

Question 22.
You need screws that are \(\frac{13}{8}\) inches long to build a birdhouse. Which size of screws should you use?

Answer:
Given,
You need screws that are \(\frac{13}{8}\) inches long to build a birdhouse.
Convert from improper fraction to the mixed fraction.
\(\frac{13}{8}\) = 1 \(\frac{5}{8}\)
8 × 1 + 5 = 13
So, you should use 1 \(\frac{5}{8}\) inches of screws.

Question 23.
You and your friend each measure the distance between two bean bag toss boards. You record the distance as 3\(\frac{3}{5}\) meters. Your friend records the distance as \(\frac{18}{5}\) meters. Did you and your friend record the same distance? Explain.

Answer:
Given that,
You and your friend each measure the distance between two bean bag toss boards.
You record the distance as 3\(\frac{3}{5}\) meters. Your friend records the distance as \(\frac{18}{5}\) meters.
3\(\frac{3}{5}\)
5 × 3 + 3 = 18
3\(\frac{3}{5}\) = \(\frac{18}{5}\)
Yes you and your friend record the same distance.

Question 24.
DIG DEEPER!
You use a \(\frac{1}{3}\)-cup scoop to measure 3\(\frac{1}{3}\) cups of rice. How many times do you fill the scoop?

Answer:
Given,
You use a \(\frac{1}{3}\)-cup scoop to measure 3\(\frac{1}{3}\) cups of rice.
\(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) = \(\frac{10}{3}\)
You need to measure 10 times to fill the scoop.

Question 25.
DIG DEEPER!
A sunflower plant is \(\frac{127}{10}\) centimeters tall. A snapdragon plant is 8\(\frac{9}{10}\) centimeters tall. Which plant is taller? Explain.

Answer:
Given that,
A sunflower plant is \(\frac{127}{10}\) centimeters tall. A snapdragon plant is 8\(\frac{9}{10}\) centimeters tall.
8\(\frac{9}{10}\) = \(\frac{89}{10}\)
\(\frac{127}{10}\) is greater than \(\frac{89}{10}\)
So, sunflower plant is taller.

Model Fractions and Mixed Numbers Homework & Practice 8.6

Write a mixed number as a fraction.

Question 1.
1\(\frac{7}{10}\)

Answer: \(\frac{17}{10}\)

Explanation:
Step 1
Multiply the denominator by the whole number
10 × 1 = 10
Step 2
Add the answer from Step 1 to the numerator
10 + 7 = 17
Step 3
Write an answer from Step 2 over the denominator
=17/10

Question 2.
1\(\frac{5}{6}\)

Answer: \(\frac{11}{6}\)

Explanation:
Step 1
Multiply the denominator by the whole number
6 × 1 = 6
Step 2
Add the answer from Step 1 to the numerator
6 + 5 = 11
Step 3
Write an answer from Step 2 over the denominator
11/6

Question 3.
2\(\frac{2}{3}\)

Answer: \(\frac{8}{3}\)

Explanation:
Step 1
Multiply the denominator by the whole number
3 × 2 = 6
Step 2
Add the answer from Step 1 to the numerator
6 + 2 = 8
Step 3
Write an answer from Step 2 over the denominator
8/3

Question 4.
4\(\frac{1}{2}\)

Answer: \(\frac{9}{2}\)

Explanation:
Step 1
Multiply the denominator by the whole number
2 × 4 = 8
Step 2
Add the answer from Step 1 to the numerator
8 + 1 = 9
Step 3
Write an answer from Step 2 over the denominator
9/2

Question 5.
3\(\frac{2}{8}\)

Answer: \(\frac{13}{4}\)

Explanation:
Step 1
Multiply the denominator by the whole number
8 × 3 = 24
Step 2
Add the answer from Step 1 to the numerator
24 + 2 = 26
Step 3
Write an answer from Step 2 over the denominator
26/8

Question 6.
9\(\frac{8}{12}\).

Answer: \(\frac{29}{3}\)

Explanation:
Step 1
Multiply the denominator by the whole number
12 × 9 = 108
Step 2
Add the answer from Step 1 to the numerator
108 + 8 = 116
Step 3
Write an answer from Step 2 over the denominator
116/12 = \(\frac{29}{3}\)

write the fraction as a mixed number or a whole number.

Question 7.
\(\frac{7}{5}\)

Answer: 1 \(\frac{2}{5}\)

Explanation:
Given the expression \(\frac{7}{5}\)
We have to convert the improper fraction to the mixed fraction.
7 ÷ 5=1R2
\(\frac{7}{5}\) = 1 \(\frac{2}{5}\)

Question 8.
\(\frac{10}{3}\)

Answer: 3 \(\frac{1}{3}\)

Explanation:
Given the expression \(\frac{10}{3}\)
We have to convert the improper fraction to the mixed fraction.
10÷3=3R1
\(\frac{10}{3}\) = 3 \(\frac{1}{3}\)

Question 9.
\(\frac{15}{4}\)

Answer: 3 \(\frac{3}{4}\)

Explanation:
Given the expression \(\frac{15}{4}\)
We have to convert the improper fraction to the mixed fraction.
15÷4=3 R 3
\(\frac{15}{4}\) = 3 \(\frac{3}{4}\)

Question 10.
\(\frac{32}{6}\)

Answer: 5 \(\frac{1}{3}\)

Explanation:
Given the expression \(\frac{32}{6}\)
We have to convert the improper fraction to the mixed fraction.
\(\frac{32}{6}\) = \(\frac{16}{3}\)
16÷3=5R1
\(\frac{32}{6}\) = 5 \(\frac{1}{3}\)

Question 11.
\(\frac{75}{8}\)

Answer: 9 \(\frac{3}{8}\)

Explanation:
Given the expression \(\frac{75}{8}\)
We have to convert the improper fraction to the mixed fraction.
75÷8=9R3
\(\frac{75}{8}\) = 9 \(\frac{3}{8}\)

Question 12.
\(\frac{40}{10}\)

Answer: 4

Explanation:
Given the expression \(\frac{40}{10}\)
We have to convert the improper fraction to the mixed fraction.
\(\frac{40}{10}\) = \(\frac{4}{1}\) = 4
Thus \(\frac{40}{10}\) = 4

Compare

Question 13.

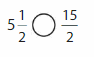
Answer: <

Explanation:
We have to convert the improper fraction to the mixed fraction.
5 \(\frac{1}{2}\) = \(\frac{11}{2}\)
\(\frac{11}{2}\) < \(\frac{15}{2}\)

Question 14.

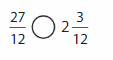
Answer: =

Explanation:
We have to convert the improper fraction to the mixed fraction.
\(\frac{27}{12}\) = \(\frac{27}{12}\)

Question 15.

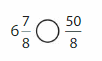
Answer:

Explanation:
We have to convert the improper fraction to the mixed fraction.
6 \(\frac{7}{8}\) = \(\frac{55}{8}\)
\(\frac{55}{8}\) > \(\frac{50}{8}\)

Question 16.
Number Sense
Complete the number line.

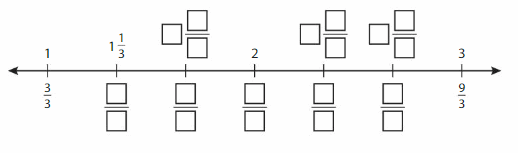
Answer:

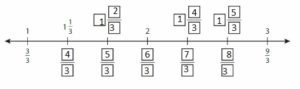
Question 17.
Modeling Real Life
You need pencil lead that is \(\frac{12}{10}\) millimeters thick to complete an art project. Which size of pencil lead should you use?

Answer:
Given,
You need pencil lead that is \(\frac{12}{10}\) millimeters thick to complete an art project.
1 \(\frac{1}{10}\) = \(\frac{11}{10}\)
1 \(\frac{2}{10}\) = \(\frac{12}{10}\)
1 \(\frac{4}{10}\) = \(\frac{14}{10}\)
You should use 2nd pencil lead.

Question 18.
DIG DEEPER!
You have a \(\frac{1}{4}\)-cup measuring cup and a \(\frac{1}{2}\)-cup measuring cup. What are two ways you can 2\(\frac{3}{4}\) cups of water?

Answer:
Given,
You have a \(\frac{1}{4}\)-cup measuring cup and a \(\frac{1}{2}\)-cup measuring cup.
\(\frac{1}{4}\) + \(\frac{1}{2}\) = \(\frac{3}{4}\)
2\(\frac{3}{4}\) – \(\frac{3}{4}\) = 2

Review & Refresh

Question 19.
67 × 31 = ___

Answer:
Multiply the two numbers 67 and 31.
67 × 31 = 2077

Question 20.
83 × 47 = ___

Answer:
Multiply the two numbers 83 and 47.
83 × 47 = 3901

Lesson 8.7 Add Mixed Numbers

Use model to find 2\(\frac{3}{8}\) + 1\(\frac{1}{8}\).

Answer: 3 \(\frac{1}{2}\)

Explanation:
Add the fractional parts and then the whole numbers.
Rewriting our equation with parts separated
=2+3/8+1+1/8
Solving the whole number parts
2+1=3
Solving the fraction parts
3/8+1/8=4/8
Reducing the fraction part, 4/8,
4/8=1/2
Combining the whole and fraction parts
3+1/2=3 1/2

Construct Arguments
How can you use the whole number parts and the fractional parts to add mixed numbers with like denominators? Explain why your method makes sense.

Answer: To add mixed numbers, we first add the whole numbers together, and then the fractions. If the sum of the fractions is an improper fraction, then we change it to a mixed number.

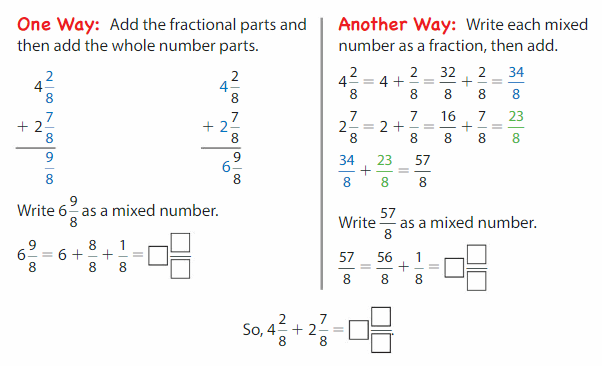
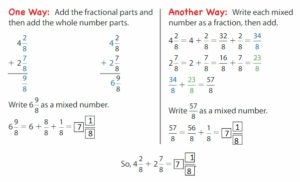
Think and Grow: Add Mixed Numbers

To add mixed numbers, add the fractional parts and add the whole number parts. Another way to add mixed numbers is to rewrite each number as a fraction, then add.

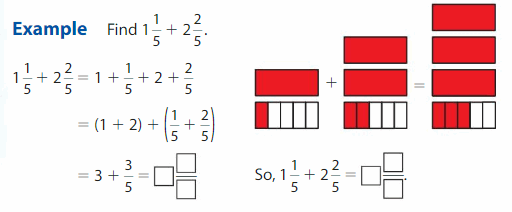
Answer:

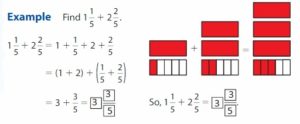
Example
Find 4\(\frac{2}{8}\) + 2\(\frac{7}{8}\).

Answer:

Apply and Grow: Practice

Add.

Question 3.
5\(\frac{1}{3}\) + 3\(\frac{2}{3}\) = ___

Answer: 9

Explanation:
Add the fractional parts and then the whole numbers.
Rewriting our equation with parts separated
=5+1/3+3+2/3
Solving the whole number parts
5+3=8
Solving the fraction parts
1/3+2/3=33
Reducing the fraction part, 3/3,
3/3=1/1
Simplifying the fraction part, 1/1,
1/1=1
Combining the whole and fraction parts
8+1=9

Question 4.
2\(\frac{8}{12}\) + 7\(\frac{5}{12}\) = ___

Answer: 10 \(\frac{1}{12}\)

Explanation:
Add the fractional parts and then the whole numbers.
Rewriting our equation with parts separated
=2+8/12+7+5/12
Solving the whole number parts
2+7=9
Solving the fraction parts
8/12+5/12=13/12
Simplifying the fraction part, 13/12,
13/12=1 1/12
Combining the whole and fraction parts
9+1+1/12=10 1/12

Question 5.
4 + 1\(\frac{1}{2}\) = ___

Answer: 5 \(\frac{1}{2}\)

Explanation:
Add the fractional parts and then the whole numbers.
Rewriting our equation with parts separated
=4+1+1/2
Solving the whole number parts
4+1=5
Combining the whole and fraction parts
5+1/2=5 1/2

Question 6.

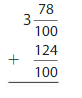
Answer: 5 \(\frac{2}{100}\)

Explanation:
Add the fractional parts and then the whole numbers.
Rewriting our equation with parts separated
=78/100+124/100 + 3
Solving the fraction parts
78/100+124/100=202/100
3 + 202/100 = 5 \(\frac{2}{100}\)

Question 7.

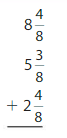
Answer: 16 \(\frac{3}{8}\)

Explanation:
Add the fractional parts and then the whole numbers.
8 + 5 + 2 = 15
\(\frac{4}{8}\) + \(\frac{3}{8}\) + \(\frac{4}{8}\) = (4 + 4 + 3)/8 = \(\frac{11}{8}\)
\(\frac{11}{8}\) = 1 \(\frac{3}{8}\)
15 + 1\(\frac{3}{8}\) = 16 \(\frac{3}{8}\)

Question 8.

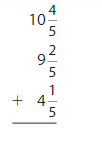
Answer: 24 \(\frac{2}{5}\)

Explanation:
Add the fractional parts and then the whole numbers.
10 + 9 + 4 = 23
\(\frac{4}{5}\) + \(\frac{2}{5}\) + \(\frac{1}{5}\) = \(\frac{7}{5}\)
Convert it into the mixed fraction.
\(\frac{7}{5}\) = 1 \(\frac{2}{5}\)
23 + 1 \(\frac{2}{5}\) = 24 \(\frac{2}{5}\)

Question 9.
Number Sense
Explain how to use the addition properties to find mentally. Then find the sum.

Answer: 16 \(\frac{3}{4}\)

Explanation:
Add the fractional parts and then the whole numbers.
6 + 8 + 1 = 15
\(\frac{3}{4}\) + \(\frac{2}{4}\) + \(\frac{1}{4}\) = \(\frac{6}{4}\)
Convert it into the mixed fraction.
\(\frac{6}{4}\) = 1 \(\frac{2}{4}\)
15 + 1 \(\frac{2}{4}\) = 16 \(\frac{2}{4}\)

Question 10.
DIG DEEPER!
When adding mixed numbers, is it always necessary to write the sum as a mixed number? Explain.

Answer: To add mixed numbers, we first add the whole numbers together, and then the fractions. If the sum of the fractions is an improper fraction, then we change it to a mixed number.

Question 11.
DIG DEEPER!
Find the unknown number.

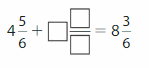
Answer:
Let the unknown number be x.
4 \(\frac{5}{6}\) + x = 8 \(\frac{3}{6}\)
x = 8 \(\frac{3}{6}\) – 4 \(\frac{5}{6}\)
x = 3 \(\frac{2}{3}\)

Think and Grow: Modeling Real Life

Example
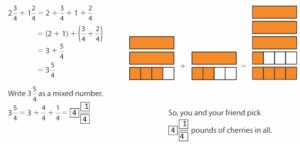
You pick 2\(\frac{3}{4}\) pounds of cherries. Your friend picks 1\(\frac{2}{4}\) pounds of cherries. How many pounds of cherries do you and your friend pick in all?

Add the amounts of cherries you and your friend each pick.

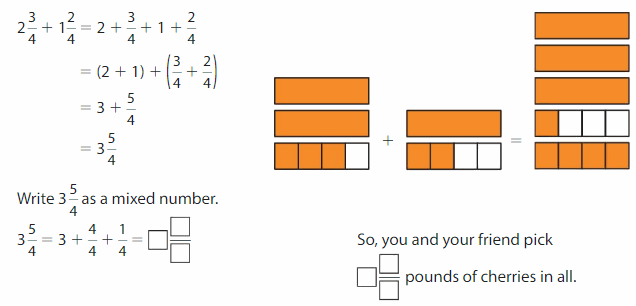
Answer:
You pick 2\(\frac{3}{4}\) pounds of cherries. Your friend picks 1\(\frac{2}{4}\) pounds of cherries.

Show and Grow

Question 12.
Before noon, 2\(\frac{3}{8}\) inches of snow falls in a city. Afternoon, 4\(\frac{6}{8}\) inches of snow falls. How many inches of snow falls in the city that day?

Answer:
Given that,
Before noon, 2\(\frac{3}{8}\) inches of snow falls in a city. Afternoon, 4\(\frac{6}{8}\) inches of snow falls.
2\(\frac{3}{8}\) + 4\(\frac{6}{8}\)
2 + 4 = 6
\(\frac{3}{8}\) + \(\frac{6}{8}\) = \(\frac{9}{8}\)
Convert it into the mixed fraction.
\(\frac{9}{8}\) = 1 \(\frac{1}{8}\)
1 \(\frac{1}{8}\) inches of snow falls in the city that day.

Question 13.
DIG DEEPER!
A student driver must practice driving at night for a total of at least 10 hours. Has the student met the nighttime driving requirement yet?

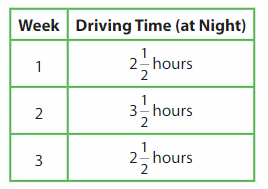
Answer:
2 \(\frac{1}{2}\) + 3 \(\frac{1}{2}\) + 2 \(\frac{1}{2}\)
First add the whole numbers
2 + 3 + 2 = 7
\(\frac{1}{2}\) + \(\frac{1}{2}\) + \(\frac{1}{2}\) = 1 \(\frac{1}{2}\)
7 + 1 \(\frac{1}{2}\) = 8 \(\frac{1}{2}\)

Add Mixed Numbers Homework & Practice 8.7

Add.

Question 1.

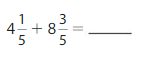
Answer: 12 \(\frac{4}{5}\)

Explanation:
Add the fractional parts and then the whole numbers.
4 + 8 = 12
Add the fractions
\(\frac{1}{5}\) + \(\frac{3}{5}\) = \(\frac{4}{5}\)
Now add the fractions and thw whole numbers
12 + \(\frac{4}{5}\) = 12 \(\frac{4}{5}\)

Question 2.

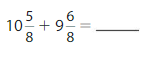
Answer: 20 \(\frac{3}{8}\)

Explanation:
Add the fractional parts and then the whole numbers.
10 + 9 = 19
Add the fractions
\(\frac{5}{8}\) + \(\frac{6}{8}\) = \(\frac{11}{8}\)
Convert it into the mixed fraction.
\(\frac{11}{8}\) = 1 \(\frac{3}{8}\)
Now add the fractions and the whole numbers
19 + 1 \(\frac{3}{8}\) = 20 \(\frac{3}{8}\)

Question 3.

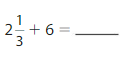
Answer: 8 \(\frac{1}{3}\)

Explanation:
Add the fractional parts and then the whole numbers.
2 + 6 = 8
Now add the fractions and the whole numbers
\(\frac{1}{3}\) + 8 = 8 \(\frac{1}{3}\)

Question 4.

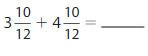
Answer:

Explanation:
Add the fractional parts and then the whole numbers.
3 + 4 = 7
\(\frac{10}{12}\) + \(\frac{10}{12}\) = \(\frac{20}{12}\)
Convert it into the mixed fraction.
\(\frac{20}{12}\) = 1 \(\frac{8}{12}\)
Now add the fractions and the whole numbers
7 + 1 \(\frac{8}{12}\) = 8 \(\frac{8}{12}\)

Question 5.

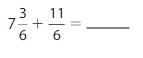
Answer: 9 \(\frac{2}{6}\)

Explanation:
Add the fractional parts and then the whole numbers.
Convert it into the mixed fraction.
\(\frac{11}{6}\) = 1 \(\frac{5}{6}\)
7 + 1 = 8
\(\frac{3}{6}\) + \(\frac{5}{6}\) = \(\frac{8}{6}\)
Now add the fractions and the whole numbers
8 + \(\frac{8}{6}\)
\(\frac{8}{6}\) = 1 \(\frac{2}{6}\)
8 + 1 \(\frac{2}{6}\) = 9 \(\frac{2}{6}\)

Question 6.

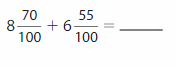
Answer: 15 \(\frac{1}{4}\)

Explanation:
Add the fractional parts and then the whole numbers.
Rewriting our equation with parts separated
=8+70/100+6+55/100
Solving the whole number parts
8+6=14
Solving the fraction parts
70/100+55/100=125/100
Reducing the fraction part, 125/100,
125/100=5/4
Simplifying the fraction part, 5/4,
5/4=1 1/4
Combining the whole and fraction parts
14+1+1/4= 15 \(\frac{1}{4}\)

Add

Question 7.

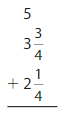
Answer: 11

Explanation:
Add the fractional parts and then the whole numbers.
5 + 3 + 2 = 10
Now add the fractional part,
3/4 + 1/4 = 1
10 + 1 = 11

Question 8.

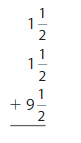
Answer: 12 \(\frac{1}{2}\)

Explanation:
Add the fractional parts and then the whole numbers.
Add the whole numbers
1 + 1 + 9 = 11
\(\frac{1}{2}\) + \(\frac{1}{2}\) + \(\frac{1}{2}\) = 1 \(\frac{1}{2}\)
Combining the whole and fraction parts
11 + 1 \(\frac{1}{2}\) = 12 \(\frac{1}{2}\)

Question 9.

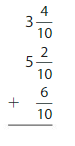
Answer: 9 \(\frac{2}{10}\)

Explanation:
Add the fractional parts and then the whole numbers.
3 + 5 = 8
\(\frac{4}{10}\) + \(\frac{2}{10}\) + \(\frac{6}{10}\) = \(\frac{12}{10}\)
Convert it into the mixed fraction.
\(\frac{12}{10}\) = 1 \(\frac{2}{10}\)
Combining the whole and fraction parts
8 + 1 \(\frac{2}{10}\) = 9 \(\frac{2}{10}\)

Question 10.
Structure
Find 7\(\frac{4}{5}\) + 8\(\frac{2}{5}\) two different ways. Which way do you prefer? Why?

Answer:

Explanation:
Add the fractional parts and then the whole numbers.
7\(\frac{4}{5}\) + 8\(\frac{2}{5}\)
Add the fractional parts and then the whole numbers.
7 + 8 = 15
\(\frac{4}{5}\) + \(\frac{2}{5}\) = \(\frac{6}{5}\)
Convert it into the mixed fraction.
\(\frac{6}{5}\) = 1 \(\frac{1}{5}\)
15 + 1 \(\frac{1}{5}\) = 16 \(\frac{1}{5}\)

Question 11.
Modeling Real Life
A homeowner has two strings of lights. One is 8\(\frac{1}{3}\) yards long. The other is 16\(\frac{2}{3}\) yards long. He connects the strings of lights. How long will the string of lights be in all?

Answer:
Given,
A homeowner has two strings of lights. One is 8 \(\frac{1}{3}\) yards long. The other is 16 \(\frac{2}{3}\) yards long. He connects the strings of lights.
8 \(\frac{1}{3}\) + 16 \(\frac{2}{3}\) = 24 \(\frac{3}{3}\)
\(\frac{3}{3}\) = 1
24 + 1 = 25

Question 12.
DIG DEEPER!
You can play the song “Mary Had a Little Lamb” by striking three glasses filled with water to make the tone. The first glass needs 1\(\frac{3}{4}\) cups, the second glass needs 1\(\frac{1}{2}\) cups, and the third glass needs 1\(\frac{1}{4}\) cups of water. How much water do you need in all?

Answer:
Given that,
You can play the song “Mary Had a Little Lamb” by striking three glasses filled with water to make the tone. The first glass needs 1\(\frac{3}{4}\)cups, the second glass needs 1\(\frac{1}{2}\) cups, and the third glass needs 1\(\frac{1}{4}\) cups of water.
1\(\frac{1}{2}\) + 1\(\frac{1}{4}\) + 1\(\frac{3}{4}\)
1 + 1 + 1 = 3
\(\frac{1}{2}\) + \(\frac{1}{4}\) + \(\frac{3}{4}\) = 1 \(\frac{1}{2}\)
3 + 1 \(\frac{1}{2}\) = 4 \(\frac{1}{2}\)
Therefore you need 4 \(\frac{1}{2}\) cups of water.

Review & Refresh

Write the first six numbers in the pattern. Then describe another feature of the pattern.

Question 13.
Rule: Add 11.
First number: 22

Answer:
The first number is 22 you need to add 11 to it.
22 + 11 = 33
33 + 11 = 44
44 + 11 = 55
55 + 11 = 66
66 + 11 = 77
77 + 11 = 88

Question 14.
Rule: Multiply by 4.
First number: 7

Answer:
The first number is 7. You need to multiply by 4.
7 × 4 = 28
28 × 4 = 112
112 × 4 = 448
448 × 4 = 1792
1792 × 4 = 7168
7168 × 4 = 28672

Lesson 8.8 Subtract Mixed Numbers

Explore and Grow

Use a model to find 2\(\frac{3}{8}\) – 1\(\frac{1}{8}\).

Answer:
2\(\frac{3}{8}\) – 1\(\frac{1}{8}\).
First subtract the whole numbers
2 – 1 = 1
\(\frac{3}{8}\) – \(\frac{1}{8}\) = \(\frac{2}{8}\)
Combine the whole numbers and fractions.
1 \(\frac{2}{8}\) = 1 \(\frac{1}{4}\)

Construct Arguments
How can you use the whole number parts and the fractional parts to subtract mixed numbers with like denominators? Explain why your method makes sense.

Answer:
First, you have to subtract the whole number parts and then subtract the fraction parts with like denominators.
You can subtract the mixed fractions by using the number line or model.

Think and Grow: subtract Mixed Numbers

To subtract mixed numbers, subtract the fractional parts and subtract the whole number parts. Another way to subtract mixed numbers is to rewrite each number as a fraction, then subtract.

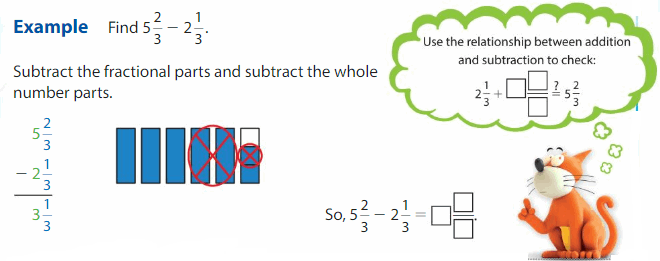
Answer:

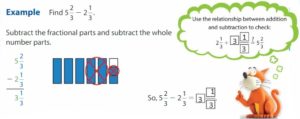
Example
Find 5\(\frac{3}{6}\) – 4\(\frac{5}{6}\).

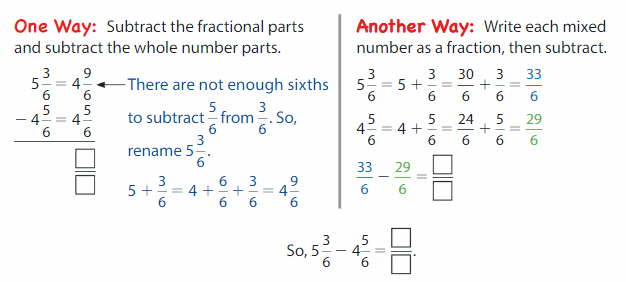
Answer:

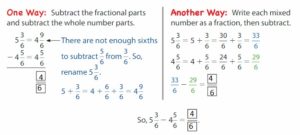
Show and Grow

Subtract

Question 1.
5\(\frac{4}{5}\) – 1\(\frac{2}{5}\) = ____

Answer:
There are enough fifths.
Subtract the whole numbers
5 – 1 = 4
\(\frac{4}{5}\) – \(\frac{2}{5}\) =\(\frac{2}{5}\)
4 + \(\frac{2}{5}\) = 4 \(\frac{2}{5}\)
Thus, 5\(\frac{4}{5}\) – 1\(\frac{2}{5}\) = 4 \(\frac{2}{5}\)

Question 2.
7\(\frac{1}{3}\) – 2\(\frac{2}{3}\) = _____

Answer:
There are not enough thirds.
7\(\frac{1}{3}\) = 6 \(\frac{3}{3}\) + \(\frac{1}{3}\) = 6\(\frac{4}{3}\)
Subtract the whole numbers
6 – 2 = 4
\(\frac{4}{3}\) – \(\frac{2}{3}\) = \(\frac{2}{3}\)
4 + \(\frac{2}{3}\) = 4 \(\frac{2}{3}\)

Question 3.

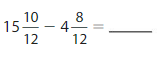
Answer:
There are enough twelfths.
Subtract the whole numbers
15 – 4 = 11
\(\frac{10}{12}\) – \(\frac{8}{12}\) = \(\frac{2}{12}\)
11 + \(\frac{2}{12}\) = 11 \(\frac{2}{12}\)

Question 4.

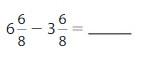
Answer:
There are enough eighths.
\(\frac{6}{8}\) – \(\frac{6}{8}\) = 0
Subtract the whole numbers
6 – 3 = 3
3 + 0 = 3

Question 5.

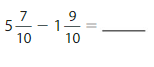
Answer:
There are not enough tenths.
5 \(\frac{7}{10}\) can be written as 4 \(\frac{17}{10}\)
4 \(\frac{17}{10}\) – 1 \(\frac{9}{10}\)
Subtract the whole numbers
4 – 1 = 3
Subtract the fractional parts
\(\frac{17}{10}\) – \(\frac{9}{10}\) = \(\frac{8}{10}\)
3 + \(\frac{8}{10}\) = 3 \(\frac{8}{10}\)

Question 6.

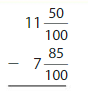
Answer:
There are not enough hundreds.
11 \(\frac{50}{100}\) can be written as 10 \(\frac{150}{100}\)
Subtract the whole numbers
10 – 7 = 3
Subtract the fractional parts
\(\frac{150}{100}\) – \(\frac{85}{100}\) = \(\frac{65}{100}\)
3 + \(\frac{65}{100}\) = 3 \(\frac{65}{100}\)

Question 7.

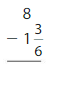
Answer:
There are not enough sixths.
8 can be written as 7 \(\frac{6}{6}\)
Subtract the whole numbers
7 – 1 = 6
Subtract the fractional parts
\(\frac{6}{6}\) – \(\frac{3}{6}\) = \(\frac{3}{6}\)
6 + \(\frac{3}{6}\) = 6 \(\frac{3}{6}\)

Question 8.

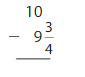
Answer:
There are not enough fourths.
10 can be written as 9 \(\frac{4}{4}\)
Subtract the whole numbers
9 – 9 = 0
Subtract the fractional parts
\(\frac{4}{4}\) – \(\frac{3}{4}\) = \(\frac{1}{4}\)
0 + \(\frac{1}{4}\)  = \(\frac{1}{4}\)

Question 9.
YOU BE THE TEACHER
Your friend says the difference of 9 and 2\(\frac{3}{5}\) is 7\(\frac{3}{5}\). Is your friend correct? Explain.

Answer:
9 – 2\(\frac{3}{5}\) = 7 \(\frac{3}{5}\)
Thus by this we can say that your friend is correct.

Question 10.
Writing
Explain how adding and subtracting mixed numbers are similar and different.

Answer:
Any mixed number can also be written as an improper fraction, in which the numerator is larger than the denominator.
Subtracting mixed numbers is very similar to adding them.
Write both fractions as equivalent fractions with a denominator. Then subtract the fractions.

Question 11.
DIG DEEPER!
Write two mixed numbers with like denominators that have a sum of 5\(\frac{2}{3}\) and a difference of 1.

Answer:
5\(\frac{2}{3}\) = 3 \(\frac{1}{3}\) + 2\(\frac{1}{3}\)
Now if you subtract the same fraction you need to get the difference as 1.
3 \(\frac{1}{3}\) – 2\(\frac{1}{3}\)
3 – 2 = 1
\(\frac{1}{3}\) – \(\frac{1}{3}\) = 0
So, 3 \(\frac{1}{3}\) – 2\(\frac{1}{3}\) = 1

Think and Grow: Modeling Real Life

Example
A replica of the Eiffel Tower is 6 inches tall. It is 2\(\frac{2}{5}\) inches taller than a replica of the Space Needle. How tall is the replica of the Space Needle?
Find the difference between the height of the Eiffel Tower replica, 6 inches, and 2\(\frac{2}{5}\) inches.

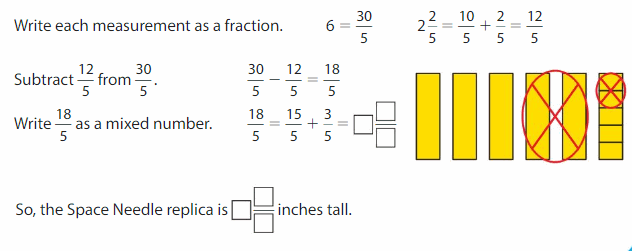
Answer:
Given,
A replica of the Eiffel Tower is 6 inches tall. It is 2\(\frac{2}{5}\) inches taller than a replica of the Space Needle.

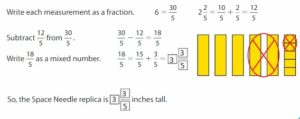
Show and Grow

Question 12.
A cook has a 5-pound bag of potatoes. He uses 2\(\frac{1}{3}\) pounds of potatoes to make a casserole. How many pounds of potatoes are left?

Answer:
Given,
A cook has a 5-pound bag of potatoes. He uses 2\(\frac{1}{3}\) pounds of potatoes to make a casserole.
5 – 2\(\frac{1}{3}\)
4 \(\frac{3}{3}\) – 2\(\frac{1}{3}\)
Subtract the whole numbers
4 – 2 = 2
Subtract the fractional parts
\(\frac{3}{3}\) – \(\frac{1}{3}\) = \(\frac{2}{3}\)
2 \(\frac{2}{3}\)
Thus 2 \(\frac{2}{3}\) pounds of potatoes are left.

Question 13.
A half-marathon is 13\(\frac{1}{10}\) miles long. A competitor runs 9\(\frac{6}{10}\) miles. How many miles does the competitor have left to run?

Answer:
Given,
A half-marathon is 13\(\frac{1}{10}\) miles long. A competitor runs 9\(\frac{6}{10}\) miles.
13\(\frac{1}{10}\) – 9\(\frac{6}{10}\)
12 \(\frac{11}{10}\) – 9\(\frac{6}{10}\)
Subtract the whole numbers
12 – 9 = 3
\(\frac{11}{10}\) – \(\frac{6}{10}\) = \(\frac{5}{10}\)
3 + \(\frac{5}{10}\) = 3 \(\frac{1}{2}\)
The competitor has left 3 \(\frac{1}{2}\) miles to run.

Question 14.
DIG DEEPER!
You want to mail a package that weighs 18\(\frac{2}{4}\) ounces. The weight limit is 13 ounces, so you remove 4\(\frac{3}{4}\) ounces of items from the package. Does the lighter package meet the weight requirement? If not, how much more weight do you need to remove?

Answer:
Given that,
You want to mail a package that weighs 18\(\frac{2}{4}\) ounces.
The weight limit is 13 ounces, so you remove 4\(\frac{3}{4}\) ounces of items from the package.
18\(\frac{2}{4}\) – 4\(\frac{3}{4}\) = 13 \(\frac{3}{4}\)
13 \(\frac{3}{4}\) – 13 = \(\frac{3}{4}\)
Thus you need to remove \(\frac{3}{4}\) ounces more.

Subtract Mixed Numbers Homework & Practice 8.8

Subtract

Question 1.

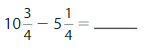
Answer: 5 \(\frac{1}{2}\)

Explanation:
Rewriting our equation with parts separated
=10+3/4−5−1/4
Solving the whole number parts
10−5=5
Solving the fraction parts
3/4−1/4=2/4
Reducing the fraction part, 2/4,
2/4=1/2
Combining the whole and fraction parts
5+1/2=5 1/2

Question 2.

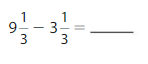
Answer: 6

Explanation:
Rewriting our equation with parts separated
9 + 1/3 – 3 – 1/3
9 – 3 = 6
So, 9 \(\frac{1}{3}\) – 3 \(\frac{1}{3}\) = 6

Question 3.

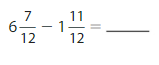
Answer: 4 \(\frac{2}{3}\)

Explanation:
Rewriting our equation with parts separated
=6+7/12−1−11/12
Solving the whole number parts
6−1=5
Solving the fraction parts
7/12−11/12=−4/12
Reducing the fraction part, 4/12,
−4/12=−1/3
Combining the whole and fraction parts
5−1/3=4 2/3

Question 4.

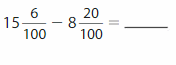
Answer: 6 \(\frac{43}{50}\)

Explanation:
Rewriting our equation with parts separated
=15+6/100−8−20/100
Solving the whole number parts
15−8=7
Solving the fraction parts
6/100−20/100=−14/100
Reducing the fraction part, 14/100,
−14/100=−7/50
Combining the whole and fraction parts
7−7/50=6 43/50

Question 5.

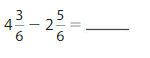
Answer: 1 \(\frac{2}{3}\)

Explanation:
Rewriting our equation with parts separated
=4+3/6−2−5/6
Solving the whole number parts
4−2=2
Solving the fraction parts
3/6−5/6=−2/6
Reducing the fraction part, 2/6,
−2/6=−1/3
Combining the whole and fraction parts
2−1/3=1 2/3

Question 6.

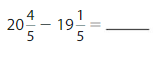
Answer: 1 \(\frac{3}{5}\)

Explanation:
20 – 19 = 1
\(\frac{4}{5}\) – \(\frac{1}{5}\) = \(\frac{3}{5}\)
1 + \(\frac{3}{5}\) = 1 \(\frac{3}{5}\)

Subtract.

Question 7.

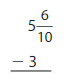
Answer: 2 \(\frac{3}{5}\)

Explanation:
Rewriting our equation with parts separated
=5+6/10−3
Solving the whole number parts
5−3=2
Combining the whole and fraction parts
2+6/10=2 6/10

Question 8.

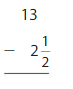
Answer: 10 \(\frac{1}{2}\)

Explanation:
Rewriting our equation with parts separated
=13−2−1/2
Solving the whole number parts
13−2=11
Combining the whole and fraction parts
11−1/2=10 1/2

Question 9.

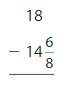
Answer: 3 \(\frac{1}{4}\)

Explanation:
Rewriting our equation with parts separated
=18−14−6/8
Solving the whole number parts
18−14=4
Combining the whole and fraction parts
4−6/8=3 2/8

Question 10.
Reasoning
Explain why you rename 4\(\frac{1}{3}\) when finding 4\(\frac{1}{3}\) – \(\frac{2}{3}\) .

Answer:
4\(\frac{1}{3}\) – \(\frac{2}{3}\)
4 can be written as 3 \(\frac{3}{3}\)
3 \(\frac{3}{3}\) – \(\frac{2}{3}\)
3 + \(\frac{3}{3}\) – \(\frac{2}{3}\)
3 + \(\frac{1}{3}\) = 3 \(\frac{1}{3}\)
So, 4\(\frac{1}{3}\) – \(\frac{2}{3}\) = 3 \(\frac{1}{3}\)

Question 11.
DIG DEEPER!
Find the unknown number.

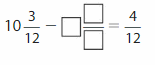
Answer:
Let the unknown number be x.
10 \(\frac{3}{12}\) – x = \(\frac{4}{12}\)
10 \(\frac{3}{12}\) –  \(\frac{4}{12}\) = x
9 \(\frac{15}{12}\) –  \(\frac{4}{12}\) = x
x = 9 \(\frac{11}{12}\)
Thus the unknown number is 9 \(\frac{11}{12}\).

Question 12.
Modeling Real Life
A rare flower found in Indonesian rain forests can grow wider than a car tire. How much wider is the flower than a car tire that is 1\(\frac{11}{12}\) feet wide?

Answer:
Given,
A rare flower found in Indonesian rain forests can grow wider than a car tire.
3 – 1\(\frac{11}{12}\)
2 \(\frac{12}{12}\) – 1\(\frac{11}{12}\)
= 1 \(\frac{1}{12}\)

Question 13.
Modeling Real Life
Your tablet battery is fully charged. You use \(\frac{32}{100}\) of the charge listening to music, and \(\frac{13}{100}\) of the charge playing games. What fraction of the charge remains on your tablet battery?

Answer:
Given,
Your tablet battery is fully charged. You use \(\frac{32}{100}\) of the charge listening to music, and \(\frac{13}{100}\) of the charge playing games.
\(\frac{32}{100}\) – \(\frac{13}{100}\) = \(\frac{19}{100}\)
Thus \(\frac{19}{100}\) fraction of the charge remains on your tablet battery.

Review & Refresh

Divide. Then check your answer.

Question 14.

Answer:
Divide 84 by 5
84/5 = 16.8

Question 15.

Answer:
Divide 51 by 4.
51/4 = 12.75

Question 16.

Answer:
Divide 89 by 8.
89/8 = 11.125

Lesson 8.9 Problem Solving: Fractions

Explore and Grow

Make a plan to solve the problem.

The table shows the tusk lengths of two elephants. Which elephant’s tusks have a greater total length? How much greater?

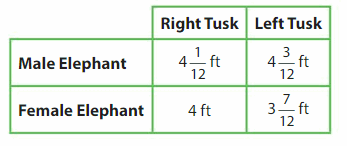
Answer:
Male Elephant = 4 1/12 + 4 3/12 = 8 4/12
Female Elephant = 4 + 3 7/12 = 7 7/12
The Right Tusk of a Male Elephant is greater than Female Elephant.
The left tusk of a Male Elephant is greater than Female Elephant.
Thus the total length of the Male Elephant is greater than Female Elephant.

Make Sense of Problems
A \(\frac{7}{12}\)-foot long piece of one of the male elephant’s tusks breaks off. Does this change your plan to solve the problem? Will this change the answer? Explain.

Answer:
A \(\frac{7}{12}\)-foot long piece of one of the male elephant’s tusks breaks off.
8 4/12 – 7 7/12 = 3/4
No, if \(\frac{7}{12}\)-foot long piece of one of the male elephant’s tusks breaks off it will not change the answer. Still, the Male Elephant is greater than Female Elephant.

Think and Grow: Problem Solving: Fractions

Example
A family spends 2\(\frac{2}{4}\) hours traveling to a theme park, 7\(\frac{1}{4}\) hours at the theme park, and 2\(\frac{3}{4}\) hours traveling home. How much more time does the family spend at the theme park than traveling?

Understand the Problem

What do you know?

• The family spends 2\(\frac{2}{4}\) hours traveling to the theme park, 7\(\frac{1}{4}\) hours at the theme park, 2\(\frac{3}{4}\) hours traveling home.
  What do you need to find?
• You need to find how much more time the family spends at the theme park than the traveling.

Make a plan

How will you solve it?

• Add 2\(\frac{2}{4}\) and 2\(\frac{3}{4}\) to find how much time the family spends traveling.
• Then subtract the sum from 7\(\frac{1}{4}\) to find how much more time they spend at the theme park.

Solve
So, the family spends ___ more hours at the theme park than traveling.

Show and Grow

Question 1.
Explain how you can check your answer in each step of the example above.

Answer:

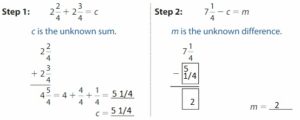
So, the family spends 2 more hours at the theme park than traveling.

Apply any and Grow: Practice

Understand the problem. What do you know? What do you need to find? Explain.

Answer:

• The family spends 2\(\frac{2}{4}\) hours traveling to the theme park, 7\(\frac{1}{4}\) hours at the theme park, 2\(\frac{3}{4}\) hours traveling home.
  What do you need to find?
• You need to find how much more time the family spends at the theme park than the traveling.

Question 2.
You are making a sand art bottle. You fill \(\frac{1}{6}\) of the bottle with pink sand, \(\frac{3}{6}\) with red sand, and \(\frac{2}{6}\) with white sand. How much of the bottle is filled?

Answer:
Given that,
You are making a sand art bottle. You fill \(\frac{1}{6}\) of the bottle with pink sand, \(\frac{3}{6}\) with red sand, and \(\frac{2}{6}\) with white sand.
\(\frac{1}{6}\) + \(\frac{3}{6}\) + \(\frac{2}{6}\) = \(\frac{1}{6}\)
Thus \(\frac{1}{6}\) of the bottle is filled.

Question 3.
Your friend has \(\frac{1}{8}\) of a photo album filled with beach photographs and \(\frac{4}{8}\) of the album filled with photos of friends. What fraction of the photo album is left?

Answer:
Given that,
Your friend has \(\frac{1}{8}\) of a photo album filled with beach photographs and \(\frac{4}{8}\) of the album filled with photos of friends.
\(\frac{1}{8}\) + \(\frac{4}{8}\) = \(\frac{5}{8}\)
\(\frac{8}{8}\) – \(\frac{5}{8}\) = \(\frac{3}{8}\)
Thus \(\frac{3}{8}\) fraction of the photo album is left.

Understand the problem. Then make a plan. How will you solve? Explain.

Question 4.
In Race A, an Olympic swimmer swims 100 meters in 62\(\frac{25}{100}\) seconds. In Race B, she cuts 2\(\frac{38}{100}\) seconds off her Race A time. How many seconds does she need to cut off her Race B time to swim 100 meters in 58\(\frac{45}{100}\) seconds?

Answer:
Given,
In Race A, an Olympic swimmer swims 100 meters in 62\(\frac{25}{100}\) seconds. In Race B, she cuts 2\(\frac{38}{100}\) seconds off her Race A time.
62\(\frac{25}{100}\) – 2\(\frac{38}{100}\) = 59 \(\frac{87}{100}\)
59 \(\frac{87}{100}\) – 58\(\frac{45}{100}\) = 1 \(\frac{42}{100}\)
She need 1 \(\frac{42}{100}\) to cut off her Race B time to swim 100 meters in 58\(\frac{45}{100}\) seconds.

Question 5.
A semi-truck has 2 fuel tanks that each hold the same amount of fuel. A truck driver fills up both tanks and uses \(\frac{3}{4}\) tank of gasoline driving to his first stop. He uses \(\frac{2}{4}\) tank of gasoline driving to his second stop. How much gasoline does he have left?

Answer:
Given that,
A semi-truck has 2 fuel tanks that each hold the same amount of fuel. A truck driver fills up both tanks and uses \(\frac{3}{4}\) tank of gasoline driving to his first stop. He uses \(\frac{2}{4}\) tank of gasoline driving to his second stop.
\(\frac{3}{4}\) + \(\frac{2}{4}\) = \(\frac{5}{4}\)
2 – \(\frac{5}{4}\) = \(\frac{3}{4}\)
Thus \(\frac{3}{4}\) gasoline has left.

Question 6.
A bootlace worm holds the record as the longest animal at 180 feet long. How much longer is it than 2 blue whales combined?

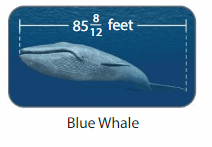
Answer:
Given,
A bootlace worm holds the record as the longest animal at 180 feet long.
1 blue whale = 85 \(\frac{8}{12}\)
2 blue whales = 85 \(\frac{8}{12}\) + 85 \(\frac{8}{12}\) = 171 \(\frac{1}{3}\)
180 – 171 \(\frac{1}{3}\)
179 \(\frac{3}{3}\) – 171 \(\frac{1}{3}\) = 8 \(\frac{2}{3}\)

Think and Grow: Modeling Real Life

Example
You walk \(\frac{1}{10}\) kilometer on Monday, \(\frac{3}{10}\) kilometer on Tuesday, and \(\frac{5}{10}\) kilometer on Wednesday. You continue the pattern on Thursday and Friday. How many kilometers do you walk in all?
Think: What do you know? What do you need to find? How will you solve?

Step 1: Identify the pattern.

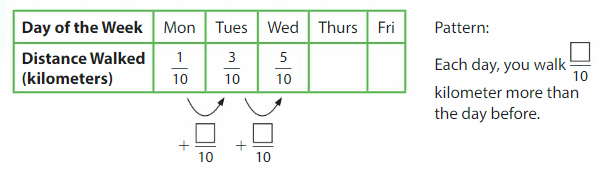
Step 2: Use the pattern to find the distances you walk on Thursday and Friday.

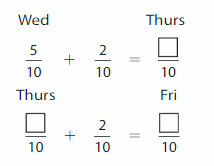
Step 3: Add all of the distances.

.

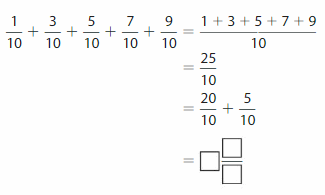
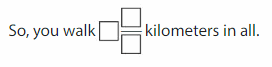Answer:
Step 1: Identify the pattern.

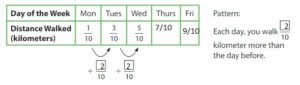
Step 2: Use the pattern to find the distances you walk on Thursday and Friday.

Step 3: Add all of the distances.

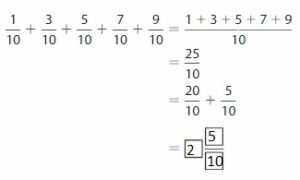
So, you walk 2 \(\frac{5}{10}\) kilometers in all.

Show and Grow

Question 7.
You save \(\frac{1}{4}\) dollar the first week, \(\frac{2}{4}\) dollar the next week, and dollar \(\frac{3}{4}\) dollar the following week. You continue the pattern for 3 more weeks. How much money do you save after 6 weeks?

Answer:
You save \(\frac{1}{4}\) dollar the first week, \(\frac{2}{4}\) dollar the next week, and dollar \(\frac{3}{4}\) dollar the following week. You continue the pattern for 3 more weeks.
\(\frac{1}{4}\), \(\frac{2}{4}\), \(\frac{3}{4}\), \(\frac{4}{4}\), \(\frac{5}{4}\), \(\frac{6}{4}\)
You save \(\frac{6}{4}\) dollar after 6 weeks.

Problem Solving: Fractions Homework & Practice 8.9

Question 1.
An older washing machine uses 170\(\frac{3}{10}\) liters of water per load. A new, high-efficiency, washing machine uses 75\(\frac{7}{10}\) fewer liters than the older washing machine. How many liters of water will the high-efficiency washing machine use for 2 loads of laundry?

Answer:
Given,
An older washing machine uses 170\(\frac{3}{10}\) liters of water per load. A new, high-efficiency, washing machine uses 75\(\frac{7}{10}\) fewer liters than the older washing machine.
75\(\frac{7}{10}\) + 75\(\frac{7}{10}\) = 151\(\frac{2}{5}\)
170\(\frac{3}{10}\) – 151\(\frac{2}{5}\) = 18 \(\frac{9}{10}\)

Question 2.
A student jumps 40 \(\frac{5}{12}\) inches for the high jump. On his second try, he jumps 1\(\frac{8}{12}\) inches higher. He can tie the school record if he raises the bar another 3\(\frac{10}{12}\) inches and successfully jumps over it. What is the school record for the high jump?

Answer:
Given,
A student jumps 40 \(\frac{5}{12}\) inches for the high jump.
On his second try, he jumps 1\(\frac{8}{12}\) inches higher.
He can tie the school record if he raises the bar another 3\(\frac{10}{12}\) inches and successfully jumps over it.
40 \(\frac{5}{12}\) + 1 \(\frac{8}{12}\) = 42 \(\frac{1}{12}\)
40 \(\frac{5}{12}\) + 3\(\frac{10}{12}\) = 44 \(\frac{3}{12}\)
44 \(\frac{3}{12}\) is the school record for the high jump.

Question 3.
You are shipping three care packages. The first package weighs 10\(\frac{1}{10}\) pounds. The second weighs 5\(\frac{7}{10}\) pounds, and the third weighs 25\(\frac{8}{10}\) pounds. What is the total weight of the packages?

Answer:
Given,
You are shipping three care packages. The first package weighs 10\(\frac{1}{10}\) pounds.
The second weighs 5\(\frac{7}{10}\) pounds, and the third weighs 25\(\frac{8}{10}\) pounds.
10\(\frac{1}{10}\) + 5\(\frac{7}{10}\) + 25\(\frac{8}{10}\) = 41 \(\frac{6}{10}\)
The total weight of the packages is 41 \(\frac{6}{10}\) pounds.

Question 4.
A person’s arm span is approximately equal to the person’s height. How tall is this fourth grader according to his arm span?

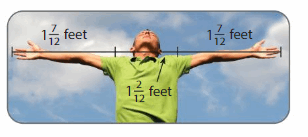
Answer:
Given,
A person’s arm span is approximately equal to the person’s height.
By using the pattern we can find the arm span of the fourth-grader i.e., 1 \(\frac{7}{12}\)

Question 5.
Writing
Write and solve a two-step word problem with mixed numbers that can be solved using addition or subtraction.

Answer:
I have 5 \(\frac{8}{12}\) episodes of my favorite series download onto my computer. I Downloaded some yesterday and \(\frac{7}{12}\) of the episodes this morning. The download speed was really slow. What fraction of the episodes did I download yesterday?
5 \(\frac{8}{12}\) – \(\frac{7}{12}\) = 5 \(\frac{1}{12}\) = \(\frac{61}{12}\)

Question 6.
Modeling Real Life
Your friend walks \(\frac{2}{10}\) mile to school each day. She walks the same distance home. How many miles does she walk to and from school in one 5-day school week?

Answer:
Given,
Your friend walks \(\frac{2}{10}\) mile to school each day. She walks the same distance home.
\(\frac{2}{10}\) + \(\frac{2}{10}\) = \(\frac{4}{10}\)
5 × \(\frac{4}{10}\) = \(\frac{20}{10}\) = 2
Thus she walk to and from school in one 5-day school week is 2 miles.

Question 7.
DIG DEEPER!
A store sells cashews in \(\frac{2}{3}\)-pound bags. You buy some bags and repackage the cashews into 1-pound bags. What is the least number of bags you should buy so that you do not have any cashews left over?

Answer:
Given,
A store sells cashews in \(\frac{2}{3}\)-pound bags. You buy some bags and repackage the cashews into 1-pound bags.
1 – \(\frac{2}{3}\) = \(\frac{1}{3}\)
Thus \(\frac{1}{3}\) pound of cashews left over.

Review & Refresh

Compare.

Question 8.

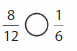
Answer: >

Explanation:
\(\frac{8}{12}\) = \(\frac{4}{6}\)
\(\frac{4}{6}\) > \(\frac{1}{6}\)

Question 9.

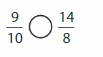
Answer: <

Explanation:
First, make the denominators common.
\(\frac{9}{10}\) = \(\frac{18}{20}\)
\(\frac{14}{8}\) = \(\frac{35}{20}\)
\(\frac{18}{20}\) < \(\frac{35}{20}\)

Question 10.

Answer: >

Explanation:
First, make the denominators common.
\(\frac{3}{4}\)
\(\frac{1}{2}\) × 2/2 = \(\frac{2}{4}\)
\(\frac{3}{4}\) > \(\frac{2}{4}\)

Add and Subtract Fractions Performance Task 8

The notes on sheet music tell you what note to play and how long to hold each note. The table shows how long you hold some notes compared to the length of one whole note.

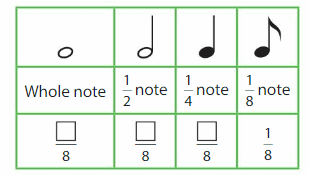
1. a. Complete the table by writing equivalent fractions.

Answer:

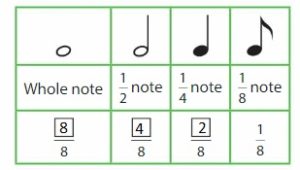
A whole note is nothing but 1 so the fraction is 8/8.
1/2 note is nothing but 4/8.
1/4 note is nothing but 2/8.

b. Each group of notes represents one measure. What is the sum of the values of the notes in each measure?

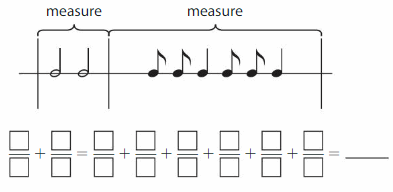
Answer:

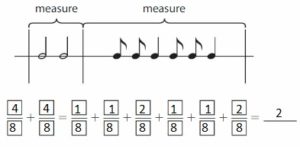
c. Draw the missing note to complete each measure.

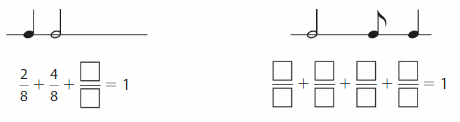
Answer:

d. Draw one measure of notes where the sum of the values is 1. Show your work.
___________

Answer:

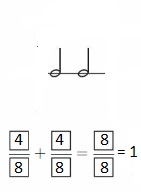
e. Write the fraction represented by the sum of the notes. Then write the fraction as a sum of fractions in two different ways.

Answer:
= \(\frac{1}{8}\) + \(\frac{4}{8}\) + \(\frac{2}{8}\) = \(\frac{7}{8}\)

Add and Subtract Fractions Activity

Three In a Row: Fraction Add or Subtract

Directions:

1. Players take turns.
2. On your turn, spin both spinners. Choose whether to add or subtract.
3. Add or subtract the mixed number and fraction. Cover the sum or difference.
4. If the sum or difference is already covered, you lose your turn.
5. The first player to get three in a row wins!

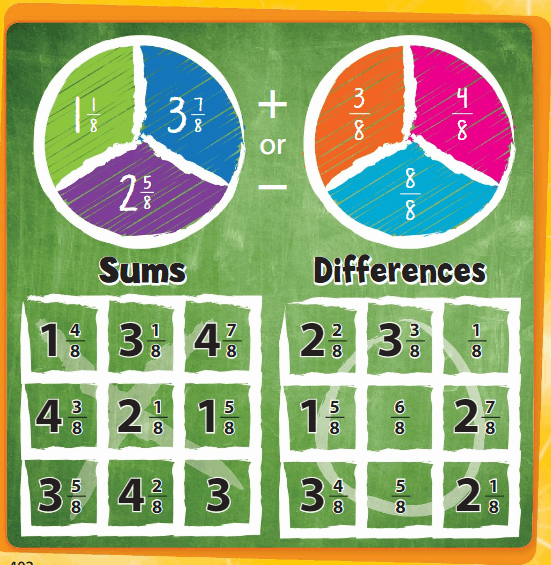
Answer:
1 \(\frac{1}{8}\) + \(\frac{3}{8}\) = 1 + \(\frac{1}{8}\) + \(\frac{3}{8}\) = 1 \(\frac{4}{8}\)
3 \(\frac{7}{8}\) + \(\frac{8}{8}\) = 3 + \(\frac{7}{8}\) + 1 = 4 \(\frac{7}{8}\)
2 \(\frac{5}{8}\) + \(\frac{4}{8}\) = 2 + \(\frac{5}{8}\) + \(\frac{4}{8}\) = 3 \(\frac{1}{8}\)

Add and Subtract Fractions Chapter Practice 8

8.1 Use Models to Add Fractions

Find the sum. Explain how you used the model to add.

Question 1.

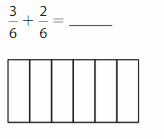
Answer: 5/6

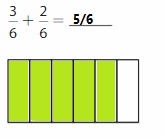
Question 2.

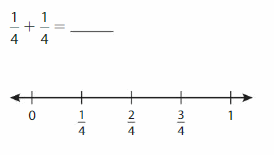
Answer:

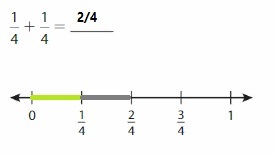
Find the sum. Use a model or a number line to help.

Question 3.

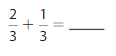
Answer:

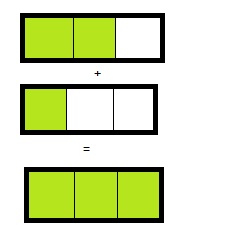
Question 4.

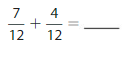
Answer:

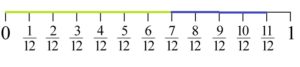
Question 5.

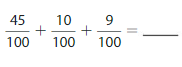
Answer:
Denominators are the same so add the numerators.
\(\frac{45}{100}\) + \(\frac{10}{100}\) + \(\frac{9}{100}\) = \(\frac{64}{100}\)

8.2 Decompose Fractions

Write the fraction as a sum of unit fractions.

Question 6.
\(\frac{2}{12}\)

Answer:
The unit fraction for \(\frac{2}{12}\) is \(\frac{1}{12}\) + \(\frac{1}{12}\)

Question 7.
\(\frac{3}{3}\)

Answer: The unit fraction for \(\frac{3}{3}\) is \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\)

Write the fraction as a sum of fractions in two different ways.

Question 8.
\(\frac{5}{8}\)

Answer:
The unit fraction for \(\frac{5}{8}\) is \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)

Question 9.
\(\frac{6}{100}\)

Answer:
The unit fraction for \(\frac{6}{100}\) is \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\) + \(\frac{1}{100}\)

Question 10.
\(\frac{90}{100}\)

Answer:
\(\frac{90}{100}\) = 9/10
The unit fraction for \(\frac{9}{10}\) is \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\) + \(\frac{1}{10}\)

Question 11.
\(\frac{4}{5}\)

Answer:
The unit fraction for \(\frac{4}{5}\) is \(\frac{1}{5}\) + \(\frac{1}{5}\) + \(\frac{1}{5}\) + \(\frac{1}{5}\)

8.3 Add Fractions with Like Denominators

Add

Question 12.

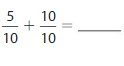
Answer:
Denominators are the same so add the numerators.
\(\frac{5}{10}\) + \(\frac{10}{10}\) = \(\frac{15}{10}\)

Question 13.

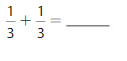
Answer:

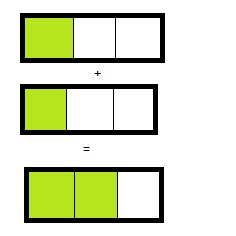
Denominators are the same so add the numerators.
\(\frac{1}{3}\) + \(\frac{1}{3}\) = \(\frac{2}{3}\)

Question 14.

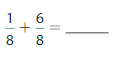
Answer:
Denominators are the same so add the numerators.
\(\frac{1}{8}\) + \(\frac{6}{8}\) = \(\frac{7}{8}\)

Question 15.

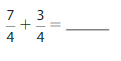
Answer:
Denominators are the same so add the numerators.
\(\frac{7}{4}\) + \(\frac{3}{4}\) = \(\frac{11}{4}\)

Question 16.

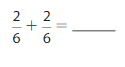
Answer:
Denominators are the same so add the numerators.
\(\frac{2}{6}\) + \(\frac{2}{6}\) = \(\frac{4}{6}\)

Question 17.

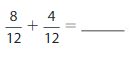
Answer:
Denominators are the same so add the numerators.
\(\frac{8}{12}\) + \(\frac{4}{12}\) = \(\frac{12}{12}\) = 1

Question 18.
Logic
When you add two of me you get \(\frac{100}{100}\). What fraction am I?

Answer: \(\frac{50}{100}\)
If you add \(\frac{50}{100}\) two times you get \(\frac{100}{100}\)
\(\frac{50}{100}\) + \(\frac{50}{100}\) = \(\frac{100}{100}\)

8.4 Use Models to Subtract Fractions

Find the difference. Explain how you used the model to subtract.

Question 19.

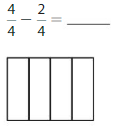
Answer:

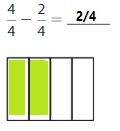
Question 20.

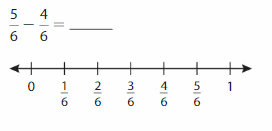
Answer:

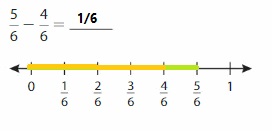
Find the difference. Use a model or a number line to help.

Question 21.

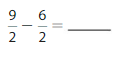
Answer: 3/2

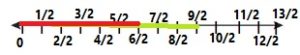
Question 22.

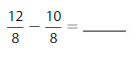
Answer:

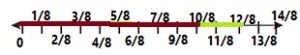
Question 23.

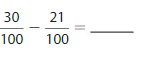
Answer:
Denominators are the same so subtract the numerators.
\(\frac{30}{100}\) – \(\frac{21}{100}\) = (30 – 21)/100 = \(\frac{9}{100}\)

Question 24.
Modeling Real Life
A football team wins \(\frac{7}{10}\) of their games this season. They lose \(\frac{3}{10}\) of their games. How many more games does the team win than lose?

Answer:
Given that,
A football team wins \(\frac{7}{10}\) of their games this season. They lose \(\frac{3}{10}\) of their games.
\(\frac{7}{10}\) – \(\frac{3}{10}\) = \(\frac{4}{10}\)
Thus \(\frac{4}{10}\) more games the team win than lose.

8.5 Subtract Fractions with Like Denominators

Subtract.

Question 25.

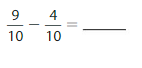
Answer: \(\frac{5}{10}\)

Explanation:
Denominators are the same so subtract the numerators.
\(\frac{9}{10}\) – \(\frac{4}{10}\) = \(\frac{5}{10}\)

Question 26.

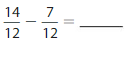
Answer: \(\frac{7}{12}\)

Explanation:
Denominators are the same so subtract the numerators.
\(\frac{14}{12}\) – \(\frac{7}{12}\) = \(\frac{7}{12}\)

Question 27.

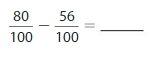
Answer: \(\frac{24}{100}\)

Explanation:
Denominators are the same so subtract the numerators.
\(\frac{80}{100}\) – \(\frac{56}{100}\) = \(\frac{24}{100}\)

Question 28.

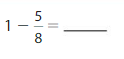
Answer: 3/8\(\frac{3}{8}\)

Explanation:
Denominators are the same so subtract the numerators.
1 can be written as \(\frac{8}{8}\)
\(\frac{8}{8}\) – \(\frac{5}{8}\) = \(\frac{3}{8}\)

Question 29

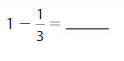
Answer: \(\frac{2}{3}\)

Explanation:
Denominators are the same so subtract the numerators.
1 can be written as \(\frac{3}{3}\)
\(\frac{3}{3}\) – \(\frac{1}{3}\) = \(\frac{2}{3}\)

Question 30.

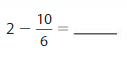
Answer: \(\frac{2}{6}\)

Explanation:
Denominators are the same so subtract the numerators.
2 can be written as \(\frac{12}{6}\)
\(\frac{12}{6}\) – \(\frac{10}{6}\) = \(\frac{2}{6}\)

8.6 Model Fractions and Mixed Numbers

Write the mixed number as a fraction.

Question 31.
1 \(\frac{6}{8}\)

Answer: \(\frac{7}{4}\)

Explanation:
Step 1
Multiply the denominator by the whole number
8 × 1 = 8
Step 2
Add the answer from Step 1 to the numerator
8 + 6 = 14
Step 3
Write an answer from Step 2 over the denominator
14/8 = \(\frac{7}{4}\)

Question 32.
4 \(\frac{1}{2}\)

Answer: \(\frac{9}{2}\)

Explanation:
Step 1
Multiply the denominator by the whole number
2 × 4 = 8
Step 2
Add the answer from Step 1 to the numerator
8 + 1 = 9
Step 3
Write an answer from Step 2 over the denominator
\(\frac{9}{2}\)

Question 33.
5 \(\frac{10}{12}\)

Answer: \(\frac{35}{6}\)

Explanation:
Step 1
Multiply the denominator by the whole number
12 × 5 = 60
Step 2
Add the answer from Step 1 to the numerator
60 + 10 = 70
Step 3
Write an answer from Step 2 over the denominator
70/12 = \(\frac{35}{6}\)

Write the fraction as a mixed number or a whole number.

Question 34.
\(\frac{17}{4}\)

Answer: 4 \(\frac{1}{4}\)

Explanation:
Converting from improper fraction to the mixed fraction.
\(\frac{17}{4}\) = 4 \(\frac{1}{4}\)

Question 35.
\(\frac{30}{6}\)

Answer: 5

Explanation:
Converting from improper fraction to the mixed fraction.
6 divides 30 five times.
So, \(\frac{30}{6}\) = 5

Question 36.
\(\frac{63}{10}\)

Answer: 6 \(\frac{3}{10}\)

Explanation:
Converting from improper fraction to the mixed fraction.
\(\frac{63}{10}\) = 63 ÷ 10
= 6 \(\frac{3}{10}\)

Compare.

Question 37.

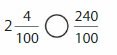
Answer: <

Explanation:
2 4/100
Step 1
Multiply the denominator by the whole number
100 × 2 = 200
Step 2
Add the answer from Step 1 to the numerator
200 + 4 = 204
Step 3
Write an answer from Step 2 over the denominator
204/100
240/100
Step 1
Multiply the denominator by the whole number
100 × 2 = 200
Step 2
Add the answer from Step 1 to the numerator
200 + 40 = 240
Step 3
Write an answer from Step 2 over the denominator
240/100
204/100 < 240/100

Question 38.

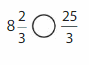
Answer: >

Explanation:
Step 1
Multiply the denominator by the whole number
3 × 8 = 24
Step 2
Add the answer from Step 1 to the numerator
24 + 2 = 26
Step 3
Write an answer from Step 2 over the denominator
26/3
26/3 > 25/3

Question 39.

Answer: =

Explanation:
25/5 = 5
5 = 5

Question 40.
Which One Doesn’t Belong? Which expression does not belong with the other three?

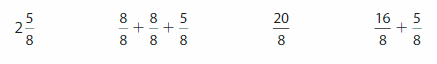
Answer: 20/8 does not belong with the other three.

8.7 Add Mixed Numbers

Add.

Question 41.

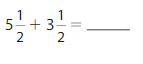
Answer: 9

Explanation:
Rewriting our equation with parts separated
=5+1/2+3+1/2
Solving the whole number parts
5+3=8
Solving the fraction parts
1/2+1/2=2/2
Reducing the fraction part, 2/2,
2/2=1/1
Simplifying the fraction part, 1/1,
1/1=1
Combining the whole and fraction parts
8+1=9

Question 42.

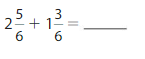
Answer: 4 1/3

Explanation:
Rewriting our equation with parts separated
=2+5/6+1+3/6
Solving the whole number parts
2+1=3
Solving the fraction parts
5/6+3/6 = 8/6
8/6 = 4/3
4/3 = 4 1/3

Question 43.

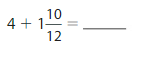
Answer: 5 5/6

Explanation:
Rewriting our equation with parts separated
=4+1+10/12
Solving the whole number parts
4+1=5
Combining the whole and fraction parts
5+10/12= 5 10/12 = 5 5/6

Question 44.

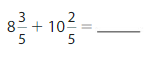
Answer: 19

Explanation:
Rewriting our equation with parts separated
=8+3/5+10+2/5
Solving the whole number parts
8+10=18
Solving the fraction parts
3/5+2/5=5/5
Simplifying the fraction part, 1/1,
1/1 = 1
Combining the whole and fraction parts
18+1=19

Question 45.

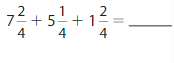
Answer: 14 1/4

Explanation:
Rewriting our equation with parts separated
=7+2/4+1+2/4
Solving the whole number parts
7+1=8
Solving the fraction parts
2/4+2/4=4/4
Reducing the fraction part, 4/4,
4/4=1/1
Simplifying the fraction part, 1/1,
1/1=1
Combining the whole and fraction parts
8+1=9
9 + 5 1/4
Rewriting our equation with parts separated
=9+5+1/4
Solving the whole number parts
9+5=14
Combining the whole and fraction parts
14+1/4=14 1/4

Question 46.

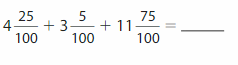
Answer: 19 5/100

Explanation:
Rewriting our equation with parts separated
=4+25/100+11+75/100
Solving the whole number parts
4+11=15
Solving the fraction parts
25/100+75/100=100/100
Reducing the fraction part, 100/100,
100/100=11
Simplifying the fraction part, 1/1,
1/1=1
Combining the whole and fraction parts
15+1=16
Rewriting our equation with parts separated
=16+3+5/100
Solving the whole number parts
16+3=19
Combining the whole and fraction parts
19+5/100=19 5/100

8.8 Subtract Mixed Numbers

Subtract

Question 47.

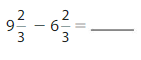
Answer: 3

Explanation:
Rewriting our equation with parts separated
=9+2/3-6-2/3
Solving the whole number parts
9−6=3
Solving the fraction parts
2/3−2/3=0/3
Simplifying the fraction part, 0/3,
0/3=0
Combining the whole and fraction parts
3+0=3

Question 48.

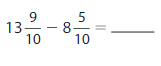
Answer: 5 2/5

Explanation:
Rewriting our equation with parts separated
=13+9/10−8−5/10
Solving the whole number parts
13−8=5
Solving the fraction parts
9/10−5/10=4/10
Reducing the fraction part, 4/10,
4/10=2/5
Combining the whole and fraction parts
5+2/5=5 2/5

Question 49.

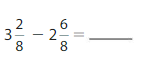
Answer: 1/2

Explanation:
Rewriting our equation with parts separated
=3+2/8−2−6/8
Solving the whole number parts
3−2=1
Solving the fraction parts
2/8−6/8=−4/8
Reducing the fraction part, 4/8,
−4/8=−1/2
Combining the whole and fraction parts
1−1/2=1/2

Question 50.

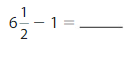
Answer: 5 1/2

Explanation:
6 + 1/2 – 1 = 5 1/2

Question 51.

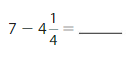
Answer: 2 3/4

Explanation:
Rewriting our equation with parts separated
=7−4−1/4
Solving the whole number parts
7−4=3
Combining the whole and fraction parts
3−1/4=2 3/4

Question 52.

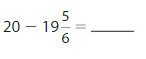
Answer: 1/6

Explanation:
Rewriting our equation with parts separated
=20−19−5/6
Solving the whole number parts
20−19=1
Combining the whole and fraction parts
1−5/6=1/6

8.9 Problem Solving: Fractions

Question 53.
You give \(\frac{3}{12}\) of your bag of grapes to one friend and \(\frac{5}{12}\) of your bag to another friend. What fraction of the bag of grapes do you have left?

Answer:
Given that,
You give \(\frac{3}{12}\) of your bag of grapes to one friend and \(\frac{5}{12}\) of your bag to another friend
\(\frac{3}{12}\) + \(\frac{5}{12}\) = \(\frac{8}{12}\)
\(\frac{12}{12}\) – \(\frac{8}{12}\) = \(\frac{4}{12}\)
Thus \(\frac{4}{12}\) fraction of the bag of grapes are left.

Final Words:

Hope you are all satisfied with the solutions provided in the BIM Grade 4 Chapter 8 Add and Subtract Fractions pdf. If you have any doubts regarding the problems you can ask your doubts in the below comment box. We are ready to clarify your doubts at any time. Stay with us to get the solutions of all 4th-grade chapters.