In geometry, the triangle is a three-sided polygon that has three sides, three vertices and three angles. Here we will discuss classifying triangles on the basis of their sides and angles. Interested students can get the different types of triangles and their definitions with images in the following sections.
Classifying Triangles
A triangle is a closed figure made up of three line segments intersecting at their endpoints. Each triangle has three sides, three vertices and three interior angles. According to triangle sum theory, the sum of interior angles in a triangle is 180 degrees. Triangles are classified into three types on the basis of sides and interior angles.
The types of triangles based on their sides are listed here:
- Equilateral Triangle
- Isosceles Triangle
- Scalene Triangle
The different types of triangles based on their interior angles are given here:
- Acute Triangle
- Right Triangle
- Obtuse Triangle
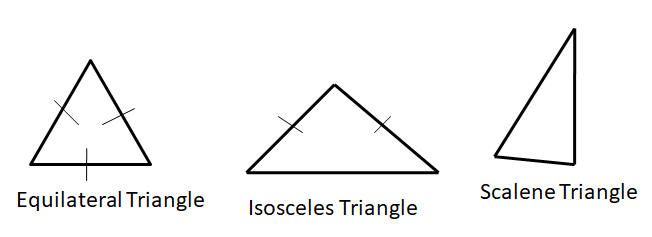
Types of Triangles Based on Their Sides
The three types of triangles based on their sides are equilateral triangle, scalene triangle and isosceles triangle. The definition and other properties of each of them are given here.
- Equilateral Triangle: A triangle that has all three sides of equal length, then it is called an equilateral triangle.
- Isosceles Triangle: A triangle that has any two sides of equal length, then it is called an isosceles triangle.
- Scalene Triangle: A triangle that has all three sides of different lengths, then it is called a scalene triangle.
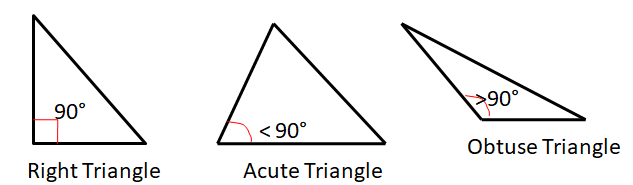
Classification of Triangles Based on Their Angles
The classification of triangles on the basis of interior angles are provided here.
- Acute Angles Triangle: It is a triangle that has all its angles are less than 90°.
- Right Angled Triangle: It is a triangle where one of its angles is equal to 90° or right angle.
- Obtuse Angled Triangle: It is a triangle that has any of its one angles more than 90°.
Types of Triangles Based on Sides, Angles
Triangles are classified into various types based on their angles, sides as follows:
- Equiangular or Equilateral Triangle: If all the sides and interior angles of a triangle are equal, then it is called an equilateral or equiangular triangle.
- Obtuse Isosceles Triangle: A triangle that has 2 sides of equal length and one angle is greater than 90 degrees is called an obtuse isosceles triangle.
- Acute Isosceles Triangle: A triangle that has one angle less than 90 degrees and any two sides of equal length is called an acute isosceles triangle.
- Isosceles Right Triangle: When a triangle contains any 2 sides of equal length, one angle is right, then it is called an isosceles right triangle.
- Right Scalene Triangle: A triangle that has all sides of different lengths, one angle is equal to 90 degrees is called a right scalene triangle.
- Obtuse Scalene Triangle: A triangle that has one angle obtuse and sides of different lengths is called an obtuse scalene triangle.
- Acute Scalene Triangle: A triangle contains 3 sides of different lengths, one angle is acute is called an acute scalene triangle.
The following are the important points that should be remembered while classifying the triangles.
- All triangles have two acute angles.
- The sum of internal angles is always 180 degrees.
- In an equilateral or equiangular triangle, each angle is 60 degrees.
Examples on Classification of Triangles
Example 1:
Identify the type of the triangle, if one of its angles is 100° based on the type of triangle.
Solution:
Given that, one of the angles in the triangle is 100° which is greater than 90°.
If a triangle has one of its angles more than 90° means it is an obtuse-angled triangle.
So, it is an obtuse triangle and the other two angles are acute angles.
Example 2:
The side lengths of a triangle are different. Identify the type of triangle.
Solution:
Given that, all the sides of a triangle are different.
If a triangle that has all three sides of different lengths is called a scalene triangle.
So, it is a scalene triangle.
FAQ’s on Triangle Classification
1. What are the 3 triangle classifications?
Based on the sides, the triangles are classified into 3 types. They are Equilateral Triangle, Scalene Triangle, Isosceles Triangle.
2. Mention the classification of triangle based on its angle?
A triangle is classified into 3 types based on its angle. They are Acute Triangle, Obtuse Triangle, Right Triangle.
3. Can an acute triangle be an equilateral triangle?
Yes, but all cute triangles are not equilateral triangles. Because acute triangle means it must have one angle less than 90 degrees and equilateral or equiangular triangle means all its angles are 60 degrees.
4. Which types of triangles have 3 lines of symmetry?
Equilateral triangles have 3 lines of symmetry as three lines of symmetry can pass through the vertex of this triangle.