I think you guys are familiar with the chapter coordinate geometry. We can see the distance formula in the coordinate geometry to find the distance between the coordinate points. In this article, we will learn what is distance formula is and the distance between two points. For example to find the distance between the lengths of the triangle in the coordinate plane we will use the distance formula. Scroll down this page to know more interesting points about the distance formula.
See More: Distance and Section Formulae
Distance Formula – Definition
Distance formula in coordinate geometry can be used to find the distance between two points, distance between two parallel lines, and distance between the points in a plane. The distance between the two parallel lines is the shortest distance from one point to the other point.
How do you find the Distance between the Points?
The distance between two points of the coordinate plane can be found using the distance formula. (x, y) are the ordered pairs that represent coordinates of the points. x-coordinate is the distance of the point from the x-axis and the y-coordinate is the distance of the point from the y-axis. We can find the distance between the points using the relevant formulas. We will find the distance between the two points in 2D and 3D planes using the Pythagoras theorem.
Derivation of Distance Formula
By using the Pythagoras theorem we can derive the distance formula.
AB² = AC² + BC²
d² = (x2 – x1)² + (y2 – y1)²
Squaring on both sides
d = √(x2 – x1)² + (y2 – y1)²
Hence proved
Distance Formula for two points
The distance formula for two points is used to find the distance between two points A(x1, y1) and B(x2, y2).
d = √(x2 – x1)² + (y2 – y1)²
where,
d = distance between the points
(x1, y1) and (x2, y2) are the ordered pairs of the two coordinate planes.
Distance Formula for Three Points
The distance formula for two points is used to find the distance between two points A(x1, y1, z1) and B(x2, y2, z3).
d = √(x2 – x1)² + (y2 – y1)² + (z2 – z1)²
where,
d = distance between the points
(x1, y1, z1) and (x2, y2, z2) are the ordered pairs of the two coordinate planes.
Distance from Point to Line
Consider Ax + By + C = 0 be an equation of a line and P be any point in the cartesian-coordinate plane having coordinates P(x1, y1).
PQ = |Ax1 + By1 + C|/√A² + B²
Distance between Two Parallel Lines
The distance between two parallel lines is equal to the Perpendicular distance between them.
let y = mx + c1 and y = mx + c2 be the two parallel lines
The formula to find the shortest distance between two non-intersecting lines is as follows
d = |c1 – c2|/√A² + B²
Distance Formula Examples
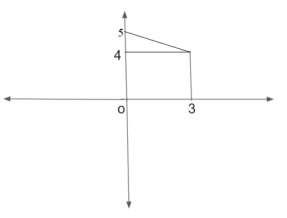
Example 1.
Find the distance between the points (3,4) and (0, 5)
Solution:
The formula for the distance D between two points (a,b) and (c,d) is given by
D = √(c – a)² + (d – b)²
Apply the formula given above to find distance D between the points (3,4) and(0,5) as follows.
D = √(0 – 3)² + (5 – 4)²
√(-3)² + (1)²
√4 + 1
√5 = 3.23
Example 2.
Find the distance point (2,4) and the midpoint of the line segment joining (1,3) and (4,5).
Solution:
We first find the coordinates of the midpoint M of the segment joining (2,3) and (4,5).
We know that
Midpoint formula = [a1 + a2/2 , b1 + b2/2]
M = [1 + 4/2, 3 + 5/2]
M = [5/2, 4]
We now use the distance formula to find the distance between the points (2,4) and (5/2,4)
D = √(a2 – a1)² + (b2 – b1)²
D = √(5/2 – 2)² + (4 – 4)²
D = √ (5/2 – 2)²
D = √(0.5)²
D = √0.25
D = 0.5.
Therefore the distance is 0.5
Example 3.
Find a relationship between x and y so that the triangle whose vertices are given by (x,y), (2,2) and (4,3) is a right triangle with the hypotenuse defined by the points (2,2) and (4,3).
Solution:
Let us use the distance formula to find the length of the hypotenuse.
h = √(a2 – a1)² + (b2 – b1)²
h = √(4 -2)² + (3 -2)²
h = √(2)² + (1)²
h = √4 + 1
h = √5 = 2.23
We now use the distance formula to find the sizes of the two other sides a and b of the triangle.
a = √(x – 2)² + (y – 2)²
b = √(x – 4)² + (y – 3)²
Pythagorean theorem gives
(2.23)² = (x – 2)² + (y – 2)² + (x – 4)² + (y – 3)²
Expand the squares, simply and complete the squares to rewrite the above relationship between x and y follows
(2.23)² = x² – 4x – 4 + y² – 4y + 4 + x² – 8x + 16 + y² – 6y + 9
(2.23)² = 2x² + 2y² – 12x – 10y + 25
2x² + 2y² – 12x – 10y = 4.97 – 25
2x² + 2y² – 12x – 10y = – 20.03
Example 4.
The town network is mapped on a coordinate grid with the origin being at city hall. Evans’s house is located at point (2,6) and Billy’s house is located at (1,4). How far is it from Evans’ house to Billy’s house?
Solution:
Using the distance formula
D = √(x2 – x1)² + (y2 – y1)²
D =√ (1 – 6)² + (4 – 6)²
D = √(-5)² + (-2)²
D = √25 + 4
D = √29
D = 5.38
Example 5.
Find a relationship between x and y so that the distance between the points (2,4) is equal to 6.
Solution:
We apply the distance formula
6 = √(2 – x)² + (4 – y)²
Square both sides
36 = (2 – x)² + (4 – y)²
The above relationship between x and y is the equation of the circle.
36 = 4 – 2x + x² + 16 – 8y + y²
36 = x² + y² – 2x – 8y + 20
x² + y² – 2x – 8y = 36 – 20
x² + y² – 2x – 8y = 16
FAQs on Distance Formula
1. What is the Distance Formula in Coordinate Geometry?
The distance formula is used to find the distance between two points A(x1, y1) and B(x2, y2).
d = √(x2 – x1)² + (y2 – y1)²
2. What is the distance between A and B?
The distance from A to B is the same as the distance from B to A. In order to derive the formula for the distance between two points in the plane, we consider two points A(a,b) and B(c,d).
3. What is the distance formula?
The distance formula is used to find the distance between two points A(x1, y1) and B(x2, y2).