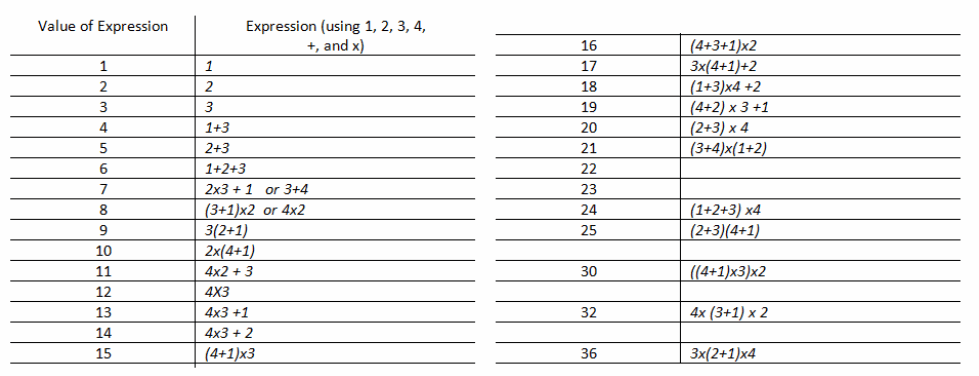
Engage NY Eureka Math Algebra 1 Module 1 Lesson 6 Answer Key
Eureka Math Algebra 1 Module 1 Lesson 6 Exercise Answer Key
Exercise 1.
The following is known as the 4-number game. It challenges students to write each positive integer as a combination of the digits 1, 2, 3, and 4; each used at most once, combined via the operations of addition and multiplication only, as well as grouping symbols. For example, 24 can be expressed as (1+3)(2+4). Students may use parentheses or not, at their own discretion (as long as their expressions evaluate to the given number, following the order of operations). Digits may not be juxtaposed to represent larger whole numbers, so the numerals 1 and 2 cannot be used to create the number 12 for instance.
Play the 4-number game as a competition within pairs. Give students three minutes to express the longest list of numbers they can, each written in terms of the digits 1, 2, 3, and 4. Students may want to use small dry erase boards to play this game or pencil and paper. Optionally, consider splitting up the tasks (e.g., 1–8, 9–20, 21–30, 31–36) and assign them to different groups.
Below are some sample expressions students may build and a suggested structure for displaying possible expressions on the board as students call out what they have created.
When reviewing the game, it is likely that students will have different expressions for the same number (see answers to rows 7 and 8 given below). Share alternative expressions on the board, and discuss as a class the validity of the expressions.
Challenge students to come up with more than one way to create the number 21.
After students share their results, ask these questions:
→ What seems to be the first counting number that cannot be created using only the numbers 1, 2, 3, and 4 and the operations of multiplication and addition?
→ 22
→ What seems to be the largest number that can be made in the 4-number game?
→ (1+2)(3)(4)=36
We can now launch into an interesting investigation to find structure in the game.
→ Suppose we were playing the 2-number game. What seems to be the largest number you could be create using the numbers 1 and 2 (each at most once) and the operations of multiplication and addition?
→ (1+2)=3
→ Suppose we were playing the 3-number game. What seems to be the largest number that could created using the numbers 1, 2, and 3 (each at most once) and the operations of multiplication and addition?
→ (1+2)(3) = 9
Encourage students to generalize the pattern for the 5-number game and the N-number game and think about why this pattern for an expression gives the largest attainable number.
→ Add 1 and 2, and then multiply the remaining numbers. For the 5-number game the largest number would be (1+2)(3)(4)(5)=180. For the N-number game, the largest number would be
(1+2)(3)(4)(5)…(n).
Exercises
Exercise 2.
Using the numbers 1, 2, 3, 4 only once and the operations + or × as many times as you like, write an expression that evaluates to 16. Use this expression and any combination of those symbols as many times as you like to write an expression that evaluates to 816.
Answer:
(4+3+1)×2
((4+3+1)×2)((4+3+1)×2+(4+3+1)×2+(4+3+1)×2)+(4+3+1)×2 +(4+3+1)×2+(4+3+1)×2
Exercise 3.
Define the rules of a game as follows:
a. Begin by choosing an initial set of symbols, variable or numeric, as a starting set of expressions.
Answer:
3, x, y, and a
b. Generate more expressions by placing any previously created expressions into the blanks of the addition operator: ______+ _______.
Answer:
3+a or x+y or y+3 or a+a
→ Let’s play the game using 3, x, y, and a as our set of starting expressions.
Write the symbols to be used on the board. (These are not provided in the student materials.)
→ Can you see that:
Part (1) of the game gives us 3, x, y, and a to start.
Part (2) gives us expressions, such as 3+a or x+y or y+3 or a+a.
Repeated use of part (2) then gives (3+a)+3 or x+(x+y) and (x+y)+(x+y),
and then ((x+y)+(x+y))+a for example.
Make sure students are clear that in this version of the game, we are going to limit ourselves to addition (no multiplication).
→ Take 1 minute to generate as many expressions as possible, following the rules of the game. (Time students for 1 minute.)
→ Compare your list with your neighbor. Did your neighbor follow the rules of the game?
→ If the rules are followed, strings of sums are generated. Is that correct?
→ Is it possible to create the expression 4x+5y+3a from the game?
Ask a student to verbally describe how he did it and show the string of sums on the board.
(((x+y)+(x+y))+((x+y)+(x+y)))+((y+a)+(a+a))
Exercise 4.
Roma says that collecting like terms can be seen as an application of the distributive property. Is writing x+x=2x an application of the distributive property?
Answer:
Roma is correct. By the distributive property, we have
x+x=1∙x+1∙x=(1+1)x=2x.
Exercises 5–7 (7 minutes)
Have students work the following exercises one at a time, taking time to discuss the solutions between each one.
Exercise 5.
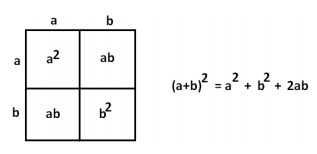
Leela is convinced that (a+b)2=a2+b2. Do you think she is right? Use a picture to illustrate your reasoning.
Answer:
Leela is not right.
Exercise 6.
Draw a picture to represent the expression (a+b+1)×(b+1).
Answer:
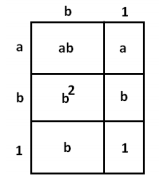
A good discussion to have with students is whether it is important that the length of 1 in the width be the same as the length of 1 in the height. Continue the discussion as to whether how the picture is labeled is important to representing the quantities.
Exercise 7.
Draw a picture to represent the expression (a+b)×(c+d)×(e+f+g).
Answer:
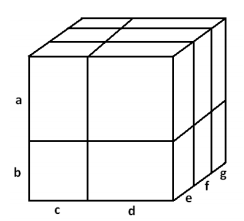
Eureka Math Algebra 1 Module 1 Lesson 6 Problem Set Answer Key
Question 1.
Insert parentheses to make each statement true.
a. 2+3×42+1=81
Answer:
(2+3)×42+1=81
b. 2+3×42+1=85
Answer:
(2+3)×(42+1)=85
c. 2+3×42+1=51
Answer:
2+(3×42 )+1=51 (or no parentheses at all is acceptable as well)
d. 2+3×42+1=53
Answer:
2+3×(42+1)=53
Question 2.
Using starting symbols of w, q, 2, and -2, which of the following expressions will NOT appear when following the rules of the game played in Exercise 3?
a. 7w+3q+(-2)
b. q-2
c. w-q
d. 2w+6
e. -2w+2
Answer:
The expressions in parts (c) and (e) cannot be obtained by following the rules of the game played in Exercise 3. The expression in part (d) appears as w+w+2+2+2, which is equivalent to 2w+6.
Question 3.
Luke wants to play the 4-number game with the numbers 1, 2, 3, and 4 and the operations of addition, multiplication, AND subtraction.
Leoni responds, “Or we just could play the 4-number game with just the operations of addition and multiplication, but now with the numbers -1, -2, -3, -4, 1, 2, 3, and 4 instead.”
What observation is Leoni trying to point out to Luke?
Answer:
Subtraction can be viewed as the addition of a negative (e.g., x-4=x+(-4)). By introducing negative integers, we need not consider subtraction as a new operation.
Question 4.
Consider the expression: (x+3)∙(y+1)∙(x+2).
a. Draw a picture to represent the expression.
Answer:
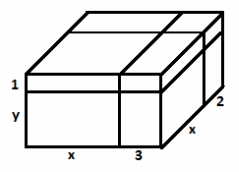
b. Write an equivalent expression by applying the distributive property.
Answer:
(y+1)(x+3)(x+2)= x2 y+5xy+6y+x2+5x+6
Question 5.
a. Given that a>b, which of the shaded regions is larger and why?
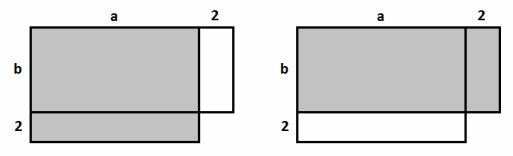
Answer:
The shaded region from the image on the left is larger than the shaded region from the image on the right. Both images are made up of the region of area a×b plus another region of either 2a (for the image on the left) or 2b (for the image on the right). Since a>b, 2a>2b.
b. Consider the expressions 851×29 and 849×31. Which would result in a larger product? Use a diagram to demonstrate your result.
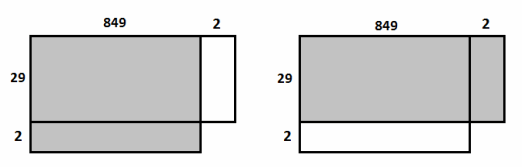
Answer:
851∙29 can be written as (849+2)29=849∙29+2∙29 and
849∙31 can be written as 849(29+2)=849∙29+2∙849.
Since 2∙29<2∙849, the product 849∙31 is the larger product.
Question 6.
Consider the following diagram.
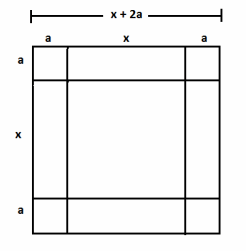
Edna looked at the diagram and then highlighted the four small rectangles shown and concluded:
(x+2a)2=x2+4a(x+a).
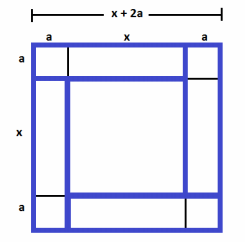
a. Michael, when he saw the picture, highlighted four rectangles and concluded:
(x+2a)2=x2+2ax+2a(x+2a).
Which four rectangles and one square did he highlight?
Answer:
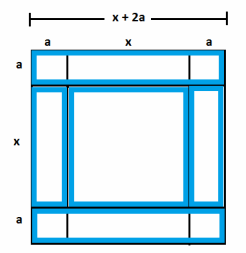
b. Jill, when she saw the picture, highlighted eight rectangles and squares (not including the square in the middle) to conclude:
(x+2a)2=x2+4ax+4a2.
Which eight rectangles and squares did she highlight?
Answer:
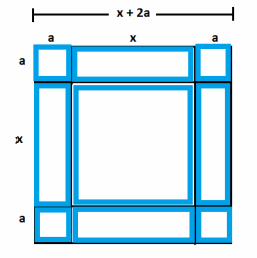
c. When Fatima saw the picture, she concluded: (x+2a)2=x2+4a(x+2a)-4a2. She claims she highlighted just four rectangles to conclude this. Identify the four rectangles she highlighted, and explain how using them, she arrived at the expression x2+4a(x+2a)-4a2.
Answer:
She highlighted each of the four rectangles that form a rim around the inner square. In doing so, she double counted each of the four a×a corners and, therefore, needed to subtract 4a2.
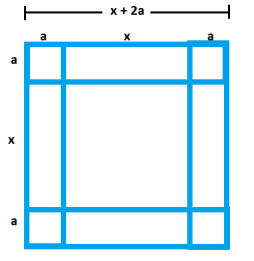
b. Is each student’s technique correct? Explain why or why not.
Answer:
Yes, all of the techniques are right. You can see how each one is correct using the diagrams. Students broke the overall area into parts and added up the parts. In Fatima’s case, she ended up counting certain areas twice and had to compensate by subtracting those areas back out of her sum.
Eureka Math Algebra 1 Module 1 Lesson 6 Exit Ticket Answer Key
Consider the expression: (x+y+3)×(y+1).
Question 1.
Draw a picture to represent the expression.
Answer:
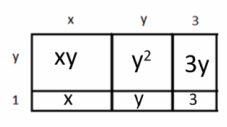
Question 2.
Write an equivalent expression by applying the distributive property.
Answer:
(x+y+3)×(y+1)=xy+ x+y2+4y+3