Eureka Math Algebra 1 Module 3 Lesson 7 Answer Key

Engage NY Eureka Math Algebra 1 Module 3 Lesson 7 Answer Key

Eureka Math Algebra 1 Module 3 Lesson 7 Example Answer Key

Example 1.
a. Malik bought a new car for $15,000. As he drove it off the lot, his best friend, Will, told him that the car’s value just dropped by 15% and that it would continue to depreciate 15% of its current value each year. If the car’s value is now $12,750 (according to Will), what will its value be after 5 years?
Complete the table below to determine the car’s value after each of the next five years. Round each value to the nearest cent.

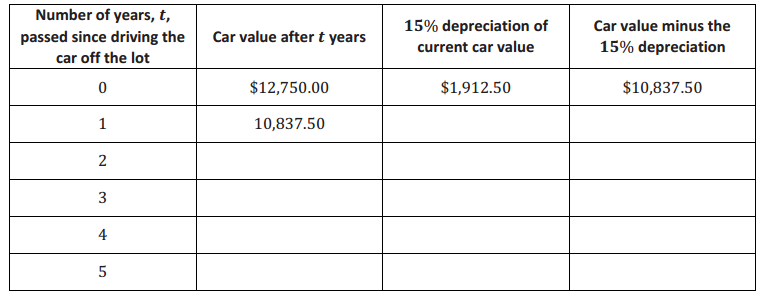
Answer:

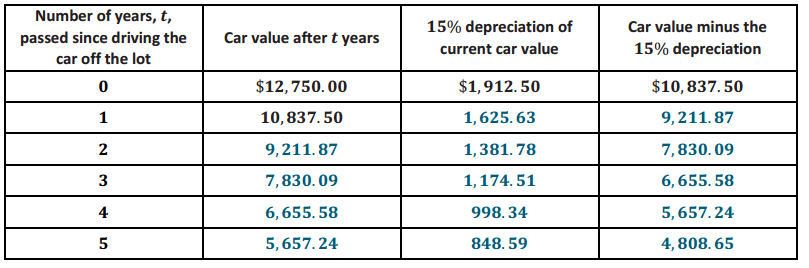
b. Write an explicit formula for the sequence that models the value of Malik’s car t years after driving it off the lot.
Answer:
v(t) = 12750(0.85)^t

c. Use the formula from part (b) to determine the value of Malik’s car five years after its purchase. Round your answer to the nearest cent. Compare the value with the value in the table. Are they the same?
Answer:
v(t) = 12750(0.85)⁵ ≈ 5657.24 It is approximately the same value. Note that small differences could be attributed to rounding.

d. Use the formula from part (b) to determine the value of Malik’s car 7 years after its purchase. Round your answer to the nearest cent.
Answer:
v(t) = 12750(0.85)⁷ ≈ 4087.36

Eureka Math Algebra 1 Module 3 Lesson 7 Exercise Answer Key

Exercises 1–6

Exercise 1.
Identify the initial value in each formula below, and state whether the formula models exponential growth or exponential decay. Justify your responses.
a. f(t) = 2(\(\frac{2}{5}\))^t
Answer:
a = 2; decay; b < 1

b. f(t) = 2(\(\frac{5}{3}\))^t
Answer:
a = 2; growth; b > 1

c. f(t) = \(\frac{2}{3}\)(3)^t
Answer:
a = \(\frac{2}{3}\); growth; b > 1

d. f(t) = \(\frac{2}{3}\) (\(\frac{1}{3}\))^t
Answer:
a = \(\frac{2}{3}\); decay; b < 1

e. f(t) = \(\frac{3}{2}\) (\(\frac{2}{3}\))^t
Answer:
a = \(\frac{3}{2}\); decay; b < 1

Exercise 2.
If a person takes a given dosage d of a particular medication, then the formula f(t) = d(〖0.8)〗^t represents the concentration of the medication in the bloodstream t hours later. If Charlotte takes 200 mg of the medication at 6:00 a.m., how much remains in her bloodstream at 10:00 a.m.? How long does it take for the concentration to drop below 1 mg?
Answer:
81.92 mg of the medication remains in her bloodstream at 10:00 a.m.; it would take about 24 hours to drop below 1 mg.
Note: Expect students to arrive at the estimate of 24 hours using a guess – and – check procedure.

Exercise 3.
When you breathe normally, about 12% of the air in your lungs is replaced with each breath. Write an explicit formula for the sequence that models the amount of the original air left in your lungs, given that the initial volume of air is 500 ml. Use your model to determine how much of the original 500 ml remains after 50 breaths.
Answer:
a(n) = 500(1 – 0.12)ⁿ, where n is the number of breaths. After 50 breaths, only 0.83 ml of the original 500 ml remains in your lungs.

Exercise 4.
Ryan bought a new computer for $2,100. The value of the computer decreases by 50% each year. When will the value drop below $300?
Answer:
After 3 years, the value will be $262.50.

Exercise 5.
Kelli’s mom takes a 400 mg dose of aspirin. Each hour, the amount of aspirin in a person’s system decreases by about 29%. How much aspirin is left in her system after 6 hours?
Answer:
51 mg

Exercise 6.
According to the International Basketball Association (FIBA), a basketball must be inflated to a pressure such that when it is dropped from a height of 1,800 mm, it rebounds to a height of 1,300 mm. Maddie decides to test the rebound – ability of her new basketball. She assumes that the ratio of each rebound height to the previous rebound height remains the same at \(\frac{1300}{1800}\). Let f(n) be the height of the basketball after n bounces. Complete the chart below to reflect the heights Maddie expects to measure.

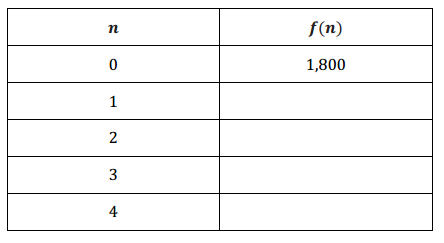
Answer:

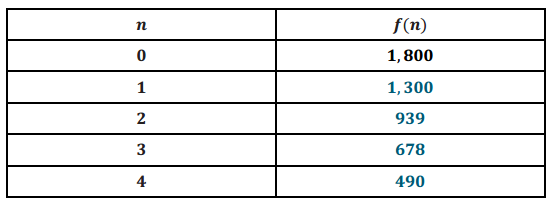
a. Write the explicit formula for the sequence that models the height of Maddie’s basketball after any number of bounces.
Answer:
f(n) = 1800(\(\frac{13}{18}\))ⁿ

b. Plot the points from the table. Connect the points with a smooth curve, and then use the curve to estimate the bounce number at which the rebound height drops below 200 mm.

Answer:

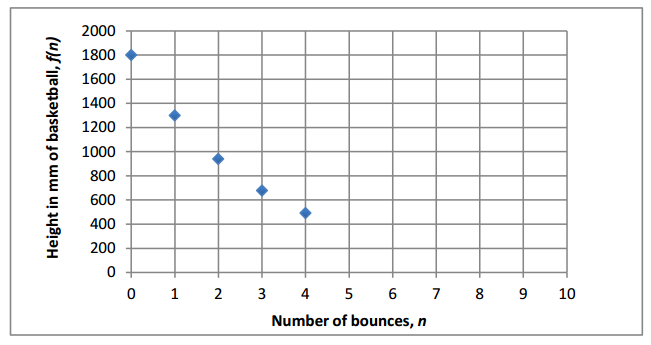
At the seventh rebound, the rebound height falls below 200 mm.

Eureka Math Algebra 1 Module 3 Lesson 7 Problem Set Answer Key

Question 1.
From 2000 to 2013, the value of the U.S. dollar has been shrinking. The value of the U.S. dollar over time (v(t)) can be modeled by the following formula:
v(t) = 1.36(0.9758)^t, where t is the number of years since 2000

a. How much was a dollar worth in the year 2005?
Answer:
$1.20

b. Graph the points (t, v(t)) for integer values of 0 ≤ t ≤ 14.
Answer:

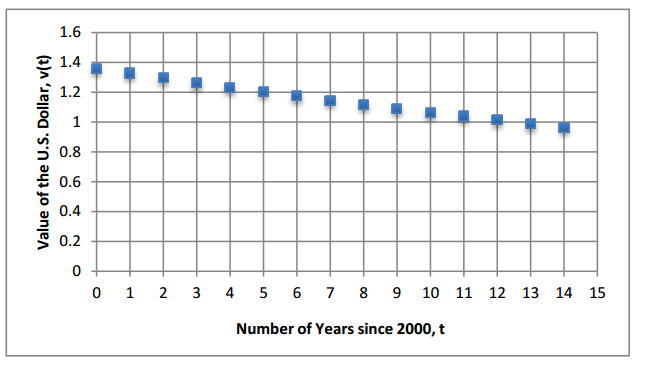
c. Estimate the year in which the value of the dollar fell below $1.00.
Answer:
2013

Question 2.
A construction company purchased some equipment costing $300,000. The value of the equipment depreciates (decreases) at a rate of 14% per year.
a. Write a formula that models the value of the equipment each year.
Answer:
v(t) = 300 000(0.86)^t, where t is the number of years after the purchase

b. What is the value of the equipment after 9 years?
Answer:
$77,198

c. Graph the points (t, v(t)) for integer values of 0 ≤ t ≤ 15.
Answer:

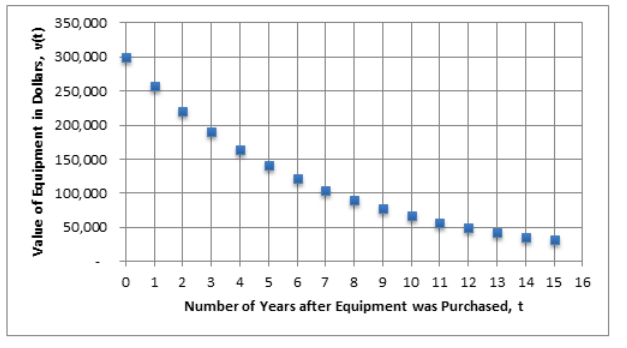
d. Estimate when the equipment will have a value of $50,000.
Answer:
After 12 years

Question 3.
The number of newly reported cases of HIV (in thousands) in the United States from 2000 to 2010 can be modeled by the following formula:
f(t) = 41(0.9842)^t, where t is the number of years after 2000

a. Identify the growth factor.
Answer:
0.9842

b. Calculate the estimated number of new HIV cases reported in 2004.
Answer:
38,470

c. Graph the points (t, f(t)) for integer values of 0 ≤ t ≤ 10.
Answer:

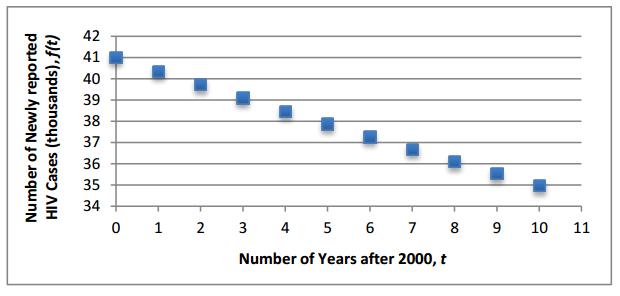
d. During what year did the number of newly reported HIV cases drop below 36,000?
Answer:
2009

Question 4.
Doug drank a soda with 130 mg of caffeine. Each hour, the caffeine in the body diminishes by about 12%.
a. Write a formula to model the amount of caffeine remaining in Doug’s system each hour.
Answer:
c(t) = 130(0.88)^t, where t is the number of hours after Doug drinks the beverage

b. How much caffeine remains in Doug’s system after 2 hours?
Answer:
101 mg

c. How long will it take for the level of caffeine in Doug’s system to drop below 50 mg?
Answer:
8 hours

Question 5.
64 teams participate in a softball tournament in which half the teams are eliminated after each round of play.
a. Write a formula to model the number of teams remaining after any given round of play.
Answer:
t(n) = 64(0.5)ⁿ, where n is the number of rounds played

b. How many teams remain in play after 3 rounds?
Answer:
8 teams

c. How many rounds of play will it take to determine which team wins the tournament?
Answer:
6 rounds

Question 6.
Sam bought a used car for $8,000. He boasted that he got a great deal since the value of the car two years ago (when it was new) was $15,000. His friend, Derek, was skeptical, stating that the value of a car typically depreciates about 25% per year, so Sam got a bad deal.
a. Use Derek’s logic to write a formula for the value of Sam’s car. Use t for the total age of the car in years.
Answer:
v(t) = 15000(0.75)^t

b. Who is right, Sam or Derek?
Answer:
Sam is right. According to Derek’s formula, the value of Sam’s car after two years is $8,437.50. If Sam paid only $8,000 for the car, he did get a great deal.

Eureka Math Algebra 1 Module 3 Lesson 7 Exit Ticket Answer Key

Question 1.
A huge Ping – Pong tournament is held in Beijing with 65,536 participants at the start of the tournament. Each round of the tournament eliminates half the participants.
a. If p(r) represents the number of participants remaining after r rounds of play, write a formula to model the number of participants remaining.
Answer:
p(r) = 65536(\(\frac{1}{2}\))^r

b. Use your model to determine how many participants remain after 10 rounds of play.
Answer:
64 participants remain after 10 rounds.

c. How many rounds of play will it take to determine the champion Ping – Pong player?
Answer:
It will take a total of 16 rounds to eliminate all but one player.