Eureka Math Algebra 2 Module 3 Lesson 19 Answer Key

Engage NY Eureka Math Algebra 2 Module 3 Lesson 19 Answer Key

Eureka Math Algebra 2 Module 3 Lesson 19 Opening Exercise Answer Key

Opening Exercise:

a. Consider the mapping diagram of the function f below. Fill in the blanks of the mapping diagram of g to construct a function that undoes each output value of f by returning the original input value of f. (The first one is done for you.)

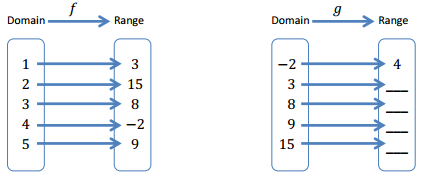
Answer:
1, 3, 5, 2

b. Write the set of Input-output paWs for the functions f and g filling the blanks below. (The set?’ for the function f has been done for you.)
Answer:
F = {(1, 3), (2, 15), (3, 8), (4, -2), (5, 9)}
G = {(-2, 4), (3, 1), (8, 3), (9, 5), (15, 2)}

c. How can the points in the set G be obtained from the points in F?
Answer:
The points in G can be obtained from the points in F by switching the first entry (first coordinate) with the second entry (second coordinate) that is, if (a, b) is a point of F, then (b, a) is a point of G.

d. Peter studied the mapping diagrams of the functions f and g above and exclaimed, “I can get the mapping diagram for g by simply taking the mapping diagram for f and reversing all of the arrows!” Is he correct?
Answer:
He is almost correct. It is true that he can reverse the arrows, but he would also need to switch the domain and range labels to reflect that the range of f is the domain of g, and the domain of f is the range of 9.

Eureka Math Algebra 2 Module 3 Lesson 19 Exercise Answer Key

Exercises:

For each function f in Exercises 1 – 5, find the formula for the corresponding inverse function g. Graph both functions on a calculator to check your work.

Exercise 1.
f(x) = 1 – 4x
Answer:

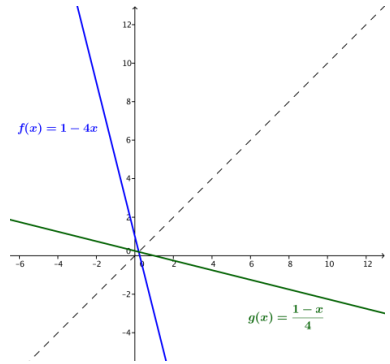
y = 1 – 4x
x = 1 – 4y
4y = 1 – x
y = \(\frac{(1-x)}{4}\)
g(x) = \(\frac{(1-x)}{4}\)

Exercise 2.
f(x) = x³ – 3
Answer:

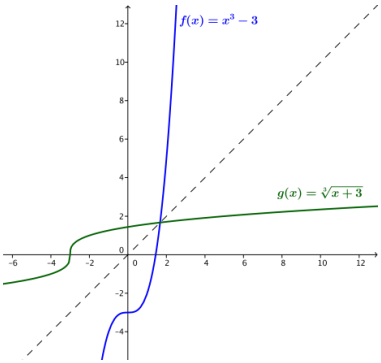
y = x³ – 3
x = y³ – 3
y³ = x + 3
y = \(\sqrt[3]{x+3}\)
g(x) = \(\sqrt[3]{x+3}\)

Exercise 3.
f(x) = 3log(x²) for x > 0
Answer:

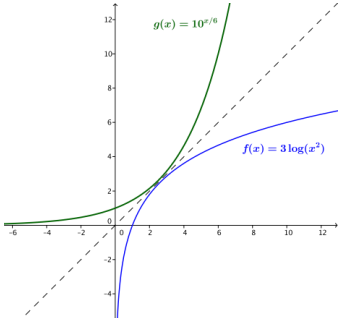
y = 3 log(x²)
x = 3 log(y²)
x = 3 . 2 log(y)
log(y) = \(\frac{x}{6}\)
y = 10^{\(\frac{x}{6}\)}
g(x) = 10^{\(\frac{x}{6}\)}

Exercise 4.
f(x) =
Answer:

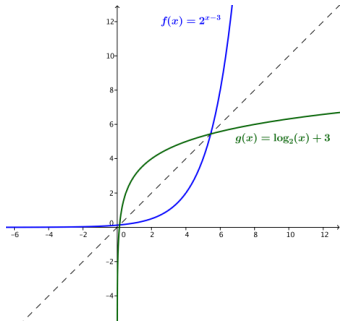
y = 2^{x – 3}
x = 2^{y – 3}
log₂(x) =y – 3
y = log₂(x) + 3
g(x) = log₂(x) + 3

Exercise 5.
f(x) = \(\frac{x+1}{x-1}\) for x ≠ 1
Answer:

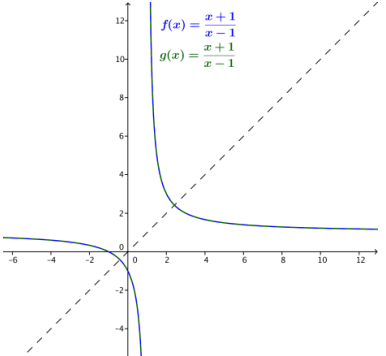
y = \(\frac{x+1}{x-1}\)
x = \(\frac{x+1}{x-1}\)
x(y – 1) = y + 1
xy – x = y + 1
xy – y = x + 1
y(x – 1) = x + 1
y = \(\frac{x+1}{x-1}\)
g(x) = \(\frac{x+1}{x-1}\) for x ≠ 1

Exercise 6.
Cindy thinks that the inverse of f(x) = x – 2 is g(x) = 2 – x. To justify her answer, she calculates f(2) = 0 and then substitutes the output 0 into g to get g(0) = 2, which gives back the original input. Show that Cindy is incorrect by using other examples from the domain and range of f.
Answer:
Answers will vary, but any point other than 2 works. For example, f(3) = 1, but g(1) = 1, not 3 as needed.

Exercise 7.
After finding the inverse for several functions, Henry claims that every function must have an inverse. R0ihanna says that his statement is not true and came up with the following example: If f(x) = |x| has an inverse, then because f(3) and f(-3) both have the same output 3, the inverse function g would have to map 3 to both 3 and -3 simultaneously, which violates the definition of a function. What is another example of a function without an inverse?
Answer:
Answers will vary. Any even degree polynomial function, such as f(x) = x², does not have an inverse.

Eureka Math Algebra 2 Module 3 Lesson 19 Example Answer Key

Example 1.
Consider the function f(x) = 2^x + 1, whose graph is shown to the right.

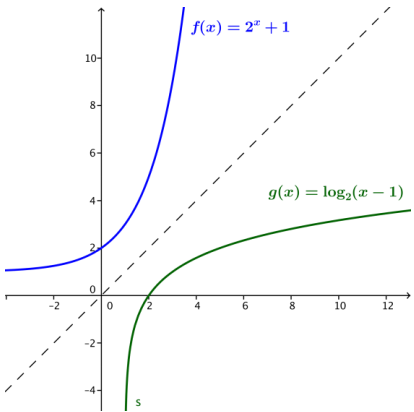
a. What are the domain and range of f?
Answer:
Since the function h(x) = 2x has domain all real numbers and range (0, ∞), we know that the translated function f(x) = 2x + 1 has domain all real numbers and range (1, ∞).

b. Sketch the graph of the inverse function g on the graph. What type of function do you expect g to be?
Answer:
Since logarithmic and exponential functions are inverses of each other, g should be some form of a logarithmic function (shown in green).

c. What are the domain and range of g? How does that relate to your answer in part (a)?
Answer:
The range of g is all real numbers, and the domain of g is (1, ∞), which makes sense since the range of g is the domain of f, and the domain of g is the range of f.

d. Find the formula for g.
Answer:
y = 2^x + 1
x = 2^y + 1
2^y = x – 1
y = log₂(x – 1)
g(x) = log₂(x – 1), for x > 1

Eureka Math Algebra 2 Module 3 Lesson 19 Problem Set Answer

Question 1.
For each function h below, find two functions f and g such that h(x) = f(g(x)). (There are many correct answers.)

a. h(x) = (3x+7)²
Answer:
Possible answer: f(x) = x², g(x) = 3x + 7

b. h(x) = \(\sqrt[3]{x^{2}-8}\)
Answer:
Possible answer: f(x) = \(\sqrt[3]{x}\) g(x) = x² – 8

c. h(x) = \(\frac{1}{2 x-3}\)
Answer:
Possible answer: f(x) = \(\frac{1}{x}\), g(x) = 2x – 3

d. h(x) = \(\frac{4}{(2 x-3)^{3}}\)
Answer:
Possible answer: f(x) = \(\frac{4}{x^{3}}\), g(x) = 2x – 3.

e. h(x) = (x+1)² + 2(x + 1)
Answer:
Possible answer: f(x) = x² + 2x, g(x) = x + 1

f. h(x) = (x + 4)^{\(\frac{4}{5}\)}
Answer:
Possible answer: f(x) = x^{\(\frac{4}{5}\)}, g(x) = x + 4

g. h(x) = \(\sqrt[3]{\log \left(x^{2}+1\right)}\)
Answer:
Possible answer: f(x) = \(\sqrt[3]{\log (x)}\), g(x) = x² + 1

h. h(x) = sin(x² + 2)
Answer:
Possible answer: f(x) = sin(x), g(x) = x² + 2

I. h(x) = ln(sin(x))
Answer:
Possible answer: f(x) = ln(x), g(x) = sin(x)

Question 2.
Let f be the function that assigns to each student in your class his or her biological mother.

a. Use the definition of function to explain why f is a function.
Answer:
The function has a well-defined domain (students in the class) and range (their mothers), and each student is assigned one and only one biological mother.

b. In order for (to have an inverse, what condition must be true about the students in your class?
Answer:
If a mother has several children in the same classroom, then there would be no way to define an inverse function that picks one and only one student for each mother. The condition that must be true is that there are no siblings in the class.

c. If we enlarged the domain to include all students in your school, would this larger domain function have an inverse?
Answer:
Probably not. Most schools have several students who are siblings.

Question 3.
The table below shows a partially filled-out set of Input-output pairs for two functions (and h that have the same finite domain of {0, 5, 10, 15, 20, 25, 30, 35, 40}.

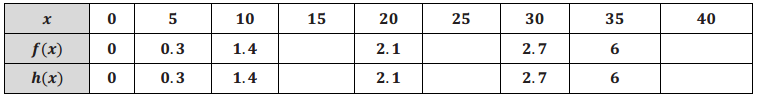
Answer:
a. Complete the table so that f is invertible but h is definitely not invertible.
Answer:
Answers will vary. For f, all output values should be different. For h, at least two output values for two different inputs should be the same number.

b. Graph both functions and use their graphs to explain why f is invertible and h is not.
Answer:
Answers will vary. The graph off has one unique output for every input, so it is possible to undo f and map each of its outputs to a unique input The graph of h has at least two input values that map to the same output value. Hence, there is no way to map that output value back to a unique multiple of 5. Hence, h cannot have an inverse function because such a correspondence is not a function.

Question 4.
Find the inverse of each of the following functions. In each case, indicate the domain and range of both the original function and its inverse.

a. f(x) = \(\frac{3 x-7}{5}\)
Answer:
x = \(\frac{3 y-7}{5}\)
5x = 3y – 7
\(\frac{5 x+7}{3}\) = y

The inverse function is g(x) = \(\frac{5 x+7}{3}\). Both functions f and g have a domain and range of all real numbers.

b. f(x) = \(\frac{5+x}{6-2 x}\)
Answer:
x = \(\frac{5+y}{6-2 y}\)
6x – 2yx = 5 + y
6x – 5 = 2yx + y
6x – 5 = (2x + 1)y
\(\frac{6 x-5}{2 x+1}\) = y
The inverse function as g(x) = \(\frac{6 x-5}{2 x+1}\).
Domain off and range of g: all real numbers x with x ≠ 3
Range off and domain of g: all real numbers x with x ≠ –\(\frac{1}{2}\)

c. f(x) = e^{x – 5}
Answer:
x = e^{y – 5}
ln(x) = y – 5
In(x) + 5 = y
The Inverse function is g(x) = ln(x) + 5.
Domain of f and range of g: all real numbers x
Range of f and domain of g: all real numbers x with x > 0.

d. f(x) = 2^{5 – 8x}
Answer:
x = 2^{5 – 8y}
log₂(x)= 5 – 8y
8y = 5 – log₂(x)
y = \(\frac{1}{8}\)(5 – log₂(x))
The inverse function is g(x) = \(\frac{1}{8}\)(5 – log₂((x)).
Domain of f and range of g: all real numbers x
Range off and domain of g: all real numbers x with x > 0.

e. f(x) = 7log(1 + 9x)
Answer:
x = 7log(1 + 9y)
\(\frac{x}{7}\) = log(1 + 9y)
10^{\(\frac{x}{7}\)} = 1 + 9y
\(\frac{1}{9}\)(10^{\(\frac{x}{7}\)}) = y
The inverse function is g(x) = \(\frac{1}{9}\)(10^{\(\frac{x}{7}\)})
Domain of f and range of g: all real numbers x with x > \(\frac{1}{9}\)
Range off and domain of g: all real numbers x

f. f(x) = 8+ ln(5 + \(\sqrt[3]{x}\))
Answer:
x = 8 + ln(5 + \(\sqrt[3]{y}\))
x – 8 = ln(5 + \(\sqrt[3]{y}\))
e^{x – 8} = 5 + \(\sqrt[3]{y}\)
e^{x – 8} – 5 = \(\sqrt[3]{y}\)
(e^{x – 8} – 5)³ = y
The inverse function is g(x) = (e^{x – 8} – 5)³
Domain off and range of g: all real numbers x with x > -125
Range of f and domain of g: all real numbers x.

g. f(x) = log\(\left(\frac{100}{3 x+2}\right)\)
Answer:
x = log\(\left(\frac{100}{3 y+2}\right)\)
x = log(100) – log(3y + 2)
x = 2 – log(3y + 2)
2 – x = log(3y + 2)
10^{2 – x} = 3y + 2
\(\frac{1}{3}\)(10^{2 – x} – 2) = y
The inverse function is g(x) = \(\frac{1}{3}\)(10^{2 – x} – 2).
Domain of f and range of g: all real numbers x with x > – \(\frac{2}{3}\)
Range of f and domain of g: all real numbers x

h. f(x) = ln(x) – ln(x + 1)
Answer:
x = ln(y) – ln(y + 1)
x = ln(\(\frac{y}{y+1}\))
e^x = \(\frac{y}{y+1}\)
ye^x + e^x = y
ye^x – y = -e^x
y(e^x – 1) = -e^x
y = \(\frac{e^{x}}{1-e^{x}}\)
The inverser function is g(x) = \(\frac{e^{x}}{1-e^{x}}\)
Domain of f and range of g: all real numbers x with x >0
Range of f and domain of g: all real numbers x < 0.

i. f(x) = \(\frac{2^{x}}{2^{x}+1}\)
Answer:
x = \(\frac{2^{y}}{2^{y}+1}\)
x2^y + x = 2^y
x 2^y – 2^y = -x
2^y = \(\frac{-x}{x-1}=\frac{x}{1-x}\)
yln(2) = ln(\(\frac{x}{1-x}\)
y = ln\(\frac{\left(\frac{x}{1-x}\right)}{\ln (2)}\)
The inverse function is g(x) = ln\(\frac{\left(\frac{x}{1-x}\right)}{\ln (2)}\)
Domain of f and range of g: all real numbers x
Range of f and domain of g: all real numbers x, 0 < x < 1

Question 5.
Even though there are no real principal square roots for negative numbers, principal cube roots do exist for negative numbers: \(\sqrt[3]{-8}\) is the real number -2 since -2 . -2 . -2 = -8. Use the identities \(\sqrt[3]{x^{3}}\) = x and ([\(\sqrt[3]{x^{3}}\))³ = x for any real number x to find the inverse of each of the functions below. In each case, indicate the domain and range of both the original function and its inverse.

a. f(x) = \(\sqrt[3]{2 x}\) for any real number x.
Answer:
y = \(\sqrt[3]{2 x}\)
x = \(\sqrt[3]{2 y}\)
x³ = 2y
2y = x³
y = \(\frac{1}{2}\)(x³)
g(x) = \(\frac{1}{2}\)(x³))
Domain of f and range of g: all real numbers x
Range of f and domain of g: all real numbers x.

b. f(x) = \(\sqrt[3]{2 x-3}\) for any real number x.
Answer:
y = \(\sqrt[3]{2 x-3}\)
x = \(\sqrt[3]{2 y-3}\)
x³ = 2y – 3
2y = x³ + 3
y = \(\frac{1}{2}\)(x³ + 3)
g(x) = \(\frac{1}{2}\)(x³ + 3)
Domain of f and range of g: all real numbers x
Range of f and domain of g: all real numbers x.

c. f(x) = (x – 1)³
Answer:
y = (x – 1)³ + 3
x = (y – 1)³ + 3
x – 3 = (y – 1)³
\(\sqrt[3]{x-3}\) = y – 1
y – 1 = \(\sqrt[3]{x-3}\)
y = \(\sqrt[3]{x-3}\) + 1
g(x) = \(\sqrt[3]{x-3}\) + 1
Domain of f and range of g: all real numbers x
Range of f and domain of g: all real numbers x.

Question 6.
Suppose that the inverse of a function is the function itself. For example, the inverse of the function f(x) = \(\frac{1}{x}\) (for x ≠ 0) is just itself again, g(x) = \(\frac{1}{x}\) (for x ≠ 0). What symmetry must the graphs of all such functions have? (Hint: Study the graph of Exercise 5 In the lesson.)
Answer:
All graphs of functions that are self-inverses are symmetric with respect to the diagonal line given by the equation y = x. That is, a reflection across the line given by y = x takes the graph back to itself.

Question 7.
There are two primary scales for measuring daily temperature: degrees Celsius and degrees Fahrenheit. The United States uses the Fahrenheit scale, and many other countries use the Celsius scale. When traveling abroad you often need to convert between these two temperature scales.

Let f be the function that Inputs a temperature measure in degrees Celsius, denoted by °C, and outputs the corresponding temperature measure in degrees Fahrenheit, denoted by °F.

a. Assuming that f is linear, we can use two points on the graph of f to determine a formula for f. In degrees Celsius, the freezing point of water is 0, and its boiling point is 100. In degrees Fahrenheit, the freezing point of water is 32, and its boiling point is 212. Use this information to find a formula for the function f.
(Hint: Plot the points and draw the graph off first, keeping careful track of the meaning of values on the x-axis and y-axis.)
Answer:
f(t) = \(\frac{9}{5}\)(t + 32)

b. If the temperature in Paris is 25°C, what is the temperature in degrees Fahrenheit?
Answer:
Since f(25) = 77, It is 77°F in Paris.

c. Find the inverse of the function f and explain its meaning in terms of degrees Fahrenheit and degrees Celsius.
Answer:
The inverse of f is g(t) = \(\frac{5}{9}\)(t – 32). Given the measure of a temperature reported in degrees Fahrenheit, the function converts that measure to degrees Celsius.

d. The graphs off and its inverse g are two lines that intersect in one point. What is that point? What is its significance in terms of degrees Celsius and degrees Fahrenheit?
Answer:
The point is (-40, -40). This means that -40°C is the same temperature as -40°F.

Extension:
Use the fact that, for b > 1, the functions f(x) = b^x and g(x) = log_b(x) are increasing to solvethe following problems. Recall that an increasing function f has the property that If both a and b are In the domain of f and a < b, then f(a) < f(b).

Question 8.
For which values of x is 2^x < \(\frac{1}{1,000,000}\)?
Answer:
2^x < \(\frac{1}{1,000,000}\)
x < log₂(\(\frac{1}{1,000,000}\)) = -log₂(1,000,000)

Question 9.
For which values of x is log₂(x) < -1,000,000?
Answer:
log₂(x) < -1,000,000
x < 2^-1,000,000

Eureka Math Algebra 2 Module 3 Lesson 19 Exit Ticket Answer Key

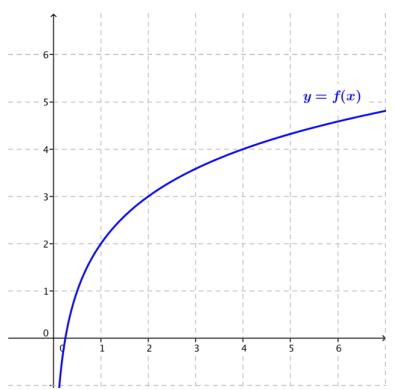
Question 1.
The graph of a function f is shown below. Sketch the graph of its inverse function g on the same axes.

Answer:

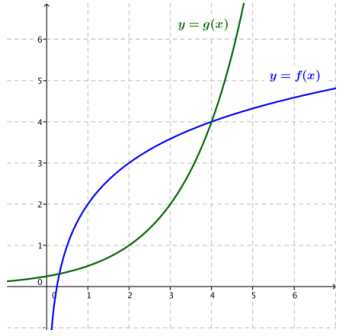
Question 2.
Explain how you made your sketch.
Answer:
Answers will vary. Example: I drew the line given by y = x and reflected the graph off across it.

Question 3.
The graph of the function f above is the function f(x) = log₂((x) + 2 for x > 0. Find a formula for the inverse of this function.
Answer:
y = log₂(x) +2
x = log₂(y) + 2
x – 2 = log₂(y)
log₂(y) = x – 2
y = 2^{x – 2}
g(x) = 2^{x – 2}