Engage NY Eureka Math 4th Grade Module 5 Lesson 25 Answer Key
Eureka Math Grade 4 Module 5 Lesson 25 Problem Set Answer Key
Question 1.
Convert each mixed number to a fraction greater than 1. Draw a number line to model your work.
a. 3\(\frac{1}{4}\)
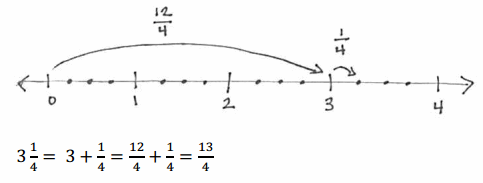
Answer:
3(1/4) = 13/4.
Explanation:
In the above-given question,
given that,
3(1/4).
3 + 1/4.
12/4 + 1/4.
13/4.
b. 2\(\frac{4}{5}\)
Answer:
2(4/5) = 14/5.
Explanation:
In the above-given question,
given that,
2(4/5).
2 + 4/5.
10/5 + 4/5.
14/5.
![]()
c. 3\(\frac{5}{8}\)
Answer:
3(5/8) = 29/8.
Explanation:
In the above-given question,
given that,
3(5/8).
3 + 5/8.
24/8 + 5/8.
29/8.
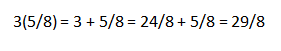
d. 4\(\frac{4}{10}\)
Answer:
4(4/10) = 44/10.
Explanation:
In the above-given question,
given that,
4(4/10).
4 + 4/10.
40/10 + 4/10.
44/10.
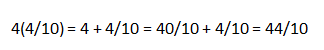
e. 4\(\frac{7}{9}\)
Answer:
4(7/9) = 43/9.
Explanation:
In the above-given question,
given that,
4(7/9).
4 + 7/9.
36/9 + 7/9.
43/9.
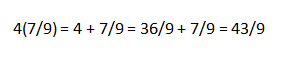
Question 2.
Convert each mixed number to a fraction greater than 1. Show your work as in the example.
(Note: 3 × \(\frac{4}{4}\) = \(\frac{3 \times 4}{4}\))
a. 3\(\frac{3}{4}\)
3\(\frac{3}{4}\) = 3 + \(\frac{3}{4}\) = (3 × \(\frac{3}{4}\)) + \(\frac{3}{4}\) = \(\frac{12}{4}\) + \(\frac{3}{4}\) = \(\frac{15}{4}\)
Answer:
3(3/4) = 15/4.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
3\(\frac{3}{4}\) = 3 + \(\frac{3}{4}\).
(3 × \(\frac{3}{4}\)) + \(\frac{3}{4}\) = \(\frac{12}{4}\) + \(\frac{3}{4}\).
\(\frac{15}{4}\).
b. 4\(\frac{1}{3}\)
Answer:
4(1/3) = 13/3.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
4\(\frac{1}{3}\) = 4 + \(\frac{1}{3}\).
(4 × \(\frac{1}{3}\)) + \(\frac{1}{3}\) = \(\frac{12}{3}\) + \(\frac{1}{3}\).
\(\frac{13}{3}\).
c. 4\(\frac{3}{5}\)
Answer:
4(3/5) = 23/5.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
4\(\frac{3}{5}\) = 4 + \(\frac{3}{5}\).
(4 × \(\frac{3}{5}\)) + \(\frac{3}{5}\) = \(\frac{20}{5}\) + \(\frac{3}{5}\).
\(\frac{23}{5}\).
d. 4\(\frac{6}{8}\)
Answer:
4(6/8) = 38/8.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
4\(\frac{6}{8}\) = 4 + \(\frac{6}{8}\).
(4 × \(\frac{6}{8}\)) + \(\frac{6}{8}\) = \(\frac{32}{8}\) + \(\frac{6}{8}\).
\(\frac{38}{8}\).
Question 3.
Convert each mixed number to a fraction greater than 1.
a. 2\(\frac{3}{4}\)
Answer:
2(3/4) = 11/4.
Explanation:
In the above-given question,
given that,
2(3/4).
2 + 3/4.
8/4 + 3/4.
11/4.
b. 2\(\frac{2}{5}\)
Answer:
2(2/5) = 12/5.
Explanation:
In the above-given question,
given that,
2(2/5).
2 + 2/5.
10/5 + 2/5.
12/5.
c. 3\(\frac{3}{6}\)
Answer:
3(3/6) = 21/6.
Explanation:
In the above-given question,
given that,
3(3/6).
3 + 3/6.
18/6 + 3/6.
21/6.
d. 3\(\frac{3}{8}\)
Answer:
3(3/8) = 27/8.
Explanation:
In the above-given question,
given that,
3(3/8).
3 + 3/8.
24/8 + 3/8.
27/8.
e. 3\(\frac{1}{10}\)
Answer:
3(1/10) = 31/10.
Explanation:
In the above-given question,
given that,
3(1/10).
3 + 1/10.
30/10 + 1/10.
31/10.
f. 4\(\frac{3}{8}\)
Answer:
4(3/8) = 35/8.
Explanation:
In the above-given question,
given that,
4(3/8).
4 + 3/8.
32/8 + 3/8.
35/8.
g. 5\(\frac{2}{3}\)
Answer:
5(2/3) = 17/3.
Explanation:
In the above-given question,
given that,
5(2/3).
5 + 2/3.
15/3 + 2/3.
17/3.
h. 6\(\frac{1}{2}\)
Answer:
6(1/2) = 13/2.
Explanation:
In the above-given question,
given that,
6(1/2).
6 + 1/2.
12/2 + 1/2.
13/2.
i. 7\(\frac{3}{10}\)
Answer:
7(3/10) = 73/10.
Explanation:
In the above-given question,
given that,
7(3/10).
7 + 3/10.
70/10 + 3/10.
73/10.
Eureka Math Grade 4 Module 5 Lesson 25 Exit Ticket Answer Key
Convert each mixed number to a fraction greater than 1.
Question 1.
3\(\frac{1}{5}\)
Answer:
3(1/5) = 16/5.
Explanation:
In the above-given question,
given that,
3(1/5).
3 + 1/5.
15/5 + 1/5.
16/5.
Question 2.
2\(\frac{3}{5}\)
Answer:
2(3/5) = 13/5.
Explanation:
In the above-given question,
given that,
2(3/5).
2 + 3/5.
10/5 + 3/5.
13/5.
Question 3.
4\(\frac{2}{9}\)
Answer:
4(2/9) = 38/9.
Explanation:
In the above-given question,
given that,
4(2/9).
4 + 2/9.
36/9 + 2/9.
38/9.
Eureka Math Grade 4 Module 5 Lesson 25 Homework Answer Key
Question 1.
Convert each mixed number to a fraction greater than 1. Draw a number line to model your work.
a. 3\(\frac{1}{4}\)
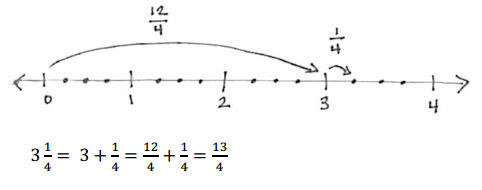
b. 4\(\frac{2}{5}\)
Answer:
4(2/5) = 22/5.
Explanation:
In the above-given question,
given that,
4(2/5).
4 + 2/5.
20/5 + 2/5.
22/5.
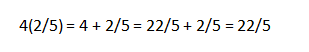
c. 5\(\frac{3}{8}\)
Answer:
5(3/8) = 43/8.
Explanation:
In the above-given question,
given that,
5(3/8).
5 + 3/8.
40/8 + 3/8.
43/8.
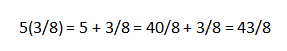
d. 3\(\frac{7}{10}\)
Answer:
3(7/10) = 37/10.
Explanation:
In the above-given question,
given that,
3(7/10).
3 + 7/10.
30/10 + 7/10.
37/10.
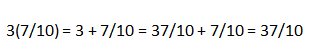
e. 6\(\frac{2}{9}\)
Answer:
6(2/9) = 56/9.
Explanation:
In the above-given question,
given that,
6(2/9).
6 + 2/9.
54/9 + 2/9.
56/9.
![]()
Question 2.
Convert each mixed number to a fraction greater than 1. Show your work as in the example.
(Note: 3 × \(\frac{4}{4}\) = \(\frac{3 \times 4}{4}\).)
a. 3\(\frac{3}{4}\)
3\(\frac{3}{4}\) = 3 + \(\frac{3}{4}\) = (3 × \(\frac{4}{4}\)) + \(\frac{3}{4}\) = \(\frac{12}{4}\) + \(\frac{3}{4}\) = \(\frac{15}{4}\)
b. 5\(\frac{2}{3}\)
Answer:
5(2/3) = 17/3.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
5\(\frac{2}{3}\) = 5 + \(\frac{2}{3}\).
(5 × \(\frac{2}{3}\)) + \(\frac{2}{3}\) = \(\frac{15}{3}\) + \(\frac{2}{3}\).
\(\frac{17}{3}\).
c. 4\(\frac{1}{5}\)
Answer:
4(1/5) = 21/5.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
4\(\frac{1}{5}\) = 4 + \(\frac{1}{5}\).
(4 × \(\frac{1}{5}\)) + \(\frac{1}{5}\) = \(\frac{20}{5}\) + \(\frac{1}{5}\).
\(\frac{21}{5}\).
d. 3\(\frac{7}{8}\)
Answer:
3(7/8) = 31/8.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
3\(\frac{7}{8}\) = 3 + \(\frac{7}{8}\).
(3 × \(\frac{7}{8}\)) + \(\frac{7}{8}\) = \(\frac{24}{8}\) + \(\frac{7}{8}\).
\(\frac{31}{8}\).
Question 3.
Convert each mixed number to a fraction greater than 1.
a. 2\(\frac{1}{3}\)
Answer:
2(1/3) = 7/3.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
2\(\frac{1}{3}\) = 2 + \(\frac{1}{3}\).
(2 × \(\frac{1}{3}\)) + \(\frac{1}{3}\) = \(\frac{6}{3}\) + \(\frac{1}{3}\).
\(\frac{7}{3}\).
b. 2\(\frac{3}{4}\)
Answer:
2(3/4) = 11/4.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
2\(\frac{3}{4}\) = 2 + \(\frac{3}{4}\).
(2 × \(\frac{3}{4}\)) + \(\frac{3}{4}\) = \(\frac{10}{4}\) + \(\frac{3}{4}\).
\(\frac{11}{4}\).
c. 3\(\frac{2}{5}\)
Answer:
3(2/5) = 17/5.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
3\(\frac{2}{5}\) = 3 + \(\frac{2}{5}\).
(3 × \(\frac{2}{5}\)) + \(\frac{2}{5}\) = \(\frac{15}{5}\) + \(\frac{2}{5}\).
\(\frac{17}{5}\).
d. 3\(\frac{1}{6}\)
Answer:
3(1/6) = 19/6.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
3\(\frac{1}{6}\) = 3 + \(\frac{1}{6}\).
(3 × \(\frac{1}{6}\)) + \(\frac{1}{6}\) = \(\frac{18}{6}\) + \(\frac{1}{6}\).
\(\frac{19}{6}\).
e. 4\(\frac{5}{12}\)
Answer:
4(5/12) = 53/12.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
4\(\frac{5}{12}\) = 4 + \(\frac{5}{12}\).
(4 × \(\frac{5}{12}\)) + \(\frac{5}{12}\) = \(\frac{48}{12}\) + \(\frac{5}{12}\).
\(\frac{53}{12}\).
f. 4\(\frac{2}{5}\)
Answer:
4(2/5) = 22/5.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
4\(\frac{2}{5}\) = 4 + \(\frac{2}{5}\).
(4 × \(\frac{2}{5}\)) + \(\frac{2}{5}\) = \(\frac{20}{5}\) + \(\frac{2}{5}\).
\(\frac{22}{5}\).
g. 4\(\frac{1}{10}\)
Answer:
4(1/10) = 41/10.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
4\(\frac{1}{10}\) = 4 + \(\frac{1}{10}\).
(4 × \(\frac{1}{10}\)) + \(\frac{1}{10}\) = \(\frac{40}{10}\) + \(\frac{1}{10}\).
\(\frac{41}{10}\).
h. 5\(\frac{1}{5}\)
Answer:
5(1/5) = 26/5.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
5\(\frac{1}{5}\) = 5 + \(\frac{1}{5}\).
(5 × \(\frac{1}{5}\)) + \(\frac{1}{5}\) = \(\frac{25}{5}\) + \(\frac{1}{5}\).
\(\frac{26}{5}\).
i. 5\(\frac{5}{6}\)
Answer:
5(5/6) = 35/6.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
5\(\frac{5}{6}\) = 5 + \(\frac{5}{6}\).
(5 × \(\frac{5}{6}\)) + \(\frac{5}{6}\) = \(\frac{30}{6}\) + \(\frac{5}{6}\).
\(\frac{35}{6}\).
j. 6\(\frac{1}{4}\)
Answer:
6(1/4) = 25/4.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
6\(\frac{1}{4}\) = 6 + \(\frac{1}{4}\).
(6 × \(\frac{1}{4}\)) + \(\frac{1}{4}\) = \(\frac{24}{4}\) + \(\frac{1}{4}\).
\(\frac{25}{4}\).
k. 7\(\frac{1}{2}\)
Answer:
7(1/2) = 15/2.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
7\(\frac{1}{2}\) = 7 + \(\frac{1}{2}\).
(7 × \(\frac{1}{2}\)) + \(\frac{1}{2}\) = \(\frac{14}{2}\) + \(\frac{1}{2}\).
\(\frac{15}{2}\).
l. 7\(\frac{11}{12}\)
Answer:
7(11/12) = 95/12.
Explanation:
In the above-given question,
given that,
convert each mixed number to a fraction greater than 1.
7\(\frac{11}{12}\) = 7 + \(\frac{11}{12}\).
(7 × \(\frac{11}{12}\)) + \(\frac{11}{12}\) = \(\frac{84}{12}\) + \(\frac{11}{12}\).
\(\frac{95}{12}\).