Engage NY Eureka Math Grade 6 Module 5 Lesson 13 Answer Key
Eureka Math Grade 6 Module 5 Lesson 13 Example Answer Key
Example 1:
Determine the volume of a cube with side lengths of 2\(\frac{1}{4}\) cm.
Answer:
V = l w h
V = (2\(\frac{1}{4}\) cm) (2\(\frac{1}{4}\) cm) (2\(\frac{1}{4}\) cm)
V = \(\frac{9}{4}\) cm × \(\frac{9}{4}\) cm × \(\frac{9}{4}\) cm
V = \(\frac{729}{64}\) cm3
Example 2:
Determine the volume of a rectangular prism with a base area of \(\frac{7}{12}\) ft2 and a height of \(\frac{1}{3}\) ft.
Answer:
V = Area of the base × height
V = (\(\frac{7}{12}\) ft2) (\(\frac{1}{3}\) ft.)
V = \(\frac{7}{36}\) ft3
Eureka Math Grade 6 Module 5 Lesson 13 Exercise Answer Key
Exercise 1.
Use the rectangular prism to answer the next set of questions.
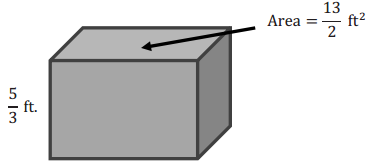
a. Determine the volume of the prism.
Answer:
V = Area of the base × height
V = (\(\frac{13}{2}\) ft2) (\(\frac{5}{3}\) ft.)
V = \(\frac{65}{6}\) ft3
b. Determine the volume of the prism if the height of the prism is doubled.
Answer:
Height × 2 = (\(\frac{5}{3}\) ft. × 2) = \(\frac{10}{3}\) ft.
V = Area of base × height
V=(\(\frac{13}{2}\) ft2)(\(\frac{10}{3}\) ft.)
V = \(\frac{130}{6}\) ft3 or 21\(\frac{2}{3}\) ft3
c. Compare the volume of the rectangular prism in part (a) with the volume of the prism in part (b). What do you notice?
Answer:
When the height of the rectangular prism is doubled, the volume is also doubled.
d. Complete and use the table below to determine the relationship between the height and volume.
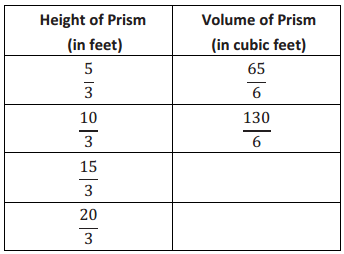
Answer:
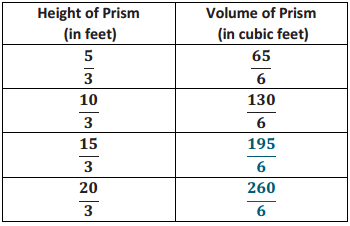
What happened to the volume when the height was tripled?
Answer:
The volume tripled.
What happened to the volume when the height was quadrupled?
Answer:
The volume quadrupled.
What conclusions can you make when the base area stays constant and only the height changes?
Answer:
Answers will vary but should include the idea of a proportional relationship. Each time the height is multiplied by a number, the original volume is multiplied by the same amount.
Exercise 2.
a. If B represents the area of the base and h represents the height, write an expression that represents the volume.
Answer:
Bh
b. If we double the height, write an expression for the new height.
Answer:
2h
c. Write an expression that represents the volume with the doubled height.
Answer:
B2h
d. Write an equivalent expression using the commutative and associative properties to show the volume Is
twice the original volume.
Answer:
2(Bh)
Exercise 3.
Use the cube to answer the following questions.
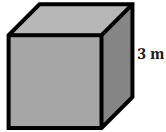
a. Determine the volume of the cube.
Answer:
V= l w h
V = (3m) (3m) (3m)
V = 27 m3
b. Determine the volume of a cube whose side lengths are half as long as the side lengths of the original cube.
Answer:
V = l w h
V = (\(\frac{3}{2}\) m) (\(\frac{3}{2}\) m) (\(\frac{3}{2}\) m)
V = \(\frac{27}{8}\) m3
c. Determine the volume if the side lengths are one-fourth as long as the original cube’s side lengths.
Answer:
V = l w h
V = (\(\frac{3}{4}\) m) (\(\frac{3}{4}\) m) (\(\frac{3}{4}\) m)
V = \(\frac{27}{64}\) m3
d. Determine the volume if the side lengths are one-sixth as long as the original cube’s side lengths.
Answer:
V = lwh
V = (\(\frac{3}{6}\) m) (\(\frac{3}{6}\) m) (\(\frac{3}{6}\) m)
V = \(\frac{27}{216}\)m3
OR
V = \(\frac{1}{8}\) m3
e. Explain the relationship between the side lengths and the volumes of the cubes.
Answer:
If each of the sides are changed by the same fractional amount, \(\frac{1}{a^{\prime}}\) of the original, then the volume of the new figure will be \(\left(\frac{1}{a}\right)^{3}\) of the original volume. For example, if the sides are \(\frac{1}{2}\) as long, then the volume will be \(\left(\frac{1}{2}\right)^{3}\) = \(\frac{1}{8}\) as much.
Exercise 4.
Check to see if the relationship you found in Exercise 3 is the same for rectangular prisms.
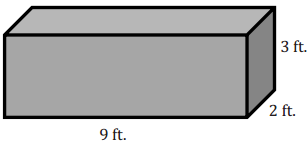
a. Determine the volume of the rectangular prism.
Answer:
V = l w h
V = (9 ft.) (2 ft.) (3 ft.)
V = 54 ft3
b. Determine the volume if all of the sides are half as long as the original lengths.
Answer:
V = l w h
V = (\(\frac{9}{2}\) ft.) (\(\frac{2}{2}\) ft.) (\(\frac{3}{2}\) ft.)
V = \(\frac{9}{2}\) ft3
OR
V = \(\frac{27}{4}\) ft3
c. Determine the volume if all of the sides are one-third as long as the original lengths.
Answer:
V = l w h
V = (\(\frac{9}{3}\) ft.) (\(\frac{2}{3}\) ft.) (\(\frac{3}{3}\) ft.)
V = \(\frac{54}{27}\) ft3
OR
V = 2 ft3
d. Is the relationship between the side lengths and the volume the same as the one that occurred in Exercise 3? Explain your answer.
Answer:
Yes, the relationship that was found in the problem with the cubes still holds true with this rectangular prism. When I found the volume of o prism with side lengths that were one-third the original, the volume was
(\(\frac{1}{3}\))3 = \(\frac{1}{27}\) the original.
Exercise 5.
a. If e represents a side length of the cube, create an expression that shows the volume of the cube.
Answer:
e3
b. If we divide the side lengths by three, create an expression for the new side length.
Answer:
\(\frac{1}{3}\) e or \(\frac{\mathrm{e}}{3}\)
c. Write an expression that represents the volume of the cube with one-third the side length.
Answer:
(\(\frac{1}{3}\) e)3 or (\(\frac{\mathrm{e}}{3}\))3
d. Write an equivalent expression to show that the volume is of the original volume.
Answer:
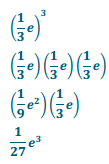
Eureka Math Grade 6 Module 5 Lesson 13 Problem Set Answer Key
Question 1.
Determine the volume of the rectangular prism.
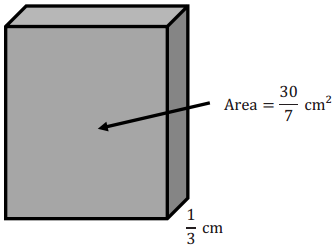
Answer:
V = Area of the base × height
V = (\(\frac{30}{7}\) cm2) (\(\frac{1}{3}\) cm)
V = \(\frac{30}{21}\) cm3
OR
V = \(\frac{10}{7}\) cm3
Question 2.
Determine the volume of the rectangular prism in Problem 1 if the height is quadrupled (multiplied by four). Then, determine the relationship between the volumes in Problem 1 and this prism.
Answer:
V = Area of base × height
V = (\(\frac{30}{7}\) cm2) (\(\frac{4}{3}\) cm)
V = \(\frac{120}{21}\) cm3
OR
V = \(\frac{40}{7}\) cm3
When the height was quadrupled, the volume was also quadrupled.
Question 3.
The area of the base of a rectangular prism can be represented by B, and the height is represented by h.
a. Write an equation that represents the volume of the prism.
Answer:
V = Bh
b. If the area of the base is doubled, write an equation that represents the volume of the prism.
Answer:
V = 2Bh
c. If the height of the prism is doubled, write an equation that represents the volume of the prism.
Answer:
V = B2h = 2Bh
d. Compare the volume in parts (b) and (c). What do you notice about the volumes?
Answer:
The expressions in part (b) and part (c) are equal to each other.
e. Write an expression for the volume of the prism if both the height and the area of the base are doubled.
Answer:
V = 2B2h = 4Bh
Question 4.
Determine the volume of a cube with a side length of 5 In.
Answer:
V = l w h
V = (5\(\frac{1}{3}\) in.) (5\(\frac{1}{3}\) in.) (5\(\frac{1}{3}\) in.)
V = \(\frac{16}{3}\) in. × \(\frac{16}{3}\) in. × \(\frac{16}{3}\) in.
V = \(\frac{4,096}{27}\) in3.
Question 5.
Use the information in Problem 4 to answer the following:
a. Determine the volume of the cube in Problem 4 if all of the side lengths are cut in half.
Answer:
V = l w h
V = (2\(\frac{2}{3}\) in.)(2\(\frac{2}{3}\) in.)(2\(\frac{2}{3}\) in.)
V = \(\frac{8}{3}\) in. × \(\frac{8}{3}\) in. × \(\frac{8}{3}\) in.
V = \(\frac{512}{27}\) in3
b. How could you determine the volume of the cube with the side lengths cut in half using the volume in Problem 4?
Answer:
Because each side is hallas long, I know that the volume is \(\frac{1}{8}\) the volume of the cube in Problem 4. This is because the length, the width, and the height were all cut in half.
\(\frac{1}{2}\)l × \(\frac{1}{2}\)w × \(\frac{1}{2}\)h = \(\frac{1}{8}\)lwh
\(\frac{1}{8}\) × \(\frac{4,096}{27}\) in3 = \(\frac{512}{27}\) in3
Question 6.
Use the rectangular prism to answer the following questions.
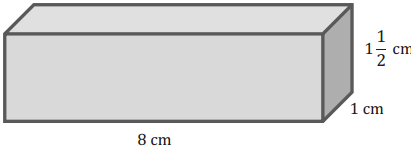
a. Complete the table.
Answer:
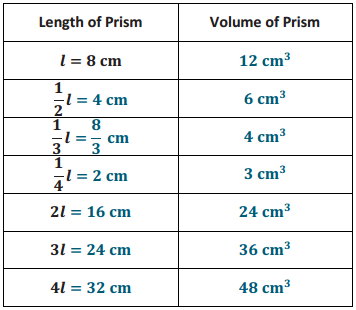
b. How did the volume change when the length was one-third as long?
Answer:
415 one-third of 12. Therefore, when the length is one-third as long, the volume is also one-third as much.
c. How did the volume change when the length was tripled?
Answer:
36 is three times as much as 12. Therefore, when the length is three times as long, the volume is also three times as much.
d. What conclusion can you make about the relationship between the volume and the length?
Answer:
When the length changes but the width and height stay the same, the change in the volume is proportional to the change in the length.
Question 7.
The sum of the volumes of two rectangular prisms, Box A and Box B, are 14.325 cm3. Box A has a volume of 5.61 cm3.
a. Let B represent the volume of Box B in cubic centimeters. Write an equation that could be used to determine the volume of Box B.
Answer:
14.325 cm3 = 5.61 cm3 + B
b. Solve the equation to determine the volume of Box B.
Answer:
B = 8.715 cm3
c. If the area of the base of Box B is 1.5 cm2, write an equation that could he used to determine the height of
Box B. Let h represent the height of Box B in centimeters.
Answer:
8.715 cm3 = (1.5 cm2)h
d. Solve the equation to determine the height of Box B.
Answer:
h = 5.81cm
Eureka Math Grade 6 Module 5 Lesson 13 Exit Ticket Answer Key
Question 1.
A new company wants to mail out samples of its hair products. The company has a sample box that is a rectangular prism with a rectangular base with an area of 23 -in. The height of the prism is 1in. Determine the volume of the sample box.
Answer:
V = Area of base × height
V = (23\(\frac{1}{3}\) in2) (1\(\frac{1}{4}\) in)
V = \(\frac{70}{3}\) in2 × \(\frac{5}{4}\) in.
V = \(\frac{350}{12}\) in3
OR
V = \(\frac{175}{6}\) in2
Question 2.
A different sample box has a height that is twice as long as the original box described in Problem 1. What is the volume of this sample box? How does the volume of this sample box compare to the volume of the sample box in Problem 1?
Answer:
V = Area of base × height
V = (23\(\frac{1}{3}\) in2)(2\(\frac{1}{2}\) in.)
V = (\(\frac{70}{3}\) in2) (\(\frac{5}{2}\) in.)
V = \(\frac{350}{6}\) in3
OR
V = \(\frac{175}{3}\) in3
By doubling the height, we have also doubled the volume.
Eureka Math Grade 6 Module 5 Lesson 13 Multiplication and Division Equations with Fractions Answer Key
Multiplication and Division Equations with Fractions:
Progression of Exercises:
Question 1.
5y = 35
Answer:
y=7
Question 2.
3m = 135
Answer:
m = 45
Question 3.
12k = 156
Answer:
k = 13
Question 4.
\(\frac{f}{3}\) = 24
Answer:
f = 72
Question 5.
\(\frac{x}{7}\) = 42
Answer:
x = 294
Question 6.
\(\frac{c}{13}\) = 18
Answer:
c = 234
Question 7.
\(\frac{2}{3}\) g = 6
Answer:
g = 9
Question 8.
\(\frac{3}{5}\) k = 9
Answer:
k = 15
Question 9.
\(\frac{3}{4}\) y = 10
Answer:
y = \(\frac{40}{3}\) = 13 \(\frac{1}{3}\)
Question 10.
\(\frac{5}{8}\) j = 9
Answer:
j = \(\frac{72}{5}\) = 14 \(\frac{2}{5}\)
Question 11.
\(\frac{3}{7}\) h = 13
Answer:
h = \(\frac{91}{3}\) = 30\(\frac{1}{3}\)
Question 12.
\(\frac{m}{4}\) = \(\frac{3}{5}\)
Answer:
m = \(\frac{12}{5}\) = 2 \(\frac{2}{5}\)
Question 13.
\(\frac{f}{3}\) = \(\frac{2}{7}\)
Answer:
f = \(\frac{6}{7}\)
Question 14.
\(\frac{2}{5}\) p = \(\frac{3}{7}\)
Answer:
p = \(\frac{15}{14}\) = 1\(\frac{1}{14}\)
Question 15.
\(\frac{3}{4}\) k = \(\frac{5}{8}\)
Answer:
k = \(\frac{20}{24}\) = \(\frac{5}{6}\)