Engage NY Eureka Math 7th Grade Module 2 Lesson 1 Answer Key
Eureka Math Grade 7 Module 2 Lesson 1 Example Answer Key
Example 2.
Counting Up and Counting Down on the Number Line
Use the number line below to practice counting up and counting down.
→ Counting up starting at 0 corresponds to positive ___ numbers.
Answer:
positive
→ Counting down starting at 0 corresponds to ___ numbers.
negative
Answer:
a. Where do you begin when locating a number on the number line?
Answer:
Start at 0.
b. What do you call the distance between a number and 0 on a number line?
Answer:
The absolute value
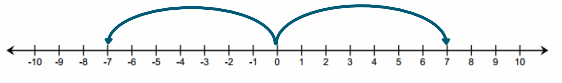
c. What is the relationship between 7 and -7?
Answer:
Answers will vary. 7 and -7 both have the same absolute values. They are both the same distance from zero, 0, but in opposite directions; therefore, 7 and -7 are opposites.
Example 3:
Using the Integer Game and the Number Line
What is the sum of the card values shown? Use the counting on method on the provided number line to justify your answer.
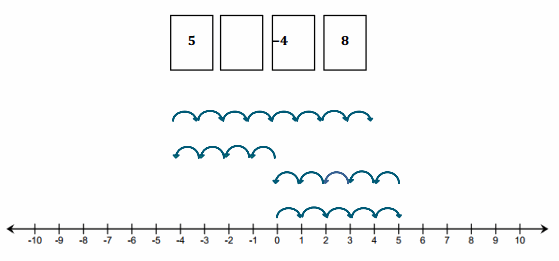
a. What is the final position on the number line? ___
Answer:
4
b. What card or combination of cards would you need to get back to 0? ___
Answer:
-4 or -1 and -3
Eureka Math Grade 7 Module 2 Lesson 1 Exercise Answer Key
Exercise 1.
Positive and Negative Numbers Review
With your partner, use the graphic organizer below to record what you know about positive and negative numbers. Add or remove statements during the whole-class discussion.

Exercise 2.
The Additive Inverse
Use the number line to answer each of the following questions.
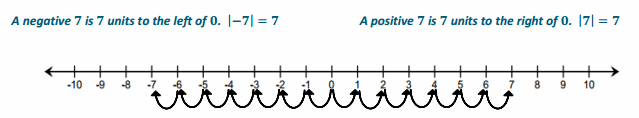
a. How far is 7 from 0 and in which direction? ___
Answer:
7 units to the right
b. What is the opposite of 7? -7
Answer:
-7
c. How far is -7 from 0 and in which direction?
Answer:
7 units to the left
d. Thinking back to our previous work, explain how you would use the counting on method to represent the following: While playing the Integer Game, the first card selected is 7, and the second card selected is -7.
Answer:
I would start at 0 and count up 7 by moving to the right. Then, I would start counting back down from 7 to 0.
e. What does this tell us about the sum of 7 and its opposite, -7?
Answer:
The sum of 7 and -7 equals 0.
7 + (-7) = 0
f. Look at the curved arrows you drew for 7 and -7. What relationship exists between these two arrows that would support your claim about the sum of 7 and -7?
Answer:
The arrows are both the same distance from 0. They are just pointing in opposite directions.
g. Do you think this will hold true for the sum of any number and its opposite? Why?
Answer:
I think this will be true for the sum of any number and its opposite because when you start at 0 on the number line and move in one direction, moving in the opposite direction the same number of times will always take you back to zero.
Eureka Math Grade 7 Module 2 Lesson 1 Exit Ticket Answer Key
Question 1.
Your hand starts with the 7 card. Find three different pairs that would complete your hand and result in a value of zero.
Answer:
Answers will vary. (-3 and -4), (-5 and -2), (-10 and 3)
Question 2.
Write an equation to model the sum of the situation below.
A hydrogen atom has a zero charge because it has one negatively charged electron and one positively charged proton.
Answer:
(-1) + 1 = 0 or 1 + (-1) = 0
Question 3.
Write an equation for each diagram below. How are these equations alike? How are they different? What is it about the diagrams that lead to these similarities and differences?
Diagram A:
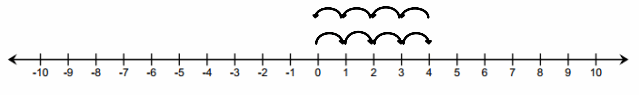
Diagram B:
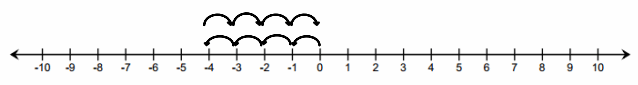
Answer:
A: 4 + (-4)=0
B: -4 + 4 = 0
Answers will vary. Both equations are adding 4 and -4. The order of the numbers is different. The direction of A shows counting up 4 and then counting down 4. The direction of B shows counting down 4 and then counting up 4.
Students may also mention that both diagrams demonstrate a sum of zero, adding opposites, or adding additive inverses.
Eureka Math Grade 7 Module 2 Lesson 1 Problem Set Answer Key
The Problem Set provides practice with real-world situations involving the additive inverse such as temperature and money. Students also explore more scenarios from the Integer Game to provide a solid foundation for Lesson 2.
For Problems 1 and 2, refer to the Integer Game.
Question 1.
You have two cards with a sum of (-12) in your hand.
a. What two cards could you have?
Answer:
Answers will vary. (-6 and -6)
b. You add two more cards to your hand, but the total sum of the cards remains the same, (-12). Give some different examples of two cards you could choose.
Answer:
Answers will vary, but numbers must be opposites. (-2 and 2) and (4 and -4)
Question 2.
Choose one card value and its additive inverse. Choose from the list below to write a real-world story problem that would model their sum.
a. Elevation: above and below sea level
Answer:
Answers will vary. (A scuba diver is 20 feet below sea level. He had to rise 20 feet in order to get back on the boat.)
b. Money: credits and debits, deposits and withdrawals
Answer:
Answers will vary. (The bank charges a fee of $5 for replacing a lost debit card. If you make a deposit of $5, what would be the sum of the fee and the deposit?)
c. Temperature: above and below 0 degrees
Answer:
Answers will vary. (The temperature of one room is 5 degrees above 0. The temperature of another room is 5 degrees below zero. What is the sum of both temperatures?)
d. Football: loss and gain of yards
Answer:
Answers will vary. (A football player gained 25 yards on the first play. On the second play, he lost 25 yards. What is his net yardage after both plays?)
Question 3.
On the number line below, the numbers h and k are the same distance from 0. Write an equation to express the value of h + k. Explain.
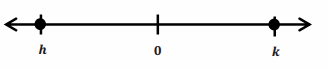
Answer:
h + k = 0 because their absolute values are equal, but their directions are opposite. k is the additive inverse of h, and h is the additive inverse of k because they are the same distance from 0. Therefore, the sum of k and h is 0, because additive inverses have a sum of 0.
Question 4.
During a football game, Kevin gained five yards on the first play. Then he lost seven yards on the second play. How many yards does Kevin need on the next play to get the team back to where they were when they started? Show your work.
Answer:
He has to gain 2 yards.
5 + (-7) + 2 = 0, 5 + (-7) = -2, and -2 + 2 = 0.
QueWrite 5.
Write an addition number sentence that corresponds to the arrows below.
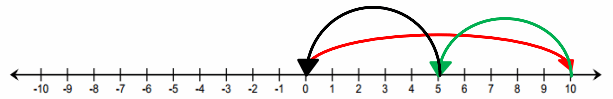
Answer:
10 + (-5) + (-5) = 0