Engage NY Eureka Math 7th Grade Module 3 Lesson 10 Answer Key
Eureka Math Grade 7 Module 3 Lesson 10 Example Answer Key
Example 1.
Estimate the measurement of x. ___
In a complete sentence, describe the angle relationship in the diagram.
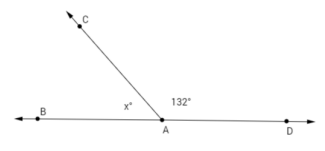
Answer:
∠BAC and ∠CAD are angles on a line and their measures have a sum of 180°.
Write an equation for the angle relationship shown in the figure and solve for x. Then, find the measures of ∠BAC and confirm your answers by measuring the angle with a protractor.
x + 132 = 180
x + 132 – 132 = 180 – 132
x = 48
m∠BAC = 48°
Example 2.
In a complete sentence, describe the angle relationship in the diagram.
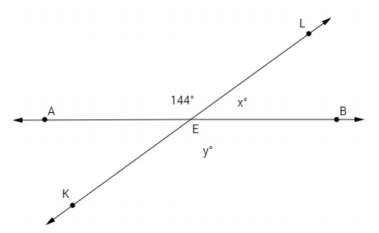
Answer:
∠AEL and ∠LEB are angles on a line and their measures have a sum of 180°. ∠AEL and ∠KEB are vertical angles and are of equal measurement.
Write an equation for the angle relationship shown in the figure and solve for x and y. Find the measurements of ∠LEB and ∠KEB.
Answer:
y = 144°; m∠KEB = 144° (or vert. ∠s are = )
x + 144 = 180
x + 144 – 144 = 180 – 144
x = 36
m∠LEB = 36°
Example 3.
In a complete sentence, describe the angle relationships in the diagram.
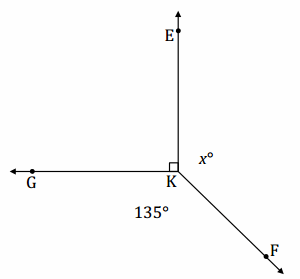
Answer:
∠GKE, ∠EKF, and ∠GKF are angles at a point and their measures have a sum of 360°.
Write an equation for the angle relationship shown in the figure and solve for x. Find the measurement of ∠EKF and confirm your answers by measuring the angle with a protractor.
Answer:
x + 90 + 135 = 360
x + 225 = 360
x + 225 – 225 = 360 – 225
x = 135
m∠EKF = 135°
Example 4.
The following two lines intersect. The ratio of the measurements of the obtuse angle to the acute angle in any adjacent angle pair in this figure is 2:1. In a complete sentence, describe the angle relationships in the diagram.
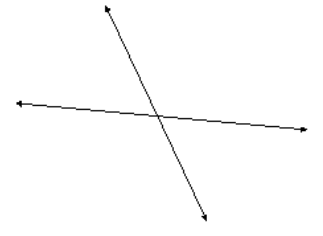
Answer:
The measurement of an obtuse angle is twice the measurement of an acute angle in the diagram.
Label the diagram with expressions that describe this relationship. Write an equation that models the angle relationship and solve for x. Find the measurements of the acute and obtuse angles.
Answer:
2x + 1x = 180
3x = 180
(\(\frac{1}{3}\))(3x) = (\(\frac{1}{3}\))(180)
x = 60
Acute angle = 60°
Obtuse angle = 2x° = 2(60°) = 120°
Eureka Math Grade 7 Module 3 Lesson 10 Opening Exercise Answer Key
Use the diagram to complete the chart.
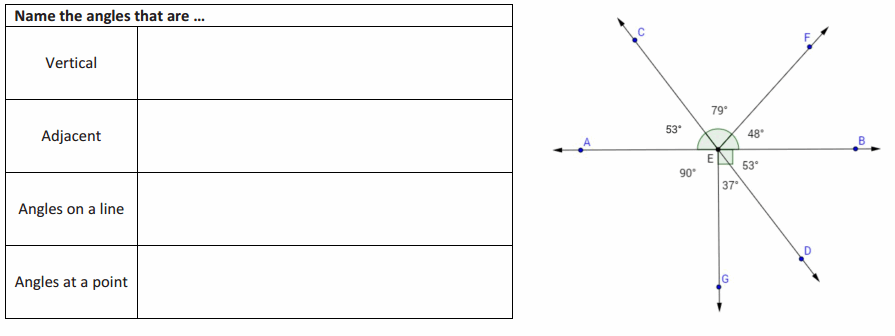
Answer:
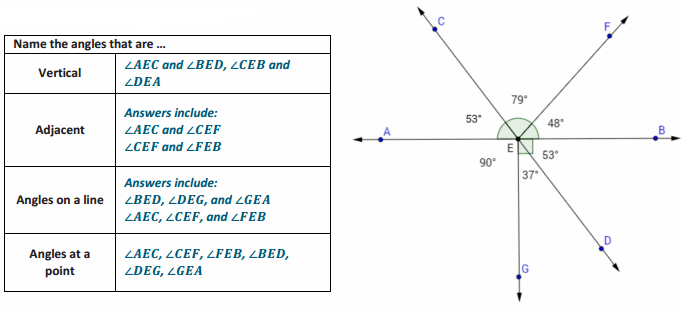
Eureka Math Grade 7 Module 3 Lesson 10 Exercise Answer Key
Exercise 1.
In a complete sentence, describe the angle relationship in the diagram.
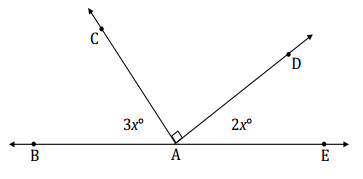
Answer:
∠BAC, ∠CAD, and ∠DAE are angles on a line and their measures have a sum of 180°.
Find the measurements of ∠BAC and ∠DAE.
Answer:
3x + 90 + 2x = 180
5x + 90 = 180
5x + 90 – 90 = 180 – 90
(\(\frac{1}{5}\))(5x) = (\(\frac{1}{5}\))(90)
x = 18
m∠BAC = 3(18°) = 54°
m∠DAE = 2(18°) = 36°
Exercise 2.
In a complete sentence, describe the angle relationships in the diagram.
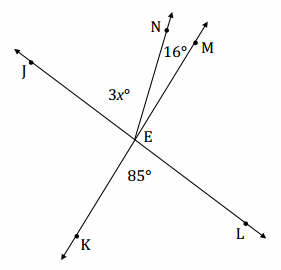
Answer:
∠JEN and ∠NEM are adjacent angles and, when added together, are the measure of ∠JEM; ∠JEM and ∠KEL are vertical angles and are of equal measurement.
Write an equation for the angle relationship shown in the figure and solve for x.
Answer:
3x + 16 = 85
3x + 16 – 16 = 85 – 16
3x = 69
(\(\frac{1}{3}\))3x = 69(\(\frac{1}{3}\))
x = 23
Exercise 3.
In a complete sentence, describe the angle relationships in the diagram.
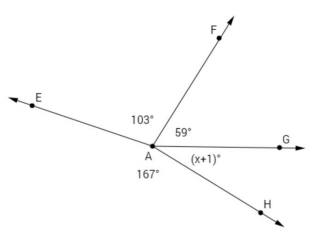
Answer:
∠EAH, ∠GAH, ∠GAF, and ∠FAE are angles at a point and their measures sum to 360°.
Find the measurement of ∠GAH.
Answer:
(x + 1) + 59 + 103 + 167 = 360
x + 1 + 59 + 103 + 167 = 360
x = 30
m∠GAH = (30 + 1)° = 31°
Exercise 4.
The ratio of m∠GFH to m∠EFH is 2∶3. In a complete sentence, describe the angle relationships in the diagram.
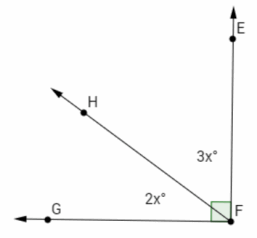
Answer:
The measurement of ∠GFH is \(\frac{2}{3}\) the measurement of ∠EFH; The measurements of ∠GFH and ∠EFH have a sum of 90°.
Find the measures of ∠GFH and ∠EFH.
Answer:
2x + 3x = 90
5x = 90
(\(\frac{1}{5}\))(5x) = (\(\frac{1}{5}\))(90)
x = 18
m∠GFH = 2(18°) = 36°
m∠EFH = 3(18°) = 54°
Eureka Math Grade 7 Module 3 Lesson 10 Problem Set Answer Key
For each question, use angle relationships to write an equation in order to solve for each variable. Determine the indicated angles. You can check your answers by measuring each angle with a protractor.
Question 1.
In a complete sentence, describe the relevant angle relationships in the following diagram. Find the measurement of ∠DAE.
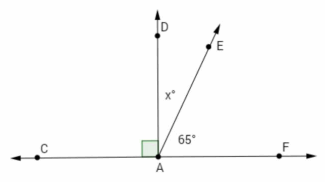
Answer:
One possible response: ∠CAD, ∠DAE, and ∠FAE are angles on a line and their measures sum to 180°.
90 + x + 65 = 180
x + 155 = 180
x + 155 – 155 = 180 – 155
x = 25
m∠DAE = 25°
Question 2.
In a complete sentence, describe the relevant angle relationships in the following diagram. Find the measurement of ∠QPR.
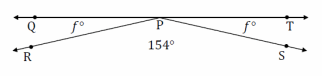
Answer:
∠QPR, ∠RPS, and ∠SPT are angles on a line and their measures sum to 180°.
f + 154 + f = 180
2f + 154 = 180
2f + 154 – 154 = 180 – 154
2f = 26
(\(\frac{1}{2}\))2f = (\(\frac{1}{2}\))26
f = 13
m∠QPR = 13°
Question 3.
In a complete sentence, describe the relevant angle relationships in the following diagram. Find the measurements of ∠CQD and ∠EQF.
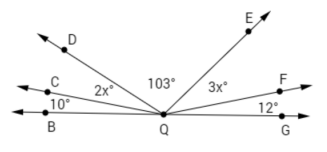
Answer:
∠BQC, ∠CQD, ∠DQE, ∠EQF, and ∠FQG are angles on a line and their measures sum to 180°.
10 + 2x + 103 + 3x + 12 = 180
5x + 125 = 180
5x + 125 – 125 = 180 – 125
5x = 55
(\(\frac{1}{5}\))5x = (\(\frac{1}{5}\))55
x = 11
m∠CQD = 2(11°) = 22°
m∠EQF = 3(11°) = 33°
Question 4.
In a complete sentence, describe the relevant angle relationships in the following diagram. Find the measure of x.
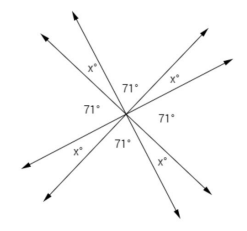
Answer:
All of the angles in the diagram are angles at a point and their measures sum to 360°.
4(x + 71) = 360
4x + 284 = 360
4x + 284 – 284 = 360 – 284
4x = 76
(\(\frac{1}{4}\))4x = (\(\frac{1}{4}\))76
x = 19
Question 5.
In a complete sentence, describe the relevant angle relationships in the following diagram. Find the measures of x and y.
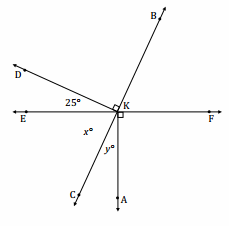
Answer:
∠CKE, ∠EKD, and ∠DKB are angles on a line and their measures sum to 180°. Since ∠FKA and ∠AKE form a straight angle and the measurement of ∠FKA is 90°, ∠AKE is 90°, making ∠CKE and ∠AKC form a right angle and their measures have a sum of 90°.
x + 25 + 90 = 180
x + 115 = 180
x + 115 – 115 = 180 – 115
x = 65
(65) + y = 90
65 – 65 + y = 90 – 65
y = 25
Question 6.
In a complete sentence, describe the relevant angle relationships in the following diagram. Find the measures of x and y.
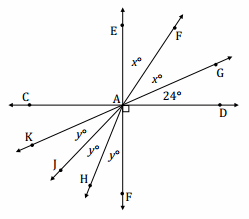
Answer:
∠EAG and ∠FAK are vertical angles and are of equal measurement. ∠EAG and ∠GAD form a right angle and their measures have a sum of 90°.
2x + 24 = 90
2x + 24 – 24 = 90 – 24
2x = 66
(\(\frac{1}{2}\))2x = (\(\frac{1}{2}\))66
x = 33
3y = 66
(\(\frac{1}{3}\))3y = (\(\frac{1}{3}\))66
y = 22
Question 7.
In a complete sentence, describe the relevant angle relationships in the following diagram. Find the measures of ∠CAD and ∠DAE.
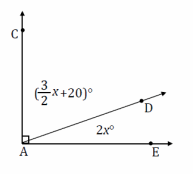
Answer:
∠CAD and ∠DAE form a right angle and their measures have a sum of 90°.
(\(\frac{3}{2}\) x + 20) + 2x = 90
\(\frac{7}{2}\) x + 20 = 90
\(\frac{7}{2}\) x + 20 – 20 = 90 – 20
\(\frac{7}{2}\) x = 70
(\(\frac{2}{7}\)) \(\frac{7}{2}\) x = 70(\(\frac{2}{7}\))
x = 20
m∠CAD = \(\frac{3}{2}\) (20°) + 20° = 50°
m∠DAE = 2(20°) = 40°
Question 8.
In a complete sentence, describe the relevant angle relationships in the following diagram. Find the measure of ∠CQG.
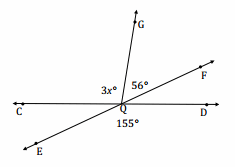
Answer:
∠DQE and ∠CQF are vertical angles and are of equal measurement. ∠CQG and ∠GQF are adjacent angles and their measures sum to the measure of ∠CQF.
3x + 56 = 155
3x + 56 – 56 = 155 – 56
3x = 99
(\(\frac{1}{3}\))3x = (\(\frac{1}{3}\))99
x = 33
m∠CQG = 3(33°) = 99°
Question 9.
The ratio of the measures of a pair of adjacent angles on a line is 4:5.
a. Find the measures of the two angles.
Answer:
∠1 = 4x, ∠2 = 5x
4x + 5x = 180
9x = 180
(\(\frac{1}{9}\))9x = (\(\frac{1}{9}\))180
x = 20
∠1 = 4(20°) = 80°
∠2 = 5(20°) = 100°
b. Draw a diagram to scale of these adjacent angles. Indicate the measurements of each angle.
Answer:
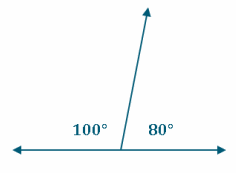
Question 10.
The ratio of the measures of three adjacent angles on a line is 3:4:5.
a. Find the measures of the three angles.
Answer:
∠1 = 3x, ∠2 = 4x, ∠3 = 5x
3x + 4x + 5x = 180
12x = 180
(\(\frac{1}{12}\))12x = (\(\frac{1}{12}\))180
x = 15
∠1 = 3(15°) = 45°
∠2 = 4(15°) = 60°
∠3 = 5(15°) = 75°
b. Draw a diagram to scale of these adjacent angles. Indicate the measurements of each angle.
Answer:
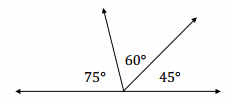
Eureka Math Grade 7 Module 3 Lesson 10 Exit Ticket Answer Key
In a complete sentence, describe the relevant angle relationships in the following diagram. That is, describe the angle relationships you could use to determine the value of x.
Answer:
∠KAE and ∠EAF are adjacent angles whose measurements are equal to ∠KAF; ∠KAF and ∠JAG are vertical angles and are of equal measurement.
Use the angle relationships described above to write an equation to solve for x. Then, determine the measurements of ∠JAH and ∠HAG.
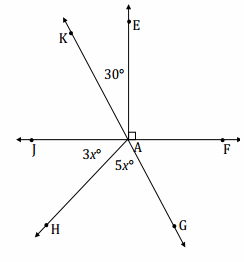
Answer:
5x + 3x = 90 + 30
8x = 120
(\(\frac{1}{8}\))(8x) = (\(\frac{1}{8}\))(120)
x = 15
m∠JAH = 3(15°) = 45°
m∠HAG = 5(15°) = 75°