Engage NY Eureka Math 8th Grade Module 2 Lesson 16 Answer Key
Engage NY Math 8th Grade Module 2 Lesson 16 Example Answer Key
Example 1.
Given a right triangle with a hypotenuse with length 13 units and a leg with length 5 units, as shown, determine the length of the other leg.
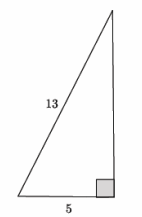
Answer:
52 +b2 =132
52 – 52 + b2 =132 -52
b2 =132 -52
b2 =169-25
b2 =144
b=12
The length of the leg is 12 units.
→ Let b represent the missing leg of the right triangle; then, by the Pythagorean theorem:
52 +b2 =132.
→ If we let a represent the missing leg of the right triangle, then by the Pythagorean theorem:
a2 +52 =132.
→ Which of these two equations is correct: 52 +b2 =132 or a2 +52 =132 ?
→ It does not matter which equation we use as long as we are showing the sum of the squares of the legs as equal to the square of the hypotenuse.
→ Using the first of our two equations, 52 +b2 =132, what can we do to solve for b in the equation?
→ We need to subtract 52 from both sides of the equation.
52 + b2 =132
52 – 52 + b2 =132 -52
b2 =132 -52
→ Point out to students that we are looking at the Pythagorean theorem in a form that allows us to find the length of one of the legs of the right triangle. That is, b2 =c2 -a2 .
→ The length of the leg of the right triangle is 12 units.
Example 2.
The Pythagorean theorem as it applies to missing side lengths of triangles in a real-world problem:
→ Suppose you have a ladder of length 13 feet. Suppose that to make it sturdy enough to climb, you must place the ladder exactly 5 feet from the wall of a building. You need to post a banner on the building 10 feet above the ground. Is the ladder long enough for you to reach the location you need to post the banner?
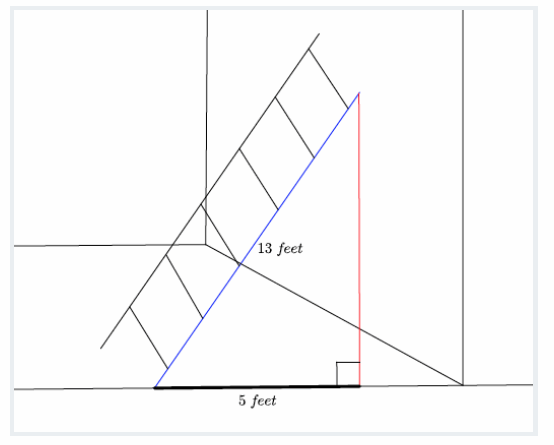
The ladder against the wall forms a right angle. For that reason, we can use the Pythagorean theorem to find out how far up the wall the ladder will reach. If we let h represent the height the ladder can reach, what equation will represent this problem?
→ 52 +h2 =132 or h2 =132 -52
→ Using either equation, we see that this is just like Example 1. We know that the missing side of the triangle is 12 feet. Is the ladder long enough for you to reach the 10-foot banner location?
→ Yes, the ladder allows us to reach 12 feet up the wall.
Example 3.
Pythagorean theorem as it applies to missing side lengths of a right triangle:
→ Given a right triangle with a hypotenuse of length 15 units and a leg of length 9, what is the length of the other leg?
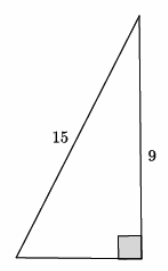
→ If we let the length of the missing leg be represented by a, what equation will allow us to determine its value?
→ a2 +92 =152 or a2 =152 -92
→ Finish the computation:
a2 =225-81
a2 =144
a=12
→ The length of the missing leg of this triangle is 12 units.
Eureka Math Grade 8 Module 2 Lesson 16 Exercise Answer Key
Exercises 1–2
Exercise 1.
Use the Pythagorean theorem to find the missing length of the leg in the right triangle.
Answer:
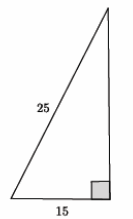
Let b represent the missing leg length; then,
152 +b2 =252
152 -152 +b2 =252 -152
b2 =625-225
b2 =400
b=20
The length of the leg is 20 units.
Exercise 2.
You have a 15-foot ladder and need to reach exactly 9 feet up the wall. How far away from the wall should you place the ladder so that you can reach your desired location?
Answer:
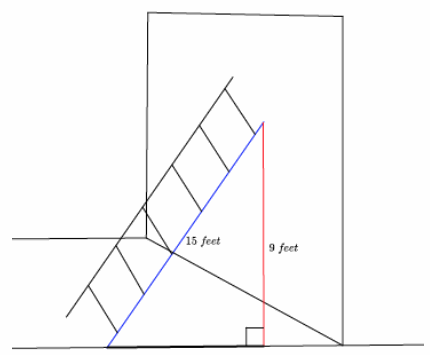
Let a represent the distance the ladder must be placed from the wall; then,
a2 +92 =152
a2 +92 -92 =152 -92
a2 =225-81
a2 =144
a=12.
The ladder must be placed exactly 12 feet from the wall.
Exercises 3–6
Exercise 3.
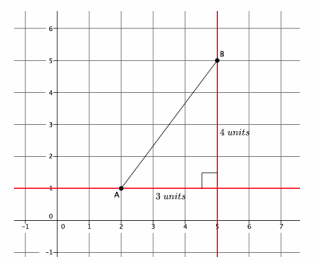
Find the length of the segment AB, if possible.
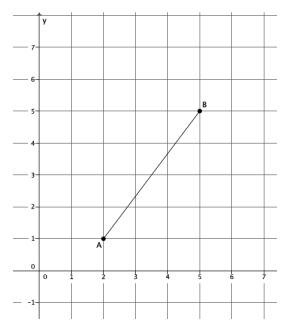
Answer:
If we let the length of segment AB be represented by c, then
32 +42 =c2
9+16=c2
25=c2
5=c.
The length of segment AB is 5 units.
Exercise 4.
Given a rectangle with dimensions 5 cm and 10 cm, as shown, find the length of the diagonal, if possible.
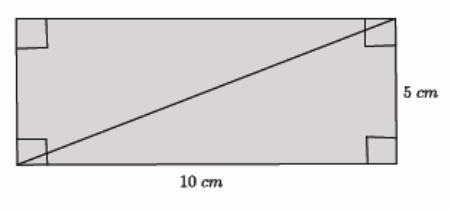
Answer:
Let c represent the length of the diagonal; then,
c2 =52 +102
c2 =25+100
c2 =125.
The measure of the length of the hypotenuse in centimeters is the positive number c that satisfies c2 =125.
Exercise 5.
A right triangle has a hypotenuse of length 13 in. and a leg with length 4 in. What is the length of the other leg?
Answer:
If we let a represent the length of the other leg, then
a2 +42 =132
a2 +42 -42 =132 -42
a2 =132 -42
a2 =169-16
a2 =153
The measure of the length of the leg in inches is the positive number a that satisfies a2 =153.
Exercise 6.
Find the length of b in the right triangle below, if possible.
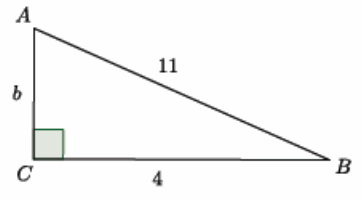
Answer:
By the Pythagorean theorem,
42 +b2 =112
42 -42 +b2 =112 -42
b2 =112 -42
b2 =121-16
a2 =105.
The length of side AC is the positive number b that satisfies b2 =105.
Eureka Math Grade 8 Module 2 Lesson 16 Problem Set Answer Key
Students practice using the Pythagorean theorem to find missing lengths in right triangles.
Question 1.
Find the length of the segment AB shown below, if possible.
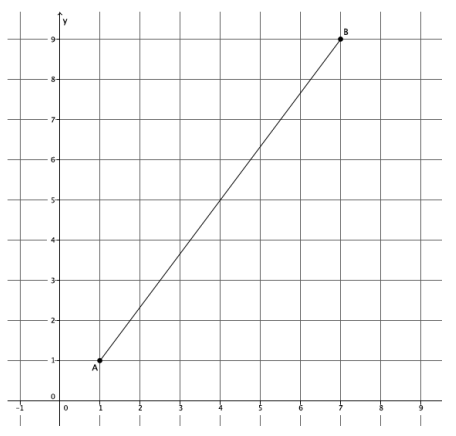
Answer:
If we let the length of segment AB be represented by c units, then by the Pythagorean theorem
62 +82 =c2
36+48=c2
100=c2
10=c
The length of the segment AB is 10 units.
Question 2.
A 20-foot ladder is placed 12 feet from the wall, as shown. How high up the wall will the ladder reach?
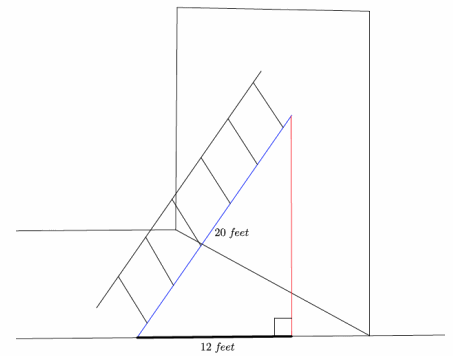
Answer:
Let the height up the wall that the ladder will reach be a feet. Then,
a2 +122 =202
a2 +122 -122 =202 -122
a2 =202 -122
a2 =400-144
a2 =256
a=16
The ladder will reach 16 feet up the wall.
Question 3.
A rectangle has dimensions 6 in. by 12 in. What is the length of the diagonal of the rectangle?
Answer:
Let the length of the diagonal be c inches. Then c is a positive number that satisfies
62 +122 =c2
36+144=c2
80=c2 .
The measure of the length of the diagonal in inches is the positive number c that satisfies c2 =180.
Use the Pythagorean theorem to find the missing side lengths for the triangles shown in Problems 4–8.
Question 4.
Determine the length of the missing side, if possible.
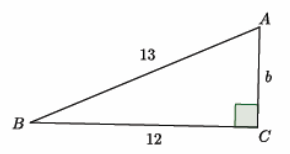
122+b2=132
122-122+b2=〖132-122
b2 =132-122
b2=169-144
b2=25
b=5
The length of the missing side is 5 units.
Question 5.
Determine the length of the missing side, if possible.
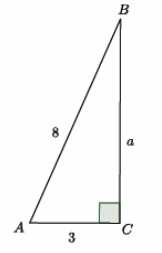
Answer:
a2+32=82
a2+32-32=82-32
a2=82-32
a2=64-9
a2=55)
The number of units of the side is given by the positive number a that satisfies a2=55.
Question 6.
Determine the length of the missing side, if possible.
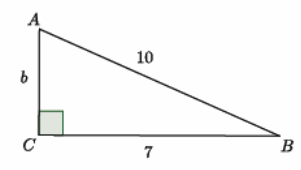
Answer:
72+b2=102
72-72+b2=102-72
b2=102-72
b2=100-49
b2=51
The number of units of the side is given by the positive number b that satisfies b2=51.
Question 7.
Determine the length of the missing side, if possible.
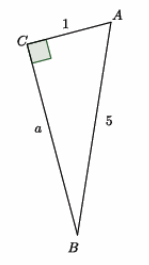
Answer:
a2+12=52
a2+12-12=52-12
a2=52-12
a2=25-1
a2=24
The number of units of the side is given by the positive number a that satisfies a2=24.
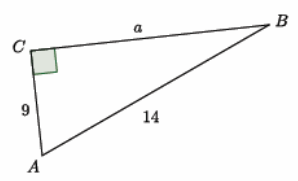
Question 8.
Determine the length of the missing side, if possible.
Answer:
a2+92=142
a2+92-92=142-92
a2=142-92
a2=196-81
a2=115
The number of units of the side is given by the positive number a that satisfies a2=115.
Eureka Math Grade 8 Module 2 Lesson 16 Exit Ticket Answer Key
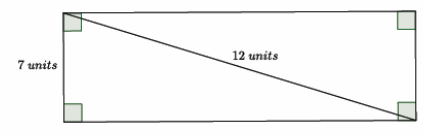
Question 1.
Find the length of the missing side of the rectangle shown below, if possible.
Answer:
Let the length of the unknown leg be a units. Then,
a2 +72 =122
a2 +72 -72 =122 -72
a2 =122 -72
a2 =144-49
a2 =95.
The number of units of the side is given by the positive number a that satisfies a2 =95.
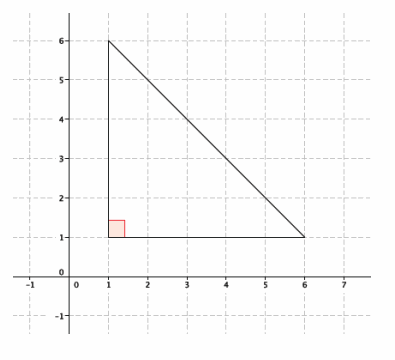
Question 2.
Find the length of all three sides of the right triangle shown below, if possible.
Answer:
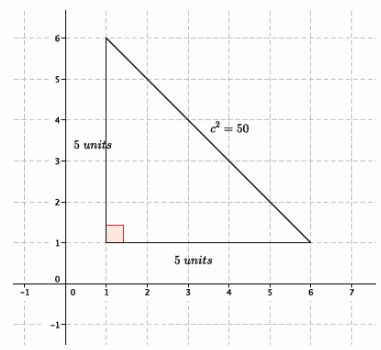
The two legs are each 5 units in length. If the hypotenuse is c units in length, then c is a positive number that satisfies
52+52=c2
25+25=c2
50=c2.
The length of the hypotenuse is given by the positive number c that satisfies c2 =50 units.