Engage NY Eureka Math 8th Grade Module 4 Lesson 26 Answer Key
Eureka Math Grade 8 Module 4 Lesson 26 Exercise Answer Key
Exercises
Exercise 1.
Sketch the graphs of the system.
y = \(\frac{2}{3}\) x + 4
y = \(\frac{4}{6}\) x – 3
Answer:
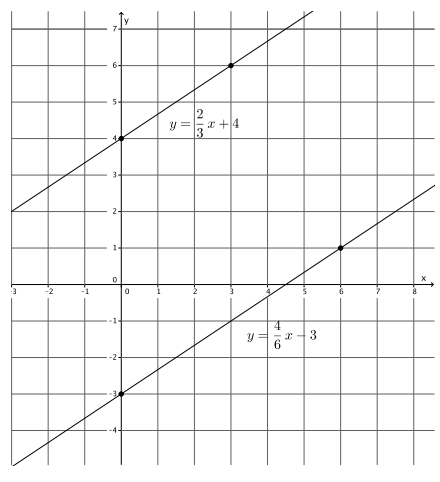
a. Identify the slope of each equation. What do you notice?
Answer:
The slope of the first equation is \(\frac{2}{3}\), and the slope of the second equation is \(\frac{4}{6}\). The slopes are equal.
b. Identify the y – intercept point of each equation. Are the y – intercept points the same or different?
Answer:
The y – intercept points are (0,4) and (0, – 3). The y – intercept points are different.
Exercise 2.
Sketch the graphs of the system.
y = – \(\frac{5}{4}\) x + 7
y = – \(\frac{5}{4}\) x + 2
Answer:
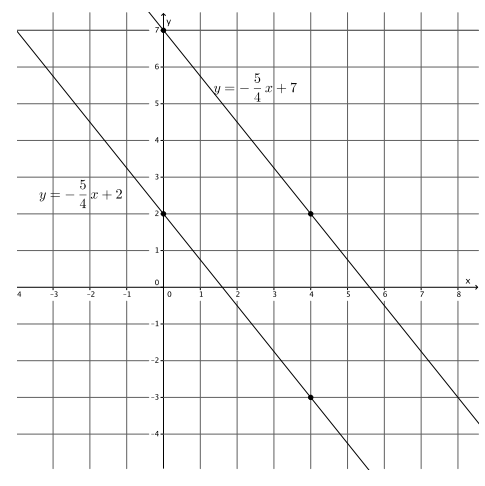
a. Identify the slope of each equation. What do you notice?
Answer:
The slope of both equations is – \(\frac{5}{4}\). The slopes are equal.
b. Identify the y – intercept point of each equation. Are the y – intercept points the same or different?
Answer:
The y – intercept points are (0,7) and (0,2). The y – intercept points are different.
Exercise 3.
Sketch the graphs of the system.
y = 2x – 5
y = 2x – 1)
Answer:
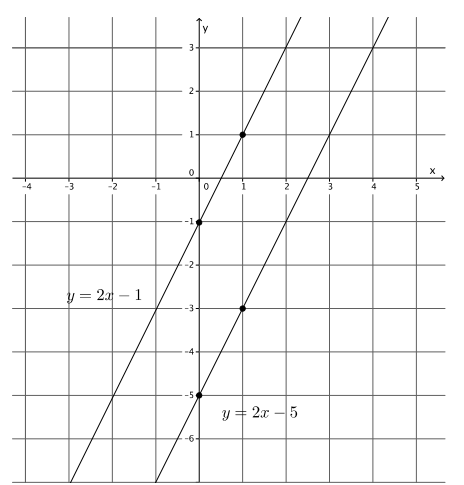
a. Identify the slope of each equation. What do you notice?
Answer:
The slope of both equations is 2. The slopes are equal.
b. Identify the y – intercept point of each equation. Are the y – intercept points the same or different?
Answer:
The y – intercept points are (0, – 5) and (0, – 1). The y – intercept points are different.
Exercise 4.
Write a system of equations that has no solution.
Answer:
Answers will vary. Verify that the system that has been written has equations that have the same slope and unique y – intercept points. Sample student solution: 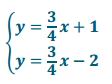
Exercise 5.
Write a system of equations that has (2,1) as a solution.
Answer:
Answers will vary. Verify that students have written a system where (2,1) is a solution to each equation. Sample student solution: 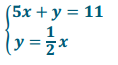
Exercise 6.
How can you tell if a system of equations has a solution or not?
Answer:
If the slopes of the equations are different, the lines will intersect at some point, and there will be a solution to the system. If the slopes of the equations are the same, and the y – intercept points are different, then the equations will graph as parallel lines, which means the system will not have a solution.
Exercise 7.
Does the system of linear equations shown below have a solution? Explain.
6x – 2y = 5
4x – 3y = 5
Answer:
Yes, this system does have a solution. The slope of the first equation is 3, and the slope of the second equation is \(\frac{4}{3}\). Since the slopes are different, these equations will graph as nonparallel lines, which means they will intersect at some point.
Exercise 8.
Does the system of linear equations shown below have a solution? Explain.
– 2x + 8y = 14
x = 4y + 1
Answer:
No, this system does not have a solution. The slope of the first equation is \(\frac{2}{8}\) = \(\frac{1}{4}\), and the slope of the second equation is \(\frac{1}{4}\). Since the slopes are the same, but the lines are distinct, these equations will graph as parallel lines. Parallel lines never intersect, which means this system has no solution.
Exercise 9.
Does the system of linear equations shown below have a solution? Explain.
12x + 3y = – 2
4x + y = 7
Answer:
No, this system does not have a solution. The slope of the first equation is – \(\frac{12}{3}\) = – 4, and the slope of the second equation is – 4. Since the slopes are the same, but the lines are distinct, these equations will graph as parallel lines. Parallel lines never intersect, which means this system has no solution.
Exercise 10.
Genny babysits for two different families. One family pays her $6 each hour and a bonus of $20 at the end of the night. The other family pays her $3 every half hour and a bonus of $25 at the end of the night. Write and solve the system of equations that represents this situation. At what number of hours do the two families pay the same for babysitting services from Genny?
Answer:
Let y represent the total amount Genny is paid for babysitting x hours. The first family pays y = 6x + 20. Since the other family pays by the half hour, 3∙2 would represent the amount Genny is paid each hour. So, the other family pays y = (3∙2)x + 25, which is the same as y = 6x + 25.
y = 6x + 20
y = 6x + 25
Since the equations in the system have the same slope and different y – intercept points, there will not be a point of intersection. That means that there will not be a number of hours for when Genny is paid the same amount by both families. The second family will always pay her $5 more than the first family.
Eureka Math Grade 8 Module 4 Lesson 26 Problem Set Answer Key
Answer Problems 1–5 without graphing the equations.
Question 1.
Does the system of linear equations shown below have a solution? Explain.
2x + 5y = 9
– 4x – 10y = 4
Answer:
No, this system does not have a solution. The slope of the first equation is – \(\frac{2}{5}\), and the slope of the second equation is – \(\frac{4}{10}\), which is equivalent to – \(\frac{2}{5}\). Since the slopes are the same, but the lines are distinct, these equations will graph as parallel lines. Parallel lines never intersect, which means this system has no solution.
Question 2.
Does the system of linear equations shown below have a solution? Explain.
\(\frac{3}{4}\) x – 3 = y
4x – 3y = 5
Answer:
Yes, this system does have a solution. The slope of the first equation is \(\frac{3}{4}\), and the slope of the second equation is \(\frac{4}{3}\). Since the slopes are different, these equations will graph as nonparallel lines, which means they will intersect at some point.
Question 3.
Does the system of linear equations shown below have a solution? Explain.
x + 7y = 8
7x – y = – 2
Answer:
Yes, this system does have a solution. The slope of the first equation is – \(\frac{1}{7}\), and the slope of the second equation is 7. Since the slopes are different, these equations will graph as nonparallel lines, which means they will intersect at some point.
Question 4.
Does the system of linear equations shown below have a solution? Explain.
y = 5x + 12
10x – 2y = 1
Answer:
No, this system does not have a solution. The slope of the first equation is 5, and the slope of the second equation is \(\frac{10}{2}\), which is equivalent to 5. Since the slopes are the same, but the lines are distinct, these equations will graph as parallel lines. Parallel lines never intersect, which means this system has no solution.
Question 5.
Does the system of linear equations shown below have a solution? Explain.
y = \(\frac{5}{3}\) x + 15
5x – 3y = 6
Answer:
No, this system does not have a solution. The slope of the first equation is \(\frac{5}{3}\), and the slope of the second equation is \(\frac{5}{3}\). Since the slopes are the same, but the lines are distinct, these equations will graph as parallel lines. Parallel lines never intersect, which means this system has no solution.
Question 6.
Given the graphs of a system of linear equations below, is there a solution to the system that we cannot see on this portion of the coordinate plane? That is, will the lines intersect somewhere on the plane not represented in the picture? Explain.
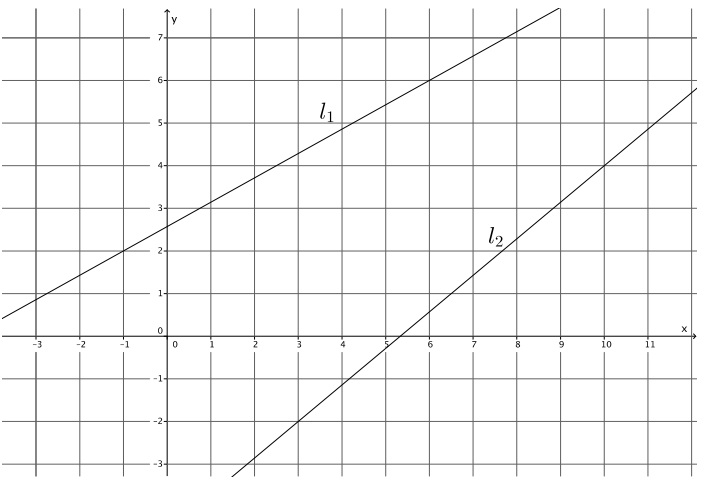
Answer:
The slope of l1 is \(\frac{4}{7}\), and the slope of l2 is \(\frac{6}{7}\). Since the slopes are different, these lines are nonparallel lines, which means they will intersect at some point. Therefore, the system of linear equations whose graphs are the given lines will have a solution.
Question 7.
Given the graphs of a system of linear equations below, is there a solution to the system that we cannot see on this portion of the coordinate plane? That is, will the lines intersect somewhere on the plane not represented in the picture? Explain.
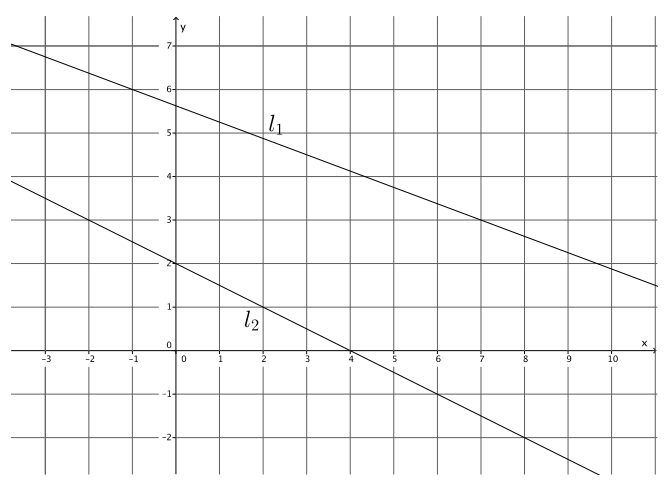
Answer:
The slope of l1 is – \(\frac{3}{8}\), and the slope of l2 is – \(\frac{1}{2}\). Since the slopes are different, these lines are nonparallel lines, which means they will intersect at some point. Therefore, the system of linear equations whose graphs are the given lines will have a solution.
Question 8.
Given the graphs of a system of linear equations below, is there a solution to the system that we cannot see on this portion of the coordinate plane? That is, will the lines intersect somewhere on the plane not represented in the picture? Explain.
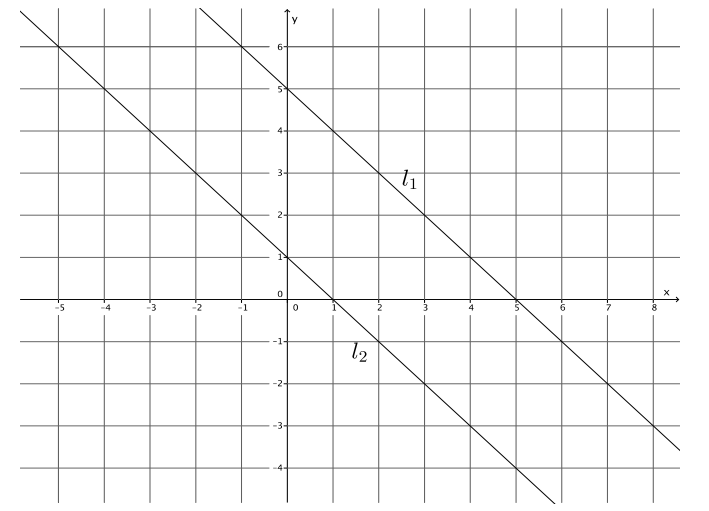
Answer:
The slope of l1 is – 1, and the slope of l2 is – 1. Since the slopes are the same the lines are parallel lines, which means they will not intersect. Therefore, the system of linear equations whose graphs are the given lines will have no solution.
Question 9.
Given the graphs of a system of linear equations below, is there a solution to the system that we cannot see on this portion of the coordinate plane? That is, will the lines intersect somewhere on the plane not represented in the picture? Explain.
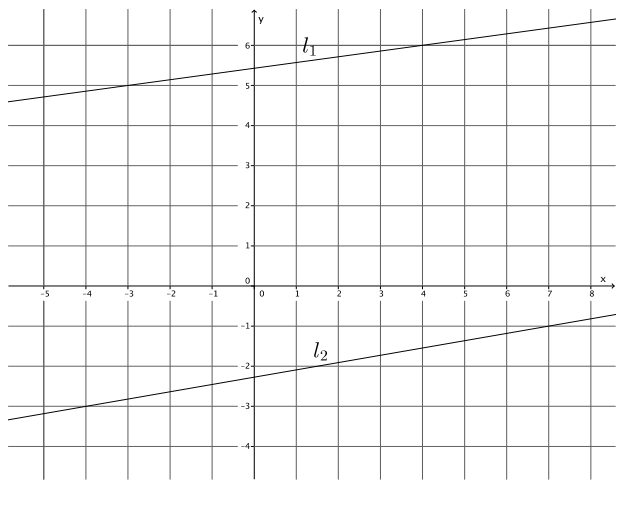
Answer:
The slope of l1 is \(\frac{1}{7}\), and the slope of l2 is \(\frac{2}{11}\). Since the slopes are different, these lines are nonparallel lines, which means they will intersect at some point. Therefore, the system of linear equations whose graphs are the given lines will have a solution.
Question 10.
Given the graphs of a system of linear equations below, is there a solution to the system that we cannot see on this portion of the coordinate plane? That is, will the lines intersect somewhere on the plane not represented in the picture? Explain.
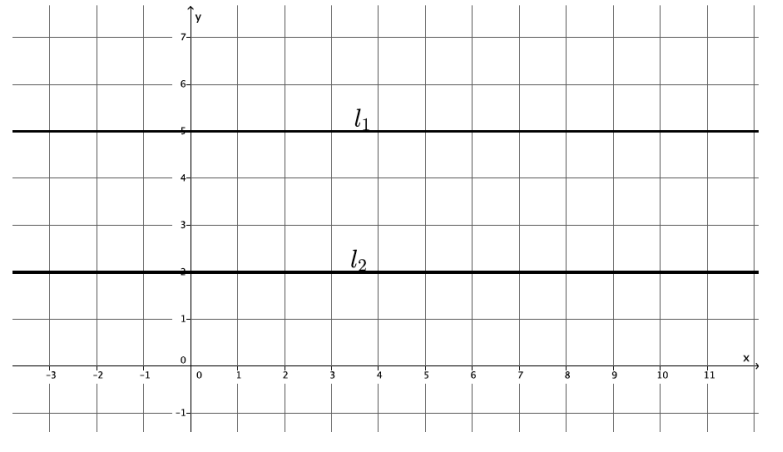
Answer:
Lines l1 and l1 are horizontal lines. That means that they are both parallel to the x – axis and, thus, are parallel to one another. Therefore, the system of linear equations whose graphs are the given lines will have no solution.
Eureka Math Grade 8 Module 4 Lesson 26 Exit Ticket Answer Key
Does each system of linear equations have a solution? Explain your answer.
Question 1.
y = \(\frac{5}{4}\) x – 3
y + 2 = \(\frac{5}{4}\) x
Answer:
No, this system does not have a solution. The slope of the first equation is \(\frac{5}{4}\) , and the slope of the second equation is \(\frac{5}{4}\). Since the slopes are the same, and they are distinct lines, these equations will graph as parallel lines. Parallel lines never intersect; therefore, this system has no solution.
Question 2.
y = \(\frac{2}{3}\) x – 5
4x – 8y = 11
Answer:
Yes, this system does have a solution. The slope of the first equation is \(\frac{2}{3}\), and the slope of the second equation is \(\frac{1}{2}\). Since the slopes are different, these equations will graph as nonparallel lines, which means they will intersect at some point.
Question 3.
\(\frac{1}{3}\) x + y = 8
x + 3y = 12
Answer:
No, this system does not have a solution. The slope of the first equation is – \(\frac{1}{3}\), and the slope of the second equation is – \(\frac{1}{3}\). Since the slopes are the same, and they are distinct lines, these equations will graph as parallel lines. Parallel lines never intersect; therefore, this system has no solution.