Eureka Math Precalculus Module 2 Lesson 23 Answer Key

Engage NY Eureka Math Precalculus Module 2 Lesson 23 Answer Key

Eureka Math Precalculus Module 2 Lesson 23 Example Answer Key

Example
An airplane flying from Dallas – Fort Worth to Atlanta veers off course to avoid a storm. The plane leaves Dallas – Fort Worth traveling 50° east of north and flies for 450 miles before turning to travel 70° east of south for 350 miles. What is the resultant displacement of the airplane? Include both the magnitude and direction of the displacement.

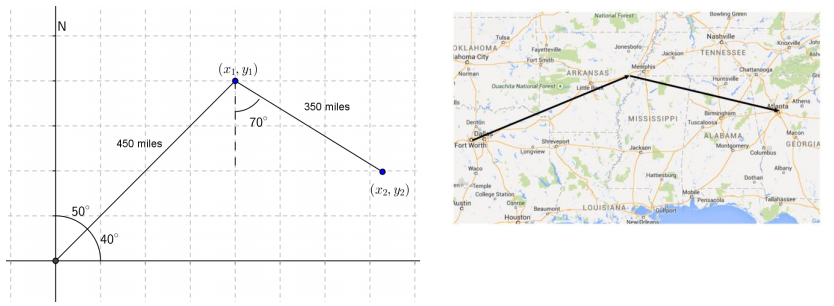
Answer:
x₁ = 450 cos⁡(40°) = 344.720
y₁ = 450 sin⁡(40°) = 289.254
x₂ = 344.720 + 328.892 = 673.612
y₂ = 289.254 – 119.707 = 169.547
d = \(\sqrt{673.612^{2} + 169.547^{2}}\) = 694.622
θ = tan ^{– 1}(\(\frac{169.547}{673.612}\)) = 14.1°

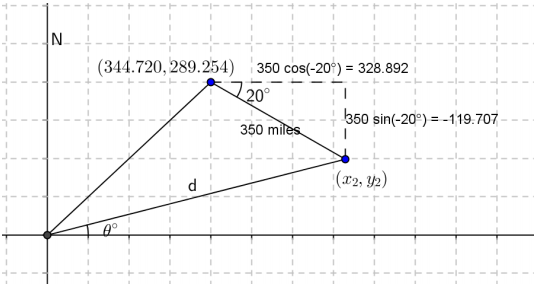
The resultant displacement is 694.622 miles at 75.9° east of north.

Eureka Math Precalculus Module 2 Lesson 23 Exercise Answer Key

Opening Exercise
Suppose a person walking through South Boston, MA travels from point A due east along E 3rd Street for 0.3 miles and then due south along K Street for 0.4 miles to end on point B (as shown on the map).

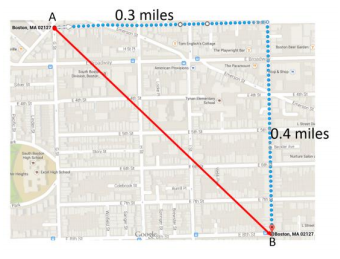
a. Find the magnitude and direction of vector \(\overrightarrow{A B}\).
|AB| = \(\sqrt{0.3^{2} + 0.4^{2}}\) = 0.5
The magnitude is 0.5 miles.
θ = tan ^{– 1}(\(\frac{0.4}{0.3}\)) = 53.1°

b. What information does vector \(\overrightarrow{A B}\) provide?
Answer:
The magnitude tells us that the person’s displacement was 0.5 miles. In other words, the person ended up 0.5 miles from the point where he or she started. The angle tells us that the person traveled 53.1° south of east.

Exercises

Exercise 1.
A motorized robot moves across the coordinate plane. Its position \(\left(\begin{array}{l}
x(t) \\
y(t)
\end{array}\right)\) at time t seconds is given by \(\left(\begin{array}{l}
x(t) \\
y(t)
\end{array}\right)\) = a + tv where a = \(\left(\begin{array}{c}
4 \\
– 10
\end{array}\right)\) and = \(\left(\begin{array}{c}
– 4 \\
3
\end{array}\right)\). The units of distance are measured in meters.
a. Where is the robot at time t = 0?
Answer:
\(\left(\begin{array}{c}
4 \\
– 10
\end{array}\right)\)

b. Plot the path of the robot.
Answer:

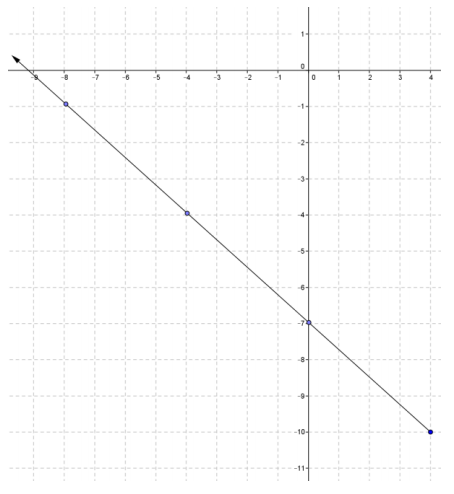
c. Describe the path of the robot.
Answer:
The robot is moving along the line y = – \(\frac{3}{4}\) x – 7.

d. Where is the robot 10 seconds after it starts moving?
Answer:
\(\left(\begin{array}{c}
– 36 \\
20
\end{array}\right)\)

e. Where is the robot when it is 10 meters from where it started?
Answer:
\(\left(\begin{array}{c}
– 4 \\
– 4
\end{array}\right)\)

f. Is the robot traveling at a constant speed? Explain, and if the speed is constant, state the robot’s speed.
Answer:
Yes, the robot is traveling at a constant speed of 5 m/s. For every one second that elapses, the robot moves 3 units up and 4 units to the left, which means the robot moves 5 meters every second.

Exercise 2.
A rowboat is crossing a river that is 500 m wide and traveling due east at a speed of 2.2 m/s. The river’s current is 0.8 m/s due south.
a. What is the resultant velocity of the boat?
Answer:
|v| = \(\sqrt{2.2^{2} + 0.8^{2}}\) = 2.34
The velocity is 2.34 m/s.
θ = tan ^{– 1}(\(\frac{0.8}{2.2}\)) = 20.0°

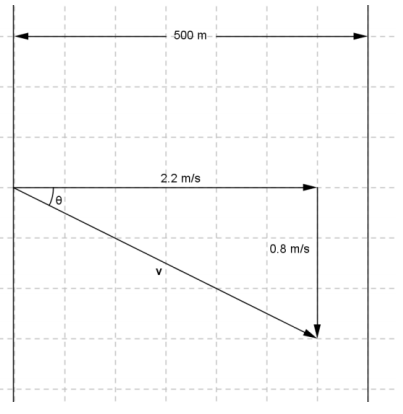
b. How long does it take for the boat to cross the river?
Answer:
t = \(\frac{500}{2.2}\) = 227.273
The time is 227.273 seconds.

c. How far downstream is the boat when it reaches the other side?
Answer:
d = 0.8(227.273) = 181.818 m
The distance is 181.818 meters.

Exercise 3.
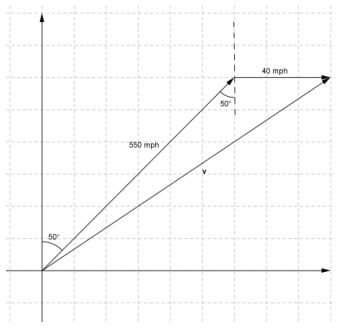
Consider the airplane from the Example that leaves Dallas – Fort Worth with a bearing of 50°. (Note that the bearing is the number of degrees east of north.) The plane is traveling at a speed of 550 mph. There is a crosswind of 40 mph due east. What is the resultant velocity of the airplane?
Answer:
|v| = \(\sqrt{550^{2} + 40^{2} – 2(550)(40) \cos \left(140^{\circ}\right)}\)
= 581.211
The velocity is 581.211 mph.

Exercise 4.
A raft floating in the water experiences an eastward force of 100 N due to the current of the water and a southeast force of 400 N due to wind.

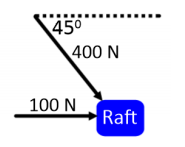
a. In what direction will the boat move?
Answer:
36.5° south of east

b. What is the magnitude of the resultant force on the boat?
Answer:
475.992 N

c. If the force due to the wind doubles, does the resultant force on the boat double? Explain or show work that supports your answer.
Answer:
No. If the force of the wind doubles, the resultant force on the boat is 873.577 N.

Eureka Math Precalculus Module 2 Lesson 23 Problem Set Answer Key

Question 1.
Suppose Madison is traveling due west for 0.5 miles and then due south for 1.2 miles.
a. Draw a picture of this scenario with her starting point labeled A, ending point B, and include the vector \(\overrightarrow{A B}\).
Answer:

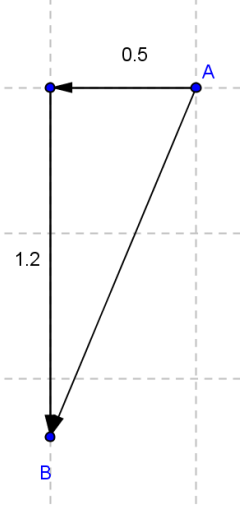
b. State the value of \(\overrightarrow{A B}\).
Answer:
\(\overrightarrow{A B}\) = \(\left(\begin{array}{l}
– 0.5 \\
– 1.2
\end{array}\right)\)

c. What is the magnitude and direction of \(\overrightarrow{A B}\)?
Answer:
\(\left\|\left(\begin{array}{l}
– 0.5 \\
– 1.2
\end{array}\right)\right\|\) = \(\sqrt{0.5^{2} + 1.2^{2}}\) = \(\sqrt{1.69}\) = 1.3
tan ^{– 1}(\(\frac{12}{5}\)) ≈ 67.380°⇒247.380°
The vector has a magnitude of 1.3 and a direction of 247.380° from east, or 67.380° south of west.

Question 2.
An object’s azimuth is the angle of rotation of its path measured clockwise from due north. For instance, an object traveling due north would have an azimuth of 0°, and due east would have an azimuth of 90°.
a. What are the azimuths for due south and due west?
Answer:
180° and 270°

b. Consider a craft on an azimuth of 215° traveling 30 knots.
i. Draw a picture representing the situation.
Answer:

ii. Find the vector representing this craft’s speed and direction.
Answer:
This vector is 215° from north, which is the same as saying 55° south of west. Thus, the x – coordinate of the vector will be 30 cos(55°) ≈ 17.207, and the y – coordinate will be 30 sin(55°) ≈ 24.575. The vector is represented by \(\left(\begin{array}{ll}
– 17.207 \\
– 24.575
\end{array}\right)\).

Question 3.
Bearings can be given from any direction, not just due north. For bearings, like azimuths, clockwise angles are represented by positive degrees and counterclockwise angles are represented by negative degrees. A ship is traveling 30° east of north at 18 kn, then turns 20°, maintaining its speed.
a. Draw a picture representing the situation.
Answer:

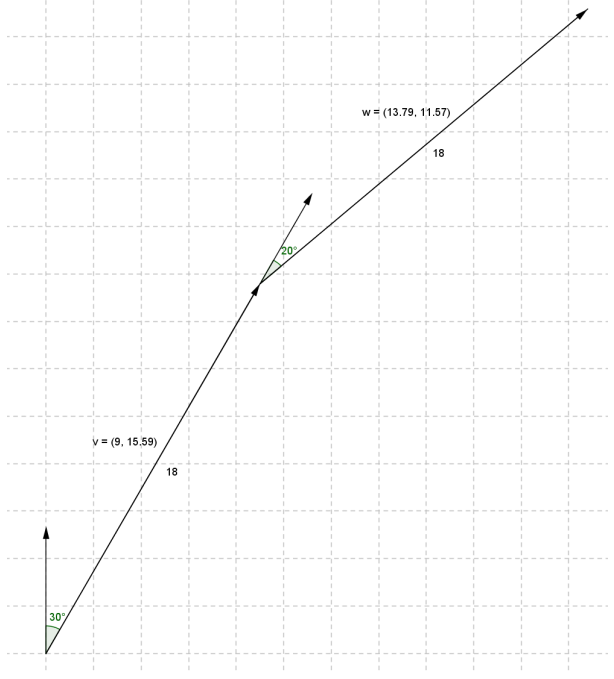
b. Find vectors v and w representing the first and second bearing.
Answer:
v = \(\left(\begin{array}{c}
9 \\
9 \sqrt{3}
\end{array}\right)\) ≈ \(\left(\begin{array}{c}
9 \\
15.588
\end{array}\right)\), w = \(\left(\begin{array}{l}
18 \sin \left(50^{\circ}\right) \\
18 \cos \left(50^{\circ}\right)
\end{array}\right)\) ≈ \(\left(\begin{array}{l}
13.789 \\
11.570
\end{array}\right)\)

c. Find the sum of v and w. What does v + w represent?
Answer:
v + w ≈ \(\left(\begin{array}{l}
22.789 \\
27.159
\end{array}\right)\)
The sum represents the ship’s position relative to its starting point.

d. If the ship travels for one hour along each bearing, then how far north of its starting position has it traveled? How far east has it traveled?
Answer:
The ship has traveled 22.789 nautical miles north and 27.159 nautical miles east.

Question 4.
A turtle starts out on a grid with coordinates \(\left(\begin{array}{c}
4 \\
– 6.5
\end{array}\right)\) where each unit is one furlong. Its horizontal location is given by the function x(t) = 4 + – 2t, and its vertical location is given by y(t) = – 6.5 + 3t for t in hours.
a. Write the turtle’s location using vectors.
Answer:
\(\left(\begin{array}{l}
x(t) \\
y(t)
\end{array}\right)\) = \(\left(\begin{array}{c}
4 \\
– 6.5
\end{array}\right) + \left(\begin{array}{c}
– 2 \\
3
\end{array}\right) t\)

b. What is the speed of the turtle?
Answer:
\(\sqrt{2^{2} + 3^{2}}\) = \(\sqrt{13}\)
The turtle is traveling at \(\sqrt{13}\) furlongs per hour.

c. If a hare’s location is given as \(\left(\begin{array}{l}
x_{h}(t) \\
y_{h}(t)
\end{array}\right)\) = a + tv where a = \(\left(\begin{array}{c}
23 \\
– 35
\end{array}\right)\) and v = \(\left(\begin{array}{c}
– 8 \\
12
\end{array}\right)\), then what is the speed of the hare? How much faster is the hare traveling than the turtle?
Answer:
\(\sqrt{8^{2} + 12^{2}}\) = \(\sqrt{208}\)
\(\sqrt{208}\) = 4\(\sqrt{13}\)
The hare is traveling at a speed of \(\sqrt{208}\) furlongs per hour, which is 4 times faster than the turtle.

d. Which creature will reach \(\left(\begin{array}{c}
– 1 \\
1
\end{array}\right)\) first?
Answer:
The turtle will reach \(\left(\begin{array}{c}
– 1 \\
1
\end{array}\right)\) at t = 2.5, and the hare will reach it at t = 3, so the turtle will beat the hare by half an hour.

Question 5.
A rocket is launched at an angle of 33° from the ground at a rate of 50 m/s.
a. How fast is the rocket traveling up, to the nearest m/s?
Answer:
50 ⋅ sin(33°) ≈ 27
The rocket is traveling up at a rate of approximately 27 m/s.

b. How fast is the rocket traveling to the right, to the nearest m/s?
Answer:
50 ⋅ cos(33°) ≈ 42
The rocket is traveling to the right at a rate of approximately 42 m/s.

c. What is the rocket’s velocity vector?
Answer:
The velocity vector is \(\left(\begin{array}{l}
42 \\
27
\end{array}\right)\).

d. Does the magnitude of the velocity vector agree with the setup of the problem? Why or why not?
Answer:
\(\left\|\left(\begin{array}{c}
42 \\
27
\end{array}\right)\right\|\) = \(\sqrt{42^{2} + 27^{2}}\) = \(\sqrt{2493}\) ≈ 49.92995
This is effectively the original speed and is only different because of rounding errors.

e. If a laser is in the path of the rocket and would like to strike the rocket, in what direction should the laser be aimed? Express your answer as a vector.
Answer:
If the laser is in the path of the rocket, then it should be aimed in the opposite direction the rocket is traveling, which is \(\left(\begin{array}{l}
– 42 \\
– 27
\end{array}\right)\).

Question 6.
A boat is drifting downriver at a rate of 5 nautical miles per hour. If the occupants of the boat want to travel to shore, do they need to overcome the current downriver? Use vectors to explain why or why not.
Answer:
The occupants do not need to overcome the current since the boat is moving downriver, and they only want to move perpendicular to the direction the stream is moving. Since they will be moving perpendicular, their motions will have no impact on the downriver rate, nor should they. Any effort fighting the current only wastes resources. Orthogonal vectors do not affect the position.

Question 7.
A group of friends moored their boats together and fell asleep on the lake. Unfortunately, their lashings came undone in the night, and they drifted apart. Gerald’s boat traveled due west along with the current of the lake which moves at a rate of \(\frac{1}{2}\) mi/hr, and Helena’s boat was pulled southeast by some pranksters and set drifting at a rate of 2 mi/hr.
a. If the boats came untied three hours ago, how far apart are the boats?
Answer:
\(\sqrt{(1.5)^{2} + 6^{2} – 2 \cdot 1.5 \cdot 6 \cdot \cos \left(135^{\circ}\right)}\) = \(\sqrt{2.25 + 36 + 9 \sqrt{2}}\) ≈ 7.13
Gerald traveled 1.5 miles to the west, and Helena traveled 6 miles to the southeast. Using the law of cosines, we see that they are approximately 7.13 miles away from each other.

b. If Gerald drops anchor, then in what direction should Helena travel in order to reunite with Gerald?
Answer:
\(\left(\begin{array}{c}
1.5 + 3 \sqrt{2} \\
3 \sqrt{2}
\end{array}\right)\)

Question 8.
Consider any two vectors in space, u and v with θ the angle between them.
a. Use the law of cosines to find the value of ‖u – v ‖.
‖u – v‖² = ‖u‖² + ‖v‖² – 2‖u‖‖v‖cos(θ)
‖u – v‖ = \(\sqrt{\|\mathrm{u}\|^{2} + \|\mathrm{v}\|^{2} – 2\|\mathrm{u}\|\|\mathrm{v}\| \cos (\theta)}\)

b. Use the law of sines to find the value of ψ, the angle between u – v and u. State any restrictions on the variables.
Answer:
\(\frac{\sin (\theta)}{\|\mathrm{u} – \mathrm{v}\|} = \frac{\sin (\psi)}{\|\mathrm{v}\|}\)
ψ = sin ^{– 1}\(\left(\frac{\|\mathrm{v}\| \sin (\theta)}{\|\mathrm{u} – \mathrm{v}\|}\right)\)
The inverse sine only returns values between – 90° and 90°, so if the angle is greater than this, then trig identities should be used to help find the value.

Eureka Math Precalculus Module 2 Lesson 23 Exit Ticket Answer Key

Question 1.
A hailstone is traveling through the sky. Its position \(\left(\begin{array}{l}
x(t) \\
y(t) \\
z(t)
\end{array}\right)\)in meters is given by \(\left(\begin{array}{l}
x(t) \\
y(t) \\
z(t)
\end{array}\right)\) = \(\left(\begin{array}{c}
0 \\
0 \\
2160
\end{array}\right) + \left(\begin{array}{c}
3 \\
– 2 \\
– 9
\end{array}\right)\)t where t is the time in seconds since the hailstone began being tracked.
a. If x(t) represents its east – west location, how quickly is the hailstone moving to the east?
Answer:
3 m/s

b. If y(t) represents its north – south location, how quickly is the hailstone moving to the south?
Answer:
2 m/s

c. What could be causing the east – west and north – south velocities for the hailstone?
Answer:
Wind is probably the number one factor affecting the speed of the hailstone.

d. If z(t) represents the height of the hailstone, how quickly is the hailstone falling?
Answer:
9 m/s

e. At what location will the hailstone hit the ground (assume z = 0 is the ground)? How long will this take?
Answer:
t = 2160 ÷ 9 = 240
The hailstone will hit the ground at 240 seconds, which is 4 minutes. In 4 minutes it will move 720 meters to the east and 480 meters to the south to impact at \(\left(\begin{array}{c}
720 \\
480 \\
0
\end{array}\right)\).

f. What is the overall speed of the hailstone? To the nearest meter, how far did the hailstone travel from t = 0 to when it hit the ground?
Answer:
\(\sqrt{3^{2} + 2^{2} + 9^{2}}\) = \(\sqrt{94}\)
240\(\sqrt{94}\) ≈ 2327
The hailstone is traveling at \(\sqrt{94}\) meters per second. It traveled approximately 2327 meters.