Eureka Math Precalculus Module 3 Lesson 9 Answer Key

Engage NY Eureka Math Precalculus Module 3 Lesson 9 Answer Key

Eureka Math Precalculus Module 3 Lesson 9 Exercise Answer Key

Exercises
Exercise 1.
Let R = 5, and let A(x) represent the area of a cross section for a circle at a distance x from the center of the sphere.

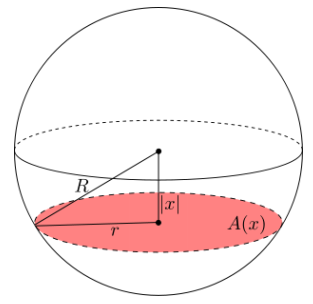
“Sphere with Cross Section” by Theneb 314 is licensed under CC BY-SA 3.0
http://creativecommons.org/licenses/by-sa/3.0/deed.en
a. Find A(0). What is special about this particular cross section?
Answer:
A(0) = π∙5² = 25π. This is the largest cross section in the sphere; it’s the area of a “great circle.”

b. Find A(1).
Answer:
When x = 1, we have r²+1² = 5². Thus, A(1) = π∙r² = π∙(5²-1² ) = π∙(25-1) = 24π.

c. Find A(3).
Answer:
When x = 3, we have r²+3² = 5². Thus, A(3) = π∙r² = π∙(5²-3² ) = π∙(25-9) = 16π.

d. Find A(4).
Answer:
When x = 4, we have r²+4² = 5². Thus, A(4) = π∙r² = π∙(5²-4² ) = π∙(25-16) = 9π.

e. Find A(5). What is special about this particular cross section?
Answer:
A(5) = 0. When the plane reaches the point where x = 5, the cross section is a single point, so the area vanishes.

Exercise 2.
Let the radius of the cylinder be R = 5, and let B(x) represent the area of the blue ring when the slicing plane is at a distance x from the top of the cylinder.

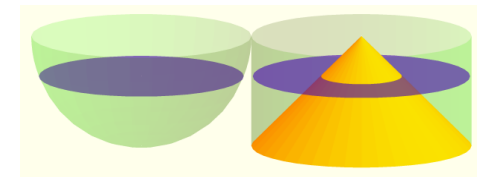
© Roberto Cardil, Matematicas Visuales
http://www.matematicasvisuales.com
a. Find B(1). Compare this area with A(1), the area of the corresponding slice of the sphere.
Answer:
B(1) = π∙5²-π∙1² = 25π-1π = 24π This is equivalent to A(1).

b. Find B(2). Compare this area with A(2), the area of the corresponding slice of the sphere.
Answer:
B(2) = π∙5²-π∙2² = 25π-4π = 21π This is equivalent to A(2).

c. Find B(3). Compare this area with A(3), the area of the corresponding slice of the sphere.
Answer:
B(3) = π∙5²-π∙3² = 25π-9π = 16π This is equivalent to A(3).

Exercise 3.
Explain how to derive the formula for the volume of a sphere with radius r.

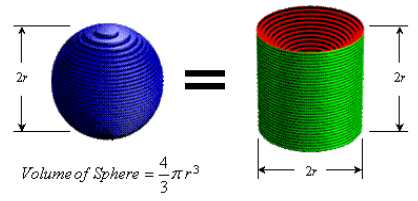
© Joe Mercer
http://www.ceemrr.com
Answer:
If we pass a plane through the sphere that is parallel to the bases of the cylinder at a distance x from the center of the sphere, we get a circle with area A(x) = π∙(r²-x²).
When the same plane intersects the cylinder, a ring is formed around the double cone. The area of this ring is B(x) = π∙r²-π∙x².
It’s easy to see that A(x) = B(x), and since both solids have height 2r, it follows from Cavalieri’s principle that the volume of the sphere is equal to the volume of the space outside the double cone.
The volume of the cylinder is V = π∙r²∙2r = 2π∙r³.
Thus, the volume of the double cone is V = \(\frac{1}{3}\)∙2π∙r³.
The volume of the space outside the double cone is therefore V = \(\frac{2}{3}\)∙2π∙r³ = \(\frac{4}{3}\) π∙r³. Since the sphere also has this volume, this is the formula for the volume of the sphere.

Eureka Math Precalculus Module 3 Lesson 9 Problem Set Answer Key

Question 1.
Consider the sphere with radius r = 4. Suppose that a plane passes through the sphere at a height y = 2 units above the center of the sphere, as shown in the figure below.

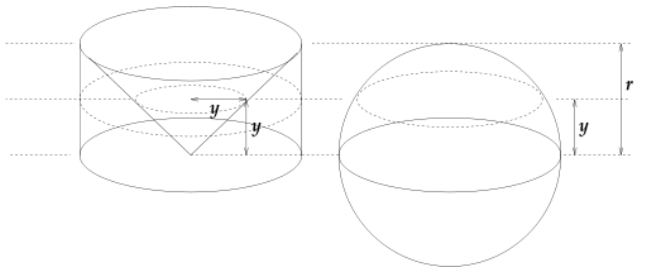
a. Find the area of the cross section of the sphere.
Answer:
The sphere has radius 4, and the cross section passes through at y = 2, which tells us that the radius at the cross section is r = \(\sqrt{4^{2}-2^{2}}\) = \(\sqrt{12}\) = 2\(\sqrt{3}\). Thus, the area of the cross section is 12π.

b. Find the area of the cross section of the cylinder that lies outside of the cone.
Answer:
The cone has radius equal to its height at the cross section, and the circle passing through it has radius equal to 4 (since the radius of the cylinder is constant).

c. Find the volume of the cylinder, the cone, and the hemisphere shown in the figure.
Answer:
The volume of the cylinder is V = πr² h = 64π cubic units. The volume of the cone is
V = \(\frac{1}{3}\) πr² h = \(\frac{64}{3}\) π cubic units. The volume of the hemisphere is twice the volume of the cone, so \(\frac{128}{3}\) π cubic units.

d. Find the volume of the sphere shown in the figure.
Answer:
The sphere is twice the volume of the hemisphere, so \(\frac{256}{3}\) π cubic units.

e. Explain using Cavalieri’s principle the formula for the volume of any single solid.
Answer:
Cavalieri’s principle tells us that to find the volume of a solid, we can examine cross sections of the solid. If another shape exists with the same height and equal areas of cross sections, then the two shapes have equal volume.

Question 2.
Give an argument for why the volume of a right prism is the same as an oblique prism with the same height.
Answer:
Since the cross sections of both a right prism and an oblique prism would be the same shape (same area), it does not matter if the object is on a slant or straight up and down; they have the same volume.

Question 3.
A paraboloid of revolution is a three-dimensional shape obtained by rotating a parabola around its axis. Consider the solid between a paraboloid described by the equation y = x² and the line y = 1.

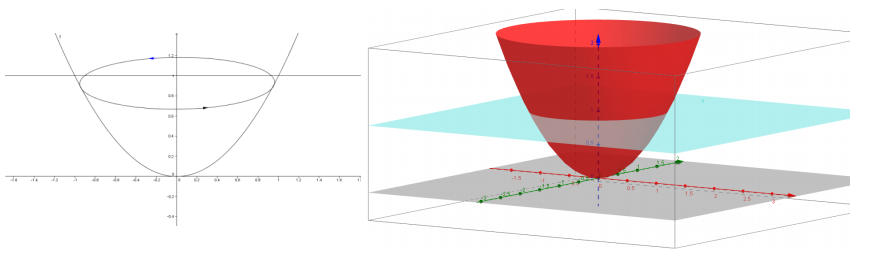
a. Cross sections perpendicular to the y-axis of this paraboloid are what shape?
Answer:
Circles

b. Find the area of the largest cross section of this solid, when y = 1.
Answer:
When y = 1,x = 1, so π1² = π.

c, Find the area of the smallest cross section of this solid, when y = 0.
Answer:
When y = 0, x = 0, so π0² = 0.

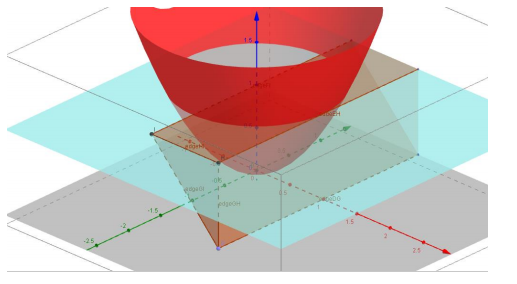
d. Consider a right triangle prism with legs of length 1, hypotenuse of length \(\sqrt{2}\), and depth π as pictured below. What shape are the cross sections of the prism perpendicular to the y-axis?

Answer:
Cross sections are rectangles.

e. Find the areas of the cross sections of the prism at y = 1 and y = 0.
Answer:
At y = 1, the width is 1, and the depth is π, so π ⋅ 1 = π.
At y = 0, the width is 0, and the depth is π, so π ⋅ 0 = 0.

f. Verify that at y = y₀, the areas of the cross sections of the paraboloid and the prism are equal.
Answer:
At y₀, the cross sections of the paraboloid have radius x = \(\sqrt{y_{0}}\), so the area is π(\(\sqrt{y_{0}}\))² = y₀ π.
Similarly, the width of the rectangle is equal to the height of the prism, so at y₀, the width is y₀, and the depth is a constant π, so the area is y₀ π.

g. Find the volume of the paraboloid between y = 0 and y = 1.
Answer:
The volume of the paraboloid is equal to the volume of the right triangular prism, which has volume V = \(\frac{1}{2}\) abh = \(\frac{1}{2}\) ⋅ 1 ⋅ 1 ⋅ π = \(\frac{\pi}{2}\).

h. Compare the volume of the paraboloid to the volume of the smallest cylinder containing it. What do you notice?
Answer:
The volume of the paraboloid is half the volume of the cylinder.

i. Let V_cyl be the volume of a cylinder, V_par be the volume of the inscribed paraboloid, and V_cone be the volume of the inscribed cone. Arrange the three volumes in order from smallest to largest.
Answer:
V_cone < V_par < V_cyl since V_cone = \(\frac{1}{3}\) V_cyl and V_par = \(\frac{1}{2}\) V_cyl

Question 4.
Consider the graph of f described by the equation f(x) = \(\frac{1}{2}\) x² for 0 ≤ x ≤ 10.
a. Find the area of the 10 rectangles with height f(i) and width 1, for i = 1, 2, 3, … , 10.
Answer:
In each case, the width is one, so the area is equal to the height of the function at that point; we get
\(\frac{1}{2}\), 2, \(\frac{9}{2}\), 8, \(\frac{25}{2}\), 18, \(\frac{49}{2}\), 32, \(\frac{81}{2}\), 50.

b. What is the total area for 0 ≤ x ≤ 10? That is, evaluate \(\sum_{i = 1}^{10}\)f(i) ⋅ Δx for Δx = 1.
Answer:
\(\sum_{i = 1}^{10}\)f(i) ⋅ Δx = 192.5

c. Draw a picture of the function and rectangles for i = 1, 2, 3.
Answer:

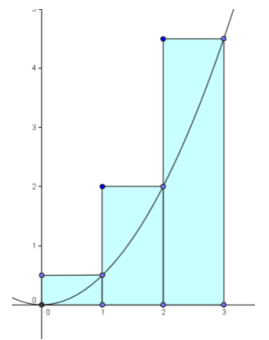
d. Is your approximation an overestimate or an underestimate?
Answer:
Since each rectangle contains more area than is under the parabola, the estimate is an overestimate.

e. How could you get a better approximation of the area under the curve?
Answer:
Answers may vary and may include that you could find smaller rectangles, you could find the underestimate by using the left endpoints, and you could cut off the triangles above the function to get a trapezoid.

Question 5.
Consider the three-dimensional solid that has square cross sections and whose height y at position x is given by the equation y = 2\(\sqrt{x}\) for 0 ≤ x ≤ 4.
a. Approximate the shape with four rectangular prisms of equal width. What is the height and volume of each rectangular prism? What is the total volume?
Answer:
The heights are 2, 2\(\sqrt{2}\), 2\(\sqrt{3}\), 4, and the volumes are 4, 8, 12, 16. The total volume is 40.

b. Approximate the shape with eight rectangular prisms of equal width. What is the height and volume of each rectangular prism? What is the total volume?
Answer:
The heights are 2\(\sqrt{\frac{1}{2}}\), 2, 2\(\sqrt{\frac{3}{2}}\), 2\(\sqrt{2}\), 2\(\sqrt{\frac{5}{2}}\), 2\(\sqrt{3}\), 2\(\sqrt{\frac{7}{2}}\), 4, and the volumes are 1, 2, 3, 4, 5, 6, 7, 8. The total volume is 36.

c. How much did your approximation improve? The volume of the shape is 32 cubic units. How close is your approximation from part (b)?
Answer:
The approximation improved by 4 cubic units. The approximation is off by 4 cubic units.

d. How many rectangular prisms would you need to be able to approximate the volume accurately?
Answer:
It is hard to say, but many would be needed to get significant accuracy, although this could be reduced by taking both an upper and a lower bound.

Eureka Math Precalculus Module 3 Lesson 9 Exit Ticket Answer Key

Question 1.
Explain how Cavalieri’s principle can be used to find the volume of any solid.
Answer:
Cavalieri’s principle tells us that to find the volume of a solid, we can examine cross sections of the solid. If another shape exists with the same height and equal areas of cross sections, then the two shapes will have equal volume.