Looking everywhere for Go Math Grade 5 Answer Key Chapter 5 Divide Decimals? Yes, you have come the right way and we have curated the Go Math Grade 5 Answer Key. Access the Step by Step Solutions provided for the Questions from Chapter Test, Practice Test, Cumulative Practice. Refer to the HMH Go math Grade 5 Chapter 5 Divide Decimals Solution Key to cross-check your Answers. Practice all Grade 5 Go Math Chapter 5 Divide Decimals for free of cost and score well.
Go Math Grade 5 Chapter 5 Divide Decimals Answer Key
You can improve math proficiency by practicing with the Go Math Grade 5 Chapter 5 Answer Key on a regular basis. The Best Guide to Prepare Math is to refer to HMH Go Math Grade 5 Answer Key. Solve as many times as possible and try to cover all the topics in it via quick links out there. Simply click on the topics you want to prepare and check all the Problems in it.
Lesson 1: Algebra • Division Patterns with Decimals
- Division Patterns with Decimals – Page No. 203
- Division Patterns with Decimals Lesson Check – Page No. 204
Lesson 2: Investigate • Divide Decimals by Whole Numbers
- Divide Decimals by Whole Numbers – Page No. 207
- Divide Decimals by Whole Numbers Lesson Check – Page No. 208
Lesson 3: Estimate Quotients
Lesson 4: Division of Decimals by Whole Numbers
- Division of Decimals by Whole Numbers – Page No. 215
- Division of Decimals by Whole Numbers Lesson Check – Page No. 216
Mid-Chapter Checkpoint
Lesson 5: Investigate • Decimal Divisions
Lesson 6: Divide Decimals
Lesson 7: Write Zeros in the Dividend
Lesson 8: Problem Solving • Decimal Operations
Chapter 5 Review/Test
- Review/Test – Page No. 235
- Review/Test – Page No. 236
- Review/Test – Page No. 237
- Review/Test – Page No. 238
Share and Show – Page No. 203
Complete the pattern.
Question 1.
456 ÷ 100 = 456
456 ÷ 101 = 45.6
456 ÷ 102 = 4.56
Think: The dividend is being divided by an increasing power of 10, so the decimal point will move to the left one place for each increasing power of 10.
456 ÷ 103 = _____
Answer:
456 ÷ 103 = 0.456
Explanation:
The dividend is being divided by an increasing power of 10, so the decimal point will move to the left one place for each increasing power of 10.
456 ÷ 100 = 456
456 ÷ 101 = 45.6
456 ÷ 102 = 4.56
456 ÷ 103 = 0.456
Complete the pattern.
Question 2.
225 ÷ 100 = _____
225 ÷ 101 = _____
225 ÷ 102 = _____
225 ÷ 103 = _____
Answer:
225 ÷ 100 = 225
225 ÷ 101 = 22.5
225 ÷ 102 = 2.25
225 ÷ 103 = 0.25
Explanation:
The dividend is being divided by an increasing power of 10, so the decimal point will move to the left one place for each increasing power of 10.
225 ÷ 100 = 225/1 = 225
225 ÷ 101 = 225/10 = 22.5
225 ÷ 102 = 225/100 = 2.25
225 ÷ 103 = 225/1,000 = 0.25

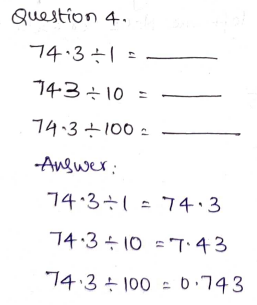

On Your Own
Complete the pattern.
Question 5.
156 ÷ 1 = _____
156 ÷ 10 = _____
156 ÷ 100 = _____
156 ÷ 1,000 = _____
Answer:
156 ÷ 1 = 156
156 ÷ 10 = 15.6
156 ÷ 100 = 1.56
156 ÷ 1,000 = 0.156
Explanation:
The dividend is being divided by an increasing power of 10, so the decimal point will move to the left one place for each increasing power of 10.
156 ÷ 1 = 156
156 ÷ 10 = 15.6
156 ÷ 100 = 1.56
156 ÷ 1,000 = 0.156
Question 6.
32 ÷ 1 = _____
32 ÷ 10 = _____
32 ÷ 100 = _____
32 ÷ 1,000 = _____
Answer:
32 ÷ 1 = 32
32 ÷ 10 = 3.2
32 ÷ 100 = 0.32
32 ÷ 1,000 = 0.032
Explanation:
The dividend is being divided by an increasing power of 10, so the decimal point will move to the left one place for each increasing power of 10.
32 ÷ 1 = 32
32 ÷ 10 = 3.2
32 ÷ 100 = 0.32
32 ÷ 1,000 = 0.032
Question 7.
16 ÷ 100 = _____
16 ÷ 101 = _____
16 ÷ 102 = _____
16 ÷ 103 = _____
Answer:
16 ÷ 100 = 16
16 ÷ 101 = 1.6
16 ÷ 102 = 0.16
16 ÷ 103 = 0.016
Explanation:
The dividend is being divided by an increasing power of 10, so the decimal point will move to the left one place for each increasing power of 10.
16 ÷ 100 = 16
16 ÷ 101 = 1.6
16 ÷ 102 = 0.16
16 ÷ 103 = 0.016
Question 8.
12.7 ÷ 1 = _____
12.7 ÷ 10 = _____
12.7 ÷ 100 = _____
12.7 ÷ 1,000 = _____
Answer:
12.7 ÷ 1 = 12.7
12.7 ÷ 10 = 1.27
12.7 ÷ 100 = 0.127
12.7 ÷ 1,000 = 0.0127
Explanation:
The dividend is being divided by an increasing power of 10, so the decimal point will move to the left one place for each increasing power of 10.
12.7 ÷ 1 = 12.7
12.7 ÷ 10 = 1.27
12.7 ÷ 100 = 0.127
12.7 ÷ 1,000 = 0.0127


Algebra Find the value of n.
Question 11.
268 ÷ n = 0.268
n = _____
Answer:
n = 1000
Explanation:
268 ÷ n = 0.268
268 = n x 0.268
n = 268 ÷ 0.268
n = 1000
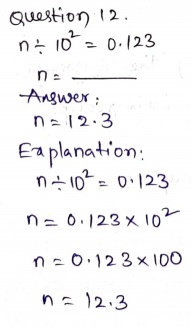
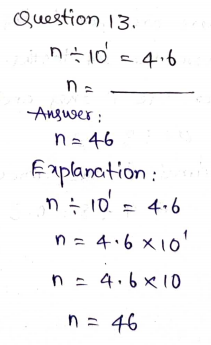
Problem Solving – Page No. 204
Use the table to solve 14–16.
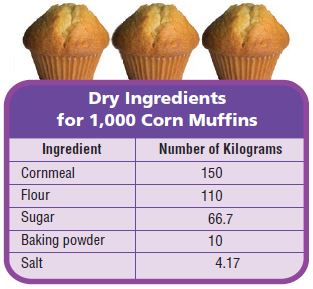
Question 14.
If each muffin contains the same amount of cornmeal, how many kilograms of cornmeal are in each corn muffin?
_____ kilograms
Answer:
0.15 kilograms
Explanation:
There are 1,000 muffins. Cornmeal = 150 Kg
If each muffin contains the same amount of cornmeal, 150 ÷ 1000 = 0.15
0.15 kilograms of cornmeal is in each corn muffin
Question 15.
If each muffin contains the same amount of sugar, how many kilograms of sugar, to the nearest thousandth, are in each corn muffin?
_____ kilograms
Answer:
0.07 kilograms
Explanation:
There are 1,000 muffins. Sugar = 66.7 kilograms
If each muffin contains the same amount of sugar, 66.7 ÷ 1000 = 0.0667.
0.0667 kilograms of sugar is in each corn muffin.
The thousandth digit is 6. 6 > 5
So, 0.07
Question 16.
The bakery decides to make only 100 corn muffins on Tuesday. How many kilograms of sugar will be needed?
_____ kilograms
Answer:
0.007 kilograms
Explanation:
The bakery decides to make only 100 corn muffins on Tuesday.
As 0.07 kilograms are required for 1,000 muffins,
for 100 muffins, (100 x 0.07) ÷ 1000 = 0.007
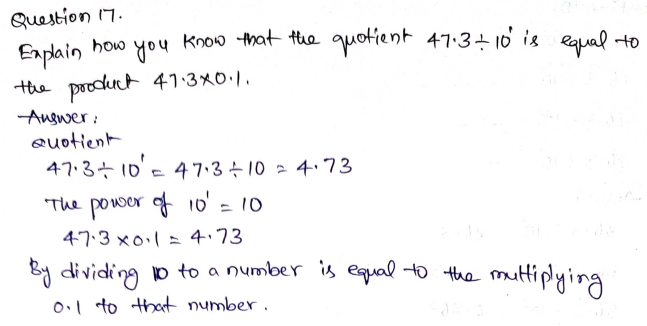
Question 18.
Test Prep Ella used 37.2 pounds of apples to make applesauce. She used one-tenth as many pounds of sugar as pounds of apples. How many pounds of sugar did Ella use?
Options:
a. 372 pounds
b. 3.72 pounds
c. 0.372 pounds
d. 0.0372 pound
Answer:
b. 3.72 pounds
Explanation:
Ella used 37.2 pounds of apples to make applesauce. She used one-tenth as many pounds of sugar as pounds of apples.
37.2 ÷ 10 = 3.72 pounds
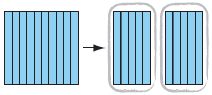
Share and Show – Page No. 207
Use the model to complete the number sentence.
Question 1.
1.6 ÷ 4 =
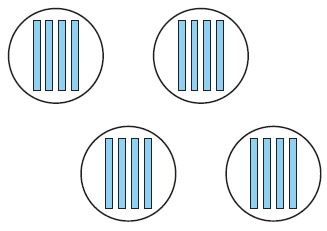
_____
Answer:
1.6 ÷ 4 = 0.4
Explanation:
1.6 ÷ 4
Share your model among 4 equal groups.
Since 1 whole cannot be shared among 4 groups without regrouping, cut your model apart to show the tenths.
1 ones = 10 tenths
10 + 6 = 16 tenths
There are 16 tenths in 1.6.
Share the tenths equally among the 4 groups.
There are 0 ones and 16 tenths in each group.
Decimal for the amount in each group = 0.4
1.6 ÷ 4 = 0.4
Question 2.
3.42 ÷ 3 =
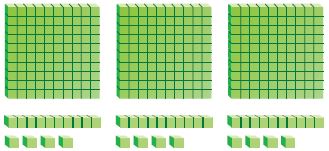
_____
Answer:
3.42 ÷ 3 = 1.14
Explanation:
3.42 ÷ 3
Share your model among 3 equal groups.
1 whole in each group. So, 3 wholes shared equally in 3 groups. 1 ones
3 ÷ 3 = 1 ones
3 tenths shared equally in 3 groups. 1 tenth has remained. 1 tenth
3 ÷ 3 = 1 tenths
1 tenth = 10 hundredths.
10 + 2 = 12 hundredths.
Share 12 hundredths equally among the 3 groups.
12 hundredths ÷ 3 = 4 hundredths.
Decimal for the amount in each group = 1.14
3.42 ÷ 3 = 1.14
Divide. Use base-ten blocks.
Question 3.
1.8 ÷ 3 = _____
Answer:
1.8 ÷ 3 = 0.6
Explanation:
1.8 ÷ 3
Share your model among 3 equal groups.
Since 1 whole cannot be shared among 3 groups without regrouping, cut your model apart to show the tenths. 0 ones
1 ones = 10 tenths
10 + 8 = 18 tenths
There are 18 tenths in 1.8.
Share the 18 tenths equally among the 3 groups.
18 ÷ 3 = 6
There are 0 ones and 18 tenths in each group.
Decimal for the amount in each group = 0.6
1.8 ÷ 3 = 0.6
Question 4.
3.6 ÷ 4 = _____
Answer:
3.6 ÷ 4 = 0.9
Explanation:
3.6 ÷ 4
Share your model among 4 equal groups.
Since 3 whole cannot be shared among 4 groups without regrouping, cut your model apart to show the tenths. 0 ones
1 ones = 10 tenths
30 + 6 = 36 tenths
There are 36 tenths in 3.6.
Share the 36 tenths equally among the 4 groups.
There are 0 ones and 36 tenths in each group.
36 ÷ 4 = 9
Decimal for the amount in each group = 0.9
3.6 ÷ 4 = 0.9
Question 5.
2.5 ÷ 5 = _____
Answer:
2.5 ÷ 5 = 0.5
Explanation:
2.5 ÷ 5
Share your model among 5 equal groups.
Since 2 whole cannot be shared among 5 groups without regrouping, cut your model apart to show the tenths. 0 ones
1 ones = 10 tenths
20 + 5 = 25 tenths
There are 25 tenths in 2.5.
Share the 25 tenths equally among the 5 groups.
There are 0 ones and 25 tenths in each group.
25 ÷ 5 = 5
Decimal for the amount in each group = 0.5
2.5 ÷ 5 = 0.5

Question 7.
3.78 ÷ 3 = _____
Answer:
3.78 ÷ 3 = 1.26
Explanation:
3.78 ÷ 3
Share your model among 3 equal groups.
1 whole in each group. So, 3 wholes shared equally in 3 groups.
3 ÷ 3 = 1 ones
6 tenths shared equally in 3 groups. 1 tenth has remained.
6 ÷ 3 = 2 tenths
1 tenth = 10 hundredths.
10 + 8 = 18 hundredths.
Share 18 hundredths equally among the 3 groups.
18 hundredths ÷ 3 = 6 hundredths.
Decimal for the amount in each group = 1.26
3.78 ÷ 3 = 1.26
Question 8.
1.33 ÷ 7 = _____
Answer:
1.33 ÷ 7 = 0.19
Explanation:
1.33 ÷ 7
Share your model among 7 equal groups.
Since 1 whole cannot be shared among 7 groups without regrouping, cut your model apart to show the tenths. 0 ones
1 ones = 10 tenths
10 + 3 = 13 tenths
There are 13 tenths in 1.3.
Share the 13 tenths equally among the 7 groups.
Share 7 tenths equally among the 3 groups. 6 tenths remained.
7 ÷ 7 = 1 tenths
Since 6 tenths cannot be shared among 7 groups without regrouping, cut your model apart to show the tenths.
1 tenths = 10 hundredths
60 + 3 = 63 hundredths
Share 63 hundredths equally among the 7 groups.
63 ÷ 7 = 9 hundredths
Decimal for the amount in each group = 0.19
1.33 ÷ 7 = 0.19
Question 9.
4.72 ÷ 4 = _____
Answer:
4.72 ÷ 4 = 1.18
Explanation:
4.72 ÷ 4
Share your model among 4 equal groups.
1 whole in each group. So, 4 wholes shared equally in 4 groups. 1 ones
4 ÷ 4 = 1 ones
4 tenths shared equally in 4 groups. 3 tenths have remained.
4 ÷ 4 = 1 tenths
1 tenth = 10 hundredths.
30 + 2 = 32 hundredths.
Share 32 hundredths equally among the 4 groups.
32 hundredths ÷ 4 = 8 hundredths.
Decimal for the amount in each group = 1.18
4.72 ÷ 4 = 1.18
Question 10.
2.52 ÷ 9 = _____
Answer:
2.52 ÷ 9 = 0.28
Explanation:
2.52 ÷ 9
Share your model among 9 equal groups.
Since 2 whole cannot be shared among 9 groups without regrouping, cut your model apart to show the tenths.
9 ÷ 9 = 1 ones
1 ones = 10 tenths
20 + 5 = 25 tenths
There are 25 tenths in 2.5.
Share the 18 tenths equally among the 9 groups. 7 tenths remained.
18 ÷ 9 = 2 tenths
1 tenth = 10 hundredths.
70 + 2 hundredths = 72 hundredths
Share the 72 hundredths equally among the 9 groups.
72 ÷ 9 = 8
Decimal for the amount in each group = 0.28
2.52 ÷ 9 = 0.28
Question 11.
6.25 ÷ 5 = _____
Answer:
6.25 ÷ 5 = 1.25
Explanation:
6.25 ÷ 5
Share your model among 5 equal groups.
1 whole in each group. So, 5 wholes shared equally in 5 groups. 1 whole remained.
5 ÷ 5 = 1 ones
1 ones = 10 tenths
10 + 2 = 12 tenths
10 tenths shared equally in 5 groups. 2 tenths have remained.
10 ÷ 5 = 2 tenths
1 tenth = 10 hundredths.
20 + 5 = 25 hundredths.
Share 25 hundredths equally among the 5 groups.
25 hundredths ÷ 5 = 5 hundredths.
Decimal for the amount in each group = 1.25
6.25 ÷ 5 = 1.25
Problem Solving – Page No. 208
What’s the Error?
Question 12.
Aida is making banners from a roll of paper that is 4.05 meters long. She will cut the paper into 3 equal lengths. How long will each banner be?

Look how Aida solved the problem. Solve the problem and correct
Find the error. the error.
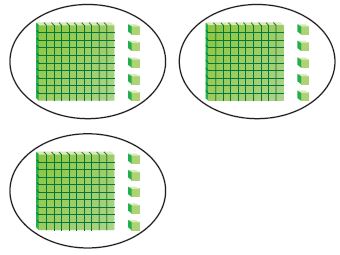
So, Aida said that each banner would be _________ meters long, but each banner should be _________ meters long.
Type below:
_________
Answer:
So, Aida said that each banner would be 4.05 meters long, but each banner should be 1.35 meters long.
So, 1 ones, 3 tenths, and 5 hundredths are shared among 3 groups.
But Aida draws only one whole and 5 hundredths among 3 groups.
Explanation:
Aida is making banners from a roll of paper that is 4.05 meters long. She will cut the paper into 3 equal lengths.
4.05 ÷ 3
Share your model among 3 equal groups.
1 whole in each group. So, 3 wholes shared equally in 5 groups. 1 whole remained.
3 ÷ 3 = 1 ones
1 ones = 10 tenths
10 + 0 = 10 tenths
9 tenths shared equally in 3 groups. 1 tenth remained.
9 ÷ 3 = 3 tenths
1 tenth = 10 hundredths.
10 + 5 = 15 hundredths.
Share 6 hundredths equally among the 3 groups.
15 hundredths ÷ 3 = 5 hundredths.
Decimal for the amount in each group = 1.35
4.05 ÷ 3 = 1.35
Question 12.
• Describe Aida’s error.
Type below:
_________
Answer:
So, Aida said that each banner would be 4.05 meters long, but each banner should be 1.35 meters long.
So, 1 ones, 3 tenths, and 5 hundredths are shared among 3 groups.
But Aida draws only one whole and 5 hundredths among 3 groups.
Question 12.
• What if the roll of paper were 4.35 meters long? How long would each banner be?
Type below:
_________
Answer:
1.45 meters long
Explanation:
If the roll paper were 4.35 meters long,
4.35 ÷ 3
Share your model among 3 equal groups.
1 whole in each group. So, 3 wholes shared equally in 5 groups. 1 whole remained.
3 ÷ 3 = 1 ones
1 ones = 10 tenths
10 + 3 = 13 tenths
12 tenths shared equally in 3 groups. 1 tenth remained.
12 ÷ 3 = 4 tenths
1 tenth = 10 hundredths.
10 + 5 = 15 hundredths.
Share 6 hundredths equally among the 3 groups.
15 hundredths ÷ 3 = 5 hundredths.
Decimal for the amount in each group = 1.45
4.35 ÷ 3 = 1.45
Share and Show – Page No. 211
Use compatible numbers to estimate the quotient.


Estimate the quotient.

Question 4.
17.9 ÷ 9
_____
Answer:
About 2
Explanation:
17.9 is closer to 18
18 ÷ 9 = 2
Question 5.
145.4 ÷ 21
_____
Answer:
about 7
Explanation:
Use a whole number greater than the dividend.
145.4 is closer to 160
21 is closer to 20
160 ÷ 20 = 8
Use a whole number less than the dividend.
145.4 is closer to 140
21 is closer to 20
140 ÷ 20 = 7
The quotient should be between 7 and 8.
145.4 is closer to 140.
So, the answer is about 7
On Your Own
Estimate the quotient.
Question 6.
15.5 ÷ 4
_____
Answer:
About 4
Explanation:
15.5 is closer to 16
16 ÷ 4 = 4
Question 7.
394.8 ÷ 7
_____
Answer:
About 60
Explanation:
394.8 is closer to 420
420 ÷ 7 = 60
About 60
Question 8.
410.5 ÷ 18
_____
Answer:
About 22
Explanation:
410.5 is closer to 396
396 ÷ 18 = 22
Question 9.
72.1 ÷ 7
_____
Answer:
About 10
Explanation:
72.1 is closer to 70
70 ÷ 7 = 10
Question 10.
32.4 ÷ 52
_____
Answer:
About 1
Explanation:
32.4 is closer to 52
52 ÷ 52 = 1
Question 11.
$134.42 ÷ 28
$ _____
Answer:
about 4
Explanation:
Use a whole number greater than the dividend.
$134.42 is closer to 150
28 is closer to 30
150 ÷ 30 = 5
Use a whole number less than the dividend.
$134.42 is closer to 120
28 is closer to 30
120 ÷ 30 = 4
The quotient should be between 4 and 5.
$134.42 is closer to $120.
So, the answer is about 4
Question 12.
21.8 ÷ 4
_____
Answer:
About 5
Explanation:
21.8 is closer to 20
20 ÷ 4 = 5
Question 13.
3.4 ÷ 5
_____
Answer:
About 1
Explanation:
3.4 is closer to 5
5 ÷ 5 = 1
About 1
Question 14.
$759.92 ÷ 42
$ _____
Answer:
About $18
Explanation:
$759.92 is closer to $756
$756 ÷ 42 = 18
About 18
Question 15.
157.5 ÷ 38
_____
Answer:
About 4
Explanation:
157.5 is closer to 152
152 ÷ 38 = 4
Question 16.
379.2 ÷ 6
_____
Answer:
About 63
Explanation:
379.2 is closer to 378
378 ÷ 6 = 63
Question 17.
108.4 ÷ 21
_____
Answer:
About 5
Explanation:
108.4 is closer to 100
21 is closer to 20
100 ÷ 20 = 5
Problem Solving – Page No. 212
Use the table to solve 18–20.

Question 18.
Estimate the average daily snowfall for Alaska’s greatest 7-day snowfall.
_____ inches
Answer:
26 inches
Explanation:
Alaska = 186.9 inches snowfall for 7 days.
For 1 day, 186.9 ÷ 7
186.9 is closer to 182
1825 ÷ 7 = 26 inches
Question 19.
How does the estimate of the average daily snowfall for Wyoming’s greatest 7-day snowfall compare to the estimate of the average daily snowfall for South Dakota’s greatest 7-day snowfall?
Type below:
_________
Answer:
South Dakota’s snowfall is greater than Wyoming’s snowfall.
Explanation:
Wyoming’s = 84.5 inches snowfall for 7 days.
For 1 day, 84.5 ÷ 7
84.5 is closer to 84
84 ÷ 7 = 12 inches
South Dakota’s = 112.7 inches snowfall for 7 days.
For 1 day, 112.7 ÷ 7
112.7 is closer to 112
112 ÷ 7 = 16 inches
Question 20.
The greatest monthly snowfall total in Alaska is 297.9 inches. This happened in February, 1953. Compare the daily average snowfall for February, 1953, with the average daily snowfall for Alaska’s greatest 7-day snowfall. Use estimation.
Type below:
_________
Answer:
The average snowfall of Alaska in February, 1953 is greater than snowfall for Alaska’s greatest 7-day snowfall.
Explanation:
Alaska = 186.9 inches snowfall for 7 days.
For 1 day, 186.9 ÷ 7
186.9 is closer to 182
1825 ÷ 7 = 26 inches
Alaska = 297.9 inches snowfall for 7 days.
For 1 day, 297.9 ÷ 7
297.9 is closer to 294
294 ÷ 7 = 42 inches

Question 22.
Test Prep A plant grew 23.8 inches over 8 weeks. Which is the best estimate of the average number of inches the plant grew each week?
Options:
a. 0.2 inch
b. 0.3 inch
c. 2 inch
d. 3 inch
Answer:
d. 3 inch
Explanation:
A plant grew 23.8 inches over 8 weeks.
23.8 is closer to 24
24 ÷ 8 = 3 inch
Share and Show – Page No. 215
Write the quotient with the decimal point placed correctly.
Question 1.
4.92 ÷ 2 = 246
_____
Answer:
2.46
Explanation:
Divide. 4 ones ÷ 2
Multiply. 2 × 2 ones = 4 ones. Subtract. 4 ones − 4 ones = 0
Check. 4 ones shared among 2 groups as 2 wholes.
Divide. 9 tenths ÷ 2
Multiply. 2 × 4 tenths = 8 tenths. Subtract. 9 tenths − 8 tenths = 1 tenths
Check. 8 tenths shared among 2 groups as 4 tenths. 1 tenth remained.
1 tenth = 10 hundredths.
10 + 2 = 12 hundredths
Divide. 12 hundredths ÷ 2
Multiply. 2 × 6 hundredths = 12 hundredths. Subtract. 12 hundredths − 12 hundredths = 0 hundredths
Check. 12 hundredths shared among 2 groups as 6 hundredths.
So, 2.46

Divide.
Question 3.
5)\(\overline { 8.65 }\)
_____
Answer:
1.73
Explanation:
8.65 ÷ 5
Divide. 8 ones ÷ 5
Multiply. 5 × 1 ones = 5ones. Subtract. 8 ones − 5 ones = 3 ones
Check. 5 ones shared among 5 groups as 1 whole.
3 one(s) cannot be shared among 5 groups without regrouping.
30 + 6 = 36 tenths
Divide. 36 tenths ÷ 5
Multiply. 5 x 7 tenths = 35 tenths. Subtract. 36 tenths − 35 tenths = 7 tenths
Check. 35 tenths shared among 5 groups as 7 tenths.
1 tenths(s) cannot be shared among 5 groups without regrouping.
1 tenth = 10 hundredths.
10 + 5 = 15 hundredths
Divide. 15 hundredths ÷ 5
Multiply. 5 x 3 hundredths = 15 hundredths. Subtract. 15 hundredths − 15 hundredths = 0 hundredths
Check. 15 hundredths shared among 5 groups as 3 hundredths.
So, 1.73
Question 4.
3)\(\overline { 2.52 }\)
_____
Answer:
0.84
Explanation:
2.52 ÷ 3
2 one(s) cannot be shared among 3 groups without regrouping.
20 + 5 = 25 tenths
Divide. 25 tenths ÷ 3
Multiply. 3 x 8 tenths = 24 tenths. Subtract. 25 tenths − 24 tenths = 1 tenths
Check. 24 tenths shared among 3 groups as 8 tenths.
1 tenths(s) cannot be shared among 3 groups without regrouping.
1 tenth = 10 hundredths.
10 + 2 = 12 hundredths
Divide. 12 hundredths ÷ 3
Multiply. 3 x 4 hundredths = 12 hundredths. Subtract. 12 hundredths − 12 hundredths = 0 hundredths
Check. 12 hundredths shared among 3 groups as 4 hundredths.
So, 0.84
Question 5.
27)\(\overline { 97.2 }\)
_____
Answer:
3.6
Explanation:
97.2 ÷ 27
Divide. 97 ones ÷ 27
Multiply. 27 x 3 ones = 81 ones. Subtract. 97 ones − 81 ones = 16 ones
Check. 81 ones shared among 27 groups as 3 whole.
16 one(s) cannot be shared among 27 groups without regrouping.
160 + 2 = 162 tenths
Divide. 162 tenths ÷ 27
Multiply. 27 x 6 tenths = 162 tenths. Subtract. 162 tenths − 162 tenths = 0 tenths
Check. 162 tenths shared among 27 groups as 6 tenths.
So, 3.6
On Your Own
Divide.
Question 6.
6)\(\overline { 8.94 }\)
_____
Answer:
1.49
Explanation:
8.94 ÷ 6
Divide. 8 ones ÷ 6
Multiply. 6 × 1 ones = 6 ones. Subtract. 8 ones − 6 ones = 2 ones
Check. 6 ones shared among 6 groups as 1 whole.
2 one(s) cannot be shared among 6 groups without regrouping.
20 + 9 = 29 tenths
Divide. 29 tenths ÷ 6
Multiply. 6 x 4 tenths = 24 tenths. Subtract. 29 tenths − 24 tenths = 5 tenths
Check. 24 tenths shared among 6 groups as 4 tenths.
5 tenths(s) cannot be shared among 6 groups without regrouping.
1 tenth = 10 hundredths.
50 + 4 = 54 hundredths
Divide. 54 hundredths ÷ 6
Multiply. 6 x 9 hundredths = 54 hundredths. Subtract. 54 hundredths − 54 hundredths = 0 hundredths
Check. 54 hundredths shared among 6 groups as 54 hundredths.
So, 1.49
Question 7.
5)\(\overline { 3.75 }\)
_____
Answer:
0.75
Explanation:
3.75 ÷ 5
3 one(s) cannot be shared among 5 groups without regrouping.
30 + 7 = 37 tenths
Divide. 37 tenths ÷ 5
Multiply. 5 x 7 tenths = 35 tenths. Subtract. 37 tenths − 35 tenths = 2 tenths
Check. 35 tenths shared among 5 groups as 7 tenths.
2 tenths(s) cannot be shared among 5 groups without regrouping.
1 tenth = 10 hundredths.
20 + 5 = 25 hundredths
Divide. 25 hundredths ÷ 5
Multiply. 5 x 5 hundredths = 25 hundredths. Subtract. 25 hundredths − 25 hundredths = 0 hundredths
Check. 25 hundredths shared among 5 groups as 25 hundredths.
So, 0.75
Question 8.
19)\(\overline { 55.1 }\)
_____
Answer:
2.9
Explanation:
55.1 ÷ 19
Divide. 55 ones ÷ 19
Multiply. 19 × 2 ones = 38 ones. Subtract. 55 ones − 38 ones = 17 ones
Check. 38 ones shared among 19 groups as 2 whole.
17 one(s) cannot be shared among 19 groups without regrouping.
170 + 1 = 171 tenths
Divide. 171 tenths ÷ 19
Multiply. 19 x 9 tenths = 171 tenths. Subtract. 171 tenths − 171 tenths = 0 tenths
Check. 171 tenths shared among 19 groups as 9 tenths.
So, 2.9
Question 9.
23)\(\overline { 52.9 }\)
_____
Answer:
2.3
Explanation:
52.9 ÷ 23
Divide. 52 ones ÷ 23
Multiply. 23 × 2 ones = 46 ones. Subtract. 52 ones − 46 ones = 6 ones
Check. 46 ones shared among 23 groups as 2 whole.
6 one(s) cannot be shared among 23 groups without regrouping.
60 + 9 = 69 tenths
Divide. 69 tenths ÷ 23
Multiply. 23 x 3 tenths = 69 tenths. Subtract. 69 tenths − 69 tenths = 0 tenths
Check. 69 tenths shared among 23 groups as 3 tenths.
So, 2.3
Question 10.
8)\(\overline { $8.24 }\)
$ _____
Answer:
Explanation:
8.24 ÷ 8
Divide. 8 ones ÷ 8
Multiply. 8 × 1 ones = 8 ones. Subtract. 8 ones − 8 ones = 0 ones
Check. 8 ones shared among 8 groups as 1 whole.
2 tenths(s) cannot be shared among 8 groups without regrouping.
1 tenth = 10 hundredths.
20 + 4 = 24 hundredths
Divide. 24 hundredths ÷ 8
Multiply. 8 x 3 hundredths = 24 hundredths. Subtract. 24 hundredths − 24 hundredths = 0 hundredths
Check. 24 hundredths shared among 8 groups as 3 hundredths.
So, 1.03


Practice: Copy and Solve Divide.
Question 12.
3)\(\overline { $7.71 }\)
$_____
Answer:
2.57
Explanation:
7.71 ÷ 3
Divide. 7 ones ÷ 3
Multiply. 3 x 2 ones = 6 ones. Subtract. 7 ones − 6 ones = 1 ones
Check. 6 ones shared among 3 groups as 2 wholes.
1 one(s) cannot be shared among 3 groups without regrouping.
10 + 7 = 17 tenths
Divide. 17 tenths ÷ 3
Multiply. 3 x 5 tenths = 15 tenths. Subtract. 17 tenths − 15 tenths = 2 tenths
Check. 15 tenths shared among 3 groups as 5 tenths.
2 tenths(s) cannot be shared among 3 groups without regrouping.
1 tenth = 10 hundredths.
20 + 1 = 21 hundredths
Divide. 21 hundredths ÷ 3
Multiply. 3 x 7 hundredths = 21 hundredths. Subtract. 21 hundredths − 21 hundredths = 0 hundredths
Check. 21 hundredths shared among 3 groups as 7 hundredths.
So, 2.57
Question 13.
14)\(\overline { 79.8 }\)
_____
Answer:
5.7
Explanation:
79.8 ÷ 14
Divide. 79 ones ÷ 14
Multiply. 14 x 5 ones = 70 ones. Subtract. 79 ones − 70 ones = 9 ones
Check. 70 ones shared among 14 groups as 5 wholes.
9 one(s) cannot be shared among 14 groups without regrouping.
90 + 8 = 98 tenths
Divide. 98 tenths ÷ 14
Multiply. 14 x 7 tenths = 98 tenths. Subtract. 98 tenths − 98 tenths = 0 tenths
Check. 98 tenths shared among 14 groups as 7 tenths.
So, 5.7
Question 14.
33)\(\overline { 25.41 }\)
_____
Answer:
0.77
Explanation:
25.41 ÷ 33
Divide. 25 ones ÷ 33
25 one(s) cannot be shared among 33 groups without regrouping.
250 + 4 = 254 tenths
Divide. 254 tenths ÷ 33
Multiply. 33 x 7 tenths = 231 tenths. Subtract. 254 tenths − 231 tenths = 23 tenths
Check. 231 tenths shared among 33 groups as 7 tenths.
23 tenths(s) cannot be shared among 33 groups without regrouping.
1 tenth = 10 hundredths.
230 + 1 = 231 hundredths
Divide. 231 hundredths ÷ 33
Multiply. 33 x 7 hundredths= 231 hundredths. Subtract. 231 hundredths− 231 hundredths = 0 hundredths
Check. 231 tenths shared among 33 groups as 7 tenths.
So, 0.77
Question 15.
7)\(\overline { 15.61 }\)
_____
Answer:
2.23
Explanation:
15.61 ÷ 7
Divide. 15 ones ÷ 7
Multiply. 7 x 2 ones = 14 ones. Subtract. 15 ones − 14 ones = 1 ones
Check. 14 ones shared among 7 groups as 2 wholes.
1 one(s) cannot be shared among 7 groups without regrouping.
10 + 6 = 16 tenths
Divide. 16 tenths ÷ 7
Multiply. 7 x 2 tenths = 14 tenths. Subtract. 16 tenths − 14 tenths = 2 tenths
Check. 14 tenths shared among 7 groups as 2 tenths.
2 tenths(s) cannot be shared among 7 groups without regrouping.
1 tenth = 10 hundredths.
20 + 1 = 21 hundredths
Divide. 21 hundredths ÷ 7
Multiply. 7 x 3 hundredths = 21 hundredths. Subtract. 21 hundredths − 21 hundredths = 0 hundredths
Check. 21 hundredths shared among 7 groups as 3 hundredths.
So, 2.23
Question 16.
14)\(\overline { 137.2 }\)
_____
Answer:
9.8
Explanation:
137.2 ÷ 14
Divide. 137 ones ÷ 14
Multiply. 14 x 9 ones = 126 ones. Subtract. 137 ones − 126 ones = 11 ones
Check. 126 ones shared among 14 groups as 9 wholes.
11 one(s) cannot be shared among 14 groups without regrouping.
110 + 2 = 112 tenths
Divide. 112 tenths ÷ 14
Multiply. 14 x 8 tenths = 112 tenths. Subtract. 112 tenths − 112 tenths = 0 tenths
Check. 112 tenths shared among 14 groups as 8 tenths.
So, 9.8
Question 17.
34)\(\overline { 523.6 }\)
_____
Answer:
15.4
Explanation:
523.6 ÷ 34
Divide. 523 ones ÷ 34
Multiply. 34 x 15 ones = 510 ones. Subtract. 523 ones − 510 ones = 13 ones
Check. 510 ones shared among 34 groups as 15 wholes.
13 one(s) cannot be shared among 34 groups without regrouping.
130 + 6 = 136 tenths
Divide. 136 tenths ÷ 34
Multiply. 34 x 4 tenths = 136 tenths. Subtract. 136 tenths − 136 tenths = 0 tenths
Check. 136 tenths shared among 34 groups as 4 tenths.
So, 15.4
Algebra Write the unknown number for each ■.
Question 18.
■ ÷ 5 = 1.21
■ = _____
Answer:
6.05
Explanation:
■ ÷ 5 = 1.21
■ = 1.21 x 5
■ = 6.05
Question 19.
46.8 ÷ 1.2 = ■
■ = _____
Answer:
39
Explanation:
46.8 ÷ 1.2 = ■
39 = ■
Question 20.
34.1 ÷ ■ = 22
■ = _____
Answer:
1.55
Explanation:
34.1 ÷ ■ = 22
■ = 34.1 ÷ 22
■ = 1.55
UNLOCK the Problem – Page No. 216
Question 21.
The standard width of 8 lanes in swimming pools used for competitions is 21.92 meters. The standard width of 9 lanes is 21.96 meters. How much wider is each lane when there are 8 lanes than when there are 9 lanes?

a. What are you asked to find?
Type below:
_________
Answer:
We have to find how much wider is each line When there are 8 lanes than when there are 9 lanes.
Question 21.
b. What operations will you use to solve the problem?
Type below:
_________
Answer:
Division
Subtraction
Question 21.
c. Show the steps you used to solve the problem.
Type below:
_________
Answer:
21.92 ÷ 8 = 2.74 meters
21.96 ÷ 9 = 2.44 meters
2.74 – 2.44 = 0.30 meters
Question 21.
d. Complete the sentences.
Each lane is ______ meters wide when there are 8 lanes.
Each lane is ______ meters wide when there are 9 lanes.
Since ______ – ______ = ______ , the lanes are ______ meter(s) wider when there are 8 lanes than when there are 9 lanes.
Type below:
_________
Answer:
Each lane is 2.74 meters wide when there are 8 lanes.
Each lane is 2.44 meters wide when there are 9 lanes.
Since 2.74 – 2.44 = 0.30, the lanes are 0.30 meter(s) wider when there are 8 lanes than when there are 9 lanes.
Question 21.
e. Fill in the bubble for the correct answer choice.
Options:
a. 0.30 meter
b. 2.44 meters
c. 2.74 meters
d. 22.28 meters
Answer:
a. 0.30 meter
Question 22.
Robert pays $32.04 for 6 student tickets to the basketball game. What is the cost of each student ticket?
Options:
a. $192.24
b. $53.40
c. $26.04
d. $5.34
Answer:
d. $5.34
Explanation:
Robert pays $32.04 for 6 student tickets to the basketball game.
$32.04 ÷ 6 = $5.34
Question 23.
Jasmine uses 14.24 pounds of fruit for 16 servings of fruit salad. If each serving contains the same amount of fruit, how much fruit is in each serving?
Options:
a. 0.089 pound
b. 0.89 pound
c. 1.76 pounds
d. 17.6 pounds
Answer:
b. 0.89 pound
Explanation:
Jasmine uses 14.24 pounds of fruit for 16 servings of fruit salad. If each serving contains the same amount of fruit, 14.24 ÷ 16 = 0.89 pounds
Mid-Chapter Checkpoint – Page No. 217
Concepts and Skills
Question 1.
Explain how the position of the decimal point changes in a quotient as you divide by increasing powers of 10.
Type below:
_________
Answer:
Dividing a number by ten shifts the decimal point of the dividend one place to the left, making that into a smaller number, the desired quotient, which is one-tenth of the dividend.
Question 2.
Explain how you can use base-ten blocks to find 2.16 ÷ 3.
Type below:
_________
Answer:
0.72
Explanation:
2.16 ÷ 3
There are 2 ones, 1 tenths, and 6 hundredths.
2.16 ÷ 3 =
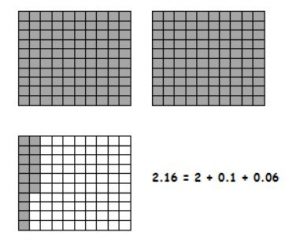
2 ones cannont be shared for 3 groups.
So, write 2 ones as 20 tenths.
20 + 1 = 21 tenths.
21 tenths ÷ 3 = 7 tenths.
7 tenths shared amount 3 groups = 21 tenths
6 hundredths ÷ 3 = 2 hundredths.
2 hundredths shared amount 3 groups = 6 hundredths.
0.72
Complete the pattern.


Question 5.
57.4 ÷ 100 = _______
57.4 ÷ 101 = _______
57.4 ÷ 102 = _______
Answer:
57.4 ÷ 100 = 57.4
57.4 ÷ 101 = 5.74
57.4 ÷ 102 = 0.574
Explanation:
57.4 ÷ 100 = 57.4 ÷ 1 = 57.4
57.4 ÷ 101 = 57.4 ÷ 10 = 5.74
57.4 ÷ 102 = 57.4 ÷ 100 = 0.574
Estimate the quotient.


Question 8.
492.6 ÷ 48 = ______
Answer:
About 10
Explanation:
492.6 is closer to 480
480 ÷ 48 = 10
Divide.
Question 9.
5)\(\overline { 4.35 }\)
_____
Answer:
0.87
Explanation:
4.35 ÷ 5
Divide. 8 ones ÷ 5
4 one(s) cannot be shared among 5 groups without regrouping.
40 + 3 = 43 tenths
Divide. 43 tenths ÷ 5
Multiply. 5 x 8 tenths = 40 tenths. Subtract. 43 tenths − 40 tenths = 3 tenths
Check. 40 tenths shared among 5 groups as 8 tenths.
3 tenths(s) cannot be shared among 5 groups without regrouping.
1 tenth = 10 hundredths.
30 + 5 = 35 hundredths
Divide. 35 hundredths ÷ 5
Multiply. 5 x 7 hundredths = 35 hundredths. Subtract. 35 hundredths − 35 hundredths = 0 hundredths
Check. 35 hundredths shared among 5 groups as 7 hundredths.
So, 0.87
Question 10.
8)\(\overline { 9.92 }\)
_____
Answer:
1.24
Explanation:
9.92 ÷ 8
Divide. 9 ones ÷ 8
Multiply. 8 × 1 ones = 8 ones. Subtract. 9 ones − 8 ones = 1 ones
Check. 8 ones shared among 8 groups as 1 whole.
1 one(s) cannot be shared among 8 groups without regrouping.
10 + 9 = 19 tenths
Divide. 19 tenths ÷ 8
Multiply. 8 x 2 tenths = 16 tenths. Subtract. 19 tenths − 16 tenths = 3 tenths
Check. 16 tenths shared among 8 groups as 2 tenths.
3 tenths(s) cannot be shared among 8 groups without regrouping.
1 tenth = 10 hundredths.
30 + 2 = 32 hundredths
Divide. 32 hundredths ÷ 8
Multiply. 8 x 4 hundredths = 32 hundredths. Subtract. 32 hundredths − 32 hundredths = 0 hundredths
Check. 32 hundredths shared among 8 groups as 4 hundredths.
So, 1.24
Question 11.
61)\(\overline { 207.4 }\)
_____
Answer:
3.4
Explanation:
207.4 ÷ 61
Divide. 207 ones ÷ 61
Multiply. 61 x 3 ones = 183 ones. Subtract. 207 ones − 183 ones = 24 ones
Check. 183 ones shared among 61 groups as 3 wholes.
24 one(s) cannot be shared among 61 groups without regrouping.
240 + 4 = 244 tenths
Divide. 244 tenths ÷ 61
Multiply. 61 x 4 tenths = 244 tenths. Subtract. 244 tenths − 244 tenths = 0 tenths
Check. 244 tenths shared among 61 groups as 4 tenths.
So, 3.4
Mid-Chapter Checkpoint – Page No. 218
Question 12.
The Westside Bakery uses 440 pounds of flour to make 1,000 loaves of bread. Each loaf contains the same amount of flour. How many pounds of flour are used in each loaf of bread?
_____ pounds
Answer:
0.440 pounds
Explanation:
The Westside Bakery uses 440 pounds of flour to make 1,000 loaves of bread. Each loaf contains the same amount of flour.
440/1000 = 0.440 pounds
Question 13.
Elise pays $21.75 for 5 student tickets to the fair. What is the cost of each student ticket?
$ _____
Answer:
$4.35
Explanation:
Elise pays $21.75 for 5 student tickets to the fair.
$21.75/5 = $4.35
Question 14.
Jason has a piece of wire that is 62.4 inches long. He cuts the wire into 3 equal pieces. Estimate the length of 1 piece of wire.
_____ in
Answer:
20.8 inches
Explanation:
Jason has a piece of wire that is 62.4 inches long. He cuts the wire into 3 equal pieces.
62.4/3 = 20.8 inches
Question 15.
Elizabeth uses 23.25 ounces of granola and 10.5 ounces of raisins for 15 servings of trail mix. If each serving contains the same amount of trail mix, how much trail mix is in each serving?
_____ ounces
Answer:
2.25 ounces
Explanation:
Elizabeth uses 23.25 ounces of granola and 10.5 ounces of raisins for 15 servings of trail mix.
23.25 + 10.5 = 33.75
33.75/15 = 2.25 ounces
Share and Show – Page No. 221
Use the model to complete the number sentence.
Question 1.
1.2 ÷ 0.3 =
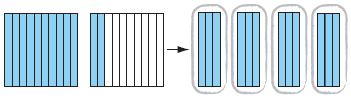
_____
Answer:
1.2 ÷ 0.3 = 4
Explanation:
There are 4 groups of 3 tenths.
So, 1.2 ÷ 0.3 = 4
Question 2.
0.45 ÷ 0.09 =
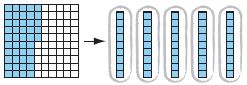
_____
Answer:
0.45 ÷ 0.09 = 5
Explanation:
There are 5 groups. Each group has 9 hundredths.
0.09 x 5 = 0.45
So, 0.45 ÷ 0.09 = 5
Question 3.
0.96 ÷ 0.24 =
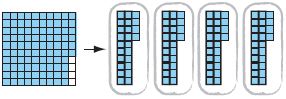
_____
Answer:
0.96 ÷ 0.24 = 4
Explanation:
There are 4 groups. Each group has 2 tenths and 4 hundredths. 24 hundredths
0.24 x 4 = 0.96
0.96 ÷ 0.24 = 4
Question 4.
1 ÷ 0.5 =
_____
Answer:
2
Explanation:
There are 2 groups. Each group has 5 tenths.
0.5 x 2 = 1.0 = 1
1 ÷ 0.5 = 2
Divide. Use decimal models.
Question 5.
1.8 ÷ 0.6 =
_____
Answer:
3
Explanation:
1.8
There are 1 one(s) and 8 tenth(s).
Cut 1.8 to show groups of 0.6.
There are 3 groups of 1.8 tenths.
1.8 ÷ 0.6 = 3
Question 6.
1.2 ÷ 0.3 =
_____
Answer:
4
Explanation:
1.2
There are 1 one(s) and 2 tenth(s).
Cut 1.2 to show groups of 0.3.
There are 4 groups of 1.2 tenths.
1.2 ÷ 0.3 = 4


Question 9.
2 ÷ 0.4 =
_____
Answer:
5
Explanation:
2
There are 2 one(s).
Cut 2 to show groups of 0.4.
There are 5 groups of 2 ones.
2 ÷ 0.4 = 5
Question 10.
2.7 ÷ 0.9 =
_____
Answer:
3
Explanation:
2.7
There are 2 one(s) and 7 tenth(s).
Cut 2.7 to show groups of 0.9.
There are 3 groups of 2.7 tenths.
2.7 ÷ 0.9 = 3
Question 11.
1.24 ÷ 0.62 =
_____
Answer:
2
Explanation:
1.24
There are 1 one(s), 2 tenth(s) and 4 hundredth(s).
Cut 1.24 to show groups of 0.62.
There are 2 groups of 1.24 hundredths.
1.24 ÷ 0.62 = 2
Question 12.
0.84 ÷ 0.14 =
_____
Answer:
6
Explanation:
0.84
There are 0 one(s), 8 tenth(s) and 4 hundredth(s).
Cut 0.84 to show groups of 0.14.
There are 6 groups of 0.84 hundredths.
0.84 ÷ 0.14 = 6

Use the model to find the unknown value.
Question 14.
2.4 ÷ □ = 3
_____
Answer:
0.8
Explanation:
2.4 ÷ □ = 3
There are 3 groups with 8 tenths in each group.
So, 2.4 ÷ 0.8 = 3
The unknown number is 0.8
Question 15.
□ ÷ 0.32 = 4

_____
Answer:
1.28
Explanation:
□ ÷ 0.32 = 4
There are 4 groups with 32 hundredths in each group.
So, 0.32 x 4 = 1.28
The unknown number is 1.28
Question 16.
Make a model to find 0.6 ÷ 0.15. Describe your model.
_____
Answer:
0.6 ÷ 0.15
Divide 6 tenths into 15 hundredths each group.
15 hundredths + 15 hundredths + 15 hundredths + 15 hundredths
4 groups.
0.6 ÷ 0.15 = 4
Question 17.
Explain, using the model, what the equation represents in Exercise 15.
Type below:
_________
Answer:
Using the decimal model, we have to find the value of 0.6 ÷ 0.15
0.6 ÷ 0.15 = 4
Problem Solving – Page No. 222
Pose a Problem

Question 18.
Emilio buys 1.2 kilograms of grapes. He separates the grapes into packages that contain 0.3 kilograms of grapes each. How many packages of grapes does Emilio make?
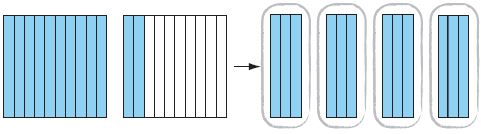
1.2 ÷ 0.3 = 4
Emilio made 4 packages of grapes.
Write a new problem using a different amount for the weight in each package. The amount should be a decimal with tenths. Use a total amount of 1.5 kilograms of grapes. Then use decimal models to solve your problem.
Pose a problem. Solve your problem. Draw a picture of the
model you used to solve your problem.
• Explain why you chose the amount you did for your problem.
Type below:
_________
Answer:
Emilio buys 1.5 kilograms of grapes. He separates the grapes into packages that contain 0.5 kilograms of grapes each.
1.5/0.5 = 3
3 groups
Share and Show – Page No. 225
Copy and complete the pattern.
Question 1.
45 ÷ 9 = _____
4.5 ÷ _____ = 5
_____ ÷ 0.09 = 5
Answer:
45 ÷ 9 = 5
4.5 ÷ 0.9 = 5
0.45 ÷ 0.09 = 5
Explanation:
45 ÷ 9 = 5
4.5 ÷ _____ = 5
45 ÷ 10 = 4.5. So, 9 ÷ 10 = 0.9
4.5 ÷ 0.9 = 5
_____ ÷ 0.09 = 5
0.9 ÷ 10 = 0.09
4.5 ÷ 10 = 0.45
0.45 ÷ 0.09 = 5
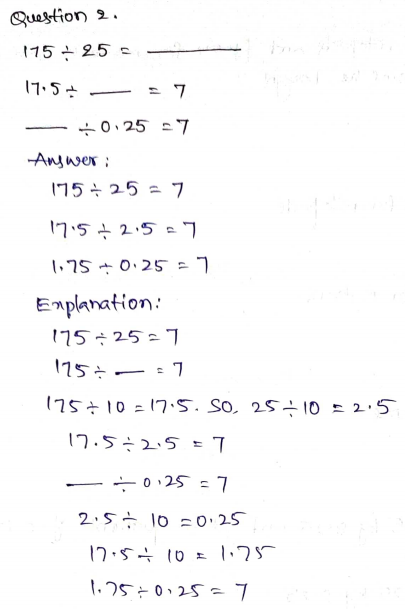
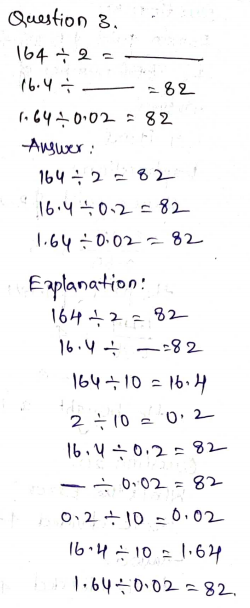
Divide.
Question 4.
1.6)\(\overline { 9.6 }\) = _____
Answer:
6
Explanation:
9.6 ÷ 1.6
9.6 x 10 = 96
1.6 x 10 = 16
96 ÷ 16 = 6
So, 9.6 ÷ 1.6 = 6
Question 5.
0.3)\(\overline { 0.24 }\) = _____
Answer:
0.8
Explanation:
0.24 ÷ 0.3
0.24 x 10 = 2.4
0.3 x 10 = 3
2.4 ÷ 3 = 0.8
0.24 ÷ 0.3 = 0.8
Question 6.
3.45 ÷ 1.5 = _____
Answer:
2.3
Explanation:
3.45 ÷ 1.5
3.45 x 10 = 34.5
1.5 x 10 = 15
34.5 ÷ 15 = 2.3
3.45 ÷ 1.5 = 2.3
On Your Own
Divide.
Question 7.
0.6)\(\overline { 13.2 }\) = _____
Answer:
22
Explanation:
13.2 ÷ 0.6
13.2 x 10 = 132
0.6 x 10 = 6
132 ÷ 6 = 22
13.2 ÷ 0.6 = 22
Question 8.
0.3)\(\overline { 0.9 }\) = _____
Answer:
3
Explanation:
0.9 ÷ 0.3
0.9 x 10 = 9
0.3 x 10 = 3
9 ÷ 3 = 3
0.9 ÷ 0.3 = 3
Question 9.
0.26)\(\overline { 1.56 }\) = _____
Answer:
6
Explanation:
1.56 ÷ 0.26
1.56 x 100 = 156
0.26 x 100 = 26
156 ÷ 26 = 6
1.56 ÷ 0.26 = 6
Question 10.
0.45)\(\overline { 5.85 }\) = _____
Answer:
13
Explanation:
5.85 ÷ 0.45
5.85 x 100 = 585
0.45 x 100 = 45
585 ÷ 45 = 13
Question 11.
0.3)\(\overline { 0.69 }\) = _____
Answer:
2.3
Explanation:
0.69 ÷ 0.3
0.69 x 10 = 6.9
0.3 x 10 = 3
6.9 ÷ 3 = 2.3
Question 12.
3.6 ÷ 0.4 = _____
Answer:
9
Explanation:
3.6 ÷ 0.4
3.6 x 10 = 36
0.4 x 10 = 4
36 ÷ 4 = 9
3.6 ÷ 0.4 = 9

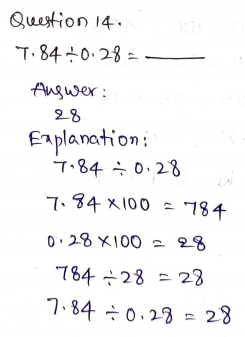
Question 15.
9.28 ÷ 2.9 = _____
Answer:
3.2
Explanation:
9.28 ÷ 2.9
9.28 x 10 = 92.8
2.9 x 10 = 29
92.8 ÷ 29 = 3.2
9.28 ÷ 2.9 = 3.2
Problem Solving – Page No. 226
Use the table to solve 16—19.

Question 16.
Connie paid $1.08 for pencils. How many pencils did she buy?
_____ pencils
Answer:
9 pencils
Explanation:
1 pencil = $0.12
Connie paid $1.08 for pencils.
$1.08 ÷ $0.12 = 9
she buys 9 pencils
Question 17.
Albert has $2.16. How many more pencils can he buy than markers?
_____
Answer:
Albert can buy 12 more pencils than markers
Explanation:
Albert has $2.16.
1 pencil = $0.12
$2.16/$0.12 = 18 pencils
1 marker = $0.36
$2.16/$0.36 = 6 markers
18 – 6 = 12 more pencils
Question 18.
How many erasers can Ayita buy for the same amount that she would pay for one notepad?
_____ erasers
Answer:
Ayita can buy 13 erasers with one notepad money.
Explanation:
1 notepad = $0.65
$0.65/$0.05 = 13
Ayita can buy 13 erasers with one notepad of money.

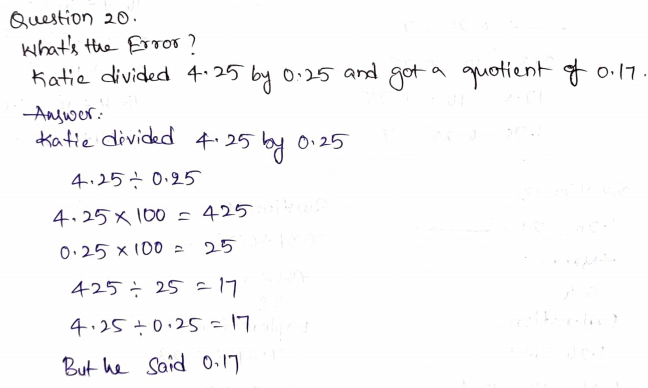
Question 21.
Test Prep Marcus bought apples that cost $0.45 per pound. He paid $1.35 for the apples. How many pounds of apples did he buy?
Options:
a. 0.3 pound
b. 2.8 pounds
c. 3 pounds
d. 30 pounds
Answer:
c. 3 pounds
Explanation:
Marcus bought apples that cost $0.45 per pound.
He paid $1.35 for the apples.
$1.35 ÷ $0.45 = 3 pounds
Share and Show – Page No. 229
Write the quotient with the decimal point placed correctly.
Question 1.
5 ÷ 0.8 = 625
_____
Answer:
5 ÷ 0.8 = 6.25
Explanation:
5 ÷ 0.8 = 5 ÷ 0.800
50 ÷ 8 = 6.25
Divide 5 ÷ 0.8 until you get remainder 0
Divide: 50 ÷ 8 = 6
8 x 6 = 48; Subtract: 50 – 48 = 2
Divide: 20 ÷ 8 = 2;
Subtract: 20 – 16 = 4
Divide: 40 ÷ 8 = 5
8 x 5 = 40; Subtract: 40 – 40 = 0
So, 6.25


Question 4.
80 ÷ 50 = 16
_____
Answer:
1.6
Explanation:
80 ÷ 50
8 ÷ 5
Divide 8 ÷ 5
5 x 1 = 5 : Subtract: 8 – 5 = 3
Divide 30 ÷ 5 = 6
80 ÷ 50 = 1.6
Divide.
Question 5.
4)\(\overline { 32.6 }\) = _____
Answer:
8.15
Explanation:
32.6 ÷ 4
Divide: 32/4 = 8
8 x 4 = 32; Subtract: 32 – 32 = 0
Divide: 6/4 = 1
4 x 1 = 4; Subtract: 6 – 4 = 2
Add zero
20 ÷ 4 = 5
So, 8.15
Question 6.
1.2)\(\overline { 9 }\) = _____
Answer:
0.1333
Explanation:
1.2/9 = 12/90; 12 < 90
12 cannot be divided by 90. So add zero to 12.
120/90 = 1; 120 – 90 = 30; 30 < 90
300/90 = 3; 300 – 270 = 30
So, 0.13333
Question 7.
15)\(\overline { 42 }\) = _____
Answer:
2.8
Explanation:
42/15 = 2
15 x 2 = 30; 42 – 30 = 12
120/15 = 8;
So, 2.8
Question 8.
0.14)\(\overline { 0.91 }\) = _____
Answer:
6.5
Explanation:
0.91/0.14 = 91/14
91/14 = 6
14 x 6 = 84; 91 – 84 = 7; 7 < 14
70/14 = 5
So, 6.5
On Your Own
Divide.
Question 9.
8)\(\overline { 84 }\) = _____
Answer:
10.5
Explanation:
84/8 = 10
8 x 10 = 80; 84 – 80 = 4;4 < 8
40/8 = 5
So, 10.5
Question 10.
2.5)\(\overline { 4 }\) = _____
Answer:
Explanation:
4/2.5 = 40/25
40/25 = 1;
40 – 25 = 15; 15 < 25
150/25 = 6
So, 1.6
Question 11.
5)\(\overline { 16.2 }\) = _____
Answer:
3.24
Explanation:
16.2/5 = 162/50
162/50 = 3
50 x 3 = 150; 162 – 150 = 12; 12 < 50
120/50 = 2
50 x 2 = 100; 120 – 100 = 20; 20 < 50
200/50 = 4
So, 3.24
Question 12.
0.6)\(\overline { 2.7 }\) = _____
Answer:
4.5
Explanation:
2.7/0.6 = 27/6
27/6 = 4
4 x 6 = 24; 27 – 24 = 3; 3 < 6
30/6 = 5
So, 4.5
Question 13.
18 ÷ 7.5 = _____
Answer:
2.4
Explanation:
18/7.5 = 180/75
180/75 = 2
75 x 2 = 150; 180 – 150 = 30; 30 < 75
300/75 = 4
So, 2.4
Question 14.
34.8 ÷ 24 = _____
Answer:
1.45
Explanation:
34.8/24 = 348/240
348/240 = 1;
240 x 1 = 240; 348 – 240 = 108 < 240
1080/240 = 4
240 x 4 = 960; 1080 – 960 = 120; 120 < 240
1200/240 = 5
So, 1.45

Question 16.
81 ÷ 18 = _____
Answer:
4.5
Explanation:
81/18 = 4
18 x 4 = 72; 81 – 72 = 9; 9 < 18
90/18 = 5
4.5
Practice: Copy and Solve Divide.
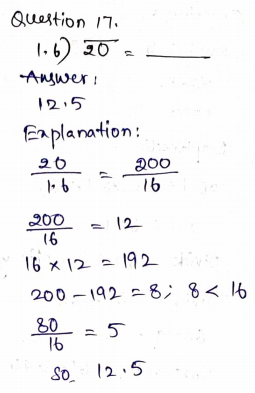
Question 18.
15)\(\overline { 4.8 }\) = _____
Answer:
0.32
Explanation:
4.8/15 = 48/150; 48 < 150
480/150 = 3
150 x 3 = 450; 480 – 450 = 30 < 150
300/150 = 2
0.32
Question 19.
0.54)\(\overline { 2.43 }\) = _____
Answer:
4.5
Explanation:
2.43/0.54 = 243/54
243/54 = 4
54 x 4 = 216; 243 – 216 = 27 < 54
270/54 = 5
4.5
Question 20.
28)\(\overline { 98 }\) = _____
Answer:
3.5
Explanation:
98/28 = 3
28 x 3 = 84; 98 – 84 = 14 < 28
140/28 = 5
3.5
Question 21.
1.8 ÷ 12 = _____
Answer:
0.15
Explanation:
1.8/12 = 18/120; 18 < 120
180/120 = 1;
120 x 1 = 120; 180 – 120 = 60; 60 < 120
600/120 = 5
0.15
Question 22.
3.5 ÷ 2.5 = _____
Answer:
1.4
Explanation:
3.5/2.5 = 35/25
35/25 = 1;
25 x 1 = 25; 35 – 25 = 10; 10 < 25
100/25 = 4
1.4
Question 23.
40 ÷ 16 = _____
Answer:
2.5
Explanation:
40/16 = 2
16 x 2 = 32; 40 – 32 = 8 < 16
80/16 = 5
2.5
Question 24.
2.24 ÷ 0.35 = _____
Answer:
6.4
Explanation:
2.24/0.35 = 224/35
224/35 = 6
35 x 6 = 210; 224 – 210 = 14 < 35
140/35 = 4
6.4
Problem Solving – Page No. 230
Solve.
Question 25.
Jerry takes trail mix on hikes. A package of dried apricots weighs 25.5 ounces. Jerry divides the apricots equally among 6 bags of trail mix. How many ounces of apricots are in each bag?
_____ ounces
Answer:
4.25 ounces
Explanation:
Jerry takes trail mix on hikes. A package of dried apricots weighs 25.5 ounces. Jerry divides the apricots equally among 6 bags of trail mix.
25.5/6 = 4.25 ounces
Question 26.
Amy has 3 pounds of raisins. She divides the raisins equally into 12 bags. How many pounds of raisins are in each bag? Tell how many zeros you had to write at the end of the dividend.
_____ pound
Answer:
4 pound
Explanation:
Amy has 3 pounds of raisins. She divides the raisins equally into 12 bags.
12/3 = 4 pound. There are no zeros required at the end of the dividend.

Question 28.
Test Prep Todd has a piece of rope that is 1.6 meters long. He cuts the rope into 5 equal pieces. What is the length of each piece?
Options:
a. 0.8 meter
b. 0.32 meter
c. 3.2 meters
d. 8 meters
Answer:
b. 0.32 meter
Explanation:
Todd has a piece of rope that is 1.6 meters long. He cuts the rope into 5 equal pieces.
1.6/5 = 0.32 meter
Connect to Science
Rate of Speed Formula
The formula for velocity, or rate of speed, is r = d ÷ t, where r represents rate of speed, d represents distance, and t represents time. For example, if an object travels 12 feet in 10 seconds, you can find its rate of speed by using the formula.
r = d ÷ t
r = 12 ÷ 10
r = 1.2 feet per second
Use division and the formula for rate of speed to solve.

Question 29.
A car travels 168 miles in 3.2 hours. Find the car’s rate of speed in miles per hour.
_____ miles per hour
Answer:
52.5 miles per hour
Explanation:
168/3.2 = 52.5 miles per hour
Question 30.
A submarine travels 90 kilometers in 4 hours. Find the submarine’s rate of speed in kilometers per hour.
_____ kilometers per hour
Answer:
22.5 kilometers per hour
Explanation:
A submarine travels 90 kilometers in 4 hours.
For an hour, 90/4 = 22.5 kilometers per hour
Share and Show – Page No. 233
Question 1.
Hector spent $36.75 for 2 DVDs with the same cost. The sales tax was $2.15. Hector also used a coupon for $1.00 off his purchase. How much did each DVD cost?
First, make a flowchart to show the information and show how you would work backward.
Type below:
_________
Answer:
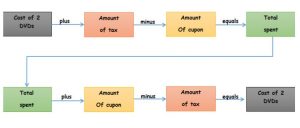
Question 1.
Then, work backward to find the cost of 2 DVDs.
Type below:
_________
Answer:
Price of the DVD is s
2s + $2.15 – $1.00 = $36.75
2s = $35.60
Question 1.
Finally, find the cost of one DVD.
So, each DVD costs ________ .
$ _____
Answer:
2s = $35.60
s = $35.60/2
s = $17.8
Question 2.
What if Hector spent $40.15 for the DVDs, the sales tax was $2.55, and he didn’t have a coupon? How much would each DVD cost?
$ _____
Answer:
Explanation:
If Hector spent $40.15 for the DVDs. The sales tax was $2.55, and he didn’t have a coupon
Price of the DVD is s
2s + $2.55 = $40.15
2s = $37.60
s = $18.8
Each DVD price =$18.8

On Your Own – Page No. 234
Question 4.
The change from a gift purchase was $3.90. Each of 6 students donated an equal amount for the gift. How much change should each student receive?
$ _____
Answer:
$0.65
Explanation:
The change from a gift purchase was $3.90. Each of the 6 students donated an equal amount for the gift.
$3.90/6 = $0.65


Question 7.
Stacy buys 3 CDs in a set for $29.98. She saved $6.44 by buying the set instead of buying the individual CDs. If each CD costs the same amount, how much does each of the 3 CDs cost when purchased individually?
$ _____
Answer:
$12.14
Explanation:
Stacy buys 3 CDs in a set for $29.98. She saved $6.44 by buying the set instead of buying the individual CDs.
Cost to buy 3 CDs = $29.98 + $6.44 = $36.42
$36.42/3 = $12.14
Question 8.
A school cafeteria sold 1,280 slices of pizza the first week, 640 the second week, and 320 the third week. If this pattern continues, in what week will the cafeteria sell 40 slices? Explain how you got your answer.
_____ th week
Answer:
sixth week
Explanation:
A school cafeteria sold 1,280 slices of pizza the first week, 640 the second week, and 320 the third week.
1280, 640, 320
1280/2 = 640
640/2 = 320
320/2 = 160
160/2 = 80
80/2 = 40
6th term shows 40. So, the answer is the sixth week.
Question 9.
Test Prep While working at the school store, John sold a jacket for $40.00 and notebooks for $1.50 each. If he collected $92.50, how many notebooks did he sell?
Options:
a. 3.5
b. 6.1
c. 35
d. 61
Answer:
c. 35
Explanation:
John sold a jacket for $40.00 and notebooks for $1.50 each.
$40 + $1.50S = $92.50
$1.50S = $52.5
S = $52.5/$1.50 = 35
Chapter Review/Test – Page No. 235
Concepts and Skills
Complete the pattern.
Question 1.
341 ÷ 1 = ________
341 ÷ 10 = ________
341 ÷ 100 = ________
341 ÷ 1,000 = ________
Answer:
341 ÷ 1 = 341
341 ÷ 10 = 34.1
341 ÷ 100 = 3.41
341 ÷ 1,000 = 0.341
Explanation:
341 ÷ 1 = 341
341 ÷ 10 = 34.1
341 ÷ 100 = 3.41
341 ÷ 1,000 = 0.341
Question 2.
15 ÷ 1 = ________
15 ÷ 10 = ________
15 ÷ 100 = ________
15 ÷ 1,000 = ________
Answer:
15 ÷ 1 = 15
15 ÷ 10 = 1.5
15 ÷ 100 = 0.15
15 ÷ 1,000 = 0.015
Explanation:
15 ÷ 1 = 15
15 ÷ 10 = 1.5
15 ÷ 100 = 0.15
15 ÷ 1,000 = 0.015
Question 3.
68.2 ÷ 100 = ________
68.2 ÷ 101 = ________
68.2 ÷ 102 = ________
Answer:
68.2 ÷ 100 = 68.2
68.2 ÷ 101 = 6.82
68.2 ÷ 102 = 0.682
Explanation:
68.2 ÷ 100 = 68.2 ÷ 1 = 68.2
68.2 ÷ 101 = 68.2 ÷ 10 = 6.82
68.2 ÷ 102 = 68.2 ÷ 100 = 0.682
Estimate the quotient.
Question 4.
49.3 ÷ 6 = _____
Answer:
About 8
Explanation:
49.3 is closer to 48
48 ÷ 6 = 8
Question 5.
3.5 ÷ 4 = _____
Answer:
about 1
Explanation:
3.5 is closer to 4
4 ÷ 4 = 1
Question 6.
396.5 ÷ 18 = _____
Answer:
About 22
Explanation:
396.5 is closer to 396
396 ÷ 18 = 22
Divide.
Question 7.
6)\(\overline { 3.24 }\) = _____
Answer:
0.54
Explanation:
3.24 ÷ 6
Divide. 3 ones ÷ 6
3 one(s) cannot be shared among 6 groups without regrouping.
30 + 2 = 32 tenths
Divide. 32 tenths ÷ 6
Multiply. 6 x 5 tenths = 30 tenths. Subtract. 32 tenths − 30 tenths = 2 tenths
Check. 30 tenths shared among 6 groups as 5 tenths.
2 tenths(s) cannot be shared among 6 groups without regrouping.
1 tenth = 10 hundredths.
20 + 4 = 24 hundredths
Divide. 24 hundredths ÷ 6
Multiply. 6 x 4 hundredths = 24 hundredths. Subtract. 24 hundredths − 24 hundredths = 0 hundredths
Check. 24 hundredths shared among 6 groups as 4 hundredths.
So, 0.54
Question 8.
5)\(\overline { 6.55 }\) = _____
Answer:
1.51
Explanation:
6.55 ÷ 5
Divide. 6 ones ÷ 5
Multiply. 5 × 1 ones = 5 ones. Subtract. 6 ones − 5 ones = 1 ones
Check. 5 ones shared among 5 groups as 1 whole.
1 one(s) cannot be shared among 5 groups without regrouping.
10 + 5 = 15 tenths
Divide. 15 tenths ÷ 5
Multiply. 5 x 3 tenths = 15 tenths. Subtract. 15 tenths − 15 tenths = 0 tenths
Check. 35 tenths shared among 5 groups as 3 tenths.
Divide. 5 hundredths ÷ 5
Multiply. 5 x 1 hundredths = 5 hundredths. Subtract. 5 hundredths − 5 hundredths = 0 hundredths
Check. 5 hundredths shared among 5 groups as 1 hundredth.
So, 1.51
Question 9.
26)\(\overline { 96.2 }\) = _____
Answer:
3.7
Explanation:
96.2 ÷ 26
Divide. 96 ones ÷ 26
Multiply. 26 × 3 ones = 78 ones. Subtract. 96 ones − 78 ones = 18 ones
Check. 78 ones shared among 26 groups as 3 wholes.
18 one(s) cannot be shared among 26 groups without regrouping.
180 + 2 = 182 tenths
Divide. 182 tenths ÷ 26
Multiply. 26 x 7 tenths = 182 tenths. Subtract. 182 tenths − 182 tenths = 0 tenths
So, 3.7
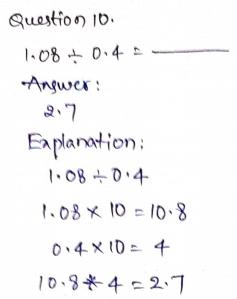
Question 12.
7.31 ÷ 1.7 = _____
Answer:
4.3
Explanation:
7.31 ÷ 1.7
7.31 x 10 = 73.1
1.7 x 10 = 17
73.1 ÷ 17 = 4.3
Question 13.
9.18 ÷ 0.9 = _____
Answer:
10.2
Explanation:
9.18 ÷ 0.9
9.18 x 10 = 91.8
0.9 x 10 = 9
91.8 ÷ 9 = 10.2
Question 14.
12.7 ÷ 5 = _____
Answer:
2.54
Explanation:
12.7 ÷ 5
12.7 x 10 = 127
5 x 10 = 50
127 ÷ 50 = 2.54
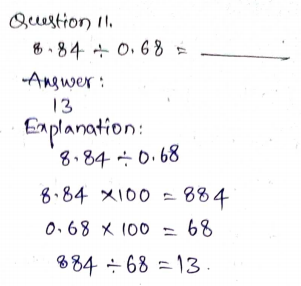
Question 15.
8.33 ÷ 0.34 = _____
Answer:
24.5
Explanation:
8.33 ÷ 0.34
8.33 x 100 = 833
0.34 x 100 = 34
833 ÷ 34 = 24.5
Chapter Review/Test – Page No. 236
Fill in the bubble completely to show your answer.
Question 16.
The Orchard Pie Company uses 95 pounds of apples to make 100 pies. Each pie contains the same amount of apples. How many pounds of apples are used in each pie?
Options:
A. 0.095 pound
B. 0.95 pound
C. 9.5 pounds
D. 95 pounds
Answer:
B. 0.95 pound
Explanation:
The Orchard Pie Company uses 95 pounds of apples to make 100 pies. Each pie contains the same amount of apples.
95/100 = 0.95 pounds
Question 17.
During a special sale, all CDs have the same price. Mr. Ortiz pays $228.85 for 23 CDs. Which is the best estimate of the price of each CD?
Options:
A. $9
B. $10
C. $12
D. $13
Answer:
B. $10
Explanation:
During a special sale, all CDs have the same price. Mr. Ortiz pays $228.85 for 23 CDs.
$228.85/23 = $9.95
Question 18.
Ryan earns $20.16 working for 3 hours. How much does he earn per hour?
Options:
A. $60.48
B. $6.82
C. $6.72
D. $6.71
Answer:
C. $6.72
Explanation:
Ryan earns $20.16 working for 3 hours.
$20.16/3 = $6.72
Question 19.
Anna hikes 6.4 miles during a 4-day vacation. If she hikes the same distance each day, how many miles does she hike each day?
Options:
A. 1.06 miles
B. 1.1 miles
C. 1.4 miles
D. 1.6 miles
Answer:
D. 1.6 miles
Explanation:
Anna hikes 6.4 miles during a 4-day vacation. If she hikes the same distance each day, 6.4/4 = 1.6 miles
Chapter Review/Test – Page No. 237
Fill in the bubble completely to show your answer.
Question 20.
Karina pays $1.92 for pencil erasers. The erasers cost $0.08 each. How many erasers does she buy?
Options:
A. 2.4
B. 2.5
C. 24
D. 25
Answer:
C. 24
Explanation:
Karina pays $1.92 for pencil erasers. The erasers cost $0.08 each.
$1.92/$0.08 = 24
Question 21.
Wyatt has 25.4 ounces of fruit juice. He divides the juice equally into 4 glasses. How much juice is in each glass?
Options:
A. 6 ounces
B. 6.35 ounces
C. 6.4 ounces
D. 6.45 ounces
Answer:
B. 6.35 ounces
Explanation:
Wyatt has 25.4 ounces of fruit juice. He divides the juice equally into 4 glasses.
25.4/4 = 6.35 ounces
Question 22.
Jacob walks 70.4 feet in 0.2 hour. If he walks at the same rate the whole time, what is his speed in feet per hour?
Options:
A. 352 feet per hour
B. 140.8 feet per hour
C. 35.2 feet per hour
D. 14.08 feet per hour
Answer:
A. 352 feet per hour
Explanation:
Jacob walks 70.4 feet in 0.2 hour. If he walks at the same rate the whole time,
7.4/0.2 = 352 feet per hour
Question 23.
Meghan earns $20.00 by walking dogs. She uses all of her earnings to buy a shirt for $12.85 and some stickers for $0.65 each. How many stickers does she buy?
Options:
A. 4.65
B. 11
C. 46
D. 110
Answer:
B. 11
Explanation:
Meghan earns $20.00 by walking dogs. She uses all of her earnings to buy a shirt for $12.85 and some stickers for $0.65 each.
$20 – $12.85 = $7.15
$7.15/$0.65 = 11
Chapter Review/Test – Page No. 238
Constructed Response

Performance Task
Question 25.
Isabella is buying art supplies. The table at the right shows the prices of the items she wants to buy.
Isabella spends $2.25 on poster boards. How many poster boards does she buy?
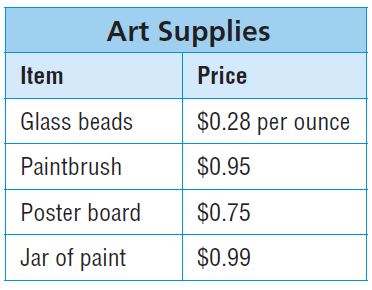
_____ posters
Answer:
3 posters
Explanation:
1 Poster Board = $0.75.
Isabella spends $2.25 on poster boards.
$2.25/$0.75 = 3
Question 25.
B Isabella spends $4.87 on paintbrushes and paint. How many of each item does she buy? Explain how you found your answer.
_______ paint brushes
_______ jars of paint
Answer:
2 paint brushes
3 jars of paint
Explanation:
paintbrushes = $0.95
Paint = $0.99
If she buys 2 paint brushes and 2 paints, she spent $1.9 for paintbrushes and $1.98 for 2 paints. The remaining amount is $0.99. So, she can buy one more paint with them. So, she can buy 2 paint brushes and 3 jars of paint.
Question 25.
C. Isabella spends less than $14.00 for glass beads, paintbrushes, poster boards, and paint. She spends $1.68 on beads and $3.96 on paint. She buys more than 3 poster boards and more than 3 paintbrushes. Find how many ounces of glass beads and how many jars of paint she buys. Then, suggest the number of poster boards and paintbrushes she might buy for the total spent.
Type below:
_________
Answer:
Isabella spends less than $14.00 for glass beads, paintbrushes, poster boards, and paint.
She spends $1.68 on beads and $3.96 on paint.
Each beads = $0.28
$1.68/$0.28 = 6 beads
Each paint = $0.99
$3.96/$0.99 = 4 paints
$14 – ($1.68 + $3.96) = $8.36.
So, $8.36 needs to spend on more than 3 poster boards and more than 3 paintbrushes.
So, Each poster board = $0.75
paintbrushes = $0.95
If she buys more than 3, 3 x $0.75 = $2.25
$0.95 x 3 = $2.85
$2.25 + $2.85 = $5.1
So, $8.36 – $5.1 = $3.26
If $0.75 x 3 = $2.25
$0.95 x 1 = $0.95
$2.25 + $0.95 = 3.2
So, she can buy 3 + 3 = 6 poster boards
and 3 + 1 = 4 paintbrushes.
Conclusion
We have given Go Math Grade 5 Answer Key Chapter 5 Divide Decimals here for free of cost. Learn the Simple Tricks and Tips to Solve Various Models on Divide Decimals. Step by Step Solutions is provided for all the Questions to make it easy for you to understand.
