Find the steps of solving first-order linear equations with an example question. By following these steps, one can solve the linear equations in one variable, two variables, and three variables easily. Also, learn how to represent the linear equations on a graph from this page. So, read this complete page to get useful information. For the sake of your convenience, we even listed Linear Equations Examples with Solutions explained in detail.
Steps on How to Solve Linear Equations?
Find the step-by-step process for solving the linear equations by addition, subtraction, multiplication, and division in the following sections.
- Transfer the variable to the one side and constants to the other side of the equality sign by performing the required arithmetic operations.
- Now, make the variable equal to constant.
- Take a number line graph.
- Represent the solution on a graph by marking *.
What is Cross Multiplication?
If an equation has a fraction on both sides, then we will use cross multiplication. The process of multiplying the numerator of the left-hand side fraction with the denominator of the right-hand side fraction and multiplying the denominator of the left-hand side fraction with the numerator of the right-hand side fraction is called cross-multiplication. And equate both products to get a linear equation.
Solving Linear Equations Examples
Example 1.
Solve 0.5 – 1.5x = 0.8 – 0.28x.
Solution:
Given that,
0.5 – 1.5x = 0.8 – 0.28x
Transfer – 1.5x from the left side to right side. And transfer 0.8 from right side to the left side.
0.5 – 0.8 = 1.5x – 0.28x
-0.3 = 1.22x
x = -0.3 / 1.22
x = -0.245
Therefore, -0.245 is the required solution.
Verification:
L.H.S = 0.5 – 1.5x
Put x = -0.245
L.H.S = 0.5 – 1.5 x (-0.245)
= 0.5 + 1.5 x 0.245
= 0.5 + 0.3675
= 0.8675
R.H.S = 0.8 – 0.28x
Put x = -0.245
R.H.S = 0.8 – 0.28 x (-0.245)
= 0.8 + 0.28 x 0.245
= 0.8 + 0.0686
= 0.8686
Since, L.H.S = R.H.S. Hence proved.
Example 2.
Solve the equation 5 – 2(x – 1) = 4(3 – x) – 2x and represent the solution graphically.
Solution:
Given linear equation is 5 – 2(x – 1) = 4(3 – x) – 2x
Remove the braces and then simplify
5 – 2x + 2 = 12 – 3x – 2x
7 – 2x = 12 – 6x
Transfer -6x to L.H.S. changes to positive 6x and 7 to R.H.S. changes to negative 7.
6x – 2x = 12 – 7
4x = 5
x = 5/4
The solution may be represented graphically on the number line by graphing linear equations.
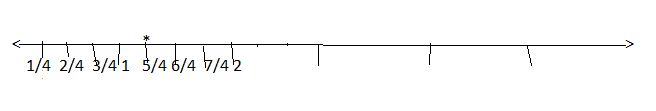
Example 3.
Solve the equation 6(2x + 3) + 5(4x – 6) – 8x = 5(3x + 1) + 6 (7x – 6) and verify your answer.
Solution:
Given linear equation is 6(2x + 3) + 5(4x – 6) – 8x = 5(3x + 1) + 6 (7x – 6)
12x + 18 + 20x – 30 – 8x = 15x + 5 + 42x – 36
24x – 12 = 57x – 31
31 – 12 = 57x – 24x
19 = 33x
19/33 = x
x = 19/33
Therefore, x = 19/33 is the solution for the equation.
Now we will verify both sides of the equation,
L.H.S = 6(2x + 3) + 5(4x – 6) – 8x
Sunstitute x = 19/33
L.H.S = 6[2 x 19/33 + 3] + 5[4 x 19/33 – 6] – 8 x 19/33
= 6[38/33 + 3] + 5[76/33 – 6] – 152/33
= 228/33 + 18 + 380/33 – 30 – 152/33
= 456/33 – 12
= (456 – 396)/33 = 60/33
R.H.S = 5(3x + 1) + 6 (7x – 6)
Sunstitute x = 19/33
R.H.S = 5[3 x 19/33 + 1] + 6[7 x 19/33 – 6]
= 5[57/33 + 1] + 6[133/33 – 6]
= 285/33 + 5 + 798/33 – 36
= 1083/33 – 31
= (1083 – 1023)/33 = 60/33
Since, L.h.S = R.H.S. Hence proved.
Example 4.
Solve 12/(x – 8) = 3/(x + 4).
Solution:
Given linear equation is 12/(x – 8) = 3/(x + 4)
Cross multiply and then remove the braces.
12(x + 4) = 3(x – 8)
12x + 48 = 3x – 24
12x – 3x = -24 – 48
9x = -72
x = -72/9 = -8
Therefore, x = -8 is the solution for the equation.
Verification:
L.H.S = 12/(x – 8)
Put x = -8
L.H.S = 12/(-8 – 8)
= 12/-16
= -3/4
R.H.S = 3/(x + 4)
Put x = -8
R.H.S = 3/(-8 + 4)
= 3/-4
= -3/4
Since L.H.S = R.H.S. Hence verified.