Linear inequations demonstrate the value of one quantity or algebraic expression which is not equal to another. These inequations are used to compare any two quantities. Get some solved examples on linear inequations and steps to draw graphs, the system of linear inequations in the following sections of this page.
- Linear Inequality and Linear Inequations
- Properties of Inequation or Inequalities
- Representation of the Solution Set of an Inequation
- Practice Test on Linear Inequations
What are Linear Inequations?
Linear inequalities are the expressions where any two values are compared by the inequality symbols. These are the equations that contain all mathematical symbols except equal to (=). The values can be numerical or algebraic or a combination of both.
The inequality symbols are < (less than), > (greater than), ≤ (less than or equal to), ≥ (greater than or equal to), ≠ (not equal to). The symbols < and > shows the strict inequalities and the symbols ≤ and ≥ represents the slack inequalities.
Example:
x < 3, x ≥ 5, y ≤ 8, p > 10, m ≠ 1.
Linear Inequalities Graphing
We can plot the graph for linear inequalities like an ordinary linear function. But, for a linear function, the graph represents a line and for inequalities, the graph shows the area of the coordinate plane that satisfies the inequality condition. The linear inequalities graph divides a coordinate plane into two parts. One part of the coordinate plane is called the borderline where it represents the solutions for inequality. The borderline represents the conditions <, >, ≤ and ≥.
Students who are willing to plot a graph for the linear inequations can refer to the following steps.
- Arrange the given linear inequation in such a way that, it should have one variable ‘y’ on the left-hand side of the symbol and the remaining equation on the right-hand side.
- Plot the graph for linear inequation by putting the random values of x.
- Draw a thicker and solid line for y≤ or y≥ and a dashed liner for y< or y> conditions.
- Now, draw shades as per the linear inequalities conditions.
System of Linear Inequalities
A system of linear inequalities in two variables includes at least two inequalities in the variables. By solving the linear inequality you will get an ordered pair. So basically, the solution to all linear inequalities and the graph of the linear inequality is the graph displaying all solutions of the system.
Questions on Linear Inequations
Example 1.
Solve the inequality x + 5 < 10?
Solution:
Given that,
x + 5 < 10
Move variable x to the one side of inequation.
= x < 10 – 5
= x < 5
Replacement set = {0, 1, 2, 3, 4, 5, 6, 7 . . . }
Solution set for the inequation x + 5 < 10 is 1, 2, 3, 4
Therefore, solution set s = {. . . 1, 2, 3, 4}
Let us mark the solution set graphically.
![]()
The solution set is marked on the number line by dots. We put three more dots indicate the infiniteness of the solution set.
Example 2.
Solve the inequation 3 < y ≤ 10?
Solution:
Given that,
3 < y ≤ 10
This has two inequations,
3 < y and y ≤ 10
Replacement set = {. . . -3, -2, -1, 0, 1, 2, 3, 4, , 6, 7, . . . }
Solution set for the inequation 3 < y is 4, 5, 6, 7, . . . i.e Q = {4, 5, 6, . . . }
Solution set for the inequation y ≤ 10 is . . . . 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 i.e P = {. . . . 0, 1, 2, 3, 4, 5, 6, 7, 8, 9}
Therefore, solution set of the given inequation = P ∩ Q = {4, 5, 6, 7, 8, 9}
Let us represent the solution set graphically.
![]()
The solution set is marked on the number line by dots.
Example 3.
Solve the inequality 4 ( x + 2 ) − 1 > 5 − 7 ( 4 − x )?
Solution:
Given that,
4 ( x + 2 ) − 1 > 5 − 7 ( 4 − x )
4 x + 8 − 1 > 5 − 28 + 7 x
4 x + 7 > − 23 + 7 x
− 3 x > − 30
x < 10
Replacement Set = {1, 2, 3, 4, 5 . . . }
Solution set is 1, 2, 3, 4, 5, 6, 7, 8, 9 i.e S = {1, 2, 3, 4, 5, 6, 7, 8, 9}
Let us represent the solution set graphically.
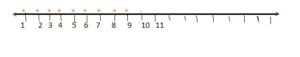
The solution set is marked on the number line by dots.
Frequently Asked Questions on Linear Inequations
1. What is meant by linear inequations?
Linear inequations involve linear expressions in two variables by using the relational symbols like <, >, ≤, ≥, and ≠.
2. How do you solve linear inequation?
Solving linear inequations having a single variable is very easy. All you have to do is simplify both the sides of the condition and get the variable term on one side and all other terms on the other side. And them either multiply/ divide the coefficient of the variable to obtain the solution.
3. What are the examples of linear inequations?
Some of the examples of linear inequality are x < 8, y > 15, z ≠ 7 + 9, p + 1 ≤ 2, 3 ≥ (z + 15).