Pythagora’s theorem was introduced by the famous mathematician named Pythagoras. The other names are Pythagorean theorem, Pythagoras triplets. The theorem is defined as the sum of the squares of the legs of a right-angled triangle is same as the square of the hypotenuse side. In the following sections, we solve different types of questions on establishing riders on Pythagora’s theorem.
Pythagoras’ Theorem
The Pythagorean theorem states that if a triangle is said to be a right-angled triangle, the square of the hypotenuse side is equal to the sum of the squares of the base and perpendicular sides. Here, the hypotenuse is the longest side or the side which is opposite to 90 degrees in a triangle. The perpendicular side is nothing but the altitude of the triangle and the remaining is base.
The Pythagoras equation is c² = a²+ b². Where a, b, c are sides of the right triangle. Its formula can be Hypotenuse² = Perpendicular²+ Base². Some of the applications are woodworking, surveying, engineering and construction field and so on.
Riders Based on Pythagoras’ Theorem
The rider is a combination of two or more right triangles. The following are the various types of examples on establishing riders based on the Pythagorean theorem.
- In a quadrilateral ABCD the diagonals AC, BD intersect at a right angle, then AB² + CD² = AD² + BC².
- In a rectangle PQRS, O is the point inside the rectangle, then OP² + OR² = OQ²+ OS².
- In ∆XYZ, ∠Z is acute and XM ⊥ YZ, M being the foot of the perpendicular, then 2YZ . ZM = YZ² + ZX² – XY².
- In ∆XYZ, ∠Z = 90° and ZM ⊥ XY, where M is the foot of the perpendicular, then \(\frac { 1 }{ ZM² } \) = \(\frac { 1 }{ YZ² } \) + \(\frac { 1 }{ XZ² } \).
Problems on Establishing Riders Based on Pythagoras’ Theorem
Problem 1:
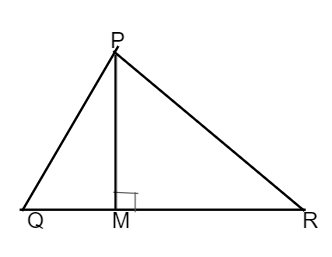
In ∆PQR, ∠R is acute and PM ⊥ QZ, M being the foot of the perpendicular. Prove that 2QR ∙ RM = QR² + RP² – PQ².
Solution:
∆PMQ is a right-angled triangle.
Using Pythagorean theorem, PQ² = PM² + QM²
PQ² = PM² + (QR – MR)²
PQ² = PM² + QR²+ MR² – 2 . QR . MR
= (PM² + MR²) + QR² – 2 . QR . MR
= PR² – 2 . QR . MR + QR²
2QR . MR = PR² + QR² – PQ²
Hence proved.
Problem 2:
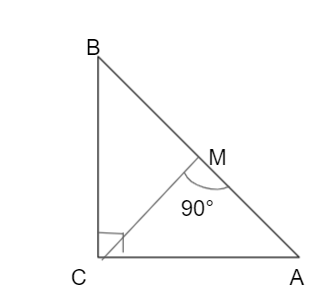
In ∆ABC, ∠C = 90° and CM ⊥ AY, M is the foot of the perpendicular. Prove that latex]\frac { 1 }{ CM² } [/latex] = \(\frac { 1 }{ BC² } \) + \(\frac { 1 }{ AC² } \).
Solution:
In ∆ABC and ∆MBC,
∠ACB = ∠CMB = 90°, and ∠ABC = ∠CBM (Common Angle)
Using AA criterion of similarity, ∆ABC ∼ ∆CBM.
\(\frac { AB }{ BC } \) = \(\frac { AC }{ CM } \)
So, AB . CM = BC . AC
Therefore, CM = \(\frac { BC . AC }{ AB } \)
Using Pythagora’s theorem, \(\frac { 1 }{ CM² } \) = \(\frac { AB² }{ BC² . AC² } \)
= \(\frac { AC² + BC² }{ BC² . AC² } \)
\(\frac { 1 }{ CM² } \) = \(\frac { 1 }{ BC² } \) + \(\frac { 1 }{ AC² } \)
Hence, proved.
Problem 3:
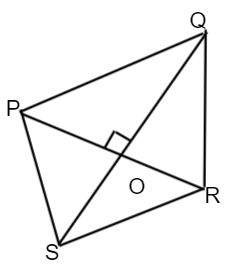
In the quadrilateral PQRS the diagonals PR and QS intersects at a right angle. Prove that PQ²+ RS² = PS² + QR².
Solution:
The point of intersection of two diagonals is O. The angle of intersection is the right angle.
In the right-angle ∆POQ, PQ² = OP² + OQ²
In the right-angle ∆ROS, RS² = OR² + OS²
So, PQ² + RS² = OP² + OQ² + OR² + OS² —- (i)
In the right-angle ∆POS, PS² = OP² + OS²
In the right-angle ∆QOR, QR² = OQ² + OR²
So, PS² + QR² = OP² + OS² + OQ² + OR² —- (ii)
From above two equations
PQ² + RS² = PS² + QR²
Hence, proved.
Problem 4:
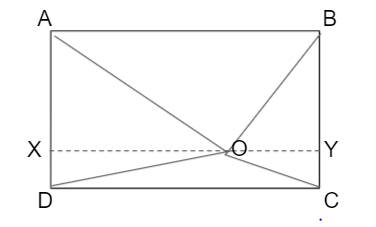
Let ABCD be a rectangle. O is a point inside the rectangle. Prove that OA² + OC² = OB² + OD².
Solution:
ABCD is a rectangle, where AB = CD = length and AD = BC = breadth
Join OA, OB< OC, and OR
Draw XY through O, parallel to AB.
As ∠BAD and ∠CDA are right angles, ∆AXO, ∆DXO, ∆CYO and ∆BYO are right-angled triangles.
Uisng Pythagora’s theorem,
OA² = AX² + OX²
OC² = CY² + OY²
OB² = BY² + OY²
OD² = DX²+ OX²
Therefore, OA² + OC² = AX² + OX² + CY² + OY² —- (i)
OB² + OD² = BY² + OY² + DX² + OX² ……… (ii)
But in the rectangle XDCY, DX = CY = breadth and in the rectangle AXYB, AX = BY = breadth
From (i) and (ii), OA² + OC² = OB² + OD²
Hence proved.
Frequently Asked Questions
1. What are riders in a triangle?
A triangle is formed by connecting three points – the rider’s seating position, the handlebar position and the footrest.
2. Where was the Pythagoras theorem used in real life?
The application of the Pythagorean theorem is in architecture woodworking, construction and engineering or physical construction projects.
3. What is Pythagoras theorem based on?
Pythagoras theorem is the well known geometric theorem that is based on an aright angled triangle.