Common Core Math

In this article, you will learn about the Decimal and Fractional Expansion of a Decimal Number. Before Proceeding further know the definitions of Decimal, Fraction, and the Place Value Chart. A Decimal is any number in the base 10 number system and is used to separate units place from tenths place in decimal. The decimal point present in between separates the Whole Number Part and Decimal Part.

Also, Read:

• Thousandths Place in Decimals
• Place Value
• Decimal Place Value Chart

Decimal Expansion of a Number

Decimal Expansion of a Number is its representation in the base-10 system. In this System, each decimal place consists of digits 0-9 arranged such that each digit is multiplied by a power of 10 decreasing from left to right and a decimal place with 10^0 is the one’s place.

Decimal Expansion of Number 1423.25 is defined as

1423.25 = 1*10³+4*10²+2*10¹+3*10⁰+2*10^-1+5*10^-2

= 1000+400+20+3+0.2+0.05

Decimal Expansion of a Number may terminate and in such case, the number is called a regular number or finite decimal. At times, the Decimal Expansion of a Number may become periodic and in such case, it is called a repeating decimal. However, the expansion may continue infinitely without repeating and it is called an irrational number.

Fractional Expansion of Decimals

In the Expanded Form of Decimal Fractions, you will learn how to read and write the Decimal Numbers. Decimal Numbers can be written in expanded form using the Place Value Chart.

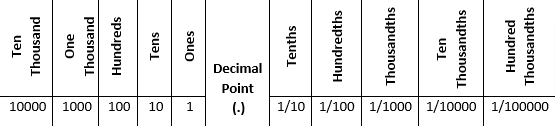
Let us understand the same by considering an example

384.264

384.264 = 3 × 100 + 8 × 10 + 4 × 1 + 2 × \(\frac { 1 }{10 } \) + 6 × \(\frac { 1 }{100 } \) + 4 × \(\frac { 1 }{1000 } \)

= 300+80+4+\(\frac { 2 }{10 } \)+\(\frac { 6 }{100 } \)+\(\frac { 4 }{1000 } \)

Solved Examples on Decimal and Fractional Expansion

1. Write the decimal and fractional expansion of 334.252?

Solution:

In Decimal Expansion

334.252 = 3*100+3*10+4*1+2*\(\frac { 1 }{10 } \)+5*\(\frac { 1 }{100 } \)+2*\(\frac { 1 }{1000 } \)

= 300+30+4+\(\frac { 2 }{10 } \)+\(\frac { 5 }{100 } \) + \(\frac { 2 }{1000 } \)

= 300+30+4+0.2+0.05+0.002

In Fractional Expansion

= 3*100+3*10+4*1+2*\(\frac { 1 }{10 } \)+5*\(\frac { 1 }{100 } \)+2*\(\frac { 1 }{1000 } \)

= 300+30+4+\(\frac { 2 }{10 } \)+\(\frac { 5 }{100 } \) + \(\frac { 2 }{1000 } \)

2. Write the decimal and fractional expansion of 543.32?

Solution:

In Decimal Expansion

543.32 = 5*100+4*10+3*1+3*\(\frac { 1 }{10 } \)+2*\(\frac { 1 }{100 } \)

= 500+40+3+\(\frac { 3 }{10 } \)+\(\frac { 2 }{100 } \)

= 500+40+3+0.3+0.02

In Fractional Expansion

= 5*100+4*10+3*1+3*\(\frac { 1 }{10 } \)+2*\(\frac { 1 }{100 } \)

= 500+40+3+\(\frac { 3 }{10 } \)+\(\frac { 2 }{100 } \)

3. Write the Decimal and Fractional Expansion of 647.345?

Solution:

In Decimal Expansion

647.345 = 6*100+4*10+7*1+3*\(\frac { 1 }{10 } \)+4*\(\frac { 1 }{100 } \)+5*\(\frac { 1 }{1000 } \)

= 600+40+7+\(\frac { 3 }{10 } \)+\(\frac { 4 }{100 } \)+\(\frac { 5 }{1000 } \)

= 600+40+7+0.3+0.04+0.005

In Fractional Expansion

647.345 = 6*100+4*10+7*1+3*\(\frac { 1 }{10 } \)+4*\(\frac { 1 }{100 } \)+5*\(\frac { 1 }{1000 } \)

= 600+40+7+\(\frac { 3 }{10 } \)+\(\frac { 4 }{100 } \)+\(\frac { 5 }{1000 } \)

FAQ’s on Decimal and Fractional Expansion

1. What is Decimal in Expanded Form?

Expanded form notation for the decimal numbers is the mathematical expression that shows the sum of the values of each digit in the number.

2. What is the Decimal Expansion of Number 164.38?

Decimal Number 164.38 can be written in expanded form by writing it as the sum of the place value of all the digits i.e. 1*100+6*10+4*1+3*\(\frac { 1 }{10 } \)+8*\(\frac { 1 }{100 } \) = 100+60+4+0.3+0.08

3. What is the Fractional Expansion of Number 94.38?

Fractional Expansion of Number 94.38 is 9*10+4*10+3*\(\frac { 1 }{10 } \)+8*\(\frac { 1 }{100 } \) which inturn results in 90+40+\(\frac { 3 }{10 } \)+\(\frac { 8 }{100 } \)

Before we learn about the Order of a Matrix let us know What is a Matrix. Matrices are defined as a rectangular array of numbers or functions. It is a rectangular array and two- dimensional. A Matrix is a rectangular array of numbers or symbols which are generally arranged in rows and columns. The plural of matrix is matrices. The entries are the numbers in the matrix and each number is known as an element.

Order a Matrix – Definition

Basically, a two-dimensional matrix consists of the number of rows and the number of columns. The order of the matrix is defined as the number of rows and columns. The number of rows is represented by ‘m’ and the number of columns is represented by ‘n’. Therefore the order of the matrix is equal to m x n, and it is also called as ‘m by n’.

The size of the matrix is written as m x n, where m is the number of rows and n is the number of columns. For example, we have a 3 x 2 matrix, the number of rows is equal to 3, and the number of columns is equal to 2.

Different Types of Matrices

There are different types of matrices, basically categorized on the basis of the value of their elements, their order,  number of rows and number of columns, etc. Now, using different conditions, the various matrix types are categorized along with their definition.

1. Row Matrix
2. Column Matrix
3. Null Matrix
4.  Square Matrix
5. Diagonal Matrix
6. Upper Triangular Matrix
7. Lower Triangular Matrix
8. Symmetric Matrix
9. Anti- Symmetric Matrix
10. Equal Matrix
11. Singular Matrix
12. Non Singular Matrix
13. Horizontal Matrix
14. Vertical Matrix
15. Unity or identity Matrix.

Row Matrix: A Matrix having only one row is called a Row Matrix. A= [a_ij]_mxn  is a row matrix , if m=1 then row matrix is represented as A= [a_ij]_1xn . It has only one row and the order of a matrix will be 1 x n. For example, A= [1 2 4 5] is row matrix of order 1 x 4.

Column Matrix: A Matrix having only one column is called a Column Matrix. A= [a_ij]_mxn  is a column matrix, if n=1 then column matrix is represented as A= [a_ij]_mx1 . It has only one column and the order of a matrix will be m x 1. Just like row matrices had only one row, column matrices have only one column. Thus the value of the column matrix will be 1.

Null Matrix: Null Matrix is also called Zero Matrix. In a matrix all the elements are zero then it is called a Zero Matrix or Null Matrix and it is generally denoted by 0. Thus, A = [a_ij]_mxn is a Zero or Null Matrix.

Unity or Identity Matrix: If a square matrix has all elements 0 and each diagonal element is non-zero, it is called an identity matrix. It is denoted by I.

Equal Matrix: If two matrices are said to be equal if they are same order if their corresponding elements are equal to the square matrix.

Square Matrix: If the number of rows and the number of columns in a matrix are equal, then it is called Square Matrix.

How to find Order of a Matrix?

A Two- Dimensional matrix consists of the number of rows (m) and a number of columns (n). The order of the matrix is equal to m x n (also pronounced as ‘m by n’).

Order of Matrix = Number of Rows x Number of Columns.

In the above, you can see, the matrix has 2 rows and 4 columns. Therefore, the order of the matrix is 2 x 4.

How will you Determine the Order of Matrix?

If a matrix has m number of rows and n number of columns, now let’s know how to find the order of the matrix.

Here few examples, how to find the order of a matrix,

[1  2  3] is an example, in this, the order of the matrix is (1 x 3), which means the number of rows (m) is 1 and the number of columns (n) is 3.

[7  5] is an example of (1 x 2) matrix, in this number of rows are (m) is 1 and number of columns (n) is 2.
\( A =\left[
\begin{matrix}
6 & 2 & 3\cr
12 & 15 & 35 \cr
\end{matrix}
\right]
\)

The order of the above matrix is (2 x 3), in this number of rows is (m) is 2, and the number of columns (n) is 3.

A matrix of the order m x n has mn elements. Hence we say that if the number of elements in a matrix is prime, then it must have one row or one column. Usually, we denote a matrix by using capital letters such as A, B, C, D, M, N, X, Y, Z, etc.

The product of m and n can be obtained in more than one ways, some of the ways are,

1. mn x 1
2. 1 x mn
3. m x n
4. n x m

Number of Elements in Matrix

Suppose, A is the order of 2 x 3. Therefore, the number of elements present in a matrix will also be 2 times 3, i.e 6.

Similarly, the other matrix order is 4 x 3, thus the number of elements will be 12 i.e. 4 times 3. If we know the order of a matrix, we can easily determine the total number of elements that the matrix has,

If a matrix is of m x n order, it will have mn element.

Order of a Matrix Examples

Example 1.

If matrix A has an 8 number of elements, then determine the order of the matrix.

Solution:

We know that number of elements is 8

Let’s write all the possible factors of the number 8

8 = 1 x 8

8 = 4 x 2

8 = 2 x 4

8 = 8 x 1

we can get the number 8 is four ways.

Therefore, there are four possible ways or orders of the matrix with 8 number of elements are 1 x 8, 2 x 4, 4 x 2, and 8 x 1.

Example 2.

If a matrix X has 7 number of elements, find the order of the matrix.

Solution:

We know that number of elements is 7

Let’s write all the possible factors of the number 7

7 = 1 x 7

7= 7 x 1

we can get the number 7 in two ways.

Therefore, there are two possible ways or orders of the matrix with 7 number of elements are 1 x 7, 7 x 1.

Example 3.

What is the order of a matrix given below?

\( A =\left[
\begin{matrix}
1 & 2 & 9\cr
18 & 12 & 15 \cr
\end{matrix}
\right]
\)

Solution:

The number of rows in a given matrix is A = 2

The number of columns in a given matrix is A = 3

Therefore, the order of matrix is  2 x 3.

Example 4.

What is the order of a matrix given below?

\( A =\left[
\begin{matrix}
4 & 5 & 7\cr
11 & 14 & 18 \cr
21 & 24 & 8 \cr
\end{matrix}
\right]
\)

Solution:

The number of rows in a given matrix is A = 3

The number of columns in a given matrix is A = 3

Therefore, the order of the matrix is 3 x 3.

FAQ’s on Order of Matrix

1. What is Matrix and Types?

A Matrix can be defined as a rectangular array of numbers or functions. A matrix consists of rows (m) and columns (n) that is m x n. Types of Matrices are :

1. Row Matrix
2. Column Matrix
3. Null Matrix
4. Equal Matrix
5. Unity or Identity Matrix
6. Square Matrix
7. Rectangular Matrix
8. Horizontal Matrix
9. Vertical Matrix
10. Scalar Matrix

2. What is the Null Matrix?

Null Matrix is also called Zero Matrix. In a matrix all the elements are zero then it is called a Zero Matrix or Null Matrix and it is generally denoted by 0. Thus, A = [a_ij]_mxn is a Zero or Null Matrix.

3. What is another name of Unity Matrix?

Another name of the unity matrix is Identity Matrix. If a square matrix has all elements 0 and each diagonal element is non-zero, it is called an Identity Matrix. It is denoted by I.

4. What is the order of Square Matrix?

A Matrix that has a number of rows is equal to a number of columns is called Square Matrix. In this matrix, all the elements are arranged in m number of rows and n number of columns . So the order of the matrix is denoted by mxn.

5. Explain a Scalar Matrix?

Scalar Matrix is similar to a square matrix. In the scalar matrix, all off-diagonal elements are equal to zero and all on diagonal elements happen to be equal. In other words, the Scalar Matrix is an identity matrices multiple.

The perimeter is the distance around a shape. A quadrilateral is a polygon that has four sides and four angles. To find the perimeter of a quadrilateral adds the measurements of four sides of it. We have given the most common types of quadrilaterals along with the perimeter of that quadrilateral. Check out the complete article and know how to find the perimeter of the quadrilateral. Some of the examples of a quadrilateral are Parallelogram, Rectangle, Square, Rhombus, and Trapezium, etc.

Also, Read:

• Sum of Angles of a Quadrilateral
• Different Types of Quadrilaterals
• Construct Different Types of Quadrilaterals

Perimeter of Different Types of Quadrilaterals

The sum of the four angles of the quadrilateral is equal to 360°. We have different types of quadrilateral. They are

1. Parallelogram
2. Rectangle
3. Square
4. Rhombus
5. Trapezium

The perimeter of the Quadrilateral is the sum of the distance around the image. That means the perimeter of a quadrilateral is equal to the sum of the four sides of the image or object. That is,

(AB + BD + DC + CA) = Perimeter of the Quadrilateral.

1. Parallelogram

Here, the lengths of the two sides of the parallelogram are equal and the breadths of the two sides of the parallelogram are equal. Opposite sides are equal in a parallelogram.
Perimeter of the Parallelogram = (AB + BD + DC + CA)
= (b + l + b + l).
Perimeter of the Parallelogram = 2(l + b).
Here, ‘l’ represents the length and ‘b’ represents the breadth of the parallelogram.
Area of the parallelogram = Base * height.

2. Rectangle

In a rectangle, both the lengths of the sides are equal and the breadths of the sides of a rectangle are equal. That means, opposite sides of the rectangle are equal.
Perimeter of the rectangle = (AB + BD + DC + CA) = (b + l + b + l) = 2(l + b).
Perimeter of the Rectangle = 2(l + b).
Area of the Rectangle = length * breadth = l *b.
Here, ‘l’ indicates the length of the rectangle and ‘b’ indicates the breadth of the rectangle.

3. Square

In this, a square is also enclosed with the four sides and the lengths of these four sides are equal.
Here, ‘a’ indicates the length of the sides of the square.
Perimeter of the square = (AB + BD + DC + CA) = (a + a + a + a) = 4a.
The perimeter of the square = 4a.
Area of the Square = Side * Side = a * a = a^2.

4. Rhombus

The lengths of the four sides of the rhombus are equal. Here, ‘a’ represents the length of the side.
Perimeter of the Rhombus = (AB + BC + CD + DA) = (a + a + a + a) = 4a.
The perimeter of the Rhombus = 4a.
Area of the Rhombus = (Base * height).

5. Trapezium

Two opposite sides of the trapezium are parallel. Perimeter of the Trapezium = (AB + BC + CD + DA).
AB + BC + CD + DA = (c + b + d + a)cm.
Area of the Trapezium = (a + b) / 2 * h.
Here, a, b, c, d are the sides of the trapezium. And ‘h’ indicates the height of the trapezium.

Perimeter of Quadrilateral Examples

1. Find the Perimeter of the Quadrilateral with the sides 2cm, 10cm, 5cm, and 20cm?

Solution:
The given information is the length of the four sides of the quadrilateral is = 2cm, 10cm, 5cm, 20cm.
The perimeter of the quadrilateral = sum of the length of the four sides of the quadrilateral.
Perimeter of the quadrilateral = (2 + 10 + 5 + 20)cm. = 37cm.

So, the perimeter of the quadrilateral is equal to 37cm.

2. The Perimeter of the quadrilateral is 40cm and the length of the three sides of the quadrilateral is 5cm, 10cm, and 5cm. Find the length of the four sides of the quadrilateral?

Solution:
The given details are the Perimeter of the quadrilateral = 40cm.
Length of the three sides of quadrilateral = 5cm, 10cm, and 5cm.
The perimeter of the quadrilateral = sum of the length of four sides of the quadrilateral.
40cm = (5 + 10 + 5 + x) cm.
40 = 20 + x.
x = 40 – 20 = 20cm.

Finally, the length of the fourth side of the quadrilateral is equal to 20cm.

3. A woman crosses a distance of 24m long while going round a quadrilateral field twice. What will be the cost of fencing the field at the rate of cost $1.20per m?

Solution:
The given information is Women crosses a distance of 24m long = perimeter of its boundary = 24 / 2 = 12m.
Cost of fencing the field for 1m = $1.20.
Then the cost of 12m of fencing the field = 12 * $1.20 = $14.4.

Therefore, the cost of the 12m fencing field is equal to $14.4.

4. One side of the square is 4cm. Find the perimeter of the square?

Solution:
As per the given information, the length of the side of the square = 4cm.
The perimeter of the square = 4a.
Here, a = 4.
By substituting the ‘a’ value in the formulae, we will get
Perimeter of the square = 4 * 4 = 16cm.

So, the perimeter of the square is equal to 16cm.

5. Length of the rectangle is 10cm and the breadth of the rectangle is 5cm. Calculate the Perimeter of the Rectangle?

Solution:
As per the given details, the Length of the rectangle (l) = 10cm.
Breadth of the rectangle (b) = 5cm.
Perimeter of the rectangle = 2(l + b).
By substituting the values in the formulae, we will get like
Perimeter of the rectangle = 2(10 + 5) = 2(15) = 30cm.

Therefore, the perimeter of the rectangle is equal to 30cm.

6. If the area of the rhombus is 20 square units and the height of the rhombus is 6 units, then calculate the base of the rhombus?

Solution:
From the given information, Area of the Rhombus = 20 Sq units.
Height of the Rhombus (h) = 6 units.
Area of the Rhombus = Base * height.
20 = Base * 6.
Base = 20 / 6 = 10 / 3 = 0.3 units.

The base of the Rhombus is equal to 0.3 units.

Frequently Asked Questions

1. What is Quadrilateral?

The quadrilateral is enclosed with four sides. It has four corners, four angles, and four sides. The total angle of the quadrilateral is equal to 360°.

2. What are the types of Quadrilateral?

The types of Quadrilateral are,
1. Square
2. Rectangle
3. Parallelogram
4. Trapezium
5. Rhombus

3. How we can find the perimeter of a quadrilateral?

The area of the quadrilateral is the sum of all sides of the quadrilateral. Yes, by adding all sides of the quadrilateral, we can find the perimeter of the quadrilateral.

Do you want to round numbers to one decimal place? If yes, then stay on this page. Here we will discuss how to correct to one decimal place. Rounding off is a type of estimation. We generally use estimation in everyday life and also in maths, physics subjects. You will also learn rounding decimals to the nearest tenth, rules, and example questions with the solutions in the following sections.

Correct to One Decimal Place – Definition

Correct to one decimal place is also known as round off to the nearest tenths. The rounding decimals technique is used to find the approximate values of a decimal number. Here the decimal numbers are rounded to the one decimal place to make them easier to read, understand instead of having lengthy string decimal places.

Rules for Rounding Decimals to Nearest Tenths or Rounding off to One Decimal Place

To correct the decimals to the nearest tenths, you have to check the below-mentioned rules.

• Rule 1: If the digit in the hundredths place of the number is lesser than 5, then remove the following digits or substitute 0 in place of them.
• Rule 2: If the digit in the hundredths of the number is equal to or greater than 5, then the digit in the tenths place is increased by 1 and the following digits are replaced by 0.

How to Round to One Decimal Place?

Have a look at the detailed step-by-step process on correct to one decimal place in the further sections.

• Identify the number to which you need to round off to the nearest tenths.
• Observe the digit in the hundredths position of the given number.
• If the observed digit is less than 5 i.e 0, 1, 2, 3, 4 then replace the following digits with 0.
• When the digit is more than 5 i.e 5, 6, 7, 8, 9 then add 1 to the digit in the tenths place and remove the following digits.

Also, Read

• Correct to Two Decimal Places
• Rounding Decimals to the Nearest Whole Number
• How To Round off to Nearest 100

Rounding to 1 Decimal Place Examples

Example 1:

Round off the following numbers to one decimal place.

(a) 5.256

(b) 17.89

(c) 146.36

Solution:

(a) The given decimal number is 5.256

We see the digit in the hundredths place is 5 then round it to the nearest tenths which is greater than the given decimal number. Since 5 = 5 then the decimal number is rounded to 5.3.

Therefore, the solution is 5.3

(b) The given decimal number is 17.89

We can identify the digit in the hundredths place is 9 then round it to the nearest hundredths which is greater than the given decimal number. Since 9 > 5 then the decimal number is rounded to 17.80.

Therefore, the solution is 17.8.

(c) The given decimal number is 146.36

We see the digit in the hundredths place is 6 then round it to the nearest tenths which is greater than the given decimal number. Since 6 > 5 then the decimal number is rounded to 146.4.

Therefore, the solution is 146.4.

Example 2:

Round off the numbers to the nearest tenths.

(i) 14.732

(ii) 80.75

(iii) 16.54

Solution:

(i) The given decimal number is 14.732

We see the digit in the hundredths place is 3 then round it to the nearest tenths which is smaller than the given decimal number. Since 3 < 5 then the decimal number is rounded to 14.7.

(ii) The given decimal number is 80.75

The digit in the hundredths place is 5. ie equal to 5

Increase the digit in the tenths place by 1 and replace the following digits by 0.

The rounded number is 80.8.

(iii) The given decimal number is 16.54

We see the digit in the hundredths place is 4 then round it to the nearest tenths which is smaller than the given decimal number. Since 4 < 5 then the decimal number is rounded to 16.5.

Example 3:

Correct the following to one decimal place.

(i) 185.04

(ii) 77.49

(iii) 111.12

Solution:

(i) The given decimal number is 185.04

We can identify the digit in the hundredths place is 4 then round it to the nearest hundredths which is smaller than the given decimal number. Since 4 < 5 then the decimal number is rounded to 185.0.

Therefore, the solution is 185.0

(ii) The given decimal number is 77.49

We can identify the digit in the hundredths place is 9 then round it to the nearest hundredths which is greater than the given decimal number. Since 9 > 5 then the decimal number is rounded to 77.5.

Therefore, the solution is 77.5

(iii) The given decimal number is 111.12

We can identify the digit in the hundredths place is 2 then round it to the nearest hundredths which is smaller than the given decimal number. Since 2 < 5 then the decimal number is rounded to 111.1.

Therefore, the solution is 111.1.

The ratio indicates how many times a number contains another. Ratios are represented as fractions i.e a: b. The comparison or the simplified form of two quantities of the same kind is called the ratio. Interested students who want to know more about the concept of ratios can read this complete page. Here, we will discuss the basic concept of the ratio, key points, definition, and example questions.

Ratios – Definition

Ratios are an important concept in mathematics. In certain cases, the comparison of two quantities using the division method is difficult. So, at that time, we use ratio. The ratio gives us how many times one quantity is equal to another quantity.

Simply, a ratio is a number that is used to express one quantity as a fraction of another one. Two numbers in a ratio can be expressed only when they have the same unit. The sign of ratio is ‘:’. The real-life examples of a ratio are the rate of speed (distance/time), price of a material (rupees/meter, and others.

Key Points to Remember regarding Ratios

The key points to remember regarding the ratios are as follows:

• A ratio must exist between two quantities of the same kind
• To compare two things, their units should be the same
• There should be significant order of terms
• The comparison of two ratios can be performed, if the ratios are equivalent like fractions

Ratio Formulas

1. If we have two entities and you need to find the ratio of these two then the formula is defined as a: b or a/b.

Where a, b will be the entities

a is called the first term or antecedent and b is called the second term or consequent

2. If two ratios are equal, then they are proportional

a : b = c : d

d is called the fourth proportional to a, b, c

c is called third proportion to a, b

The mean proportion between a and b is √(ab)

3. If (a : b) > (c : d) = (a/b > c/d)

The compounded ratio of the ratios (a : b), (c : d), (e : f) is (ace : bdf)

4. If a: b is a ratio, then

a²: b² is a duplicate ratio

√a: √b is a sub-duplicate ratio

a³: b³ is a triplicate ratio

5. Ratio and Proportion Tricks

If a/b = x/y, then ay = bx or a/x = b/y or b/a = y/x

If a/b = x/y, then \(\frac { a + b }{ b } =\frac { x + y }{ y } \) or \(\frac { a – b }{ b } =\frac { x – y }{ y } \)

If a/b = x/y, then \(\frac { a + b }{ a – b } =\frac { x + y }{ x – y } \) this is componendo dividendo rule

Also, Read

• What is Ratio and Proportion?
• Practice Test on Ratio and Proportion
• Worked out Problems on Ratio and Proportion

Solved Examples on Ratios

Example 1:

If x : y = 4 : 7, then find (4x – y) : (2x + 3y).

Solution:

Given ratio is x : y = 4 : 7

x = 4k, y = 7k

(4x – y) : (2x + 3y) = \(\frac { (4x – y) }{ (2x + 3y) } \) = \(\frac { (4 • 4k  – 7k) }{ (2 • 4k + 3 • 7k) } \)

= \(\frac { (16k  – 7k) }{ (8k + 21k) } \) = \(\frac { 9k }{ 29k } \)

= \(\frac { 9 }{ 29 } \)

= 9 : 29

Therefore, (4x – y) : (2x + 3y) = 9 : 29.

Example 2:

If a : b = 4 : 5, b : c = 15 : 8 then find a : c.

Solution:

Given that,

a : b = 4 : 5, b : c = 15 : 8

a : b = 4 : 5 = \(\frac { 4 }{ 5 } \) —– (i)

b : c = 15 : 8 = \(\frac { 15 }{ 8 } \) —– (ii)

By multiplying (i) and (ii), we get

\(\frac { a }{ b } \) x \(\frac { b }{ c } \) = \(\frac { 4 }{ 5 } \) x \(\frac { 15 }{ 8 } \)

\(\frac { a }{ c } \) = \(\frac { 3 }{ 2 } \)

Therefore, a : c = 3 : 2

Example 3:

If a quantity is divided in the ratio of 5: 7, the larger part is 84. Find the quantity.

Solution:

Given that,

A quantity is divided in the ratio of 5: 7

Let the quantity be x

Then the two quantities are \(\frac { 5x }{ 5 + 7 } \), \(\frac { 7x }{ 5 + 7 } \)

The larger part is 84

So, \(\frac { 7x }{ 5 + 7 } \) = 84

\(\frac { 7x }{ 12 } \) = 84

7x = 84 • 12

7x = 1008

x = \(\frac { 1008 }{ 7 } \)

x = 144

Therefore, the quantity is 144.

Example 4:

If (3a + 5b) : (7a – 4b) = 7 : 4 then find the ratio a : b.

Solution:

Given that,

(3a + 5b) : (7a – 4b) = 7 : 4

\(\frac { 3a + 5b }{ 7a – 4b } \) = \(\frac { 7 }{ 4 } \)

4(3a + 5b) = 7(7a – 4b)

12a + 20b = 49a – 28 b

20b + 28b = 49a – 12a

48b = 37a

\(\frac { 48 }{ 37 } \) = \(\frac { a }{ b } \)

So, a : b = 48 : 37

Frequently Asked Questions on Ratios

1. What are the different types of ratios?

The different types of ratios are compounded ratio, duplicate ratio, triplicate ratio, subtriplicate ratio, subduplicate ratio, the ratio of equalities, reciprocal ratio, the ratio of inequalities, the ratio of greater inequalities, and the ratio of lesser inequalities.

2. What are the 3 ways of writing ratios?

The three most used ways to write a ratio are given here. The first one is fraction 2/5. The second method is using a word to i.e 2 to 5. Finally, the third one is using the ratio colon between two numbers, 2: 5.

3. Define ratio with an example?

The ratio is a mathematical expression represented in the form of a: b, where a and b are two integers. It can also be expressed as a fraction. It is used to compare things or quantities. The example is 3: 4 = 3/4.

4. Write the differences between ratio and proportion?

The ratio is helpful to compare two things of the same unit whereas proportion is used to express the relation between two ratios. The ratio is represented using a colon: or slash / and proportion is represented using a double colon:: or equal to symbol =. The keyword to identify a ratio is “to every” and the proportion is “out of”.

Are you searching for a tool that computes the addition of two binary numbers using 1’s complement method? If yes, then you have reached the correct place. Here we are giving the detailed steps on how binary addition of two numbers with 1s complement. You can also get Binary Addition Using 1s Complement definition, how to get 1s complement of a binary number, and example questions to improve the levels of understanding.

What is Binary Addition?

A binary number is a number with the base 2. Binary addition is one of the basic arithmetic operations. The binary system has only two digits 0 and 1. The binary code uses the digits 0’s and 1’s to make certain processes turn on or off. The process of the binary addition operation is very familiar to the decimal system by adjusting to the base 2.

Before attempting the binary addition, you must have complete knowledge of how the place works in the binary number system. Almost all modern digital computers and electronic circuits perform the binary operation by representing each bit as a voltage signal. The binary bit 0 means OFF state, 1 means ON state.

1’s Complement of a Binary Number

We have a simple algorithm to convert a binary number into 1’s complement. To get 1’s complement of a binary number, invert the given binary number. You can also implement a logic circuit using only NOT gate for each bit of binary number input.

Example:

Calculate the 1’s complement of a binary number 10101110

Invert each bit of a given binary number

So, 1’s complement of 10101110 is 01010001.

Also, Read

• Binary Addition using 2’S Complement
• Binary Subtraction
• Hexadecimal Addition and Subtraction

Binary Addition Using 1’s Complement

Binary addition means simply performing an addition operation between two binary numbers. You can add two binary numbers digit by digit just like decimal numbers. The rules for adding two binary digits is 0 + 0 = 0, 1 + 0 = 1, 0 + 1 = 1, 1 + 1 = 10(1 is carry). At first, convert the given numbers to the 1’s complement and add those numbers using thee rules. Also, have a look at the detailed process on how to add binary numbers using 1’s complement in the following section.

How to do Binary Addition Using 1s Complement?

The process of adding binary numbers purely depends on their sign and magnitude. Here are the different cases.

Case I: Adding a positive and a negative number. If the positive number has a greater magnitude

Here, the addition of numbers is performed after taking 1’s complement of the negative number, and at the end round carry of the sum is added to the LSB (least significant bit).

Example: + 1101 – 1011

+ 1101 ⇒ 0 1 1 0 1

– 1011 ⇒ 1 0 1 0 0 (1’s complement)

⇒ 0 0 0 0 1 + 1 = 0 0 0 1 0

Hence the sum is +0010.

Case II: If the negative number has a greater magnitude

Here the addition is done in the same way as in case I but there will be non-end-around carry. The sum is obtained by taking the 1’s complement of the magnitude bits of the result and the sum is negative.

Example: 1011 – 1101

+ 1 0 1 1 ⇒ 0 1 0 1 1

– 1 1 0 1 ⇒ 1 0 0 1 0 (1’s complement)

⇒ 1 1 1 0 1

The sum is 1’s complement of 1101

Hence the sum is – 0010.

Case III: If two binary numbers are negative.

To add two negative binary numbers, 1’s complements of both the numbers are taken later addition is performed. In this case, an end-around carry will always appear. This along with a carry from the MSB will generate a 1 in the sign bit. 1’s complement of the magnitude bits of the result of an addition will give the final sum.

Example: -0110 and -0111

– 0 1 1 0 ⇒ 1 1 0 0 1 (1’s complement)

– 0 1 1 1 ⇒ 1 1 0 0 0 (1’s complement)

⇒ 1 0 0 0 1 + 1 = 1 0 0 1 0

1’s complement of 0010 is 1101 and the sign bit is 1

Hence the sum is -1101.

Binary Addition Using 1s Complement Examples

Example 1:

Calculate the sum of 0100, -1000 using the 1’s complement.

Solution:

The given binary numbers are 0100, -1000

1’s complement of -1000 is 10111

0 0 1 0 0 + 1 0 1 1 1 = 1 1 0 1 1

1’s complement of 1011 is 0100

Hence the sum is -0100.

Example 2:

Find the sum of 10000, -00111 using the 1’s complement.

Solution:

The given binary numbers are 10000, -00111

Find the 1’s complement of the negative number i.e 00111

1’s complement of 000111 = 111000

010000 + 111000 = 1 0 0 1 0 0 0

0 0 1 0 0 0 + 1 = 0 0 1 0 0 1

Hence, the sum is 001001.

Example 3:

Find the sum of -0100, -0010 using the 1’s complement method.

Solution:

The given numbers are -0100, -0010

Find the 1’s complement of the negative numbers

1’s complement of 0100 is 1011

1’s complement of 0010 is 1101

1 0 1 1 + 1 1 0 1 = 1 1 0 0 0

1 1 0 0 0 + 1 = 1 1 0 0 1

1’s complement of 1 0 01 is 0 1 1 0 and 1 is the sign bit

Hence, the sum is -0110

Example 4:

Find the sum of 1000 and -0101 using the 1’s complement.

Solution:

The given binary numbers are 1000 and -0101.

Find the 1’s complement of the negative number

1’s complement of 0101 is 11010.

0 1 0 0 0 + 1 1 0 1 0 = 1 0 0 0 1 0

1 0 0 0 1 0 + 1 = 1 0 0 0 1 1

Hence the sum is 00011.

FAQ’s on Binary Addition using 1’s Complement

1. How to find 1’s complement of a binary number?

To get the 1’s complement of binary numbers, just invert the number. Inversion means placing 1’s in place of 0’s and 0’s in place of 1’s. Otherwise, you can also use NOT logic gate to find the 1’s complement.

2. How to add two negative binary numbers using the 1’s complement?

For adding two negative binary numbers with the 1’s complement, just find the 1’s complement of both numbers. Add those 1’s complement numbers and add 1 to the obtained result. Then you will get 1 in place of the sign bit. Get the 1’s complement of the obtained sum to get the final result.

3. What’s the purpose of using complements in binary number operations?

Compared to other systems for representing the signed numbers 1’s complement, 2’s complement has the advantage that the fundamental arithmetic operations are identical to those for unsigned binary numbers.

The equation of a straight line represents each and every point on that line. Let us consider the straight line created by passing through the two points such as (a1, b1) and (a2, b2). There are no curves present in the straight line. The basic equation for the straight line in Two-point for is ax + by + c = 0. Here, a, b, c are constants, and x and y are variables.

How to find the Equation of a Straight Line Passing Through Two Points?

The below diagram shows the straight line in two-point form. In this, (a1, b1) is the starting point of the straight line, and (a2, b2) is the ending point of the straight line.

The equation of the straight line which is passing through the two points A(a1, b1) and B(a2, b2) is b – b1 = (b2 – b1) / (a2 – a1) * (a – a1). P(a, b)is the joining point of A and B.
The straight line is passing through the two points A and B. Then the slope of the line AB = (b1 – b2) / (a1- a2).———( 1 ).
Now, the straight line is passing through the two – points A and P. Then the slope of the line AP = (b – b1) / (a – a1). ——- ( 2 ).
To find out the equation of a straight line with two point form, we need to equate the two equations. That is,
Slope of the line AP = Slope of the line AB.
(b – b1) / (a – a1) = (b1 – b2) / (a1 – a2).
(b – b1) = (b1 – b2) / (a1 – a2) * (a – a1).
Equation for the straight line of two – point form is equal to (b – b1) = (b1 – b2) / (a1 – a2) * (a – a1).
Let us consider the slope (b1 – b2) / (a1 – a2) as ‘m’. substitute the value in the above equation. Then straight line equation becomes as
(b – b1) = m(a – a1).

Steps to Solve Equation of the Straight Line Problems

Follow the three steps to find the Equation of the Straight Line. They are

1. Find the slope of the line.
2. Put the slope and one point into the “Point-Slope Formula”.
3. Simplify the equation.

Also, See:

• Angle Between Two Straight Lines

Two – Point Form Examples with Solutions

1. Find the Equation of the straight line passing through the points (1, 2), and (3, -2)?

Solution:
The given details are (a1, b1) = (1, 2) and (a2, b2) = (3, -2).
The equation for the straight line is (b – b1) = (b1 – b2) / (a1 – a2) * (a – a1).
Substitute the values in the above equation, then we will get
(b – 2) = (2 – (-2)) / (1 – 3) * (a – 1).
(b – 2) = (2 + 2) / (-2) * (a – 1).
(b – 2) = 4 / (-2) * (a – 1).
(b – 2) = -2 * ( a – 1).
b – 2 = -2a + 1.
2a + b – 2 -1 = 0.
2a + b – 3 = 0.

Finally the straight line equation is 2a + b – 3 = 0.

2. Find the equation of the straight line joining the points (-2, 4) and (5, 6)?

Solution:
As per the given information, the straight line is created by joining the two – points. They are (a1, b1) = (-2, 4) and (a2, b2) = (5, 6).
Equation for the straight line of joining two points is (b – b1) = (b1 – b2) / (a1 – a2) * (a – a1).
Substitute the values in the above equation, then we will get like
(b – 4) = (4 – 6) / (-2 – 5) * (a – (-2)).
(b – 4) = (- 2 / – 7) *(a + 2).
(b –4) (7) = 2 * (a + 2).
7b – 28 = 2a + 4.
-2a + 7b – 28 – 4 = 0.
-2a + 7b -32 = 0.

Therefore, the final equation for the straight line is -2a +7b – 32 = 0.

3. Find the straight-line equation with the help of two points (6, 2) and (8, 4)?

Solution:
The given information is the straight line is crossing the two points. They are (a1, b1) = (6, 2) and (a2, b2) = (8, 4).
The equation for the straight line in Two – point form is (b – b1) = (b1 – b2) / (a1 – a2) * (a – a1).
Substitute the values in the above equation, we will get
(b – 2) = (2 – 4) / ( 6 – 8) * ( a – 6).
(b – 2) = ( – 2) / ( – 2) * (a – 6).
(b – 2) = (a – 6).
-a + b – 2 + 6 = 0.
-a + b + 4 = 0.
a – b – 4 = 0.

Finally, the equation for the straight line in two – points is equal to a – b – 4 = 0.

4. Find the equation of a straight line that is passing through points A(6, 6) and B(10, 4)?

Solution:
As per the given information, the straight line is passing through the two points. They are
(a1, b1) = (6, 6) and (a2, b2) = (10, 4).
The basic equation for the straight line in two – point form is (b – b1) = (b1 – b2) / (a1 – a2) * (a – a1).
Substitute the above values in the equation. Then we will get like
(b – 6) = (6 – 4) / (6 – 10) * (a – 6).
(b – 6) = 2 / (-4) * (a – 6).
(b – 6) = 1 / (-2) * (a – 6).
-2 ( b – 6) = a – 6.
-2b + 12 = a – 6.
-a – 2b + 12 + 6 = 0.
a + 2b – 18 = 0.

Finally the equation for the straight line is a + 2b – 18 = 0.

5. Find the values of a for which the points A(a, -2), B(4, 2) and C(8, 10) are collinear?

Solution:
As per the given information, the given points A(a, -2), B(4, 2), and C(8, 10) are collinear.
(a, b) = (a, -2), (a1, b1) = (4, 2), and (a2, b2) = (8, 10)
So, the slope of the AB = slope of the BC.
(b1 – b) / (a1 – a) = (b2 – b1) / (a2 – a1).
Substitute the values in the above equation. Then we will get
(2 – (-2)) / ( 4 – a) = (10 – 2) / (8 – 4).
(2 + 2) / (4 – a) = 8 / 4.
4 / (4 – a) = 2.
4 = 2(4 – a).
4 – a = 2.
4 – 2 = a.
a = 2.

Finally, a is equal to 2.

Decimal numbers are the part of numbers that have two parts. The decimal numbers are in the standard form representing integer and non-integer numbers. Generally, the decimal points are written in a fraction which consists of 10, 100, 1000 in the denominators. The numbers that are expressed in the decimal form are called decimal numbers. Let us check the complete concept on decimal in the below article.

Also, Read:

• Correct to Two Decimal Places
• Converting Decimals to Fractions
• Conversion of a Decimal Fraction into a Fractional Number

Decimals – Definition

A decimal number is a number that has two parts. One part has a whole number and the other part is a fractional part. Both parts are separated by decimal points. If 2.48 is a decimal number, then 2 is the whole number and 48 is the fractional part. “.” is the decimal point.

• The digits having in the whole number part are called ones, then tens, then hundreds, then thousands, and so on.
• The places after the decimal point begin with tenths, then hundredths, then thousandths, and so on………

Examples:

(i) In the decimal number 47.25, the whole number part is 47 and the decimal part is .25
(ii) In the decimal number 89.063, the whole number part is 89 and the decimal part is .063
(iii) Take the decimal number 11.056 where the whole number part is 11 and the decimal part is .056

Types of Decimal Numbers

Decimal numbers are classifieds into different types. They are given with definitions and examples explained in detail.

Recurring Decimal Numbers – Recurring Decimal Numbers are Repeating or Non-Terminating Decimals.
Examples of Recurring Decimal Numbers are 2.123123 (Finite) and 4.252525252525… (Infinite)

Non-Recurring Decimal Numbers – Non-Recurring Decimal Numbers are Non Repeating or Terminating Decimals.
Examples of Non-Recurring Decimal Numbers are 5.14812 (Finite) and 2.5428454845…. (Infinite)

Decimal Fraction – Decimal Fraction is the fraction that consists of the denominator as powers of ten.
Examples are 72.66 = 7266/100 and 43.536 = 43536/1000.

Converting a Decimal Number into Decimal Fraction
To convert the Decimal Number into Decimal Fraction, place the 1 in the denominator and remove the decimal point from the given number. The 1 is followed by the number of zeros that are equal to the number of digits given after the decimal point.

Examples:
1. 47.59
The given decimal number is 47.59
47.59 = 4759/100
4 represents the power of 101 that is the tenths position.
7 represents the power of 100 that is the unit’s position.
5 represents the power of 10-1 that is the one-tenth position.
9 represents the power of 10-2 that is the one-hundredths position.
So that is how each digit is represented by a particular power of 10 in the decimal number.
2. 61.27
The given decimal number is 61.27
61.27 = 6127/100
6 represents the power of 101 that is the tenths position.
1 represents the power of 100 that is the unit’s position.
2 represents the power of 10-1 that is the one-tenth position.
7 represents the power of 10-2 that is the one-hundredths position.
So that is how each digit is represented by a particular power of 10 in the decimal number.

Place Value in Decimals

Place value of a number in decimals is the position of every digit that helps to find its value. The position of each digit that before and after the decimal point is different. Check out the below examples to know each digit’s place value.

Examples:
Let us take a number 296.

• The position of “2” is in One’s place, which means 2 ones (i.e. 2).
• The position of “9” is in the Ten’s place, which means 9 tens (i.e. ninety).
• The position of “6” is in the Hundred’s place, which means 6 hundred.
• As we go left, each position becomes ten times greater.
• Hence, we read it as “two hundred ninety-six”.

As each digit, we move to the left side the value becomes 10 times greater than the previous value.

• The tens place digit is 10 times bigger than Ones.
• The hundreds place digit is 10 times bigger than Tens.

If we consider a decimal number, the digits after the decimal points will become 10 times smaller than other digits. The digits present on the left side of the decimal are multiplied with the positive powers of ten in increasing order from right to left. The digits present in the right of the decimal point are multiplied with the negative powers of 10 in increasing order from left to right.

Example:
1. 61.28
The decimal expansion of the given number 61.28 is
[(6 * 10) + (1 * 1)] + [(2 * 0.1) + (5 * 0.01)]

Properties of Decimals

We have given the main properties of the decimal numbers below those are under multiplication and division operations. Check out all the properties of decimals given below.

• When two decimal numbers are multiplied with each other, then the result will be a decimal number.
• When a decimal number and a whole number are multiplied with each other, then the result will be a decimal number.
• If any decimal fraction is multiplied by 1, the product remains the same decimal fraction by itself.
• If any decimal fraction is multiplied by 0, then the product becomes 0.
• When a decimal number divided by 1, then the quotient must be a decimal number.
• Also, when a decimal number is divided by the same number, then the quotient becomes 1.
• If in case, 0 divided by any decimal number, the quotient becomes 0.
• The division of a decimal number by 0 is not applicable and possible as the reciprocal of 0 does not exist.

Arithmetic Operations on Decimals

We can perform addition, subtraction, multiplication, and division operations on Decimals easily. Check out the below concepts to understand different Arithmetic Operations on Decimals.

Addition Operation on Decimals
When you add two decimal numbers, line up the decimal points of the given numbers and add them. If you don’t see a decimal point, then that is only a whole number.

Subtraction Operation on Decimals
Subtraction Operation on Decimals is also similar to the Addition Operation on Decimals. You need to line up the decimal point of the given numbers and subtract the values.

Multiplication Operation on Decimals
Multiplication Operation on Decimals is like integers as if the decimal point not present. Firstly, find out the product and count the number after the decimal point in the given numbers. The count will let you know how many numbers present after the decimal point in the result.

Division Operation on Decimals
The Division Operation on Decimals is simply dividing the given two decimal numbers. Move the decimal points to make them whole numbers. Then, perform the division operation like normal integers.

Decimal to Fraction Conversion

We consider the digits after the decimal point as the tenths, hundredths, thousandths, and so on. Write down the decimal numbers in the expanded form and simplify the values.
Example:
Let us consider a decimal number 5.21
Conver it into a fraction number.
The expanded form of 5.21 is 521 x (1/100) = 521/100.

Fraction to Decimal Conversion

To convert the fraction number into a decimal number, divide the numerator by denominator.
Example: 9/7 is a fraction. If it is divided, we get 1.285714

Decimal Problems with Solutions

Example 1:

Convert 6/10 in decimal form?
Solution:
Given fraction number is 6/10.
To convert fraction to decimal, divide 6 by 10, we get the decimal form.
Thus, 6/10 = 0.6
Hence, the decimal form of 6/10 is 0.6.

Example 2:

Express 2.36 in fraction form?
Solution:
The given decimal number is 2.36
The expanded form of 2.36 is
= 236 x (1/100)
= 236 /100
= 118/50
= 59/25
Hence, the equivalent fraction for 2.36 is 59/25.

Frequently Asked Questions on Decimals

1. What is meant by Decimal?

A decimal is a number that mainly has two parts named as a whole number part and a fractional part separated by a decimal point.

2. What are the different types of decimals?

There are two types of decimals considered. They are

• Terminating decimals (or) Non-recurring decimals
• Non-terminating decimals (or) Recurring decimals

3. Write the expanded form of 85.3?

The expanded form of 85.3 is 80 + 5 + (3/10)

4. How to convert fractions to decimals?

Factions to decimals are converted by dividing the numerator by the denominator value.

A polygon is a two-dimensional figure that consists of a finite number of sides. The sides of a polygon are connected by line segments. The end connected by the line segments is called the vertex. There are Different Types of Polygons available in geometry. Poly means many and gon means an angle. The area and perimeter of the polygon completely depend on their shape. Any polygon can be classified depends on its sides and vertices. If a polygon has four sides and four vertices, then that polygon is named a quadrilateral.

Also, Read:

• Convex and Concave Polygons
• Regular and Irregular Polygon

Types of Polygons and their Properties

Based on the sides and vertices, the polygons are classified into different types. Check out the below polygons and their properties along with the examples and images.

• Regular Polygons
• Irregular Polygons
• Concave Polygons
• Convex Polygons
• Trigons
• Quadrilateral Polygons
• Pentagon Polygons
• Hexagon Polygons
• Equilateral Polygons
• Equiangular Polygons

Regular Polygon

A Polygon is said to be a Regular Polygon when all of its sides are equal. Also, a regular polygon has all the interior angles are the same. For example, a regular hexagon consists of equal six sides, and also its interior angles give a total of 120 degrees.

Examples:

• A square consists of all its sides equal to 4cm, and also all the angles are at 90°.
• A regular pentagon that has 5 equal sides. All the interior angles measure 108 degrees.
• An equilateral triangle has all three sides equal to 8cm and angles measure to 60°.

Irregular Polygon

A Polygon is said to be an irregular polygon when all of its sides are not equal. They have irregular shapes. Also, the angles of irregular polygons are not equal.

Examples:

• A triangle with unequal sides.
• A quadrilateral with unequal sides.

Convex Polygon

A Polygon is said to be a convex polygon when the measure of the interior angle is less than 180 degrees. The corners of a convex polygon are always outwards. Convex Polygon is completely opposite to the concave polygon.

Example:

An irregular hexagon that vertices are completely outwards.

Concave Polygon

A Polygon is said to be a concave polygon when there are at least one angle measures more than 180 degrees. The corners of a concave polygon present inwards and also outwards.

Trigons

A Polygon is said to be a trigon that has three sides. Trigons are also known as triangles. The triangles are classifieds into different categories. They are
Scalene Triangle: It has all sides that are unequal.
Isosceles Triangle: It has two sides that are equal.
Equilateral Triangle: All sides are equal in length and also all angles are 60 degrees.

Quadrilateral Polygon

A Polygon is said to be a Quadrilateral Polygon when it has four sides. Examples of Quadrilateral Polygon are square, rectangle, rhombus, and parallelogram.

Pentagon Polygon

A Polygon is said to be a Pentagon Polygon when it has five sides. If all the sides of a pentagon polygon are equal, then it is called a regular pentagon. If not it is called an irregular pentagon.

Hexagons

A polygon is called a hexagon when that has six sides and six vertices. If all the six sides of a hexagon are equal, then it is called a regular hexagon. Also, in a regular hexagon, all the interior and exterior angles are equal.

Equilateral Polygons

The polygons are called equilateral polygons when it has all sides are equal. An equilateral triangle, a square, etc., are examples of Equilateral Polygons.

Equiangular Polygons

The polygons are called Equiangular Polygons when all of their interior angles are equal. An example is a rectangle.

Types of Polygons with Sides 3-20

Below is the Classification of Polygons according to the number of sides and the angle measure. They are as follows

Name of the Polygons	Sides	Vertices	Angle
Triangle (also called Trigon)	3	3	60°
Quadrilateral (also called Tetragon)	4	4	90°
Pentagon	5	5	108°
Hexagon	6	6	120°
Heptagon	7	7	128.571°
Octagon	8	8	135°
Nonagon (also called Enneagon)	9	9	140°
Decagon	10	10	144°
Hendecagon	11	11	147.27
Dodecagon	12	12	150
Tridecagon or triskaidecagon	13	13	152.3
Tetradecagon or tetrakaidecago	14	14	154.28
Pendedecagon	15	15	156
Hexdecagon	16	16	157.5
Heptdecagon	17	17	158.82
Octdecagon	18	18	160
Enneadecagon	19	19	161.05
Icosagon	20	20	162
n-gon	n	n	(n-2)× 180° / n

FAQs on Different Types of Polygons?

1. What is a Polygon?

A polygon is a two-dimensional figure that consists of a finite number of sides.

2. How many different types of Polygons are there?

There are two different types of Polygons namely Regular and Irregular. However, depending on the sides and vertices they are furthermore classified as Concave Polygons, Convex Polygons, Trigons, Quadrilateral Polygons, Pentagon Polygons, Hexagon Polygons, Equilateral Polygons, Equiangular Polygons, etc.

3. What is a 9 sided shape called?

9 Sided Shape is Called an Enneagon or Nonagon.

Worksheet on Decimal Word Problems will help the students to explore their knowledge of decimal word problems. Solve all the problems to learn the depth concept of Decimal Word Problems. Know the definition, properties, different operations performed on decimals by visiting our website. We have given the complete decimal concepts along with examples. Check out the Decimal Word Problems Worksheet and know the various strategies to solve problems in an easy way.

Also, Read:

• Decimals
• Converting Decimals to Fractions

What are Decimals?

The decimal is a number that has the whole number and the fractional part separated by a decimal point. The point between the whole number and fractions part is known as the decimal point. For example, 24.9 is the decimal number where 24 is a whole number and 9 is the fractional part after the decimal. We have included addition, subtraction, multiplication, and division decimal word problems below.

Word Problems on Decimals

Have a look at all the problems given below and get a grip on all types of problems on decimals.

1. Anshika scored 521 marks out of 600 in the final examination. How many marks did she lose?

Solution:

Given that Anshika scored 521 marks out of 600 in the final examination.
So, the total marks are 600.
She scored 521 marks.
To get the marks she loses in an examination, we have to subtract 521 marks from 600.
Now, subtract 521 from 600.
600 – 521 = 79 marks.

Anshika lost 79 marks in the final examination.

---

2. Arun had 0.24 liters of cold drink. Kiran had 0.68 liters more cold drink. How much cold drink did they have together?

Solution:

Given that Arun had 0.87 liters of cold drink. Kiran had 0.92 liters more cold drink.
Arun = 0.24 litres of cold drink
Kiran = 0.68 liters more cold drink
To find the cold drink they have together we have to add both cold drink liters.
So, add 0.24 liters of cold drink and 0.68 liters more cold drink
0.24 liters of cold drink + 0.68 liters more cold drink = 0.92 liters of cold drink.

They have 0.92 liters of cold drink together.

---

3. The weight of a baby lion was 169.24 kg. After two years, his weight increased by 107.64 kg. Find the weight of the lion after two years?

Solution:

Given that the weight of a baby lion was 169.24 kg. After two years, his weight increased by 107.64 kg.
The lion’s present age is 169.24 kg.
After two years, his weight increased by 107.64 kg.
Now, add both weights to get the lion’s age after two years.
So, add 169.24 kg and 107.64 kg
169.24 kg + 107.64 kg = 276.88 kg

After two years, the lion weight is 276.88 kg.

---

4. Ganesh had a rope of 54.28 m. He cut the rope into two pieces. If the length of one piece was 13.26 m, what was the length of the other piece?

Solution:

Given that Ganesh had a rope of 54.28 m. He cut the rope into two pieces.
The total length of the rope is 54.28 m.
The rope is cut into two pieces.
If the length of one piece was 13.26 m, let the other piece’s length is x.
Add two pieces 13.26 m and x m.
13.26 m + x m = 54.28 m
x = 54.28 m – 13.26 m
x = 41.02 m

The length of the other piece is 41.02 m.

---

5. Each side of a regular polygon is 21.7 m and its perimeter is 108.5 m. Find the number of sides of the polygon?

Solution:

Given that each side of a regular polygon is 21.7 m and its perimeter is 108.5 m.
The perimeter of a polygon is the sum of all sides of a polygon.
To find the number of sides of the polygon, divide the perimeter with each side of a regular polygon is 21.7 m.
Divide 108.5 m with 21.7 m
108.5 m/21.7 m = 5.

The number of sides of the polygon is 5.

---

6. Fariha took 2.4 minutes to complete the race and Anil took 2.1 minutes to complete the race. Who won the race?

Solution:

Given that Fariha took 2.4 minutes to complete the race and Anil took 2.1 minutes to complete the race.
To find the winner of the race, we need to compare the time taken by each of them. The one who traveled in less time is the winner of the race.
Fariha took 2.4 minutes.
Anil took 2.1 minutes.
2.1 minutes is less time compared to 2.4 minutes.
Therefore, Anil took less time to complete the race.

Anil won the race.

---

7. The annual rainfall received by Arunachal Pradesh is 261.5 cm and that by Assam is 297.4 cm. Who received less rainfall?

Solution:

Given that the annual rainfall received by Arunachal Pradesh is 261.5 cm and that by Assam is 297.4 cm.
To find the less rainfall received, we need to compare the rainfall in two states. The place where the less cm rainfall occurred will be the answer.
Arunachal Pradesh = 261.5 cm rainfall
Assam = 297.4 cm rainfall
261.5 cm rainfall is less time compared to 297.4 cm rainfall.
Therefore, Arunachal Pradesh received less rainfall.

The answer is Arunachal Pradesh.

---

8. Rishu’s height is 134.51 cm. She stands on a tool of height 9.40 cm. What is the combined height now?

Solution:

Given that Rishu’s height is 134.51 cm. She stands on a tool of height 9.40 cm.
To find the combined height, add both given heights.
Add 134.51 cm and 9.40 cm
134.51 cm + 9.40 cm = 143.91 cm.

The combined height is 143.91 cm.

---

9. The milkman delivers 6.03 liter of milk to a house in the morning and 3.230 liters in the evening. What is the total quantity of milk delivered by the milkman?

Solution:

Given that the milkman delivers 6.03 liter of milk to a house in the morning and 3.230 liters in the evening.
To get the total quantity of milk delivered by the milkman, combine the milk he delivers in the morning and also in the evening.
Milkman delivers 6.03 liter of milk in the morning
Milkman delivers 3.230 liters of milk in the evening
Add 6.03 liter of milk and 3.230 liters of milk.
6.03 liter of milk + 3.230 liters of milk = 9.26 liters of milk

The total quantity of milk delivered by the milkman = 9.26 liters of milk.

---

10. Rebecca‘s kite is flying at a height of 28.3 m and Shelly’s at a height of 32.6 m from the ground. Whose kite is flying high and by how much?

Solution:

Given that Rebecca‘s kite is flying at a height of 28.3 m and Shelly’s at a height of 32.6 m from the ground.
To find whose kite is flying high, compare the two heights. The largest height of the kite considered to be flying high.
Rebecca‘s kite is flying at a height of 28.3 m
Shelly’s at a height of 32.6 m
32.6 m is greater than the 28.3 m.
Shelly’s kite is flying high.
To find how much the kite is flying high, subtract the heights.
Subtract 28.3 m from 32.6 m.
32.6 m – 28.3 m = 4.3 m

Shelly’s kite is flying high of 4.3 m than Rebecca‘s kite.

---

11. A bike travels 39.2 km in 7 hours. How much distance will it travel in 1 hour?

Solution:

Given that a bike travels 39.2 km in 7 hours.
Now, to find the distance it will travel in 1 hour, we have to divide the 39.2 km by 7 hours.
Divide 39.2 km by 7 hours.
39.2 km/7 hours = 5.6km/hr

The bike travels 5.6km in one hour.

---

12. Ron jogged 3.3 km, Mike jogged 4.8 times more distance than Ron. Find the distance covered by Mike?

Solution:

Given that Ron jogged 3.3 km, Mike jogged 4.8 times more distance than Ron.
The distance covered by Mike, multiply the Ron jogged km with the Mike jogged 4.8 times more distance than Ron.
The distance covered by Ron 3.3 kilometers.
Mike jogged 4.8 times more distance than Ron.
Multiply 3.3 kilometers and 4.8 kilometers.
The distance jogged is 3.3 kilometers × 4.8 kilometers = 15.84 kilometers.

The distance covered by mike is 15.84 kilometers.

---

13. The daily consumption of milk in a house is 4.36 liters. How much milk will be consumed in 30 days?

Solution:

Given that the daily consumption of milk in a house is 4.36 liters.
To find the milk that will be consumed in 30 days, we need to multiply 30 with 4.36 liters.
Multiply 4.36 liters with 30.
4.36 liters × 30 = 130.8 liters

130.8 liters of milk will be consumed in 30 days.

---

14. A tin contains 13.4 liters of oil. How many such tin contains 80.4 liters of oil?

Solution:

Given that a tin contains 13.4 liters of oil.
To find the number of tins that contain 80.4 liters of oil, we need to divide the 80.4 liters of oil by 13.4 liters of oil.
Divide 80.4 liters of oil by 13.4 liters of oil
80.4 liters of oil/13.4 liters of oil = 6.

6 tins contain 80.4 liters of oil.

---

15. Find the cost of 58.3 m cloth if the cost of 1 m cloth is $44.80.

Solution:

Given that the cost of 1 m cloth is $44.80
To find the cost of 58.3 m cloth, multiply 58.3 m cloth with $44.80.
Multiply 58.3 m cloth with $44.80
58.3 m cloth × $44.80 = $2611.84

The cost of 58.3 m cloth is $2611.84.

---

16. Shruti bought a bag for $387.05. She gave the shopkeeper 2 notes of $200. How much money will she get back?

Solution:

Given that Shruti bought a bag for $387.05. She gave the shopkeeper 2 notes of $200.
she gave 2 notes of 200
Add 2 notes cost = 200 + 200 = 400.
Now, subtract $400 from $387.05 to get the amount she gets back from the shopkeeper.
Subtract $400 from $387.05.
$400 – $387.05 = $12.95

Shruti gets back $12.95 from the shopkeeper.

---

17. A tailor needs 46.36 m of cloth for the shirts and 56.90 m for trousers. How much cloth does the tailor need in all?

Solution:

Given that a tailor needs 46.36 m of cloth for the shirts and 56.90 m for trousers.
The tailor needs all will get by adding the cloth for the shirts and cloth for the trousers.
46.36 m of cloth for the shirts
56.90 m of cloth for trousers.
Add 46.36 m and 56.90 m
46.36 m + 56.90 m = 103.26 m

The tailor needs 103.26 m cloth in total.

---

18. A spool of thread has a thread measuring 97.60 m. If 53.44 m thread has been cut, what length of thread is still left in the spool?

Solution:

Given that a spool of thread has a thread measuring 97.60 m.
If 53.44 m thread has been cut, subtract the 53.44 m thread from the thread measuring 97.60 m.
The total amount of thread is 97.60 m.
The thread that has been cut is 53.44 m.
Subtract 53.44 m from 97.60 m.
97.60 m – 53.44 m = 44.16 m.

The length of thread is still left in the spool is 44.16 m.

---

19. The cost of a chair is $3056.94. Tania wants to buy 7 chairs for her house. How much money will she pay to the shopkeeper?

Solution:

Given that the cost of a chair is $3056.94. Tania wants to buy 7 chairs for her house.
To find the money she will pay to the shopkeeper, multiply the number of chairs by the cost of the chair.
The cost of a chair is $3056.94.
The number of chairs is 7.
Multiply $3056.94 with 7.
$3056.94 × 7 = $21398.58

She will pay $21398.58 to the shopkeeper.

---

20. David has a jug full of milk. He pours the complete milk in 5 glasses, each glass of capacity 0.6 l. How much milk was there in the jug?

Solution:

Given that David has a jug full of milk. He pours the complete milk in 5 glasses, each glass of capacity 0.6 l.
To find the total milk in the jug, multiply 5 glasses of milk with 0.6 l capacity.
The total number of glasses = 5.
Each glass capacity is 0.6 l
Multiply 0.6 l with 5.
0.6 l × 5 = 3 l.

The total milk in the jug is 3 l.

---

21. Find the area of a square whose side is 2.50 m.

Solution:

Given that the side of a square is 2.50 m.
To find the area of a square, multiply 2.50 m with 2.50 m.
2.50 m × 2.50 m = 6.25 m.

The area of a square whose side is 2.50 m is 6.25 m.

---

22. The weight of 1 bag of sugar is 11.4 kg. What is the weight of 14 such bags?

Solution:

Given that the weight of 1 bag of sugar is 11.4 kg.
To find the weight of 14 such bags, multiply the weight of 1 bag of sugar with 14 bags.
Multiply the weight of 1 bag of sugar 11.4 kg with 14.
11.4 kg × 14 = 159.6 kg

The weight of 14 such bags is 159.6 kg.

---

23. A vehicle covers a distance of 10.2 km in 3.4 liters of petrol. How much distance will it cover in 1 liter of petrol?

Solution:

Given that a vehicle covers a distance of 10.2 km in 3.4 liters of petrol.
To find the distance that covers 1 liter of petrol, divide the total distance by given liters of petrol.
The total distance covered is 10.2 km.
The total liters of petrol is 3.4 liters.
Divide 10.2 km by 3.4 liters.
10.2 km/3.4 liters = 3 km/liter.

3 km covered in 1 liter of petrol.

---

24. Ron has 5.60 l of juice. He pours it into 8 glasses equally. How much juice is there in each glass?

Solution:

Given that Ron has 5.60 l of juice. He pours it into 8 glasses equally.
The total amount of juice is 5.60 l.
The number of glasses is 8.
To find out the juice in each glass, divide the 5.60 l by 8.
divide the 5.60 l by 8
5.60 l/8 = 0.7 l.

0.7 l juice is there in each glass.

---

25. Shelly has a ribbon of length 44.44 m. She cuts it into 11 equal parts. What is the length of each equal part?

Solution:

Given that Shelly has a ribbon of length 44.44 m. She cuts it into 11 equal parts.
To find the length of each equal part, divide the length of the ribbon by the number of equal parts.
The ribbon length is 44.44 m.
The number of equal parts is 11.
Divide 44.44 m by 11.
44.44 m/11 = 4.4 m.

4.4 m is the length of each equal part.

---

26. The cost of 4 pens is $49.24. What is the cost of 1 pen?

Solution:

Given that the cost of 4 pens is $49.24.
To find the cost of each pen, divide the total amount by the number of pens.
The total cost of the pens is $49.24.
The total number of pens is 4.
Divide $49.24 by 4.
$49.24/4 = $12.31

The cost of 1 pen is $12.31.

---

27. The weight of a box is 141.029 kg. What will be the weight of 23 such boxes?

Solution:

Given that the weight of a box is 141.029 kg.
To find the weight of 23 such boxes, multiply 141.029 kg by 23.
Divide 141.029 kg by 23.
141.029 kg × 23 = 3243.667 kg.

The weight of 23 such boxes is 3243.667 kg.

---

28. Sonia has 18.48 l of juice. She pours it into 8 jars equally. How much juice is there in each jar?

Solution:

Given that Sonia has 18.48 l of juice. She pours it into 8 jars equally.
To find the amount of juice in each jar, divide the total liters of juice by the number of jars.
The total liters of juice is 18.48 l.
The number of jars is 8.
Divide 18.48 l of juice by 8 jars.
18.48 l/8 = 2.31 liters

2.31 liters of juice present in each jar.

---

Round off is a type of estimation. Estimation is used in subjects like mathematics and physics. Round off means making a number simpler by keeping its value intact closer to the next number. Round off to nearest 10 is nothing but making the unit digits of the number to zero and getting the estimated nearest 10 for that number. Check the rules, detailed steps, and solved examples on rounding the numbers to the nearest 10.

Round off to Nearest 10 – Definition

Round off is a process of making a number simpler to read and remember. It is done for the whole numbers, decimals for various places of tens, thousands, hundreds, etc. Round off to Nearest 10 means writing the nearest 10 of the given number. By using the Rounding Numbers to the Nearest 10, you can easily estimate the answer quickly and easily. It is also used to get the average score of people in the class.

Get More Related Articles

• Round off to Nearest 100
• Rounding Decimals to the Nearest Whole Number
• Correct to Two Decimal Places

Rules for Rounding Numbers to Nearest 10

We have two different rules for rounding numbers to the nearest 10. They are explained in the below modules

• Rule 1: When rounding the numbers to the nearest 10, if the digit in the unit’s place is less than 5 or between 0 and 4, then the unit’s place of the number is replaced by 0.
• Rule 2: If the digit in the unit’s place is greater than or equal to 5 or between 5 and 9, then the unit’s place is replaced by 0, and the tens place of the number is increased by 1.

How to Round Numbers to Nearest 10?

Follow the below-listed procedure to learn Round off to Nearest 10. They are along the lines

• Get a whole number for rounding.
• Identify the digit in the unit’s place.
• If the digit is between 0 and 4, then place zero in the unit’s place of the number.
• If the digit is or 6 or 7 or 8 or 9, then place zero in the unit place and add 1 to the tens place of the number.
• Now, write the new number as a rounded or estimated number.

Rounding to the Nearest Ten Examples

Example 1:

Round the following numbers to the nearest 10.

(i) 63

(ii) 578

(iii) 1052

Solution:

(i) The given number is 63

We see the digit in the unit place is 3 means that is less than 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place.

Therefore, rounding off 63 to the nearest 10 is 60.

(ii) The given number is 578

We see the digit in the unit place is 8 means that is more than 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place and increase digit in tens place by 1.

Therefore, rounding off 578 to the nearest 10 is 580.

(iii) The given number is 1052

We see the digit in the unit place is 2 means that is less than 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place.

Therefore, rounding off 1052 to the nearest 10 is 1050.

Example 2:

Round off the below-mentioned numbers to the nearest 10.

(i) 167

(ii) 55

(iii) 109

Solution:

(i) The given number is 167

We see the digit in the unit place is 7 means that is greater than 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place and increase digit in tens place by 1.

Therefore, the obtained number is 170.

(ii) The given number is 55

We see the digit in the unit place is 5 means that is equal to 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place and increase digit in tens place by 1.

Therefore, rounding off 55 to the nearest 10 is 60.

(iii) The given number is 109

We see the digit in the unit place is 9 means that is more than 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place and increase digit in tens place by 1.

Therefore, round off 109 to the nearest 10 is 110.

Example 3:

Round off to Nearest 10.

(i) 221

(ii) 854

(iii) 57

Solution:

(i) The given number is 221

We see the digit in the unit place is 1 means that is lesser than 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place.

Therefore, round off 221 to the nearest 10 is 220.

(ii) The given number is 854

We see the digit in the unit place is 4 means that is less than 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place.

Therefore, round off 854 to the nearest 10 is 850.

(iii) The given number is 57

We see the digit in the unit place is 7 means that is more than 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place and increase digit in tens place by 1.

Therefore, round off 57 to the nearest 10 is 60.

Example 4:

Round the following numbers to the nearest tens.

(i) 5526

(ii) 328

Solution:

(i) The given number is 5526

We see the digit in the unit place is 6 means that is more than 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place and increase digit in tens place by 1.

Therefore, round off 5526 to the nearest 10 is 5530.

(ii) The given number is 328

We see the digit in the unit place is 8 means that is more than 5. So, we round to the nearest multiple of a ten which is less than the number. So place zero in the unit place and increase digit in tens place by 1.

Therefore, round off 328 to the nearest 10 is 330.