A Decimal Number consists of a whole number part and a fractional part separated by a decimal point. If you wish to learn completely about Decimal Places and How to Learn to Count Decimal Places. Know about Decimal Place Value Chart Definition, Facts, Solved Examples in the further modules. We are sure you will be familiar with the Decimal Places by the end of this article.
What is meant by Decimal Places?
A Decimal Number consists of both whole number part and decimal number part. The digits right to the decimal point are called the Decimal Part and the digits left to the decimal point are called the whole number part. The Number of Digits present in the decimal part of the given decimal number is known as Decimal Places.
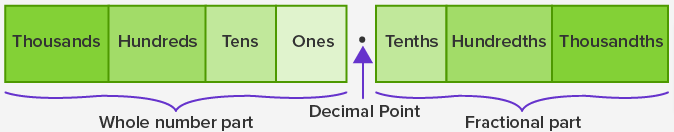
Based on the position of the digit in the number it has a value named place value. For Example, the Place Value of the digit 2 in 1234.45 is 200 as 2 is in the hundreds place. However, if you interchange the digits 3 and 2 we get a new number i.e. 1324.45. In 1324.45 the place value of a digit is 20 as it is in the tens place.
How to Learn to Count Decimal Places?
The number of digits present in the decimal part of the given decimal number is nothing but the Decimal Places. Check out the below-listed examples to understand how to read and count the decimal places for the given decimal number.
For Example:
Decimal Number 5.34 has 2 decimal places.
Decimal Number 0.376 has 3 decimal places.
The Number 86.261 has 3 decimal places.
The Number 912.67 has 2 decimal places.
To better understand the Decimal Numbers you need to be aware of the Place Value.
For Example Decimal Number 51.048053 Place Value is explained clearly below.

In the above-illustrated example, 51 is the whole number part and 048053 is the decimal or fractional part.
Whole Number Part
Place of 1 is Ones and its place value is 1
Place of 5 is Tens and its place value is 50
Decimal Number Part
Place of 0 is Tenths and its place value is 0*\(\frac { 1 }{ 10 } \) = 0
Place of 4 is hundredths and its place value is 4*\(\frac { 1 }{ 100 } \) = \(\frac { 4 }{ 100 } \) = 0.04
Place of 8 is Thousandths and its place value is 8*\(\frac { 1 }{ 1000 } \) = \(\frac { 8 }{ 1000 } \) = 0.008
Place of 0 is Ten Thousandths and its place value is 0*\(\frac { 1 }{ 10,000 } \) = 0
Place of 5 is Hundred Thousandths and its place value is 5*\(\frac { 1 }{ 100,000 } \) = \(\frac { 5 }{ 100,000 } \) = 0.00005
Place of 3 is Millionths and its place value is 3*\(\frac { 1 }{ 1000000 } \) = \(\frac { 3 }{ 1000000 } \) = 0.000003
= 5*10+1*1+0*10-1+4*10-2+8*10-3+0*10-4+5*10-5+3*10-6
= 51.048053
FAQs on Decimal Places
1. What are Decimal Places?
Decimal Places are nothing but the number of digits next to the decimal point or in the decimal part.
2. How do you find the Decimal Places?
Firstly, count the number of digits after the decimal point and the number itself tells the Decimal Places for a particular decimal number.
3. How many decimal places are there in the Decimal Number 32.4356?
The number of Decimal Places in the Decimal Number 32.4356 is 4.