Do you want to more of the concept Mixed Recurring Decimals such as Definitions, Examples, How to Identify a Mixed Recurring Decimal, etc? If so, this is the right place where you can access them completely. Also, learn about how a mixed recurring decimal can be represented simply by going through the further modules.
Do Read Similar Articles:
What is meant by Mixed Recurring Decimal?
A Decimal in which at least one of the digits next to the decimal point is non-repeated and some digits are repeated is known as a Mixed Recurring Decimal.
Example: 5/18 in decimal form is written as 0.277777…
It is called a Mixed Recurring Decimal as the digit 2 after the decimal point is not repeated and the digit 7 is repeating. We can write the same mixed recurring decimal in other forms as 0.2\(\overline{7}\)
How to Identify a Mixed Recurring Decimal?
Go through the below-listed steps and easily identify whether it is a mixed recurring decimal or not. They are in the below fashion
- Initially, divide the numerator with the denominator from the given fraction.
- Check if at least one of the digits next to the decimal point is non-repetitive and some of the digits repeat or not.
- If the condition above is satisfied then the given fraction is a mixed recurring decimal.
Mixed Recurring Decimals Examples
1. What is \(\frac { 19 }{ 6 } \) in Decimal Form and State Whether it is a Mixed Recurring Decimal or not?
Solution:
Given Fraction is \(\frac { 19 }{ 6 } \)
Firstly, divide the numerator with the denominator.
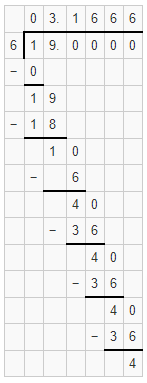
After dividing we got the result as 3.166666….
Since it has one non-repetitive digit in the decimal and some other digits are repeating it is said to be a Mixed Recurring Decimal and can be written as 3.1\(\overline{6}\)
Therefore, \(\frac { 19 }{ 6 } \) converted to decimal form is 3.1\(\overline{6}\)
2. What is \(\frac { 35 }{ 6 } \) in Decimal Form and State Whether it is a Mixed Recurring Decimal or not?
Solution:
Given fraction is \(\frac { 35 }{ 6 } \)
Simply divide the numerator with the denominator
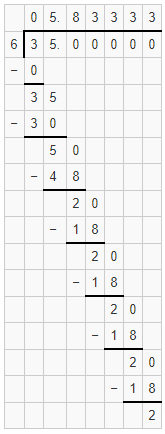
After performing the division we have the result 5.833333….
Since it has one non-repetitive digit 8 in the decimal and some other digits are repeating it is said to be a Mixed Recurring Decimal and can be written as 5.8\(\overline{3}\)
Therefore, \(\frac { 35 }{ 6 } \) converted to decimal form is 5.8\(\overline{3}\)
FAQs on Mixed Recurring Decimal
1. What is a Mixed Recurring Decimal?
A Decimal in which at least one of the digits next to the decimal point doesn’t repeat and some other digits repeat then it is called a Mixed Recurring Decimal.
2. How to Determine a Mixed Recurring Decimal?
Simply divide the numerator with the denominator. Check if at least one digit after the decimal point is not repeated and few other digits repeat. Then it is said to be a Mixed Recurring Decimal.
3. Is 0.1252525….. a Mixed Recurring Decimal?
Yes, 0.1252525…. is a Mixed Recurring Decimal as 1 is not repeating and the remaining digits 25 are repeating continuously.