Engage NY Eureka Math Algebra 1 Module 5 Lesson 8 Answer Key
Eureka Math Algebra 1 Module 5 Lesson 8 Example Answer Key
Example 1.
Christine has $500 to deposit in a savings account, and she is trying to decide between two banks. Bank A offers 10% annual interest compounded quarterly. Rather than compounding interest for smaller accounts, Bank B offers to add $15 quarterly to any account with a balance of less than $1,000 for every quarter, as long as there are no withdrawals. Christine has decided that she will neither withdraw, nor make a deposit for a number of years.
Develop a model that will help Christine decide which bank to use.
Answer:
Students may decide to use a table, graph, or equation to model this situation. For this situation, a table allows comparison of the balances at the two banks. It appears that Bank B has a higher balance until the fourth year.
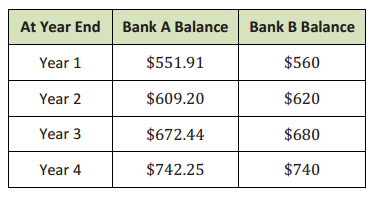
It is tempting to just say that at more than 3 years, Bank A is better. If students reach this conclusion, use the following line of questioning:
Can we give a more exact answer? Since the interest is compounded quarterly, we may want to consider the quarters of year 3. If after choosing a bank, Christine wanted to make sure her money was earning as much as possible, after which quarter would she make the withdrawal?
Since the balances intersect in year 4, we can look at the balances for each quarter of that year:
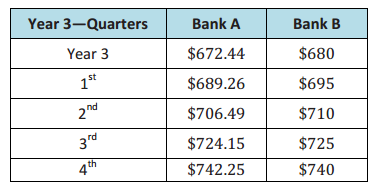
We can see from this table that it is not until the 4th quarter that Bank A begins to make more money for Christine. If she chooses Bank A, she should leave her money there for more than 3 years and 9 months. If she chooses Bank B, she should withdraw before the 4th quarter of Year 3.
Bank A: A(t) = 500(1 + \(\frac{0.10}{4}\))4t or 500(1.025)4t
Bank B: B(t) = 500 + 60t (Since her money earns $15 quarterly, then $15(4), or $60 per year.)
Example 2.
Alex designed a new snowboard. He wants to market it and make a profit. The total initial cost for manufacturing set – up, advertising, etc. is $500,000, and the materials to make the snowboards cost $100 per board.
The demand function for selling a similar snowboard is D(p) = 50 000 – 100p, where p represents the selling price (in dollars) of each snowboard.
a. Write an expression for each of the following in terms of p.
Demand Function (number of units that will sell)
Answer:
This is given as 50 000 – 100p.
Revenue (number of units that will sell)(price per unit, p)
Answer:
(50 000 – 100p)p = 50 000p – 100p2
Total Cost (cost for producing the snowboards)
Answer:
This is the total overhead costs plus the cost per snowboard times the number of snowboards:
500 000 + 100(50 000 – 100p)
500 000 + 5 000 000 – 10 000p
5 500 000 – 10 000p
b. Write an expression to represent the profit.
Answer:
Profit = Total Revenue – Total Cost
= (50 000p – 100p2 ) – (5 500 000 – 10 000p)
Therefore, the profit function is f(p) = – 100p2 + 60 000p – 5 500 000.
c. What is the selling price of the snowboard that will give the maximum profit?
Answer:
Solve for the vertex of the quadratic function using completing the square.
(f(p) = – 100p2 + 60 000p – 5 500 000
= – 100(p2 – 600p + ) – 5 500 000
= – 100(p2 – 600p + 3002 ) – 5 500 000 + 100(300)2
= – 100(p – 300)2 – 5 500 000 + 9 000 000
= – 100(p – 300)2 + 3 500 000)
The maximum point is (300,3 500 000). Therefore, the selling price that will yield the maximum profit is $300.
d. What is the maximum profit Alex can make?
Answer:
According to the vertex form, the maximum profit would be $3,500,000 for selling at a price of $300 for each snowboard.
Eureka Math Algebra 1 Module 5 Lesson 8 Exercise Answer Key
Exercises
Alvin just turned 16 years old. His grandmother told him that she will give him $10,000 to buy any car he wants whenever he is ready. Alvin wants to be able to buy his dream car by his 21st birthday, and he wants a 2009 Avatar Z, which he could purchase today for $25,000. The car depreciates (reduces in value) at a rate is 15% per year. He wants to figure out how long it would take for his $10,000 to be enough to buy the car, without investing the $10,000.
Exercise 1.
Write the function that models the depreciated value of the car after n number of years.
Answer:
f(n) = 25 000(1 – 0.15)n or f(n) = 25 000(0.85)n
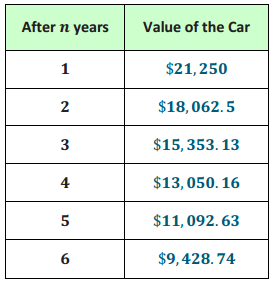
a. Will he be able to afford to buy the car when he turns 21? Explain why or why not.
Answer:
f(n) = 25 000(0.85)n
f(5) = 25 000(0.85)5 = 11 092.63
No, he will not be able to afford to buy the car in 5 years because the value of the car will still be $11,092.63, and he has only $10,000.
b. Given the same rate of depreciation, after how many years will the value of the car be less than $5,000?
Answer:
f(10) = 25 000(0.85)10 = 4921.86
In 10 years, the value of the car will be less than $5,000.
c. If the same rate of depreciation were to continue indefinitely, after how many years would the value of the car be approximately $1?
Answer:
f(n) = 25 000(0.85)n = 1
In about 62 years, the value of the car is approximately $1.
Exercise 2.
Sophia plans to invest $1,000 in each of three banks.
Bank A offers an annual interest rate of 12%, compounded annually.
Bank B offers an annual interest rate of 12%, compounded quarterly.
Bank C offers an annual interest rate of 12%, compounded monthly.
a. Write the function that describes the growth of investment for each bank in n years.
Answer:
Bank A A(n) = 1000(1.12)n
Bank B B(n) = 1000(1 + \(\frac{0.14}{4}\))4n or 1000(1.03)4n
Bank C C(n) = 1000(1 + \(\frac{0.12}{12}\))12n or 1000(1.01)12n
b. How many years will it take to double her initial investment for each bank? (Round to the nearest whole dollar.)
Answer:
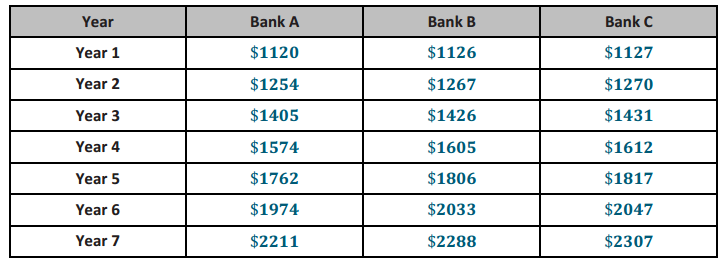
For Bank A, her money will double after 7 years.
For Banks B and C, her investment will double its value during the 6th year.
c. Sophia went to Bank D. The bank offers a “double your money” program for an initial investment of $1,000 in five years, compounded annually. What is the annual interest rate for Bank D?
Answer:
Given information for Bank D:
Initial investment = $1,000
Number of Years = 5 (She would have $2,000 in 5 years.)
Compounded annually:
2×1000 = 1000(1 + r)5
2000 = 1000(1 + r)5
\(\frac{2000}{1000}\) = \(\frac{1000(1 + r)^{5}}{1000}\)
2 = (1 + r)5
Since we see that 2 is the 5th power of a number, we must take the 5th root of 2.
\(\sqrt [ 5 ]{ 2 }\) = (1 + r)
1.1487 = 1 + r
1.1487 – 1 = r
r = 0.1487 or 14.87% (annual interest rate for Bank D)
Eureka Math Algebra 1 Module 5 Lesson 8 Problem Set Answer Key
Question 1.
Maria invested $10,000 in the stock market. Unfortunately, the value of her investment has been dropping at an average rate of 3% each year.
a. Write the function that best models the situation.
Answer:
f(n) = 10 000(1 – 0.03)n or 10 000(0.97)n
where n represents the number of years since the initial investment
b. If the trend continues, how much will her investment be worth in 5 years?
Answer:
f(n) = 10 000(0.97)n
f(5) = 10 000(0.97)5
f(5) = 8587.34 or $8,587.34
c. Given the situation, what should she do with her investment?
Answer:
There are multiple answers, and there is no wrong answer. Sample Responses:
Answer 1: After two years, she should pull out her money and invest it in another company.
Answer 2: According to experts, she should not touch her investment and wait for it to bounce back.
Question 2.
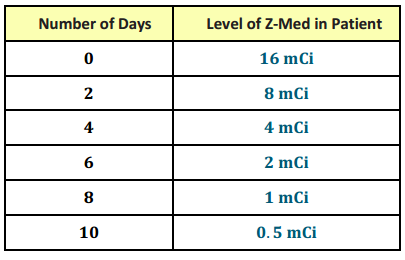
The half – life of the radioactive material in Z – Med, a medication used for certain types of therapy, is 2 days. A patient receives a 16 mCi dose (millicuries, a measure of radiation) in his treatment. (Half – life means that the radioactive material decays to the point where only half is left.)
a. Make a table to show the level of Z – Med in the patient’s body after n days.
Answer:
b. Write a formula to model the half – life of Z – Med for n days. (Be careful here. Make sure that the formula works for both odd and even numbers of days.)
Answer:
f(n) = 16(\(\frac{1}{2}\))\(\frac{n}{2}\)
where n represents the number of days after the initial measurement of 16 mCi
c. How much radioactive material from Z – Med is left in the patient’s body after 20 days of receiving the medicine?
Answer:
For n = 20
f(20) = 16(0.5)10
f(20) = 0.015625
After ten days, there is 0.015625 mCi of the radioactive material in Z – Med left in the patient’s body.
Question 3.
Suppose a male and a female of a certain species of animal were taken to a deserted island. The population of this species quadruples (multiplies by 4) every year. Assume that the animals have an abundant food supply and that there are no predators on the island.
a. What is an equation that can be used to model the population of the species?
Answer:
f(n) = 2(4)n
where n represents the number of years after their arrival at the island
b. What will the population of the species be after 5 years?
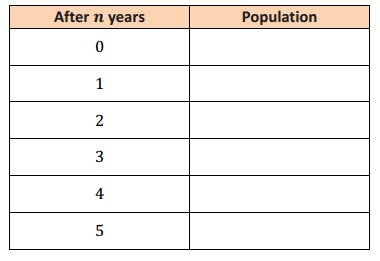
Answer:
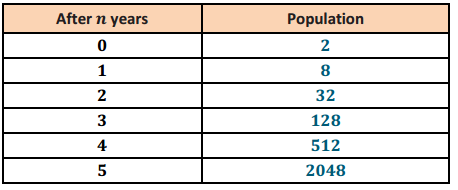
In 5 years, the population of the animals will reach 2,048.
c. Write an equation to find how many years it will take for the population of the animals to exceed 1 million. Find the number of years, either by using the equation or a table
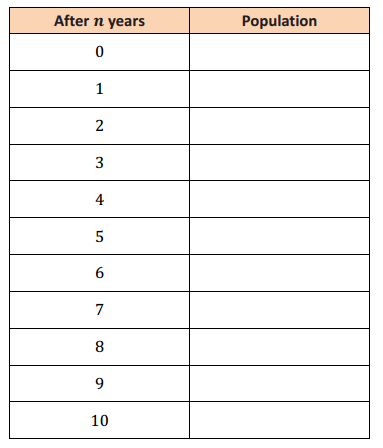
Answer:
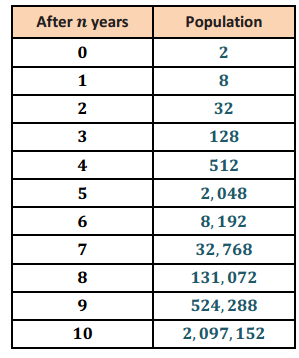
Using the equation: 2(4)n = 1 000 000
4n = 500 000
Note: Students will likely be unable to solve this equation without using trial and error (educated guessing). They may come up with 2(4)9.5 = 1 048 576 using this method.
Question 4.
The revenue of a company for a given month is represented as R(x) = 1,500x – x2 and its costs as C(x) = 1,500 + 1,000x. What is the selling price, x, of its product that would yield the maximum profit? Show or explain your answer.
Answer:
P(x) = Revenue – Cost
P(x) = R(x) – C(x)
P(x) = (1500x – x2) – (1500 + 1000x)
P(x) = – x2 + 500x – 1500
Profit Function
To find the vertex, we can complete the square for the function:
P(x) = – x2 + 500x – 1500
= – 1(x2 – 500x + ) – 1500 Group the x – terms and factor out the – 1.
= – 1(x2 – 500x + 62 500) – 1500 + 62 500 Complete the square.
P(x) = – 1(x – 250)2 + 61 000
So, the maximum point will be (250,61 000), and the selling price should be $250 per unit to yield a maximum profit of $61,000.
Eureka Math Algebra 1 Module 5 Lesson 8 Exit Ticket Answer Key
Answer the following question. Look back at this (or other) lessons if you need help with the business formulas.
Jerry and Carlos each have $1,000 and are trying to increase their savings. Jerry will keep his money at home and add $50 per month from his part – time job. Carlos will put his money in a bank account that earns a 4% yearly interest rate, compounded monthly. Who has a better plan for increasing his savings?
Answer:
Jerry’s savings: J(n) = 1000 + 10(n), where n represents the number of months
Carlos’s savings: C(n) = 1000(1 + \(\frac{0.06}{12}\))n, where n represents the number of months
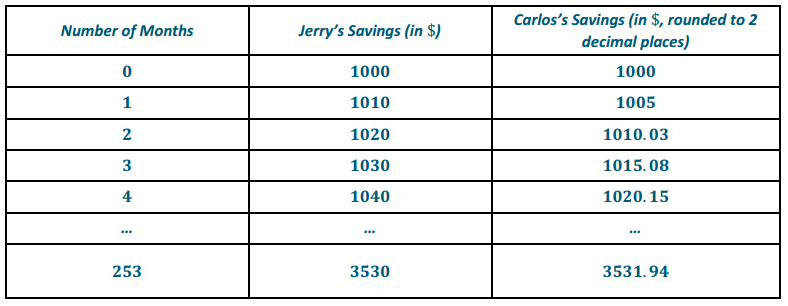
In the short term, Jerry’s plan is better, but I know that Carlos’s plan will eventually exceed Jerry’s because an increasing exponential model will always outgain an increasing linear model eventually. If they continued saving for 253 months, Carlos’s plan would be better.