Eureka Math

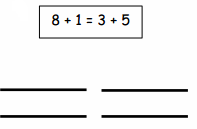
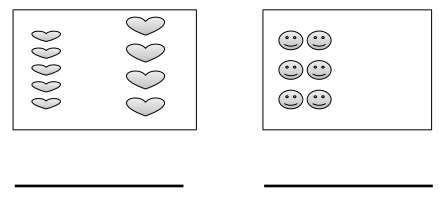
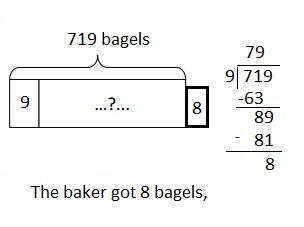
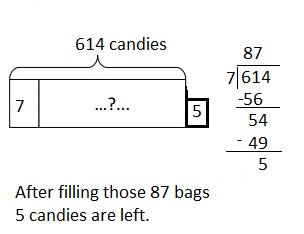
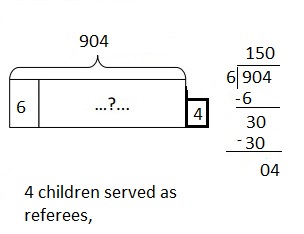
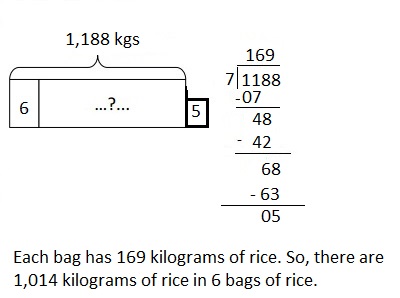
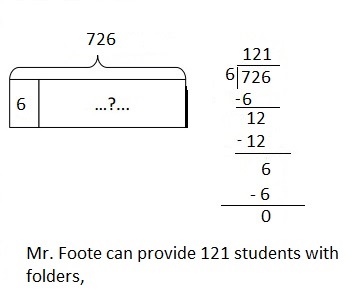
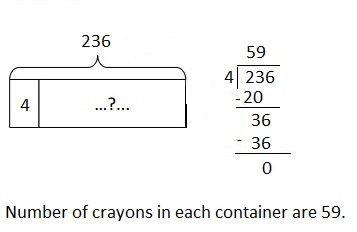
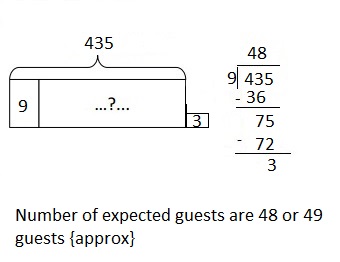
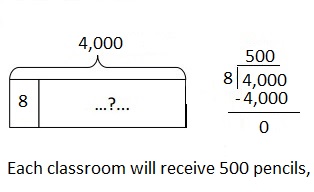
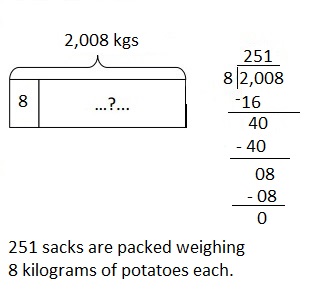
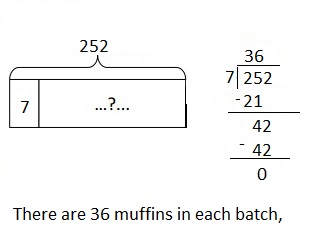
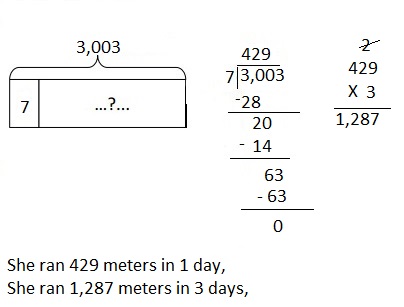
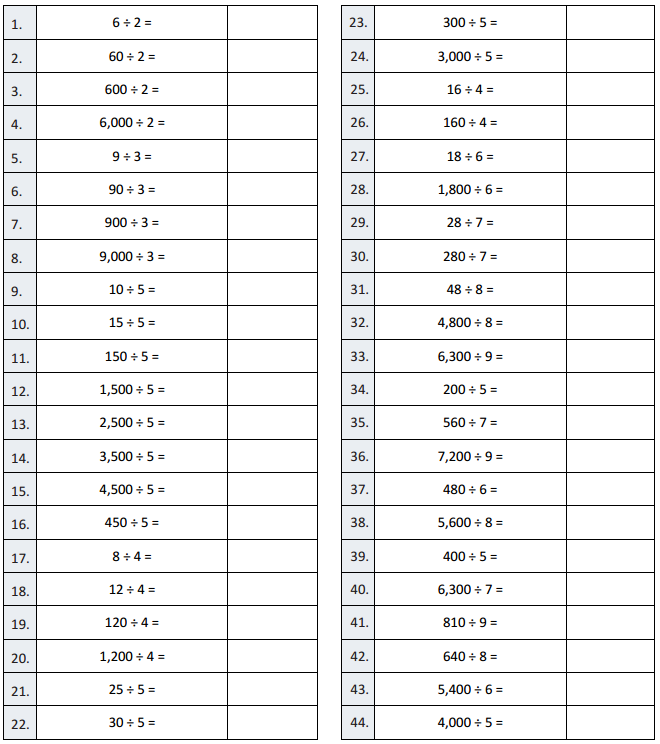
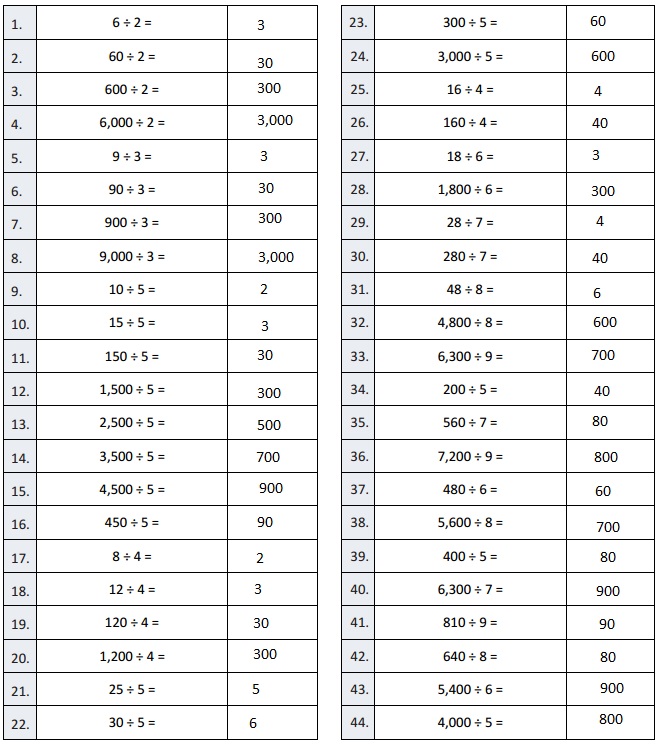
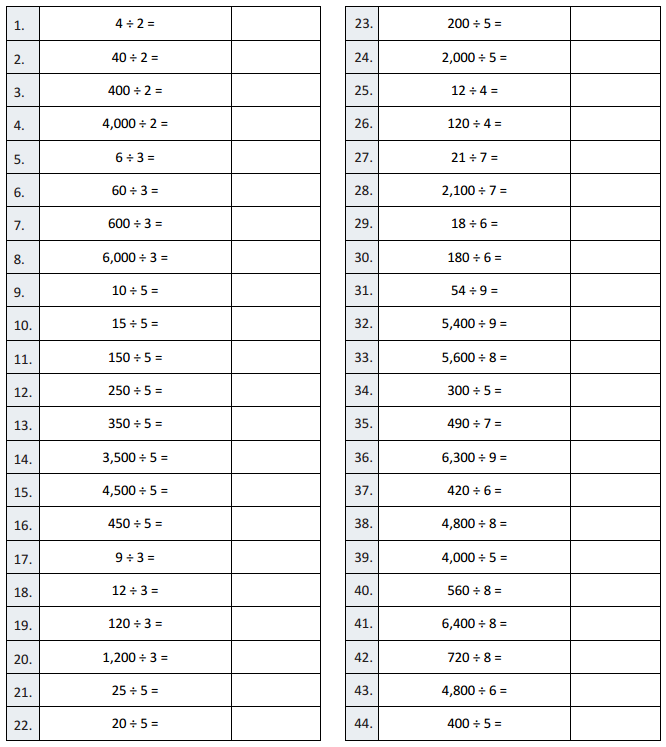
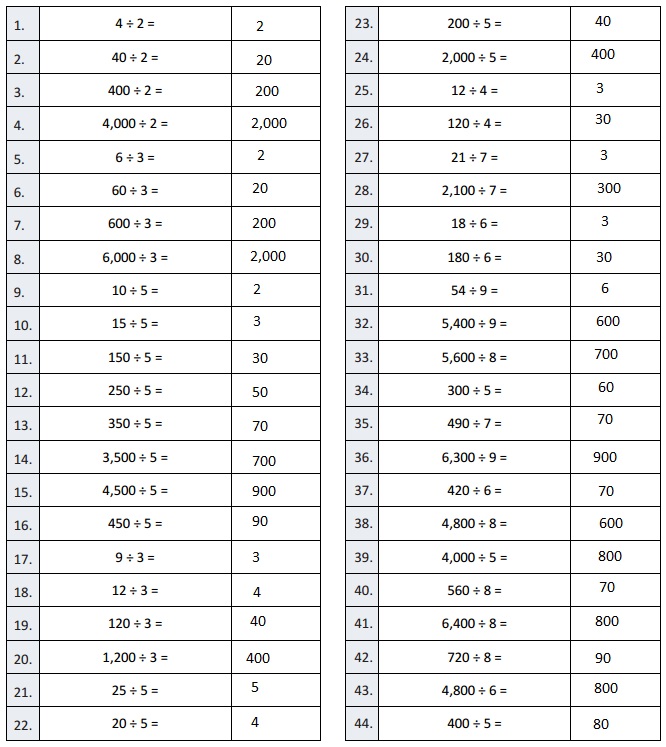
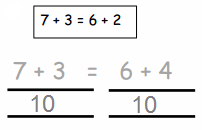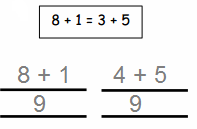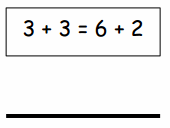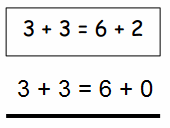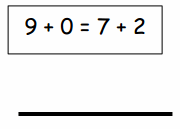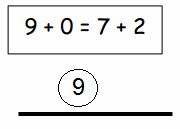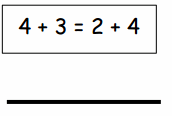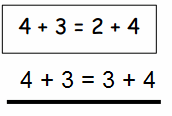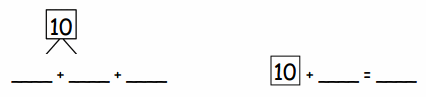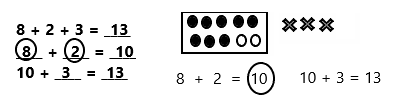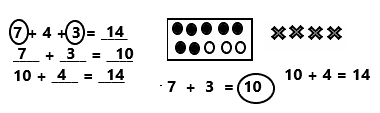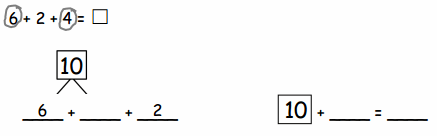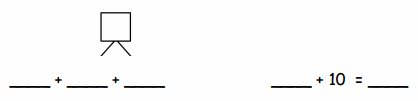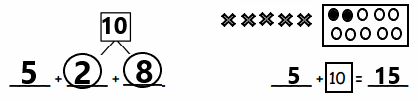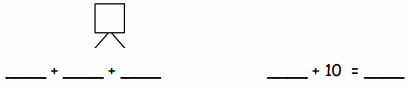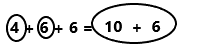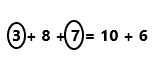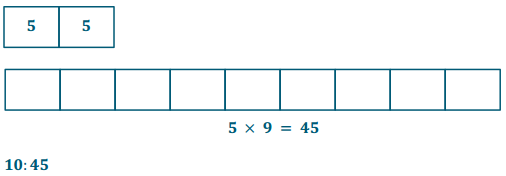Engage NY Eureka Math 7th Grade Module 2 Lesson 4 Answer Key

Eureka Math Grade 7 Module 2 Lesson 4 Example Answer Key

Example 1:
Rule for Adding Integers with Same Signs
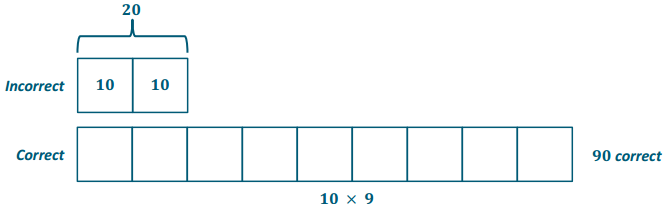
a. Represent the sum of 3+5 using arrows on the number line.

Answer:

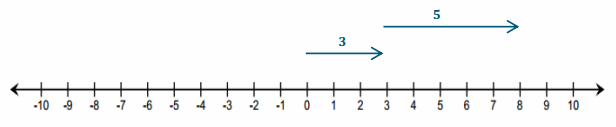
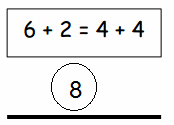
3 + 5 = 8

i. How long is the arrow that represents 3?
Answer:
3 units

b. What direction does it point?
Answer:
Right/up

iii. How long is the arrow that represents 5?
Answer:
5 units

iv. What direction does it point?
Answer:
Right/up

v. What is the sum?
Answer:
8

vi. If you were to represent the sum using an arrow, how long would the arrow be, and what direction would it point?
Answer:
The arrow would be 8 units long and point to the right (or up on a vertical number line).

vii. What is the relationship between the arrow representing the number on the number line and the absolute value of the number?
Answer:
The length of an arrow representing a number is equal to the absolute value of the number.

vii. Do you think that adding two positive numbers will always give you a greater positive number? Why?
Answer:
Yes, because the absolute values are positive, so the sum will be a greater positive. On a number line, adding them would move you farther away from 0 (to the right or above) on a number line.

From part (a), use the same questions to elicit feedback.
→ In the Integer Game, I would combine -3 and -5 to give me -8.

b. Represent the sum of -3 + (-5) using arrows that represent -3 and -5 on the number line.

Answer:

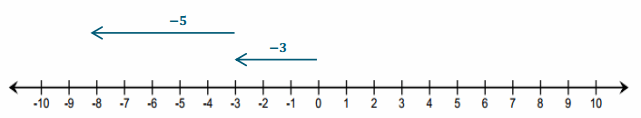
-3 + (-5) = -8

i. How long is the arrow that represents -3?
Answer:
3 units

ii. What direction does it point?
Answer:
Left/down

iii. How long is the arrow that represents -5?
Answer:
5 units

iv. What direction does it point?
Answer:
Left/down

iv. What is the sum?
Answer:
-8

vi. If you were to represent the sum using an arrow, how long would the arrow be, and what direction would it point?
Answer:
The arrow would be 8 units long and point to the left (or down on a vertical number line).

vii. Do you think that adding two negative numbers will always give you a smaller negative number? Why?
Answer:
Yes, because the absolute values of negative numbers are positive, so the sum will be a greater positive. However, the opposite of a greater positive is a smaller negative. On a number line, adding two negative numbers would move you farther away from 0 (to the left or below) on a number line.

c. What do both examples have in common?
Answer:
The length of the arrow representing the sum of two numbers with the same sign is the same as the sum of the absolute values of both numbers.

The teacher writes the rule for adding integers with the same sign.

RULE: Add rational numbers with the same sign by adding the absolute values and using the common sign.

Example 2.
Rule for Adding Opposite Signs
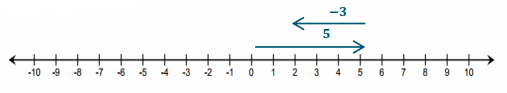
a. Represent 5 + (-3) using arrows on the number line.

Answer:
5 + (-3) = 2

i. How long is the arrow that represents 5?
Answer:
5 units

ii. What direction does it point?
Answer:
Right/up

iii. How long is the arrow that represents -3?
Answer:
3 units

iv. What direction does it point?
Answer:
Left/down

v. Which arrow is longer?
Answer:
The arrow that is five units long, or the first arrow.

vi. What is the sum? If you were to represent the sum using an arrow, how long would the arrow be, and what direction would it point?
Answer:
5 + (-3) = 2
The arrow would be 2 units long and point right/up.

b. Represent the 4 + (-7) using arrows on the number line.

Answer:
4 + (-7) = -3

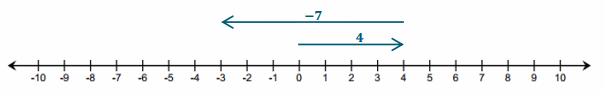
i. In the two examples above, what is the relationship between the length of the arrow representing the sum and the lengths of the arrows representing the two addends?
Answer:
The length of the arrow representing the sum is equal to the difference of the absolute values of the lengths of both arrows representing the two addends.

ii. What is the relationship between the direction of the arrow representing the sum and the direction of the arrows representing the two addends?
Answer:
The direction of the arrow representing the sum has the same direction as the arrow of the addend with the greater absolute value.

iii. Write a rule that will give the length and direction of the arrow representing the sum of two values that have opposite signs.
Answer:
The length of the arrow of the sum is the difference of the absolute values of the two addends. The direction of the arrow of the sum is the same as the direction of the longer arrow.

The teacher writes the rule for adding integers with opposite signs.

RULE: Add rational numbers with opposite signs by subtracting the absolute values and using the sign of the integer with the greater absolute value

Example 3.
Applying Integer Addition Rules to Rational Numbers
Find the sum of 6 + (-2\(\frac{1}{4}\)). The addition of rational numbers follows the same rules of addition for integers.
a. Find the absolute values of the numbers.
Answer:
|6| = 6
|-2\(\frac{1}{4}\)| = 2 \(\frac{1}{4}\)

b. Subtract the absolute values.
6 – 2\(\frac{1}{4}\) = 6 – \(\frac{9}{4}\) = \(\frac{24}{4}\) – \(\frac{9}{4}\) = \(\frac{15}{4}\) = 3\(\frac{3}{4}\)
Answer:

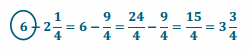
c. The answer will take the sign of the number that has the greater absolute value.
Answer:
Since 6 has the greater absolute value (arrow length), my answer will be positive 3 \(\frac{3}{4}\) .

Eureka Math Grade 7 Module 2 Lesson 3 Exercise Answer Key

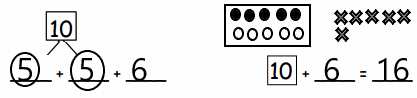
Exercise 2.
a. Decide whether the sum will be positive or negative without actually calculating the sum.
i. -4 + (-2) ___
Answer:
Negative

ii. 5 + 9 ___
Answer:
Positive

iii. -6 + (-3) ____
Answer:
Negative

iv. -1 + (-11) ___
Answer:
Negative

v. 3 + 5 + 7 ___
Answer:
Positive

vi. -20 + (-15) __
Answer:
Negative

b. Find the sum.

i. 15 + 7
Answer:
22

ii. -4 + (-16)
Answer:
-20

iii. -18 + (-64)
Answer:
-82

iv. -205 + (-123)
Answer:
-328

Exercise 3.
Circle the integer with the greater absolute value. Decide whether the sum will be positive or negative without actually calculating the sum.

i. -1 + 2
Answer:

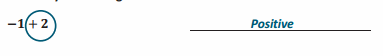
ii. 5 + (-9)
Answer:

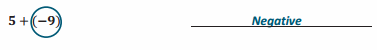
iii. -6 + 3
Answer:

iv. -11 + 1
Answer:

b. Find the sum.

i. -10 + 7
Answer:
-3

ii. 8 + (-16)
Answer:
-8

iii. -12 + (65)
Answer:
53

iv. 105 + (-126)
Answer:
-21

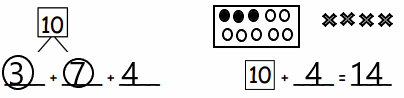
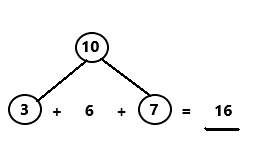
Exercise 4.
Solve the following problems. Show your work.
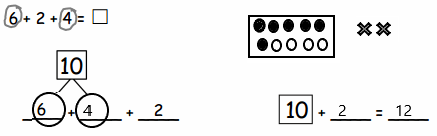
a. Find the sum of
Answer:
|-18| = 18
|7| = 7
18 – 7 = 11
-11

b. If the temperature outside was 73 degrees at 5:00 p.m., but it fell 19 degrees by 10:00 p.m., what is the temperature at 10:00 p.m.? Write an equation and solve.
Answer:

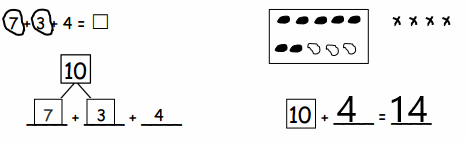
c. Write an addition sentence, and find the sum using the diagram below.

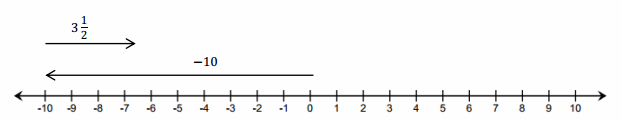
Answer:

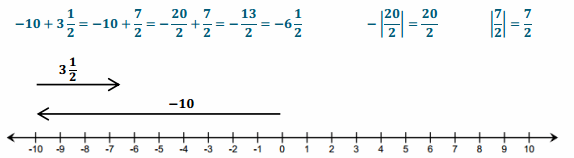
Eureka Math Grade 7 Module 2 Lesson 3 Exit Ticket Answer Key

Question 1.
Write an addition problem that has a sum of -4 \(\frac{4}{5}\) and
a. The two addends have the same sign.
Answer:
Answers will vary. -1 \(\frac{4}{5}\) + (-3) = -4 \(\frac{4}{5}\) .

b. The two addends have different signs.
Answer:
Answers will vary. 1.8 + (-6.6) = -4.8.

Question 2.
In the Integer Game, what card would you need to draw to get a score of 0 if you have a -16, -35, and 18 in your hand?
Answer:
-16 + (-35) + 18 = -33, so I would need to draw a 33 because 33 is the additive inverse of -33.
-33 + 33 = 0.

Eureka Math Grade 7 Module 2 Lesson 3 Problem Set Answer Key

Question 1.
Find the sum. Show your work to justify your answer.
a. 4 + 17
Answer:
4 + 17 = 21

b. -6 + (-12)
Answer:
-6 + (-12) = -18

c. 2.2 + (-3.7)
Answer:
2.2 + (-3.7) = -1.5

d. -3 + (-5) + 8
Answer:
-3 + (-5) + 8 = -8 + 8 = 0

e. \(\frac{1}{3}\) +(-2 \(\frac{1}{4}\))
Answer:
\(\frac{1}{3}\) +(-2 \(\frac{1}{4}\)) = \(\frac{1}{3}\) + (-\(\frac{9}{4}\) ) = \(\frac{4}{12}\) + (-\(\frac{27}{12}\) )= – \(\frac{23}{12}\) = -1\(\frac{11}{12}\)

Question 2.
Which of these story problems describes the sum 19+(-12)? Check all that apply. Show your work to justify your answer.
___ Jared’s dad paid him $19 for raking the leaves from the yard on Wednesday. Jared spent $12 at the movie theater on Friday. How much money does Jared have left?
Answer:
X

___ Jared owed his brother $19 for raking the leaves while Jared was sick. Jared’s dad gave him $12 for doing his chores for the week. How much money does Jared have now?

___ Jared’s grandmother gave him $19 for his birthday. He bought $8 worth of candy and spent another $4 on a new comic book. How much money does Jared have left over?
Answer:
X

Question 3.
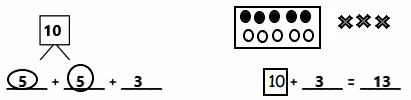
Use the diagram below to complete each part.

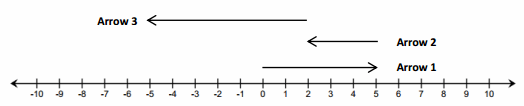
Answer:

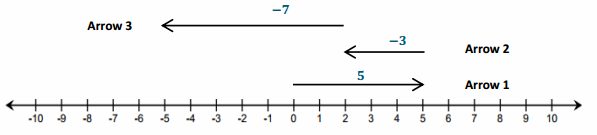
a. Label each arrow with the number the arrow represents.

b. How long is each arrow? What direction does each arrow point?

Answer:

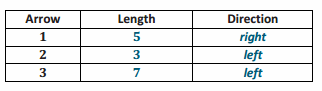
c. Write an equation that represents the sum of the numbers. Find the sum.
Answer:
5 + (-3) + (-7) = -5

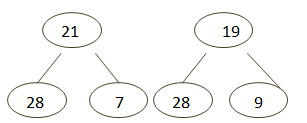
Question 4.
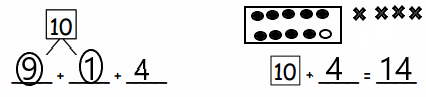
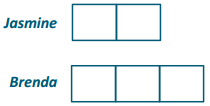
Jennifer and Katie were playing the Integer Game in class. Their hands are represented below.

a. What is the value of each of their hands? Show your work to support your answer.
Answer:
Jennifer’s hand has a value of -3 because 5 + (-8) = -3. Katie’s hand has a value of -2 because
-9 + 7 = -2.

b. If Jennifer drew two more cards, is it possible for the value of her hand not to change? Explain why or why not.
Answer:
It is possible for her hand not to change. Jennifer could get any two cards that are the exact opposites such as (-2) and 2. Numbers that are exact opposites are called additive inverses, and they sum to 0. Adding the number 0 to anything will not change the value.

c. If Katie wanted to win the game by getting a score of 0, what card would she need? Explain.
Answer:
Katie would need to draw a 2 because the additive inverse of -2 is 2. -2 + 2 = 0.

d. If Jennifer drew -1 and -2, what would be her new score? Show your work to support your answer.
Answer:
Jennifer’s new score would be -6 because -3 + (-1) + (-2) = -6.

Engage NY Eureka Math 1st Grade Module 1 Lesson 18 Answer Key

Eureka Math Grade 1 Module 1 Lesson 18 Problem Set Answer Key

Question 1.
Add. Color the balloons that match the number in the boy’s mind. Find expressions that are equal. Connect them below with = to make true number sentences.
a.

Answer:

b.

Answer:

Question 2.
Are these number sentences true?

If it is false, rewrite the number sentence to make it true.

a. 3 + 1 = 2 + 2
____________

Answer:
3 + 1 = 2 + 2 
4 = 4

b. 9 + 1 = 1 + 2
____________

Answer:
9 + 1 = 1 + 2
9 + 1 = 1 + 2
10 not equal to 3
correct expression is
9 + 1 = 1 + 9
10 = 10

c. 2 + 3 = 1 + 4
____________

Answer:
2 + 3 = 1 + 4
5 = 5

d. 5 + 1 = 4 + 2
____________

Answer:
5 + 1 = 4 + 2
6 = 6

e. 4 + 3 = 3 + 5
____________

Answer:
4 + 3 = 3 + 5
7 not equal to 8
correct expression is
4 + 3 = 3 + 4
7 = 7

f. 0 + 10 = 2 + 8
____________

Answer:
0 + 10 = 2 + 8
10 = 10

g. 6 + 3 = 4 + 5
____________
Answer:
6 + 3 = 4 + 5
9 = 9

h. 3 + 7 = 2 + 6
____________

Answer:
3 + 7 = 2 + 6
10 not equal to 8
correct expression is
3 + 7 = 7 + 3
10 = 10

Question 3.
Write a number in the expression and solve.
a. 1 + __ = 3 + 2

Answer:
1 + 4 = 3 + 2 

b. __ + 4 = 2 + 5

Answer:
1 + 4 = 2 + 5

c. __ + 5 = 6 + __

Answer:
2+ 5 = 6 + 1

d. 7 + __ = 8 + __

Answer:
7 + 1= 8 + 0

Eureka Math Grade 1 Module 1 Lesson 18 Exit Ticket Answer Key

Find two ways to fix each number sentence to make it true.

a.

Answer:

b.

Answer:

Eureka Math Grade 1 Module 1 Lesson 18 Homework Answer Key

Question 1.
The pictures below are not equal. Make the pictures equal, and write a true number sentence.

Answer:

Question 2.
Circle the true number sentences, and rewrite the false sentences to make them true.
a.

Answer:

b.

Answer:

c.

Answer:

d.

Answer:

e.

Answer:

f.

Answer:

g.

Answer:

h.

Answer:

i.

Answer:

Question 3.
Find the missing part to make the number of sentences true.

a. 8 + 0 = __ + 4

Answer:
8 + 0 = 4 + 4

b. 7 + 2 = 9 + __

Answer:
7 + 2 = 9 + 0

c. 5 + 2 = 4 + __

Answer:
5 + 2 = 4 + 3

d. 5 + __ = 6 + 0

Answer:
5 + 1 = 6 + 0

e. 5 + 4 = __ + 3

Answer:
5 + 4 = 6 + 3

Engage NY Eureka Math 4th Grade Module 3 Lesson 32 Answer Key

Eureka Math Grade 4 Module 3 Lesson 32 Problem Set Answer Key

Solve the following problems. Draw tape diagrams to help you solve. If there is a remainder, shade in a small portion of the tape diagram to represent that portion of the whole.

Question 1.
A concert hall contains 8 sections of seats with the same number of seats in each section. If there are 248 seats, how many seats are in each section?
Answer:
31 seats are there in each section,

Explanation:
Given a concert hall of 248 seats has 8 sections,
So the number of seats in each section are 31.

Question 2.
In one day, the bakery made 719 bagels. The bagels were divided into 9 equal shipments. A few bagels were left over and given to the baker. How many bagels did the baker get?
Answer:
The baker got 8 bagels,

Explanation:
Given in one day, the bakery made 719 bagels.
The bagels were divided into 9 equal shipments.
There are 9 shipments each to be loaded with equal number of bagels which is 79. After all the bagels were loaded 8 bagels were left which were given to the baker.

Question 3.
The sweet shop has 614 pieces of candy. They packed the candy into bags with 7 pieces in each bag. How many bags of candy did they fill? How many pieces of candy were left?
Answer:
The number of candies left are 5,

Explanation:
According to the problem number of candies in each bag are 7,
So number of bags filled are 87. After filling those 87 bags 5 candies are left.

Question 4.
There were 904 children signed up for the relay race. If there were 6 children on each team, how many teams were made?
The remaining children served as referees. How many children served as referees?
Answer:
4 children served as referees,

Explanation:
According to the problem number of children in each team is 6.
Number of teams formed are 904 ÷ 6 = 150 teams.
But after formation of those 150 teams 4 children were left who were made referees.

Question 5.
1,188 kilograms of rice are divided into 7 sacks. How many kilograms of rice are in 6 sacks of rice? How many kilograms of rice remain?
Answer:
Each bag has 169 kilograms of rice. So, there are 1,014 kilograms of rice in 6 bags of rice,

Explanation:
According to the problem 7 sacs are to be divided with 1,188 kilograms of rice, So each sack of rice gets 169 kilograms of rice and 5 kilograms of rice is left.

Eureka Math Grade 4 Module 3 Lesson 32 Exit Ticket Answer Key

Solve the following problems. Draw tape diagrams to help you solve. If there is a remainder, shade in a small portion of the tape diagram to represent that portion of the whole.

Question 1.
Mr. Foote needs exactly 6 folders for each fourth-grade student at Hoover Elementary School. If he bought 726 folders, to how many students can he supply folders?
Answer:
Mr. Foote can provide 121 students with folders,

Explanation:
Mr. Foote should provide 6 folders to each child since he has 726 folders he can provide 121 students with folders.

Question 2.
Mrs. Terrance has a large bin of 236 crayons. She divides them equally among four containers. How many crayons does Mrs. Terrance have in each container?
Answer:
Number of crayons in each container are 59,

Explanation:
According to the problem number of crayons are 236 which have to be distibuted into 4 containers, So number of crayons in each container  are 59.

Eureka Math Grade 4 Module 3 Lesson 32 Homework Answer Key

Solve the following problems. Draw tape diagrams to help you solve. If there is a remainder, shade in a small portion of the tape diagram to represent that portion of the whole.

Question 1.
Meneca bought a package of 435 party favors to give to the guests at her birthday party. She calculated that she could give 9 party favors to each guest. How many guests is she expecting?
Answer:
Number of expected guests are 48 or 49 guests {approx},

Explanation:
according to the problem meneca brought 435 party favours.
she expcted she could give each guest 9 party favours. so, we can say she approximately expected 48 to 49 guests.

Question 2.
4,000 pencils were donated to an elementary school. If 8 classrooms shared the pencils equally, how many pencils did each class receive?
Answer:
Each classroom will receive 500 pencils,

Explanation:
according to the problem there are 4,000 pecils which have to be shared among 8 classroom, So each classroom will receive 500 pencils.

Question 3.
2,008 kilograms of potatoes were packed into sacks weighing 8 kilograms each. How many sacks were packed?
Answer:
251 sacks are packed weighing 8 kilograms of potatoes each,

Explanation:
2,008 kilograms of potatoes should be divided among sacks each having 8 kilograms of potates. So the number sacks packed are 251.

Question 4.
A baker made 7 batches of muffins. There was a total of 252 muffins. If there was the same number of muffins in each batch, how many muffins were in a batch?
Answer:
There are 36 muffins in each batch,

Explanation:
According to the problem the baker baked 252 muffins in total,
In each batch he baked equal number of muffins, that is 7 batches.
So each batch had 36 muffins in total.

Question 5.
Samantha ran 3,003 meters in 7 days. If she ran the same distance each day, how far did Samantha run in 3 days?
Answer:
She ran 1,287 meters in 3 days,

Explanation:
Samantha ran 3,003 meters in 7 days. So, she ran 429 meters
in 1 day. Number of meters she ran in three days is 1,287.

Eureka Math Grade 4 Module 3 Lesson 32 Fluency Template Answer Key

__12______________
shapes
Answer:
A. Trapezoid,
B. Square,
C. Parallelogram,
D. Rectangle,
E. Prism,
F. Tetrahedron,
G. Rhombus,
H. Parallelogram,
I. Rectangle,
J. Trapezium,
K. Parallelogram,
L. Trapezium,

Engage NY Eureka Math 4th Grade Module 3 Lesson 31 Answer Key

Eureka Math Grade 4 Module 3 Lesson 31 Sprint Answer Key

Divide.

Answer:

Question 1.
6 ÷ 2 =
Answer:
6 ÷ 2 = 3,

Explanation:
Divided 6 ÷ 2 we get quotient 3.

Question 2.
60 ÷ 2 =
Answer:
60 ÷ 2 = 30,

Explanation:
Divided 60 ÷ 2 we get quotient 30.

Question 3.
600 ÷ 2 =
Answer:
600 ÷ 2 = 300,

Explanation:
Divided 600 ÷ 2 we get quotient 300.

Question 4.
6,000 ÷ 2 =
Answer:
6,000 ÷ 2 = 3,000,

Explanation:
Divided 6,000 ÷ 2 we get quotient 3,000.

Question 5.
9 ÷ 3 =
Answer:
9 ÷ 3 = 3,

Explanation:
Divided 9 ÷ 3 we get quotient 3.

Question 6.
90 ÷ 3 =
Answer:
90 ÷ 3 = 30,

Explanation:
Divided 90 ÷ 3 we get quotient 30.

Question 7.
900 ÷ 3 =
Answer:
900 ÷ 3 = 300,

Explanation:
Divided 900 ÷ 3 we get quotient 3.

Question 8.
9,000 ÷ 3 =
Answer:
9,000 ÷ 3 = 3,000,

Explanation:
Divided 9,000 ÷ 3 we get quotient 3,000.

Question 9.
10 ÷ 5 =
Answer:
10 ÷ 5 = 2,

Explanation:
Divided 10 ÷ 5 we get quotient 2.

Question 10.
15 ÷ 5 =
Answer:
15 ÷ 5 = 3,

Explanation:
Divided 15 ÷ 5 we get quotient 3.

Question 11.
150 ÷ 5 =
Answer:
150 ÷ 5 = 3,

Explanation:
Divided 150 ÷ 5 we get quotient 30.

Question 12.
1,500 ÷ 5 =
Answer:
1,500 ÷ 5 = 300,

Explanation:
Divided 1,500 ÷ 5 we get quotient 300.

Question 13.
2,500 ÷ 5 =
Answer:
2,500 ÷ 5 = 500,

Explanation:
Divided 2,500 ÷ 5 we get quotient 500.

Question 14.
3,500 ÷ 5 =
Answer:
3,500 ÷ 5 = 700,

Explanation:
Divided 3,500 ÷ 5 we get quotient 700.

Question 15.
4,500 ÷ 5 =
Answer:
4,500 ÷ 5 = 900,

Explanation:
Divided 4,500 ÷ 5 we get quotient 900.

Question 16.
450 ÷ 5 =
Answer:
450 ÷ 5 = 90,

Explanation:
Divided 450 ÷ 5 we get quotient 90.

Question 17.
8 ÷ 4 =
Answer:
8 ÷ 4 = 2,

Explanation:
Divided 8 ÷ 4 we get quotient 2.

Question 18.
12 ÷ 4 =
Answer:
12 ÷ 4 = 3,

Explanation:
Divided 12 ÷ 4 we get quotient 3.

Question 19.
120 ÷ 4 =
Answer:
120 ÷ 4 = 30,

Explanation:
Divided 120 ÷ 4 we get quotient 30.

Question 20.
1,200 ÷ 4 =
Answer:
1,200 ÷ 4 = 300,

Explanation:
Divided 1,200 ÷ 4 we get quotient 300.

Question 21.
25 ÷ 5 =
Answer:
25 ÷ 5 = 5,

Explanation:
Divided 25 ÷ 5 we get quotient 5.

Question 22.
30 ÷ 5 =
Answer:
30 ÷ 5 = 6,

Explanation:
Divided 30 ÷ 5 we get quotient 6.

Question 23.
300 ÷ 5 =
Answer:
300 ÷ 5 = 60,

Explanation:
Divided 300 ÷ 5 we get quotient 60.

Question 24.
3,000 ÷ 5 =
Answer:
3,000 ÷ 5 = 600,

Explanation:
Divided 3,000 ÷ 5 we get quotient 600.

Question 25.
16 ÷ 4 =
Answer:
16 ÷ 4 = 4,

Explanation:
Divided 16 ÷ 4 we get quotient 4.

Question 26.
160 ÷ 4 =
Answer:
160 ÷ 4 = 40,

Explanation:
Divided 160 ÷ 4 we get quotient 40.

Question 27.
18 ÷ 6 =
Answer:
18 ÷ 6 = 3,

Explanation:
Divided 18 ÷ 6 we get quotient 3.

Question 28.
1,800 ÷ 6 =
Answer:
1,800 ÷ 6 = 300,

Explanation:
Divided 1,800 ÷ 6 we get quotient 300.

Question 29.
28 ÷ 7 =
Answer:
28 ÷ 7 = 4,

Explanation:
Divided 28 ÷ 7 we get quotient 4.

Question 30.
280 ÷ 7 =
Answer:
280 ÷ 7 = 40,

Explanation:
Divided 280 ÷ 7 we get quotient 40.

Question 31.
48 ÷ 8 =
Answer:
48 ÷ 8 = 6,

Explanation:
Divided 48 ÷ 8 we get quotient 6.

Question 32.
4,800 ÷ 8 =
Answer:
4,800 ÷ 8 = 600,

Explanation:
Divided 4,800 ÷ 8 we get quotient 600.

Question 33.
6,300 ÷ 9 =
Answer:
6,300 ÷ 9 = 7,

Explanation:
Divided 6,300 ÷ 9 we get quotient 7.

Question 34.
200 ÷ 5 =
Answer:
200 ÷ 5 = 40,

Explanation:
Divided 200 ÷ 5 we get quotient 40.

Question 35.
560 ÷ 7 =
Answer:
560 ÷ 7 = 80,

Explanation:
Divided 560 ÷ 7 we get quotient 80.

Question 36.
7,200 ÷ 9 =
Answer:
7,200 ÷ 9 = 800,

Explanation:
Divided 7,200 ÷ 9 we get quotient 800.

Question 37.
480 ÷ 6 =
Answer:
480 ÷ 6 = 80,

Explanation:
Divided 480 ÷ 6 we get quotient 80.

Question 38.
5,600 ÷ 8 =
Answer:
5,600 ÷ 8 = 700,

Explanation:
Divided 5,600 ÷ 8 we get quotient 700.

Question 39.
400 ÷ 5 =
Answer:
400 ÷ 5 = 80,

Explanation:
Divided 400 ÷ 5 we get quotient 80.

Question 40.
6,300 ÷ 7 =
Answer:
6,300 ÷ 7 = 900,

Explanation:
Divided 6,300 ÷ 7 we get quotient 900.

Question 41.
810 ÷ 9 =
Answer:
810 ÷ 9 = 90,

Explanation:
Divided 810 ÷ 9 we get quotient 90.

Question 42.
640 ÷ 8 =
Answer:
640 ÷ 8 = 80,

Explanation:
Divided 640 ÷ 8 we get quotient 80.

Question 43.
5,400 ÷ 6 =
Answer:
5,400 ÷ 6 = 900,

Explanation:
Divided 5,400 ÷ 6 we get quotient 900.

Question 44.
4,000 ÷ 5 =
Answer:
4,000 ÷ 5 = 800,

Explanation:
Divided 4,000 ÷ 5 we get quotient 800.

Divide.

Answer:

Question 1.
4 ÷ 2 =
Answer:
4 ÷ 2 = 2,

Explanation:
Divided 4 ÷ 2 we get quotient 2.

Question 2.
40 ÷ 2 =
Answer:
40 ÷ 2 = 20,

Explanation:
Divided 40 ÷ 2 we get quotient 20.

Question 3.
400 ÷ 2 =
Answer:
400 ÷ 2 = 200,

Explanation:
Divided 400 ÷ 2 we get quotient 200.

Question 4.
4,000 ÷ 2 =
Answer:
4,000 ÷ 2 = 2,000,

Explanation:
Divided 4,000 ÷ 2 we get quotient 2,000.

Question 5.
6 ÷ 3 =
Answer:
6 ÷ 3 = 2,

Explanation:
Divided 6 ÷ 3 we get quotient 2.

Question 6.
60 ÷ 3 =
Answer:
60 ÷ 3 = 20,

Explanation:
Divided 60 ÷ 3 we get quotient 20.

Question 7.
600 ÷ 3 =
Answer:
600 ÷ 3 = 200,

Explanation:
Divided 600 ÷ 3 we get quotient 200.

Question 8.
6,000 ÷ 3 =
Answer:
6,000 ÷ 3 = 2,000,

Explanation:
Divided 6,000 ÷ 3 we get quotient 2,000.

Question 9.
10 ÷ 5 =
Answer:
10 ÷ 5 = 2,

Explanation:
Divided 10 ÷ 5 we get quotient 2.

Question 10.
15 ÷ 5 =
Answer:
15 ÷ 5 = 3,

Explanation:
Divided 15 ÷ 5 we get quotient 3.

Question 11.
150 ÷ 5 =
Answer:
150 ÷ 5 = 30,

Explanation:
Divided 150 ÷ 5 we get quotient 30.

Question 12.
250 ÷ 5 =
Answer:
250 ÷ 5 = 50,

Explanation:
Divided 250 ÷ 5 we get quotient 50.

Question 13.
350 ÷ 5 =
Answer:
350 ÷ 5 = 70,

Explanation:
Divided 350 ÷ 5 we get quotient 70.

Question 14.
3,500 ÷ 5 =
Answer:
3,500 ÷ 5 = 700,

Explanation:
Divided 3,500 ÷ 5 we get quotient 700.

Question 15.
4,500 ÷ 5 =
Answer:
4,500 ÷ 5 = 900,

Explanation:
Divided 4,500 ÷ 5 we get quotient 900.

Question 16.
450 ÷ 5 =
Answer:
450 ÷ 5 = 90,

Explanation:
Divided 450 ÷ 5 we get quotient 90.

Question 17.
9 ÷ 3 =
Answer:
9 ÷ 3 = 3,

Explanation:
Divided 9 ÷ 3 we get quotient 3.

Question 18.
12 ÷ 3 =
Answer:
12 ÷ 3 = 4,

Explanation:
Divided 12 ÷ 3 we get quotient 4.

Question 19.
120 ÷ 3 =
Answer:
120 ÷ 3 = 40,

Explanation:
Divided 120 ÷ 3 we get quotient 40.

Question 20.
1,200 ÷ 3 =
Answer:
1,200 ÷ 3 = 400,

Explanation:
Divided 1,200 ÷ 3 we get quotient 400.

Question 21.
25 ÷ 5 =
Answer:
25 ÷ 5 = 5,

Explanation:
Divided 25 ÷ 5 we get quotient 5.

Question 22.
20 ÷ 5 =
Answer:
20 ÷ 5 = 4,

Explanation:
Divided 20 ÷ 5 we get quotient 4.

Question 23.
200 ÷ 5 =
Answer:
200 ÷ 5 = 40,

Explanation:
Divided 200 ÷ 5 we get quotient 40.

Question 24.
2,000 ÷ 5 =
Answer:
2,000 ÷ 5 = 400,

Explanation:
Divided 2,000 ÷ 5 we get quotient 400.

Question 25.
12 ÷ 4 =
Answer:
12 ÷ 4 = 3,

Explanation:
Divided 12 ÷ 4 we get quotient 3.

Question 26.
120 ÷ 4 =
Answer:
120 ÷ 4 = 30,

Explanation:
Divided 120 ÷ 4 we get quotient 30.

Question 27.
21 ÷ 7 =
Answer:
21 ÷ 7 = 3,

Explanation:
Divided 21 ÷ 7 we get quotient 3.

Question 28.
2,100 ÷ 7 =
Answer:
2,100 ÷ 7 = 300,

Explanation:
Divided 2,100 ÷ 7 we get quotient 300.

Question 29.
18 ÷ 6 =
Answer:
18 ÷ 6 = 3,

Explanation:
Divided 18 ÷ 6 we get quotient 3.

Question 30.
180 ÷ 6 =
Answer:
180 ÷ 6 = 30,

Explanation:
Divided 180 ÷ 6 we get quotient 30.

Question 31.
54 ÷ 9 =
Answer:
54 ÷ 9 = 6,

Explanation:
Divided 54 ÷ 9 we get quotient 6.

Question 32.
5,400 ÷ 9 =
Answer:
5,400 ÷ 9 = 600,

Explanation:
Divided 5,400 ÷ 9 we get quotient 600.

Question 33.
5,600 ÷ 8 =
Answer:
5,600 ÷ 8 = 700,

Explanation:
Divided 5,600 ÷ 8 we get quotient 700.

Question 34.
300 ÷ 5 =
Answer:
300 ÷ 5 = 60,

Explanation:
Divided 300 ÷ 5 we get quotient 60.

Question 35.
490 ÷ 7 =
Answer:
490 ÷ 7 = 70,

Explanation:
Divided 490 ÷ 7 we get quotient 70.

Question 36.
6,300 ÷ 9 =
Answer:
6,300 ÷ 9 = 700,

Explanation:
Divided 6,300 ÷ 9 we get quotient 700.

Question 37.
420 ÷ 6 =
Answer:
420 ÷ 6 = 70,

Explanation:
Divided 420 ÷ 6 we get quotient 70.

Question 38.
4,800 ÷ 8 =
Answer:
4,800 ÷ 8 = 600,

Explanation:
Divided 4,800 ÷ 8 we get quotient 600.

Question 39.
4,000 ÷ 5 =
Answer:
4,000 ÷ 5 = 800,

Explanation:
Divided 4,000 ÷ 5 we get quotient 800.

Question 40.
560 ÷ 8 =
Answer:
560 ÷ 8 = 70,

Explanation:
Divided 560 ÷ 8 we get quotient 70.

Question 41.
6,400 ÷ 8 =
Answer:
6,400 ÷ 8 = 800,

Explanation:
Divided 6,400 ÷ 8 we get quotient 800.

Question 42.
720 ÷ 8 =
Answer:
720 ÷ 8 = 90,

Explanation:
Divided 720 ÷ 8 we get quotient 90.

Question 43.
4,800 ÷ 6 =
Answer:
4,800 ÷ 6 = 800,

Explanation:
Divided 4,800 ÷ 6 we get quotient 800.

Question 44.
400 ÷ 5 =
Answer:
400 ÷ 5 = 80,

Explanation:
Divided 400 ÷ 5 we get quotient 80.

Eureka Math Grade 4 Module 3 Lesson 31 Problem Set Answer Key

Draw a tape diagram and solve. The first two tape diagrams have been drawn for you. Identify if the group size or the number of groups is unknown.

Question 1.
Monique needs exactly 4 plates on each table for the banquet. If she has 312 plates, how many tables is she able to prepare?

Answer:
Monique is able to prepare 78 tables,
The number of groups is unknown,

Explanation:
Given Monique needs exactly 4 plates on each table for the banquet.
If she has 312 plates, number of tables is she able to prepare is
312 plates ÷ 4=
   78   
4|312
   28
   032
032
0
Therefore, Monique is able to prepare 78 tables, the number of groups is unknown.

Question 2.
2,365 books were donated to an elementary school. If 5 classrooms shared the books equally, how many books did each class receive?

Answer:
Each class will receive 473 books,
The group size is unknown,

Explanation:
Given 2,365 books were donated to an elementary school.
If 5 classrooms shared the books equally,
Number of books did each class will receive is 2,365 ÷ 5 =
   473   
5|2,365
   20
     36
     35
       15
       15
 0
Therefore, Each class will receive 473 books,
The group size is unknown.

Question 3.
If 1,503 kilograms of rice was packed in sacks weighing 3 kilograms each, how many sacks were packed?
Answer:
501 sacks were packed,
The number of groups is unknown,

Explanation:

Given
If 1,503 kilograms of rice was packed in sacks weighing 3 kilograms each, number of sacks were packed are 1,503 ÷ 3 = 501
as shown above, The number of groups is unknown.

Question 4.
Rita made 5 batches of cookies. There was a total of 2,400 cookies. If each batch contained the same number of cookies, how many cookies were in 4 batches?
Answer:
There were 1,920 cookies in 4 batches,
the group size is unknown,

Explanation:

Given Rita made 5 batches of cookies.
There was a total of 2,400 cookies.
If each batch contained the same number of cookies,
So number of cookies were in 4 batches are 1,920,
the group size is unknown.

Question 5.
Every day, Sarah drives the same distance to work and back home. If Sarah drove 1,005 miles in 5 days, how far did Sarah drive in 3 days?
Answer:
Sarah drove 603 miles in 3 days,
the group size is unknown,

Explanation:

Given every day, Sarah drives the same distance to work and back home.
If Sarah drove 1,005 miles in 5 days, So Sarah drive in 3 days is 603 miles,
the group size is unknown.

Eureka Math Grade 4 Module 3 Lesson Exit Ticket Answer Key

Solve the following problems. Draw tape diagrams to help you solve. Identify if the group size or the number of groups is unknown.

Question 1.
572 cars were parked in a parking garage. The same number of cars was parked on each floor. If there were 4 floors, how many cars were parked on each floor?
Answer:
Number of cars were parked on each floor is 143 cars,
the group size is unknown,

Explanation:

Given 572 cars were parked in a parking garage.
The same number of carscwas parked on each floor.
If there were 4 floors, number of cars were parked on each floor are 143 cars as shown above,the group size is unknown.

Question 2.
356 kilograms of flour were packed into sacks holding 2 kilograms each. How many sacks were packed?
Answer:
178 sacks were packed, the number of groups is unknown,

Explanation:

Given 356 kilograms of flour were packed into sacks holding 2 kilograms each. Number of sacks were packed are 356 ÷ 2 = 178 sacks,
the number of groups is unknown.

Eureka Math Grade 4 Module 3 Lesson Homework Answer Key

Solve the following problems. Draw tape diagrams to help you solve. Identify if the group size or the number of groups is unknown.

Question 1.
500 milliliters of juice was shared equally by 4 children. How many milliliters of juice did each child get?
Answer:
Each child will get 125 milliliters of juice, the group size is unknown,

Explanation:
Given 500 milliliters of juice was shared equally by 4 children.
So number of milliliters of juice did each child will get is
125 milliliters as shown above, the group size is unknown.

Question 2.
Kelly separated 618 cookies into baggies. Each baggie contained 3 cookies. How many baggies of cookies did Kelly make?
Answer:
Kelly will make 206 baggies of cookies, the number of groups is unknown,

Explanation:
Given Kelly separated 618 cookies into baggies.
Each baggie contained 3 cookies.
Number of baggies of cookies did Kelly make are 206 baggies of cookies, the number of groups is unknown.

Question 3.
Jeff biked the same distance each day for 5 days. If he traveled 350 miles altogether, how many miles did he travel each day?
Answer:
Each day Jeff traveled 70 miles, the group size is unknown,

Explanation:
Given Jeff biked the same distance each day for 5 days. If he traveled 350 miles altogether, Number of miles did Jeff traveled each day is 70 miles, the group size is unknown.

Question 4.
A piece of ribbon 876 inches long was cut by a machine into 4-inch long strips to be made into bows. How many strips were cut?
Answer:
219 strips were cut, the number of groups is unknown,

Explanation:
Given a piece of ribbon 876 inches long was cut by a machine into 4-inch long strips to be made into bows. Number of strips were cut are 219 the number of groups is unknown.

Question 5.
Five Martians equally share 1,940 Groblarx fruits. How many Groblarx fruits will 3 of the Martians receive?
Answer:
1,164 Groblarx fruits will 3 of the Martians receive,
the group size is unknown,

Explanation:
Given five Martians equally share 1,940 Groblarx fruits.
So Groblarx fruits will 3 of the Martians receive are 1,164 as shown above, the group size is unknown.

Place value charts are the important topics in maths. This will be helpful in the real-time environment. Know-how and where to apply the formulas from this page. Get a detailed explanation for all the questions here. Download Eureka Math Answers Grade 2 chapter 11 pdf for free of cost. As per your convenience, we have provided the solutions in pdf format so that you can prepare offline.

Engage NY Eureka Math 2nd Grade Module 4 Lesson 11 Answer Key

Get the guided notes for the chapter 11 Answer Key from here. This will be the best resource to enhance your math skills. The topics covered in this chapter are Place value charts. test yourself by solving questions given at the end of the chapter.

Eureka Math Grade 2 Module 4 Lesson 11 Problem Set Answer Key

Question 1.
Solve using mental math.
a. 8 – 7 = ___1__                 38 – 7 = __31___                 38 – 8 = __30____                 38 – 9 = ___29___

Answer:
8 – 7 = 1.
38 – 7 = 31.
38 – 8 = 30.
38 – 9 = 29.

Explanation:
In the above-given question,
given that,
solve using mental math.
8 – 7 = 1.
38 – 7 = 31.
38 – 8 = 30.
38 – 9 = 29.

b. 7 – 6 = _1____                 87 – 6 = __81___                 87 – 7 = ___80___                 87 – 8 = __79____

Answer:
7 – 6 = 1.
87 – 6 = 81.
87 – 7 = 80.
87 – 8 = 79.

Explanation:
In the above-given question,
given that,
solve using mental math.
7 – 6 = 1.
87 – 6 = 81.
87 – 7 = 80.
87 – 8 = 79.

Question 2.
Solve using your place value chart and place value disks. Unbundle a ten if needed. Think about which problems you can solve mentally, too!
a. 28 – 7 = __21___                  28 – 9 = ___19___

Answer:
28 – 7 = 21.
28 – 9 = 19.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
28 – 7 = 21.
28 – 9 = 19.

b. 25 – 5 = __20___                  25 – 6 = ___19___

Answer:
25 – 5 = 20.
25 – 6 = 19.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
25 – 5 = 20.
25 – 6 = 19.

c. 30 – 5 = __25___                  33 – 5 = __28____

Answer:
30 – 5 = 25.
33 – 5 = 28.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
30 – 5 = 25.
33 – 5 = 28.

d. 47 – 22 = _25____                41 – 22 = __19___

Answer:
47 – 22 = 25.
41 – 22 = 19.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
47 – 22 = 25.
41 – 22 = 19.

e. 44 – 16 = __28___                44 – 26 = __18___

Answer:
44 – 16 = 28.
44 – 26 = 18.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
44 – 16 = 28.
44 – 26 = 18.

f. 70 – 28 = __42___                80 – 28 = __52___

Answer:
70 – 28 = 42.
80 – 28 = 52.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
70 – 28 = 42.
80 – 28 = 52.

Question 3.
Solve 56 – 28, and explain your strategy.

Answer:
56 – 28 = 28.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
56 – 28 = 28.

For early finishers:

Question 4.
There are 63 problems on the math test. Tamara answered 48 problems correctly, but the rest were incorrect. How many problems did she answer incorrectly?

Answer:
The number of problems did she answer incorrectly = 15.

Explanation:
In the above-given question,
given that,
There are 63 problems on the math test.
Tamara answered 48 problems correctly, but the rest were incorrect.
63 – 48 = 15.
so the number of problems did she answer incorrectly = 15.
Question 5.
Mr. Ross has 7 fewer students than Mrs. Jordan. Mr. Ross has 35 students. How many students does Mrs. Jordan have?

Answer:
The number of students Mrs. Jordan have = 42.

Explanation:
In the above-given question,
given that,
Mr. Ross has 7 fewer students than Mrs. Jordan.
Mr. Ross has 35 students.
35 + 7 = 42.
so the number of students Mrs. Jordan have = 42.

Eureka Math Grade 2 Module 4 Lesson 11 Exit Ticket Answer Key

Solve for the missing part. Use your place value chart and place value disks.

Question 1.

Answer:
71 – 56 = 15.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
56 + 15 = 71.
71 – 15 = 56.

Question 2.

Answer:
84 – 38 = 46.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
46 + 38 = 84.
84 – 38 = 46.

Eureka Math Grade 2 Module 4 Lesson 11 Homework Answer Key

Question 1.
Solve using mental math.
a. 6 – 5 = ___1__                 26 – 5 = _21____                 26 – 6 = ___20___                 26 – 7 = __19____

Answer:
6 – 5 = 1.
26 – 5 = 21.
26 – 6 = 20.
26 – 7 = 19.

Explanation:
In the above-given question,
given that,
solve using mental math.
6 – 5 = 1.
26 – 5 = 21.
26 – 6 = 20.
26 – 7 = 19.

b. 8 – 7 = ___1__                 58 – 7 = __51___                 58 – 8 = __50____                 58 – 9 = __49____

Answer:
8 – 7 = 1.
58 – 7 = 51.
58 – 8 = 50.
58 – 9 = 49.

Explanation:
In the above-given question,
given that,
solve using mental math.
8 – 7 = 1.
58 – 7 = 51.
58 – 8 = 50.
58 – 9 = 49.

Question 2.
Solve using your place value chart and place value disks. Unbundle a ten, if needed. Think about which problems you can solve mentally, too!
a. 36 – 5 = __31___                 36 – 7 = __29___

Answer:
36 – 5 = 31.
36 – 7 = 29.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
36 – 5 = 31.
36 – 7 = 29.

b. 37 – 6 = ___31__                 37 – 8 = ___29__

Answer:
37 – 6 = 31.
37 – 8 = 29.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
37 – 6 = 31.
37 – 8 = 29.

c. 40 – 5 = __35___                 41 – 5 = __36___

Answer:
40 – 5 = 35.
41 – 5 = 36.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
40 – 5 = 35.
41 – 5 = 36.

d. 58 – 32 = __26___               58 – 29 = __29___

Answer:
58 – 32 = 26.
58 – 29 = 29.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
58 – 32 = 26.
58 – 29 = 29.

e. 60 – 26 = __34___                62 – 26 = _36____

Answer:
60 – 26 = 34.
62 – 26 = 36.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
60 – 26 = 34.
62 – 26 = 36.

f. 70 – 41 = __29___ 80 – 41 = __39___

Answer:
70 – 41 = 29.
80 – 41 = 39.

Explanation:
In the above-given question,
given that,
solve using place value chart and place value disks.
70 – 41 = 29.
80 – 41 = 39.

Question 3.
Solve and explain your strategy.
a. 41 – 27 = __14___

Answer:
41 – 27 = 14.

Explanation:
In the above-given question,
given that,
solve using mental math.
41 – 27 = 14.
14 + 27 = 41.

b. 67 – 28 = __39___

Answer:
67 – 28 = 39.

Explanation:
In the above-given question,
given that,
solve using mental math.
67 – 28 = 39.
39 + 28 = 67.

Question 4.
The number of marbles in each jar is marked on the front. Miss Clark took 37 marbles out of each jar. How many marbles are left in each jar? Complete the number sentence to find out.
a.

___45__ – _37____ = __8___

Answer:
45 – 37 = 8.

Explanation:
In the above-given question,
given that,
The number of marbles in each jar is marked on the front.
Miss Clark took 37 marbles out of each jar.
45 – 37 = 8.

b.

___52__ – __37___ = __15___

Answer:
52 -37 = 15.

Explanation:
In the above-given question,
given that,
The number of marbles in each jar is marked on the front.
Miss Clark took 37 marbles out of each jar.
52 – 37 = 15.

c.

__48___ – __37___ = ___11__

Answer:
48 – 37 = 11.

Explanation:
In the above-given question,
given that,
The number of marbles in each jar is marked on the front.
Miss Clark took 37 marbles out of each jar.
48 – 37 = 11.

d.

___55__ – __37___ = __18___

Answer:
55 – 37 = 18.

Explanation:
In the above-given question,
given that,
The number of marbles in each jar is marked on the front.
Miss Clark took 37 marbles out of each jar.
55 – 37 = 18.

Engage NY Eureka Math 1st Grade Module 2 Lesson 2 Answer Key

Eureka Math Grade 1 Module 2 Lesson 2 Problem Set Answer Key

the numbers that make ten. Draw a picture. Complete the number sentence.

Question 1.

Answer:

10 + 4 = 14
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are seven and three as we can observe in the above image. Draw a circles for seven and three. By adding seven with three results ten. ADD ten with four which results fourteen.

Question 2.
9 + 1 + 4 = ☐

Answer:

9 + 1 + 4 = 14
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are nine and one as we can observe in the above image. Draw a circles for nine and one. By adding nine with one results ten. ADD ten with four which results fourteen.

Question 3.
5 + 6 + 5 = ☐

Answer:

5 + 5+ 6 = 16
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are five and five as we can observe in the above image. Draw a circles for five and five . By adding five with five results ten. ADD ten with six which results sixteen.

Question 4.
4 + 3 + 7 = ☐

Answer:

4 + 3+ 7 = 14
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are three and seven as we can observe in the above image. Draw a circles for three and seven. By adding three with seven results ten. ADD ten with four which results fourteen.

Question 5.
2 + 7 + 8 = ☐

Answer:

2 + 7 + 8 = 17
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are two and eight as we can observe in the above image. Draw a circles for two and eight. By adding two with eight results ten. ADD ten with seven which results seventeen.

the numbers that make ten. Put them into a number bond, and solve.

Question 6.

Answer:

Explanation:
A number bond is a simple addition of two numbers that add up to give the sum. The numbers that makes ten are nine and one as we can observe in the above image. Draw a circles for nine and one. By adding nine with one results ten. ADD ten with five which results fifteen.

Question 7.
8 + 2 + 4 = ____
Answer:

8  +  2  +  4  =  14
Explanation:
A number bond is a simple addition of two numbers that add up to give the sum. The numbers that makes ten are eight and two as we can observe in the above image. Draw a circles for eight and two. By adding eight with two results ten. ADD ten with four which results fourteen.

Question 8.
3 + 5 + 5 = ____
Answer:

3   +   5   +   5   =   13
Explanation:
A number bond is a simple addition of two numbers that add up to give the sum. The numbers that makes ten are five and five as we can observe in the above image. Draw a circles for five and five. By adding five with five results ten. ADD ten with three which results thirteen.

Question 9.
3 + 6 + 7 = ____
Answer:

3  +  6  +  7  =  16
Explanation:
A number bond is a simple addition of two numbers that add up to give the sum. The numbers that makes ten are three and seven as we can observe in the above image. Draw a circles for three and seven. By adding three with seven results ten. ADD ten with six which results sixteen.

Eureka Math Grade 1 Module 2 Lesson 2 Exit Ticket Answer Key

the numbers that make ten.
Draw a picture, and complete the number sentences to solve.

a. 8 + 2 + 3 = ____
____ + ____ = ____
10 + ____ = ____
Answer:

8  +   2  +  3  =  13
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are eight and two as we can observe in the above image. Draw a circles for eight and two. By adding eight with two results ten. ADD ten with three which results thirteen.

b. 7 + 4 + 3 = ____
____ + ____ = ____
10 + ____ = ____
Answer:

7  +  4  +  3  =  14
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are seven and three as we can observe in the above image. Draw a circles for seven and three. By adding seven with three results ten. ADD ten with four which results fourteen.

Eureka Math Grade 1 Module 2 Lesson 2 Homework Answer Key

the numbers that make ten. Draw a picture. Complete the number sentence.

Question 1.

Answer:

6  +  2  +  4  =  12
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are six and four as we can observe in the above image. Draw a circles for six and four. By adding six with four results ten. ADD ten with two which results twelve.

Question 2.
5 + 3 + 5 = ☐

Answer:

5 + 3 + 5 = 13
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are five and five as we can observe in the above image. Draw a circles for five and five. By adding five with five results ten. ADD ten with three which results thirteen.

Question 3.
5 + 2 + 8 = ☐

Answer:

5 + 2 + 8 = 15
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are two and eight as we can observe in the above image. Draw a circles for two and eight. By adding two with eight results ten. ADD five with ten which results fifteen.

Question 4.
2 + 7 + 3 = ☐

Answer:

2 + 7 + 3 = 12
Explanation:
An addition sentence is a mathematical expression that shows two or more values added together and their sum. The numbers that makes ten are seven and three as we can observe in the above image. Draw a circles for seven and three. By adding seven with three results ten. ADD two with ten which results twelve.

the numbers that make ten, and put them into a number bond. Write a new number sentence.

Question 5.

Answer:

A new number sentence is 10 + 5 = 15
Explanation:
A number bond is a simple addition of two numbers that add up to give the sum. The numbers that makes ten are three and seven as we can observe in the above image. Draw a circles for three and seven. By adding three with seven results ten. ADD ten with five which results fifteen. The new number sentence is 10 + 5 = 15.

Question 6.

Answer:

A new number sentence is 4 + 10 = 14.
Explanation:
A number bond is a simple addition of two numbers that add up to give the sum. The numbers that makes ten are eight and two as we can observe in the above image. Draw a circles for eight and two. By adding eight with two results ten. ADD four with ten which results fourteen. The new number sentence is 4 + 10 = 14.

Challenge: the addends that make ten. the true number sentences.

a.
Answer:

Explanation:
The addends that make ten are five and five. Draw a circle for two addends that make ten. The number sentence is true as we can observe in the above image. The true number sentence is 10 + 3.

b. 4 + 6 + 6 = 10 + 6
Answer:

Explanation:
The addends that make ten are four and six. Draw a circle for two addends that make ten. The number sentence is true as we can observe in the above image. The true number sentence is 10 + 6. So make a circle for 10 + 6 number sentence.

c. 3 + 8 + 7 = 10 + 6
Answer:

Explanation:
The addends that make ten are three and seven. Draw a circle for two addends that make ten. The number sentence is false as we can observe in the above image. The number sentence 10 + 6 is not a correct number sentence. The correct number sentence is
10 + 8. So don’t make circle for the number sentence as shown in the above image.

d. 8 + 9 + 2 = 9 + 10
Answer:

Explanation:
The addends that make ten are eight and two. Draw a circle for two addends that make ten. The number sentence is true as we can observe in the above image. The true number sentence is 9 + 10. So make a circle for 9 + 10 number sentence.

 

Engage NY Eureka Math Geometry Module 5 Lesson 5 Answer Key

Eureka Math Geometry Module 5 Lesson 5 Example Answer Key

Example 1.
A and C are points on a circle with center O.

a. What is the intercepted arc of ∠COA? Color it red.
Answer:
\(\widehat{A C}\)

b. Draw triangle AOC. What type of triangle is it? Why?
Answer:
An isosceles triangle because OC = OA (they are radii of the same circle).

c. What can you conclude about m∠OCA and m∠OAC? Why?
Answer:
They are equal because base angles of an isosceles triangle are equal in measure.

d. Draw a point B on the circle so that O is in the interior of the inscribed angle ABC.
Answer:
The diagram should resemble the inside case of the discussion diagrams.

e. What is the intercepted arc of ∠ABC? Color it green.
Answer:
\(\widehat{A C}\)

f. What do you notice about \(\widehat{A C}\)?
Answer:
It is the same arc that was intercepted by the central angle.

g. Let the measure of ∠ABC be x and the measure of ∠AOC be y. Can you prove that y = 2x? (Hint: Draw the diameter that contains point B.)
Answer:
Let \(\overline{B D}\) be a diameter. Let x₁, y₁, x₂, and y₂ be the measures of ∠CBD, ∠COD, ∠ABD, and ∠AOD, respectively. We can express x and y in terms of these measures: x = x₁ + x₂, and y = y₁ + y₂. By the Opening Exercise, y₁ = 2x₁, and y₂ = 2x₂. Thus, y = 2x.

h. Does your conclusion support the inscribed angle theorem?
Answer:
Yes, even when the center of the circle is in the interior of the inscribed angle, the measure of the inscribed angle is equal to half the measure of the central angle that intercepts the same arc.

i. If we combine the Opening Exercise and this proof, have we finished proving the inscribed angle theorem?
Answer:
No. We still have to prove the case where the center is outside the inscribed angle.

Example 2.
A and C are points on a circle with center O.

a. Draw a point B on the circle so that O is in the exterior of the inscribed angle ABC.
Answer:
The diagram should resemble the outside case of the discussion diagrams.

b. What is the intercepted arc of ∠ABC? Color it yellow.
Answer:
\(\widehat{A C}\)

c. Let the measure of ∠ABC be x and the measure of ∠AOC be y. Can you prove that y = 2x? (Hint: Draw the diameter that contains point B.)
Answer:
Let \(\overline{B D}\) be a diameter. Let x₁, y₁, x₂, and y₂ be the measures of ∠CBD, ∠COD, ∠ABD, and ∠AOD, respectively. We can express x and y in terms of these measures: x = x₂ – x₁ and y = y₂ – y₁. By the Opening Exercise, y₁ = 2x₁ and y₂ = 2x₂. Thus, y = 2x.

d. Does your conclusion support the inscribed angle theorem?
Answer:
Yes, even when the center of the circle is in the exterior of the inscribed angle, the measure of the inscribed angle is equal to half the measure of the central angle that intercepts the same arc.

e. Have we finished proving the inscribed angle theorem?
Answer:
We have shown all cases of the inscribed angle theorem (central angle version). We do have one more case to study in Lesson 7, but it is ok not to mention it here. The last case is when the location of B is on the minor arc between A and C.

Eureka Math Geometry Module 5 Lesson 5 Exercise Answer Key

Opening Exercise
a. A and C are points on a circle with center O.

i. Draw a point B on the circle so that \(\overline{A B}\) is a diameter. Then draw the angle ABC.

ii. What angle in your diagram is an inscribed angle?
Answer:
∠ABC

iii. What angle in your diagram is a central angle?
Answer:
∠AOC

iv. What is the intercepted arc of ∠ABC?
Answer:
\(\widehat{A C}\)

v. What is the intercepted arc of ∠AOC ?
Answer:
\(\widehat{A C}\)

b. The measure of the inscribed angle ACD is x, and the measure of the central angle CAB is y. Find m∠CAB in terms of x.

Answer:
We are given that m∠ACD is x. We know that AB = AC = AD, so △CAD is an isosceles triangle, which means that m∠ADC is also x. The sum of the angles of a triangle is 180˚, so m∠CAD = 180˚ – 2x. ∠CAD and ∠CAB are supplementary meaning that m∠CAB = 180˚ – (180˚ – 2x) = 2x; therefore, y = 2x.

Exercises

Exercise 1.
Find the measure of the angle with measure x. Diagrams are not drawn to scale.
a. m∠D = 25°

Answer:
50°

b. m∠D = 15°

Answer:
15°

c. m∠BAC = 90°

Answer:
45°

d. m∠B = 32°

Answer:
58°

e. BD = AB

Answer:
x = w = 60°

f. m∠D = 19°

Answer:
71°

Exercise 2.
Toby says △BEA is a right triangle because m∠BEA = 90°. Is he correct? Justify your answer.

Answer:
Toby is not correct. m∠BEA = 95°. ∠BCD is inscribed in the same arc as the central angle, so it has a measure of 35°. This means that m∠DEC = 95° because the sum of the angles of a triangle is 180°. m∠BEA = m∠DEC since they are vertical angles, so the triangle is not right.

Exercise 3.
Let’s look at relationships between inscribed angles.
a. Examine the inscribed polygon below. Express x in terms of y and y in terms of x. Are the opposite angles in any quadrilateral inscribed in a circle supplementary? Explain.

Answer:
x = 180˚ – y; y = 180˚ – x. The angles are supplementary.

b. Examine the diagram below. How many angles have the same measure, and what are their measures in terms of x˚?

Answer:
Let C and D be the points on the circle that the original angles contain. All the angles intercepting the minor arc between C and D have measure x˚, and the angles intercepting the major arc between C and D measure 180° – x.

Exercise 4.
Find the measures of the labeled angles.
a.

Answer:
x = 28, y = 50

b.

Answer:
y = 48

c.

Answer:
x = 32

d.

Answer:
x = 36, y = 120

e.

Answer:
x = 40

f.

Answer:
x = 30

Eureka Math Geometry Module 5 Lesson 5 Problem Set Answer Key

For Problems 1–8, find the value of x. Diagrams are not drawn to scale.
Question 1.

Answer:
x = 34

Question 2.

Answer:
x = 94

Question 3.

Answer:
x = 30

Question 4.

Answer:
x = 70

Question 5.

Answer:
x = 60

Question 6.

Answer:
x = 60

Question 7.

Answer:
x = 20

Question 8.

Answer:
x = 46

Question 9.
a. The two circles shown intersect at E and F. The center of the larger circle, D, lies on the circumference of the smaller circle. If a chord of the larger circle, (FG) ̅, cuts the smaller circle at H, find x and y.

Answer:
x = 100; y = 50

b. How does this problem confirm the inscribed angle theorem?
Answer:
∠FDE is a central angle of the larger circle and is double ∠FGE, the inscribed angle of the larger circle. ∠FDE is inscribed in the smaller circle and equal in measure to ∠FHE, which is also inscribed in the smaller circle.

Question 10.
In the figure below, \(\overline{E D}\) and \(\overline{B C}\) intersect at point F.
Prove: m∠DAB + m∠EAC = 2(m∠BFD)

Proof:
Join \(\overline{B E}\).

m∠BED = \(\frac{1}{2}\)(m∠___________)

m∠EBC = \(\frac{1}{2}\)(m∠___________)

In △EBF,
m∠BEF + m∠EBF = m∠___________

\(\frac{1}{2}\)(m∠___________) + \(\frac{1}{2}\)(m∠___________) = m∠___________
∴ m∠DAB + m∠EAC = 2(m∠BFD)
Answer:
BAD; EAC; BFD; DAB, EAC, BFD

Eureka Math Geometry Module 5 Lesson 5 Exit Ticket Answer Key

Question 1.
The center of the circle below is O. If angle B has a measure of 15 degrees, find the values of x and y. Explain how you know.

Answer:
y = 15. Triangle COB is isosceles, so base angles ∠OCB and ∠OBC are congruent. m∠OBC = 15˚ = m∠OCB.
x = 30. ∠COA is a central angle inscribed in the same arc as inscribed ∠CBA. So, m∠COA = 2(m∠CBA).

Engage NY Eureka Math 8th Grade Module 4 Lesson 3 Answer Key

Eureka Math Grade 8 Module 4 Lesson 3 Exercise Answer Key

Exercise 1.
Is the equation a true statement when x=-3? In other words, is -3 a solution to the equation 6x+5=5x+8+2x? Explain.
Answer:
If we replace x with the number -3, then the left side of the equation is
6∙(-3)+5=-18+5
=-13″,”
and the right side of the equation is
5∙(-3)+8+2∙(-3)=-15+8-6
=-7-6
=-13.
Since -13=-13, then x=-3 is a solution to the equation 6x+5=5x+8+2x.
Note: Some students may have transformed the equation.

Exercise 2.
Does x=12 satisfy the equation 16-\(\frac{1}{2}\) x=\(\frac{3}{4}\) x+1? Explain.
Answer:
If we replace x with the number 12, then the left side of the equation is
16-\(\frac{1}{2}\) x=16-\(\frac{1}{2}\)∙(12)
=16-6
=10,
and the right side of the equation is
\(\frac{3}{4}\) x+1=\(\frac{3}{4}\)∙(12)+1
=9+1
=10.
Since 10=10, then x=12 is a solution to the equation 16-\(\frac{1}{2}\) x=\(\frac{3}{4}\) x+1.

Exercise 3.
Chad solved the equation 24x+4+2x=3(10x-1) and is claiming that x=2 makes the equation true. Is Chad correct? Explain.
Answer:
If we replace x with the number 2, then the left side of the equation is
24x+4+2x=24∙2+4+2∙2
=48+4+4
=56,
and the right side of the equation is
3(10x-1)=3(10∙2-1)
=3(20-1)
=3(19)
=57.
Since 56≠57, then x=2 is not a solution to the equation 24x+4+2x=3(10x-1), and Chad is not correct.

Exercise 4.
Lisa solved the equation x+6=8+7x and claimed that the solution is x=-\(\frac{1}{3}\). Is she correct? Explain.
Answer:
If we replace x with the number –\(\frac{1}{3}\), then the left side of the equation is
x+6=-\(\frac{1}{3}\)+6
=5 \(\frac{2}{3}\),
and the right side of the equation is
8+7x=8+7∙(-\(\frac{1}{3}\))
=8-\(\frac{7}{3}\)
=\(\frac{24}{3}\)–\(\frac{7}{3}\)
=\(\frac{17}{3}\).
Since 5 \(\frac{2}{3}\)=\(\frac{17}{3}\), then x=-\(\frac{1}{3}\) is a solution to the equation x+6=8+7x, and Lisa is correct.

Exercise 5.
Angel transformed the following equation from 6x+4-x=2(x+1) to 10=2(x+1). He then stated that the solution to the equation is x=4. Is he correct? Explain.
Answer:
No, Angel is not correct. He did not transform the equation correctly. The expression on the left side of the equation 6x+4-x=2(x+1) would transform to
6x+4-x=6x-x+4
=(6-1)x+4
=5x+4.
If we replace x with the number 4, then the left side of the equation is
5x+4=5∙4+4
=20+4
=24,
and the right side of the equation is
2(x+1)=2(4+1)
=2(5)
=10.
Since 24≠10, then x=4 is not a solution to the equation 6x+4-x=2(x+1), and Angel is not correct.

Exercise 6.
Claire was able to verify that x=3 was a solution to her teacher’s linear equation, but the equation got erased from the board. What might the equation have been? Identify as many equations as you can with a solution of x=3.
Answer;
Answers will vary. Ask students to share their equations and justifications as to how they knew x=3 would make a true number sentence.

Exercise 7.
Does an equation always have a solution? Could you come up with an equation that does not have a solution?
Answer:
Answers will vary. Expect students to write equations that are false. Ask students to share their equations and justifications as to how they knew the equation they wrote did not have a solution. The concept of “no solution” is introduced in Lesson 6 and solidified in Lesson 7.

Eureka Math Grade 8 Module 4 Lesson 3 Problem Set Answer Key

Students practice determining whether or not a given number is a solution to the linear equation.

Question 1.
Given that 2x+7=27 and 3x+1=28, does 2x+7=3x+1? Explain.
Answer:
No, because a linear equation is a statement about equality. We are given that 2x+7=27, but 3x+1=28. Since each linear expression is equal to a different number, 2x+7≠3x+1.

Question 2.
Is -5 a solution to the equation 6x+5=5x+8+2x? Explain.
Answer:
If we replace x with the number -5, then the left side of the equation is
6∙(-5)+5=-30+5
=-25,
and the right side of the equation is
5∙(-5)+8+2∙(-5)=-25+8-10
=-17-10
=-27.
Since -25≠-27, then -5 is not a solution of the equation 6x+5=5x+8+2x.
Note: Some students may have transformed the equation.

Question 3.
Does x=1.6 satisfy the equation 6-4x=-\(\frac{x}{4}\)? Explain.
Answer:
If we replace x with the number 1.6, then the left side of the equation is
6-4∙1.6=6-6.4
=-0.4,
and the right side of the equation is
–\(\frac{-1.6}{4}\)=-0.4.
Since -0.4=-0.4, then x=1.6 is a solution of the equation 6-4x=-\(\frac{x}{4}\).

Question 4.
Use the linear equation 3(x+1)=3x+3 to answer parts (a)–(d).
a. Does x=5 satisfy the equation above? Explain.
Answer:
If we replace x with the number 5, then the left side of the equation is
3(5+1)=3(6)
=18,
and the right side of the equation is
3x+3=3∙5+3
=15+3
=18.
Since 18=18, then x=5 is a solution of the equation 3(x+1)=3x+3.

b. Is x=-8 a solution of the equation above? Explain.
Answer:
If we replace x with the number -8, then the left side of the equation is
3(-8+1)=3(-7)
=-21,
and the right side of the equation is
3x+3=3∙(-8)+3
=-24+3
=-21.
Since -21=-21, then x=-8 is a solution of the equation 3(x+1)=3x+3.

c. Is x=\(\frac{1}{2}\) a solution of the equation above? Explain.
Answer:
If we replace x with the number \(\frac{1}{2}\), then the left side of the equation is
3(\(\frac{1}{2}\)+1)=3(\(\frac{1}{2}\)+\(\frac{2}{2}\))
=3(\(\frac{3}{2}\))
=\(\frac{9}{2}\),
and the right side of the equation is
3x+3=3∙(\(\frac{1}{2}\))+3
= \(\frac{3}{2}\)+3
=\(\frac{3}{2}\)+\(\frac{6}{2}\)
=\(\frac{9}{2}\).
Since \(\frac{9}{2}\)=\(\frac{9}{2}\), then x=\(\frac{1}{2}\) is a solution of the equation 3(x+1)=3x+3.

d. What interesting fact about the equation 3(x+1)=3x+3 is illuminated by the answers to parts (a), (b), and (c)? Why do you think this is true?
Answer:
Note to teacher: Ideally, students will notice that the equation 3(x+1)=3x+3 is an identity under the distributive law. The purpose of this problem is to prepare students for the idea that linear equations can have more than one solution, which is a topic of Lesson 7.

Eureka Math Grade 8 Module 4 Lesson 3 Exit Ticket Answer Key

Question 1.
Is 8 a solution to \(\frac{1}{2}\) x+9=13? Explain.
Answer:
If we replace x with the number 8, then the left side is \(\frac{1}{2}\) (8)+9=4+9=13, and the right side is 13. Since 13=13, then x=8 is a solution.

Question 2.
Write three different equations that have x=5 as a solution.
Answer:
Answers will vary. Accept equations where x=5 makes a true number sentence.

Question 3.
Is -3 a solution to the equation 3x-5=4+2x? Explain.
Answer:
If we replace x with the number -3, then the left side is 3(-3)-5=-9-5=-14. The right side is 4+2(-3)=4-6=-2. Since -14≠-2, then -3 is not a solution of the equation.

Engage NY Eureka Math 6th Grade Module 1 Lesson 4 Answer Key

Eureka Math Grade 6 Module 1 Lesson 4 Example Answer Key

Example 1.
The morning announcements said that two out of every seven sixth-grade students In the school have an overdue library book. Jasmine said, “That would mean 24 of us have overdue books!” Grace argued, “No way. That is way too high.” How can you determine who is right?
Answer:
You would have to know the total number of sixth-grade students, and then see if the ratio 24: total is equivalent to 2: 7.

Answer:

Eureka Math Grade 6 Module 1 Lesson 4 Exercise Answer Key

Exercise 1.
Decide whether or not each of the following pairs of ratios is equivalent.
→ If the ratios are not equivalent, find a ratio that is equivalent to the first ratio.
→ If the ratios are equivalent, identify the nonzero number, c, that could be used to multiply each number of the first ratio by in order to get the numbers for the second ratio.
a. 6: 11 and 42: 88
________ Yes, the value, c, is ________
________ No, an equivalent ratio would be ________
Answer:

________ Yes, the value, c, is ________
  x    No, an equivalent ratio would be    42: 77   
Answer:

b. 0: 5 and 0: 20
________ Yes, the value, c, is ________
________ No, an equivalent ratio would be ________
Answer:

  x _  Yes, the value, c, is   4   
________ No, an equivalent ratio would be ________

Exercise 2.
In a bag of mixed walnuts and cashews, the ratio of the number of walnuts to the number of cashews is 5: 6. Determine the number of walnuts that are in the bag if there are 54 cashews. Use a tape diagram to support your work. Justify your answer by showing that the new ratio you created of the number of walnuts to the number of cashews is equivalent to 5: 6.
Answer:

54 divided by 6 equals 9.
5 times 9 equals 45.
There are 45 walnuts in the bag.
The ratio of the number of walnuts to the number of cashews is 45: 54. That ratio is equivalent to 5: 6.

Answer:

Eureka Math Grade 6 Module 1 Lesson 4 Problem Set Answer Key

Question 1.
Use diagrams or the description of equivalent ratios to show that the ratios 2: 3, 4: 6, and 8: 12 are equivalent.
Answer:

Question 2.
Prove that 3: 8 is equivalent to 12: 32.
a. Use diagrams to support your answer.
Answer:

b. Use the description of equivalent ratios to support your answer.
Answer:
Answers will vary. Descriptions should include multiplicative comparisons, such as 12 is 3 times 4 and 32 is 8 times 4. The constant number, c, is 4.

Question 3.
The ratio of Isabella’s money to Shane’s money is 3: 11. If Isabella has $33, how much money do Shane and Isabella have together? Use diagrams to illustrate your answer.
Answer:
Isabella has $33, and Shane has $121. $33 + $121 = $154. Together, Isabella and Shane have $154. 00.

Eureka Math Grade 6 Module 1 Lesson 4 Exit Ticket Answer Key

Question 1.
There are 35 boys in the sixth grade. The number of girls in the sixth grade is 42. Lonnie says that means the ratio of the number of boys in the sixth grade to the number of girls in sixth grade is 5: 7. Is Lonnie correct? Show why or why not.
Answer:
No, Lonnie is not correct. The ratios 5:7 and 35:42 are not equivalent. They are not equivalent because 5 × 7 = 35, but 7 × 7 = 49, not 42.

Engage NY Eureka Math 6th Grade Module 1 Lesson 3 Answer Key

Eureka Math Grade 6 Module 1 Lesson 3 Exercise Answer Key

Exercise 1.
Write a one-sentence story problem about a ratio.
Answer:
Answers will vary. The ratio of the number of sunny days to the number of cloudy days in this town is 3: 1.

Write the ratio in two different forms.
Answer:
3: 1 and 3 to 1

Exercise 2.
Shanni and Mel are using ribbon to decorate a project in their art class. The ratio of the length of Shanni’s ribbon to the length of Mel’s ribbon is 7: 3.
Draw a tape diagram to represent this ratio.
Answer:

Exercise 3.
Mason and Laney ran laps to train for the long-distance running team. The ratio of the number of laps Mason ran to the number of laps Laney ran was 2 to 3.
a. If Mason ran 4 miles, how far did Laney run? Draw a tape diagram to demonstrate how you found the answer.
Answer:

b. If Laney ran 930 meters, how far did Mason run? Draw a tape diagram to determine how you found the answer.
Answer:

c. What ratios can we say are equivalent to 2: 3?
Answer:
4: 6 and 620: 930

Exercise 4.
Josie took a long multiple-choice, end-of-year vocabulary test. The ratio of the number of problems Josie got incorrect to the number of problems she got correct is 2: 9.
a. If Josie missed 8 questions, how many did she get correct? Draw a tape diagram to demonstrate how you found the answer.
Answer:

b. If Josie missed 20 questions, how many did she get correct? Draw a tape diagram to demonstrate how you found the answer.
Answer:

c. What ratios can we say are equivalent to 2: 9?
Answer:
8: 36 and 20: 90

d. Come up with another possible ratio of the number Josie got incorrect to the number she got correct.
Answer:

e. How did you find the numbers?
Answer:
Multiplied 5 × 2 and 5 × 9

f. Describe how to create equivalent ratios.
Answer:
Multiply both numbers of the ratio by the same number (any number you choose).

Eureka Math Grade 6 Module 1 Lesson 3 Problem Set Answer Key

Question 1.
Write two ratios that are equivalent to 1: 1.
Answer:
Answers will vary. 2:2, 50: 50, etc.

Question 2.
Write two ratios that are equivalent to 3: 11.
Answer:
Answers will vary. 6:22, 9:33, etc.

Question 3.
a. The ratio of the width of the rectangle to the height of the rectangle is _______ to _______.

Answer:
The ratio of the width of the rectangle to the height of the rectangle is   9     to   4    .

b. If each square in the grid has a side length of 8 mm, what is the width and height of the rectangle?
Answer:
72 mm wide and 32 mm high

Question 4.
For a project in their health class, Jasmine and Brenda recorded the amount of milk they drank every day. Jasmine drank 2 pints of milk each day, and Brenda drank 3 pints of milk each day.
a. Write a ratio of the number of pints of milk Jasmine drank to the number of pints of milk Brenda drank each day.
Answer:
2: 3

b. Represent this scenario with tape diagrams.
Answer:

c. If one pint of milk Is equivalent to 2 cups of milk, how many cups of milk did Jasmine and Brenda each drink? How do you know?
Answer:
Jasmine drank 4 cups of milk, and Brenda drank 6 cups of milk. Since each pint represents 2 cups, I multiplied
Jasmine’s 2 pints by 2 and multiplied Brenda’s 3 pints by 2.

d. Write a ratio of the number of cups of milk Jasmine drank to the number of cups of milk Brenda drank.
Answer:
4: 6

e. Are the two ratios you determined equivalent? Explain why or why not.
Answer:
2: 3 and 4: 6 are equivalent because they represent the same value. The diagrams never changed, only the value of each unit in the diagram.

Eureka Math Grade 6 Module 1 Lesson 3 Exit Ticket Answer Key

Pam and her brother both open savings accounts. Each begin with a balance of zero dollars. For every two dollars that Pam saves in her account, her brother saves five dollars in his account.

Question 1.
Determine a ratio to describe the money in Pam’s account to the money in her brother’s account.
Answer:
2: 5

Question 2.
If Pam has 40 dollars in her account, how much money does her brother have in his account? Use a tape diagram to support your answer.
Answer:

Question 3.
Record the equivalent ratio.
Answer:
40: 100

Question 4.
Create another possible ratio that describes the relationship between the amount of money in Pam’s account and the amount of money in her brother’s account.
Answer:
Answers will vary. 4: 10, 8: 20, etc.

Wanna become a master in maths? This is the right destination to learn and score maximum marks in the exams. Eureka Math Algebra 2 Answer Key provides students unlimited practice, real-time feedback, and also different question types and learning aids. So, the students can grab this wonderful opportunity of learning math problems easily with the free Engage NY Eureka Math Algebra 2 Answer Key pdf. Engage NY Math Algebra 2 Solution Key PDF Modulewise and start your learnings in a fun and engaging way.

EngageNY Math Algebra 2 Answer Key | Algebra 2 Eureka Math Answers Key PDF Free Download

All the question and answers covered in this Engage NY Eureka Math Algebra 2 Answer Key are arranged by award-winning Mathematics educators in a systematic way to understand the concepts deeply. Hence make use of the given handy solutions of all chapters covered in our Engage NY Math Algebra 2 Solutions and learn all math concepts easily whenever you want. All you have to do is to click on the links provided below. We have provided the Answer Key for Eureka Math Algebra 2 from Module 1 to Module 4.

• Eureka Math Algebra 2 Module 1 Answer Key
• Eureka Math Algebra 2 Module 2 Answer Key
• Eureka Math Algebra 2 Module 3 Answer Key
• Eureka Math Algebra 2 Module 4 Answer Key

What are the benefits of solving Eureka Algebra 2 Solution Key?

Modulewise Algebra 2 Eureka Math Answers PDF provided here are very essential for students to prepare well for the exams.

1. Practicing the Math concepts from Engage NY Eureka Math Textbook Algebra 2 Answer Key will be a great help for students and teachers in their Math Journey.
2. With the help of Eureka Math Algebra 2 Answer Key you can finish your homework in time.
3. You can enhance your performance in the practice test, assignments by using the Engage NY Algebra 2 Answer key.
4. It helps to improve your logical thinking and become master in math.

FAQs on Eureka Math Algebra 2 Answer Key Modulewise

1. How do I Use Engage NY Eureka Math Algebra 2 Answer Key to Score better marks?
   You can utilize Eureka Math Algebra 2 Answer Key and learn the tricks to solve all types of Math problems from all modules. By practicing and answering the Math sums provided in the Engage NY Eureka Math Textbook Algebra 2 Answer Key Pdf will help you to score the highest marks in math exams.
2. Where do I get Module 1 to Module 4 Eureka Math Algebra 2 Solutions?
   You can get Module Wise Engage NY Eureka Math Algebra 2 Answers in PDF Format via quick links available on our site ie, on ccssanswers.com
3. How to Download Eureka Math Algebra 2 Answer Key for all modules?
   Simply tap the direct links provided on our page and download the Engage NY Eureka Math Algebra 2 Answers in pdf format for all chapters for free of cost. You can prepare online or offline mode from ccssanswers.com