Engage NY Eureka Math Geometry Module 1 Lesson 19 Answer Key
Eureka Math Geometry Module 1 Lesson 19 Example Answer Key
Example 1.
i. Draw and label a △PQR in the space below.
ii. Use your construction tools to apply one of each of the rigid motions we have studied to it in a sequence of your choice.
iii. Use function notation to describe your chosen composition here. Label the resulting image as △XYZ: _____________________________________
iv. Complete the following sentences: (Some blanks are single words; others are phrases.)
△PQR is ___ to △XYZ because ___ map point P to point X, point Q to point Y, and point R to point Z. Rigid motions map segments onto __ and angles onto __.
Answer:
△PQR is congruent to △XYZ because rigid motions map point P to point X, point Q to point Y, and point R to point Z. Rigid motions map segments onto segments of equal length and angles onto angles of equal measure.
Example 2.
On a separate piece of paper, trace the series of figures in your composition but do NOT include the center of rotation, the line of reflection, or the vector of the applied translation.
Swap papers with a partner, and determine the composition of transformations your partner used. Use function
notation to show the composition of transformations that renders △PQR≅△XYZ.
Eureka Math Geometry Module 1 Lesson 19 Problem Set Answer Key
Question 1.
Use your understanding of congruence to explain why a triangle cannot be congruent to a quadrilateral.
a. Why can’t a triangle be congruent to a quadrilateral?
Answer:
A triangle cannot be congruent to a quadrilateral because there is no rigid motion that takes a figure with three vertices to a figure with four vertices.
b. Why can’t an isosceles triangle be congruent to a triangle that is not isosceles?
Answer:
An isosceles triangle cannot be congruent to a triangle that is not isosceles because rigid motions map segments onto segments of equal length, and the lengths of an isosceles triangle differ from those of a triangle that is not isosceles.
Question 2.
Use the figures below to answer each question:
a. △ABD≅△CDB. What rigid motion(s) maps \(\overline{C D}\) onto \(\overline{A B}\) ? Find two possible solutions.
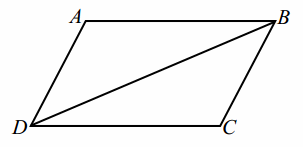
Answer:
A 180° rotation about the midpoint of \(\overline{D B}\)
A reflection over the line that joins the midpoints of \(\overline{A D}\) and \(\overline{B C}\), followed by another reflection over the line that joins the midpoints of \(\overline{A B}\) and \(\overline{D C}\)
b. All of the smaller triangles are congruent to each other. What rigid motion(s) map \(\overline{Z B}\) onto \(\overline{A Z}\) ? Find two possible solutions.
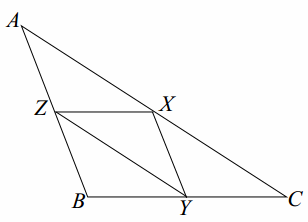
Answer:
A translation \(T_{\overline{Z A}}\)
A 180° rotation about the midpoint of \(\overline{Z Y}\) , followed by a 180° rotation about the midpoint of \(\overline{Z X}\)
Eureka Math Geometry Module 1 Lesson 19 Exit Ticket Answer Key
Assume that the following figures are drawn to scale. Use your understanding of congruence to explain why square ABCD and rhombus GHIJ are not congruent.
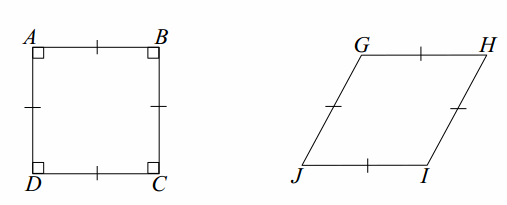
Answer:
Rigid motions map angles onto angles of equal measure, and the measures of the angles of square ABCD are all 90°, whereas the angles of rhombus GHIJ are not. Therefore, there is no rigid motion that maps square ABCD onto rhombus GHIJ.