Engage NY Eureka Math 3rd Grade Module 7 Lesson 19 Answer Key
Eureka Math Grade 3 Module 7 Lesson 19 Problem Set Answer Key
Question 1.
Use unit square tiles to make rectangles for each given number of unit squares. Complete the charts to show how many rectangles you can make for each given number of unit squares. The first one is done for you. You might not use all the spaces in each chart.
| Number of unit squares = 12 Number of rectangles I made: 3 |
|
| Width | Length |
| 1 | 12 |
| 2 | 6 |
| 3 | 4 |
Answer:
| Number of unit squares = 13 Number of rectangles I made: _________ |
|
| Width | Length |
| 1 | 13 |
| Number of unit squares = 13 Number of rectangles I made: ____1_____ |
|
| Width | Length |
| 1 | 13 |
| Number of unit squares = 14 Number of rectangles I made: __2_______ |
|
| Width | Length |
| 1 | 14 |
| 2 | 7 |
| Number of unit squares = 15 Number of rectangles I made: _____2____ |
|
| Width | Length |
| 1 | 15 |
| 2 | 6 |
| Number of unit squares = 16 Number of rectangles I made: ___3______ |
|
| Width | Length |
| 1 | 16 |
| 4 | 5 |
| 2 | 6 |
| Number of unit squares = 17 Number of rectangles I made: _____2____ |
|
| Width | Length |
| 1 | 17 |
| 2 | 7 |
| Number of unit squares = 18 Number of rectangles I made: __3______ |
|
| Width | Length |
| 1 | 18 |
| 3 | 6 |
| 2 | 9 |
Explanation:
Perimeter of rectangle = 2 ( Length + Width )
Question 2.
Create a line plot with the data you collected in Problem 1.
Number of Rectangles Made with Unit Squares

Answer:
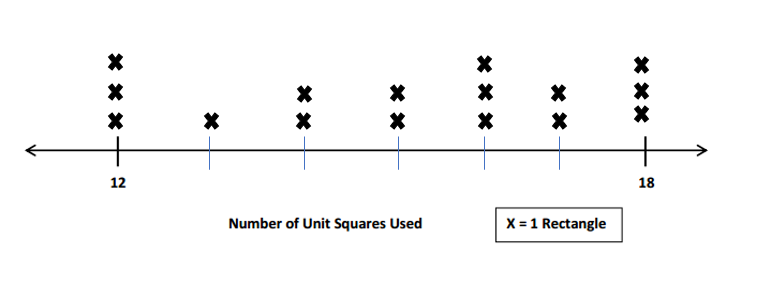
Explanation:
Number of Rectangles Made with Unit Squares in the Problem 1 are plotted using the number line as X = 1 Rectangle on each number.
Question 3.
Which numbers of unit squares produce three rectangles?
Answer:
The numbers of unit squares produce three rectangles are number 12, number 16 and the number 18.
Explanation:

The numbers of unit squares produce three rectangles are number 12, number 16 and the number 18.
Question 4.
Why do some numbers of unit squares, such as 13, only produce one rectangle?
Answer:
Some numbers likely to produce only one rectangle because they have only one pair of factors that can multiply to make that number. So, among that numbers 13 is one of the numbers.
Explanation:
Some numbers likely to produce only one and itself. They have only one pair of factors that can multiply to make that number. So, among that numbers 13 is one of the numbers.
Eureka Math Grade 3 Module 7 Lesson 19 Exit Ticket Answer Key
Use unit square tiles to make rectangles for the given number of unit squares. Complete the chart to show how many rectangles you made for the given number of unit squares. You might not use all the spaces in the chart.
| Number of unit squares = 20 Number of rectangles I made: _____4____ |
|
| Width | Length |
Answer:
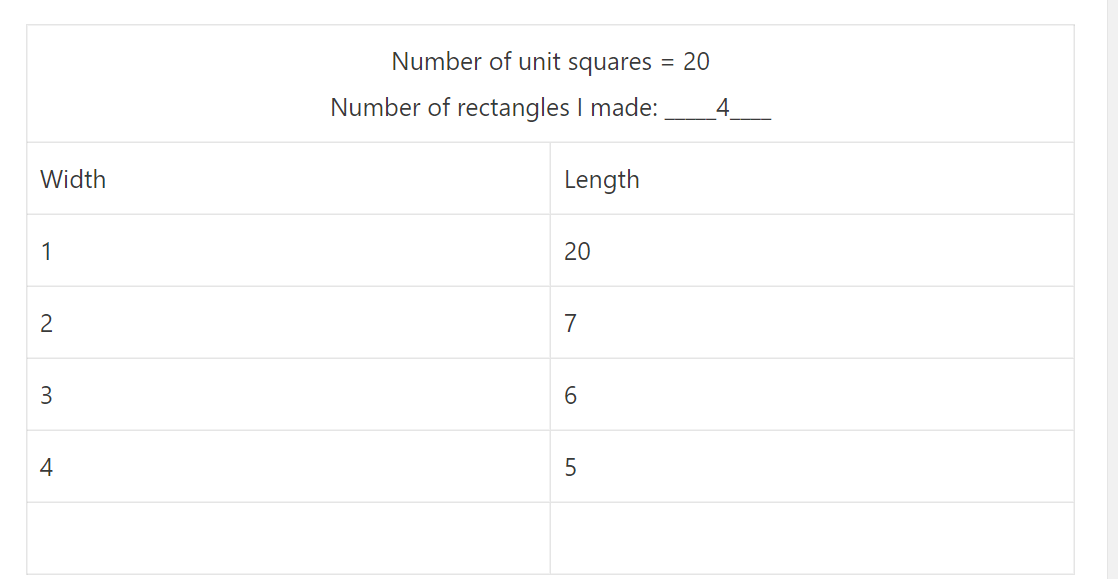
Explanation:
Number of rectangles formed by using 20 square tiles = 4.
Eureka Math Grade 3 Module 7 Lesson 19 Homework Answer Key
Question 1.
Cut out the unit squares at the bottom of the page. Then, use them to make rectangles for each given number of unit squares. Complete the charts to show how many rectangles you can make for each given number of unit squares. You might not use all the spaces in each chart.
Answer:
| Number of unit squares = 6 Number of rectangles I made: ____2___ |
|
| Width | Length |
| 1 | 6 |
| 2 | 3 |
| Number of unit squares = 7 Number of rectangles I made: ____1_____ |
|
| Width | Length |
| 1 | 7 |
| Number of unit squares = 8 Number of rectangles I made: _________ |
|
| Width | Length |
| 1 | 8 |
| 2 | 6 |
| Number of unit squares = 9 Number of rectangles I made: _________ |
|
| Width | Length |
| 1 | 9 |
| 3 | 3 |
| Number of unit squares = 10 Number of rectangles I made: _________ |
|
| Width | Length |
| 1 | 10 |
| 2 | 5 |
| Number of unit squares = 11 Number of rectangles I made: _________ |
|
| Width | Length |
| 1 | 11 |
Answer:
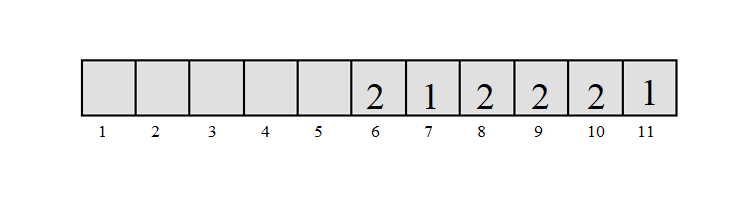 Explanation:
Explanation:
Number of rectangles formed by using given number of square tiles are counted and represented in the table chart.
Question 2.
Create a line plot with the data you collected in Problem 1.
Number of Rectangles Made with Unit Squares

a. Luke looks at the line plot and says that all odd numbers of unit squares produce only 1 rectangle. Do you agree? Why or why not?
b. How many X’s would you plot for 4 unit squares? Explain how you know.
Answer:
a. Luke looks at the line plot and says that all odd numbers of unit squares produce only 1 rectangle. No, I disagree with the Luke’s statement because only prime numbers have factors as itself and number one. Here, numbers 7 and 11 are prime numbers not odd numbers, so they have only formed one rectangle.
b. Number of rectangles formed by 4 unit square are only 2 because number 4 has factors as 1 and itself and number 2.
Explanation:
a. 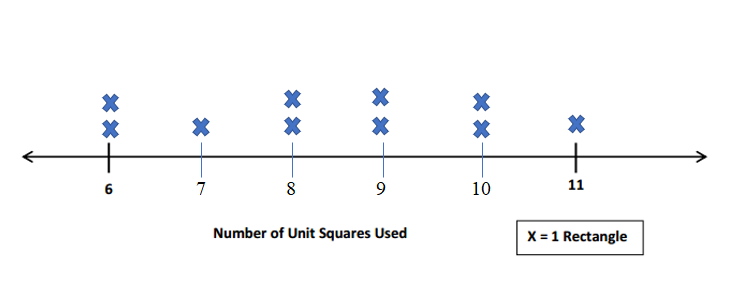
Prime numbers are the positive integers having only two factors, 1 and the integer itself.
Odd numbers are whole numbers that cannot be divided exactly into pairs.
b. Factors of number 4 =( 1, 4 ); ( 2,2 ).
Number of rectangles formed by 4 unit square are only 2 because number 4 has factors as 1 and itself and number 2.