Prasanna

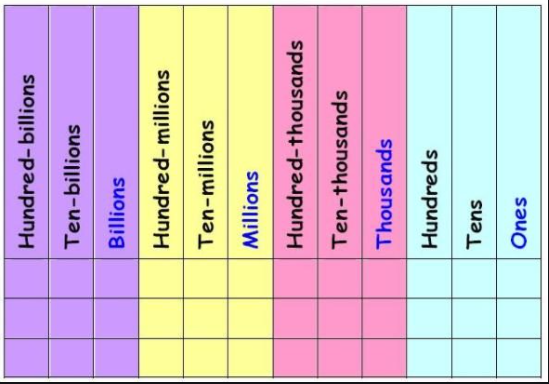
Numbers are formed by grouping the digits together. The order of place value of digits starts from right to left are units, tens, hundreds, thousands, ten thousand, lakhs, and so on. Digits are 0, 1, 2, 3, 4, 5, 6, 7, 8, 9. Follow the rules and guidelines listed in the further sections to have an idea of How Numbers are Formed. Go through the solved examples on making numbers from given digits for getting a good hold of the entire concept.

Also, Read:

• Reading and Writing Large Numbers

Rules for Formation of Numbers

We can easily make the numbers by following certain rules

• To form the greatest number, arrange the digits in descending order from Left to Right.
• To form the smallest number, arrange the digits in ascending order from left to right.
• If zero is one of the digits while forming the smaller number, it is written in place after the highest place.

Forming Smallest/Greatest Numbers using Given Digits

1. Write the greatest number using 5, 7, 8, 9, 6?

The greatest number is 98,765.

2. Write the greatest number using the digits 1,3,6,4,9?

The greatest number is  96,431.

3.Write the smallest number using the digits 7,5,1,3,8?

The smallest number is 13,578.

4. Write the smallest number using 8,6,1,3,5?

The smallest number is 13,568.

Forming the Numbers from Given Digits Examples

Example 1:

Using the digits 6, 1, 3, 0, and 5, write the smallest number.

Solution:

The smallest number is 10,356.

Example 2:

Using the digits 2,8,0,6 and 5 write the smallest number.

Solution:

The smallest number is 20,568.

Example 3:

Form the greatest number and smallest number using the digits 7, 8, 4, 3, 9?

Solution:

The greatest number using the digits is 98,743.

The smallest number using the digits is 34,789.

Example 4:

Form the greatest number and smallest number using the digits 5, 2, 4, 7, 6?

Solution:

The greatest number using the digits is 76,542.

The smallest number using the digits is 24,567.

Example 5:

Form the greatest number and smallest number using the digits 8, 1, 4, 9, 0?

Solution:

The greatest number using the digits is 98,410.

The smallest number using the digits is 10,489.

FAQs on Formation of Numbers using Given Digits

1. How the greatest number is formed?

The greatest number is formed by arranging the digits in descending order.

2. How the smallest number is formed?

The smallest number is formed by arranging the digits in ascending order.

3. Where will you place zero while forming the smallest number?

Zero is placed at one place after the highest place while forming the smallest number.

4. Form the highest number using the digits 1, 6, 9, 3, 8?

The highest number using the digits is 98631.

5. Form the smallest number using the digits 3, 9, 0, 6, 2? 

The smallest number using the digits is 20,369.

Are you confused about place values and face values of digits in a number? Here you can have ample knowledge on place values and face values. Find Definition of Place Value and Face Value, its  Properties. You can also check the solved examples of place values and face values for a better understanding of the concept. By the end of this article, we are sure you will get a complete idea of What is meant by Face Value and Place Value and their basic differences.

Read More:

• Place Value
• Place Value Chart
• Finding and Writing the Place Value

Place Value – Definition

In a number, every digit has a place value. Place value is defined as the value represented by a digit in a number on the basis of its position. In a number, the position of a digit starts from one’s place. The order of place value of digits in a number from right to left are units, tens, hundreds, thousands, ten thousand’s, Lakhs, and so on. A number is formed by grouping the digits together.

Example of Place Value:

In 8456,8 is in the thousands place and its place value is 8000.

4 is in the hundreds place and its place value is 400.

5 is in the tens place and its place value is 50.

6 is in one place and its place value is 6.

Place values of digits help us write numbers in expanded form. For example, expanded form of a number above

8456 is 8000+400+50+6.

The place value chart can help us find the place value of a number. Place value tells us how much each digit stands for.

Millions	Hundred Thousand	Ten thousand	Thousands	Hundreds	Tens	Ones
4	3	8	6	3	7	9

In 4386379,

Place value of 4=4000000

Place value of 3=300000

Place value of 8=80000

Place value of 6=6000

Place value of 3=300

Place value of 7=70

Place value of 9=9

Properties of Place Value

• The place value or face value of a one-digit number is the same. The place value and the face value of 1, 2, 3, 4, 5, 6, 7, 8, 9 are 1, 2, 3, 4, 5, 6, 7, 8, 9.
• The place value of zero is always zero. Zero may be placed at any place value in the number, the value is always zero. For Example:  In the numbers 10, 108, 250, 3067 the place value of zero is zero.
• In a two-digit number, the place value of a ten-place digit is equal to ten times the digit. Example: The place value of 5 in 53 is 5*10=50

Place Value of a Number Examples

1. The place value of 3 in 235 is 3*10=30

2. In the number 523,  digit 3 is at one place, digit 2 is at tens place, and digit 5 is at hundreds of places.

So the place value of 3 is 3, 2 is 2*10=20, 5 is 5*100=500.

Place Value of the Digit =(Face Value of the Digit)*(Value of the Place)

Thus for the place value of the digit, the digit is multiplied by the face value and value of that place.

Place Value and Face Value Questions

Example 1:

In the number 345, find the place values of digits?

The place value of 5 is 5

The place value of 4 is 4*10=40

The place value of 3 is 3*100=300

Example 2:

In the number 5678, find the place values of digits?

Solution:

The place value of 8 is 8

The place value of 7 is 7*10=70

The place value of 6 is 6*100=600

The place value of 5 is 5*1000=5000.

Example 3:

In the number 83241, find the place value of digits?

Solution:

The place value of 1 is 1

The place value of 4 is 4*10=40

The place value of 2 is 2*100=200

The place value of 3 is 3*1000=3000.

The place value of 8 is 8*10000=80000.

Example 4:

Write the place values of given numbers

1. 3 in 56321
2. 5 in 2578
3. 8 in 86731?

Solution:

The given number is 56321

The place value of 3 in 56321 is 3*100=300.

The place value of 5 in 2578 is 5*100=500.

The place value of 8 in 86731 is 8*10000=80000.

Example 5:

Write the place values of given numbers

1. 8 in 12386
2. 1 in 7178
3. 8 in 8336731?

Solution:

The place value of 8 in 12386 is 8*10=80.

The place value of 1 in 7178 is 1*100=700.

The place value of 8 in 2578 is 8*1000000=80,00000.

Example 6:

1. What is the Digit at thousands place in 5234?

2. What is the Digit at lakhs place in 152340?

3. What is the Digit at hundreds Places in 630?

Solution:

1. In 5234,4 is at units place,3 is at tens place, 2 is at hundreds places, and 5 is at thousands place. So digit at thousands place is 5.
2. In 152340, 0 is at units place,4 is at tens place,3 is at hundreds place, 2 is at thousands place,5 is at ten thousand place,1 is at lakhs place. So digit at lakhs place is 1.
3. In 630, 0 is at units place, 3 is in the tens place and 6 is in the hundreds place.

FAQ’s on Place Value and Face Value

1. Write the differences between the face value and place value?

Face value means an exact value of a digit in the number. Place value means the position of a particular digit in the number.

2. How do you represent the place values of digits?

Place values of digits are represented by units,  tens, hundreds, thousands, ten thousands, Lakhs, and so on.

3. What is the place value of digit 5 in 25461?

The place value of digit 5 in 25461 is thousands.

4. What is the face value of 7?

Face value of 7 is 7.

A number is formed by digits. Each digit has a place value. The order of place values of digits represented from right to left are units, tens, hundreds, thousands, ten thousand, lakhs, ten lakhs, and so on. The method of finding and writing the place value is explained with the following examples. Consider the numbers in numerals and find the place values of digits.

Do Read:

• Place Value
• Place Value Chart

Place Value Chart | How to find Place Value?

To better understand the concept of Place Value check out the place value chart provided below. Use it as a quick reference to learn about the place value of digits such as units, tens, hundreds, thousands, ten thousand, hundred thousands, and so on.

Place Value of Digits Examples

Example:

Find the place values of digits in 5643.

Solution:

In the number 5643, 5 is the fourth digit for the right. 5 is in the thousands place. So the place value is 5000.

6 is the third digit for the right. 6 is in the hundreds place. So the place value is 600.

4 is the second digit for the right.4 is in the tens place. So the place value is 40.

3 is the first digit for the right.3 is in the unit’s place. So the place value is 3.

The above process is shown as

5         6         4           3

|           |          |             |

5000     600     40      3

The number is written as Five thousand six hundred forty and one.

Example 2:

Find the place values of digits in 4398

Solution:

In the number 4398, 4 is the fourth digit for the right. 4 is in the thousands place. So the place value is 4000.

3 is the third digit for the right. 3 is in the hundreds place. So the place value is 300.

9 is the second digit for the right.9 is in the tens place. So the place value is 90.

8 is the first digit for the right.8 is in the unit’s place. So the place value is 8.

The above process is shown as

4         3        9              8

|           |          |             |

4000    300      90         8

The number can be written as Four thousand three hundred ninety and eight.

Example 3:

Find the place value of the digits in the number 34239

Solution:

In the number 34239, 3 is the fifth digit for the right. 3 is in the ten thousand’s place. So the place value is 30000.

4 is the fourth digit for the right. 4 is in the thousands place. So the place value is 4000.

2 is the third digit for the right.2 is in the hundred’s place. So the place value is 200.

3is the second digit for the right.3 is in the tens place. So the place value is 30.

9 is the first digit for the right.9 is in the unit’s place. So the place value is 9.

The above process is shown as

3           4              2             3              9

|              |              |             |                |

30000     4000      200         30             9

The number 34239 can be written as Thirty-four thousand two hundred thirty and nine.

Example 4:

Find the place value of the digits in the number 6423

Solution:

In the number 6423, 6 is the fourth digit for the right. 6 is in the thousands place. So the place value is 6000.

4 is the third digit for the right. 4 is in the hundreds place. So the place value is 400.

2 is the second digit for the right.2 is in the tens place. So the place value is 20.

3 is the first digit for the right.3 is in the unit’s place. So the place value is 3.

The above process is shown as

6          4           2            3

|            |           |              |

6000     400       20         3

The number can be written as six thousand four hundred twenty and three.

FAQ’S on Place Values of Digits

1. Find the place value of 5 in the number 3456?

The place value of 5 in the number 3456 is tens. i.e. 50.

2. What is the place value of 5 in the number 54216?

The place value of 5 in the number 54216 is ten thousand. i.e. 50,000.

3. Find the place value of 6 in the number 8634?

place value of digit 6 in the number is hundred. i.e. 600.

4. What is the place value of 3 in the number 6345?

place value of a digit 3 in the number is hundreds.

5. How do you write the number 4560?

4560 can be written as four thousand five hundred sixty.

By visiting this page you can have plenty of knowledge on Four-digit numbers. You can understand the definition of a four-digit number, how to compare four-digit numbers, how to arrange 4 digit numbers in ascending order and descending order. You can also find solved examples on comparison of four-digit numbers, arranging 4 digit numbers in ascending order and descending order.

Also, Check:

• Comparison of One-digit Numbers
• Comparison of Two-digit Numbers
• Comparison of Three-digit Numbers

Four Digit Numbers – Definition

A four-digit number contains four digits. According to their values, the digits are placed from right to left at one’s place, ten’s place, hundred’s place, and thousand’s place.

Examples of four-digit numbers are 3456,2789,6540 etc.

How to Compare 4 Digit Numbers?

In the comparison of the four-digit number,1) The numbers having more digits are greatest. i.e. four-digit numbers are always greater than three-digit numbers,two-digit numbers,one-digit numbers.

5436>250, 8500>85, 3451>100,7890>789

2)Thousand place digit is compared for comparing four-digit numbers.

5678>4321, 7321>4678, 9450>8567, 1523>1032

3)If thousands place of a four-digit number is equal then follow the rules of a three-digit number.

8520>8341, 6432>6312,9800>9723,7520>7120

Comparing Four-Digit Numbers Examples

Example 1:

Compare four-digit numbers 6520, 5231?

Solution:

In 6520,6 is in the thousands place.

In 5231,5 is in the thousands place.

compare thousands place of two numbers i.e. 6>5

so 6520>5231.

Example 2:

Compare four-digit numbers 4210, 3120?

Solution:

In 4210,4 is in the thousands place.

In 3120,3 is in the thousands place.

Compare thousands place of two numbers i.e. 4>3

So 4210>3120.

Example 3:

Compare four-digit numbers 9520, 9420?

Solution:

In 9520,9 is in the thousands place.

5 is in the hundreds place.

2 is in the tens place.

0 is in one place.

In 9420,9 is in the thousands place.

4 is in the hundreds place.

2 is in the tens place.

0 is in one’s place.

since thousands place of both the numbers are same. Compare hundred’s place of both the numbers.

5>4.

so 9520>9420.

Example 4:

Compare four-digit numbers 3425, 3421?

Solution:

In 3425,3 is in the thousands place.

4 is in the hundreds place.

2 is in the tens place.

5 is in one’s place.

In 3421,3 is in the thousands place.

4 is in the hundreds place.

2 is in the tens place.

1 is in one’s place.

since thousands, hundreds, and tens place are same compare one’s place of both the numbers.

5>1

so3425>3421.

5. Compare four-digit numbers 6540,5420

In 6540,6 is in the thousands place.

5 is in the hundreds place.

4 is in the tens place.

0 is in one’s place

In 5420, 5 is in the thousands place.

4 is in the hundreds place.

2 is in the tens place.

0 is in one’s place.

compare a thousand places of both the numbers i.e. 6>5. So 6540 is greater than 5420.

Numbers can be arranged in two ways. one is Ascending order and the other one is descending order.

Ascending Order

If the numbers are arranged from smallest to largest. Then it is called Ascending order.

How to arrange 4 Digit Numbers in Ascending Order?

1. Arrange the four-digit numbers 2300,4789,6520,1220,8320,6342 in Ascending order?

Four-digit numbers in ascending order are 1220, 2300, 4789, 6342, 6520,8320.

2. Arrange the four-digit numbers 3340,1783,2520,1220,8920,6142 in Ascending order?

Four-digit numbers in ascending order are 1220,1783,2520,3340,6142,8920.

3. Arrange the four-digit numbers 1340,6873,5520,2220,7920,6142 in Ascending order?

Four-digit numbers in ascending order are1340,2220,5520,6142,6873,7920.

4. Arrange the four-digit numbers 1830,3456,6754,1290,2134,8675 in Ascending order?

Four-digit numbers in ascending order are 1290, 1830,2134,3456,6754,8675.

Descending Order

If numbers are arranged from largest to smallest then it is called Descending order.

How to arrange 4 Digit Numbers in Descending Order?

1.Arrange four-digit numbers 3320, 5420, 1121, 6345, 9230, 2250 in Descending Order?

Four-digit numbers in Descending order are  9230, 6345, 5420, 3320, 2250, 1121.

2.Arrange four-digit numbers 5120, 1420, 9121, 3345, 9530, 2150 in Descending Order?

Four-digit numbers in Descending order are 9530, 9121,5120, 3345, 2150, 1420.

3.Arrange four-digit numbers 4120, 8420, 1828, 7345, 1230, 2950 in Descending Order?

Four-digit numbers in Descending order are 8420, 7345, 4120, 2950, 1828, 1230.

4. Arrange four-digit numbers 1200,6540,8324,3214,5889,4678?

Four-digit numbers in descending order are 8324, 6540, 5889, 4678, 3214, 1200.

FAQ’s  on Comparison of Four-Digit Numbers

1. How many digits are there in a four-digit number?

There are four digits in a four-digit number.

2. What is the smallest four-digit number?

The smallest four-digit number is 1000.

3. What is the greatest four-digit number?

The greatest four-digit number is 9999.

4. What is the place value of  5 in 5734?

The place value of 5 in 5734 is thousands.

To understand the relation, you need to have a clear idea of the cross-product of two sets. Because a relation is the subset of the cartesian product of two sets. Generally, a set is a collection of well-defined elements and a cartesian product is the product of two sets which has a set of ordered pairs in the form of (a, b). Where a is an element of the first set, b is an element of the second set. Basically, a relation is a rule that related an element from one set to the second element in another set. Get to know more about the domain and range of a relation.

Also, Read:

• Cartesian Product of Two Sets
• Worksheet on Math Relation

Domain and Range of a Relation – Definition

Relation: If there are two non-empty sets A, B, then the relation r is defined as the subset of cross-product A x B. That subset is the result of the relation between the elements of both sets. R = {(a, b) : a ∈ A, b ∈ B}.

Domain: The set of elements of the first set belonging to the relation R is called the domain of the relation.

Domain(r) = {a : (a, b) ∈ R}

The domain of relation from A to B is a subset of A.

Range: The set of elements of the second set belonging to the relation R is called the range of the relation.

Range(r) = {b : (a, b) ∈ R}

The range of relation from A to B is a subset of B.

Example:

If two non empty sets are P = {1, 3, 7, 6, 5}, Q = {2, 4, 8, 9, 12}

Then R is the relation “less than” from set P to set Q

The relation R = {(1, 2), (1, 4), (3, 4), (7, 9), (5, 12), (6, 8)}

Therefore, Domain(R) = {1, 3, 5, 6, 7}, Range(R) = {2, 4, 8, 9, 12}

The arrow diagram to represent the domain and range of a relation is

Domain and Range Examples and Answers

Question 1:

If A = {3, 5, 7, 6} and B = {21, 30, 18, 35, 10} and R be the relation ‘is factor of’ from A to B.

(i) Write R in the roster form and find the domain and range of a relation R.

(ii) Draw an arrow diagram to represent the relation.

Solution:

Given that,

A = {3, 5, 7, 6} and B = {21, 30, 18, 35, 10}

(i) R has elements of a, b and a is a factor of B. Where a is an element of A and b is an element of B.

Therefore, relation R in roster form = {(3, 21), (3, 18), (3, 30), (5, 30), (5, 35), (5, 10), (7, 21), (7, 35), (6, 18), (6, 30)}

The domain of the relation R is the set of all first components of R.

Range of relation R is the set of all second components of R.

So, Domain(R) = {3, 5, 6, 7}

Range(R) = {10, 18, 21, 30, 35}

(ii) The arrow diagram to represent R is

Question 2:

Find the domain and range of the relation R defined by R = {(x + 1, 2x + 3) : x ∈ {1, 2, 3, 4, 5}}

Solution:

Since x = {1, 2, 3, 4, 5}

Therefore,

x = 1 ⇒ x + 1 = 1 + 1 = 2 and 2x + 3 = 2(1) + 3 = 2 + 3 = 5

x = 2 ⇒ x + 1 = 2 + 1 = 3 and 2x + 3 = 2(2) + 3 = 4 + 3 = 7

x = 3 ⇒ x + 1 = 3 + 1 = 4 and 2x + 3 = 2(3) + 3 = 6 + 3 = 9

x = 4 ⇒ x + 1 = 4 + 1 = 5 and 2x + 3 = 2(4) + 3 = 8 + 3 = 11

x = 5 ⇒ x + 1 = 5 + 1 = 6 and 2x + 3 = 2(5) + 3 = 10 + 3 = 13

Hence relation R = {(2, 5), (3, 7), (4, 9), (5, 11), (6, 13)}

Domain of R = {a : (a, b) ∈R} = Set of first components of all ordered pair belonging to R.

Domain(R) = {2, 3, 4, 5, 6}

Range of R = {b : (a, b) ∈ R} = Set of second components of all ordered pairs belonging to R.

Range(R) = {5, 7, 9, 11, 13}

Question 3:

The arrow diagram shows the relation (R) from set A to set B. Write this relation in the roster form. Also, find the domain and range of the relation.

Solution:

R is the relation from A to B.

From the arrow diagram, we can write the relation R = {(13, q), (14, p), (16, r), (14, t), (18, q)}

The relation set R in the roster form = {(13, q), (14, p), (16, r), (14, t), (18, q)}

Domain of R = set of all first components of the relation =  {13, 14, 16, 18}

Range of R = set of all second components of the relation = {p, q, r, t}

Question 4:

If X = {4, 5, 6, 8, 9, 10} define the relation R from X to X by R = {(x, y) : y = x + 1}

(i) Draw this relation using an arrow diagram.

(ii) Write domain and range of R.

Solution:

Given set X = {4, 5, 6, 8, 9, 10}

Relation R = {(x, y) : y = x + 1}

If x = 4, then y = x + 1 = 4 + 1 = 5

If x = 5, then y = x + 1 = 5 + 1 = 6

If x = 6, then y = x + 1 = 6 + 1 = 7

If x = 8, then y = x + 1 = 8 + 1 = 9

If x = 9, then y = x + 1 = 9 + 1 = 10

If x = 10, then y = x + 1 = 10 + 1 = 11

Relation R = {4, 5), (5, 6), (8, 9), (9, 10)}

(i) The arrow diagram of relation is

(ii) Domain of the relation = set of first components of all ordered pair belonging to R.

Therefore, domain of R = {4, 5, 8, 9}

Range of the relation = set of second components of all ordered pair belonging to R.

Therefore, range of R = {5, 6, 9, 10}.

FAQs on Domain and Range of a Relation

1. How do you state that domain and range of a relation?

The domain of a relation is nothing but the set of all first components of R and the range of a relation is the set of all second components of R.

2. What is a rule for relation?

Relation a subset of the cartesian product of two sets. And it is a set of ordered pairs which has a relationship between the first set element with the second set element.

3. Write an example for the domain and range?

If relation R = {(1, 4), (2, 5), (3, 6), (4, 7)}, then the domain of relation R is {1, 2, 3, 4} and range of relation R is {4, 5, 6, 7}.

Relations are one of the main topics of the set theory. Sets, relations, and functions are interrelated. Sets are the collection of ordered elements. Relation means the connection between the two sets. Have a look at the definition of Relations in Math definition, types of relations, and solved example questions in the below sections of this page.

Also, Read: Worksheet on Math Relation

Relation in Math – Definition

A relation in math defines the relationship between two or more different sets. A set of ordered pairs is also defined as the relation. Let us take two sets, if there is a relation between them that will be established, then there is a connection between the elements of the two sets. Mapping represents the relation.

Example:

Let two sets are A = {10, 20, 30, 45, 50}, B = {a, b, c, d}

The relation from set A into B is a subset of A x B.

A x B = {10, 20, 30, 45, 50} x {a, b, c, d}

= {(10, a), (10, b) (10, c), (10, d), (20, a), (20, b), (20, c), (20, d), (30, a), (30, b), (30, c), (30, d), (45, a), (45, b), (45, c), (45, d), (50, a), (50, b), (50, c), (50, d)}

The ordered pairs (10, c), (20, b), (30, d), (50, b)

The first set A {10, 20, 30, 45, 50} represents the domain.

The second set B {a, b, c, d} represents the range.

Representation of Relation in Math

In general, the relation is denoted by R. The relation in math from set A to set B is represented in any of three ways.

• Set Builder Form
• Roster Form
• Arrow Diagram

Set Builder Form:

In the set-builder form, the relation R from setA to set B is R = {(a, b):  ∈ A, b ∈ B, a . . b}, the blank space is replaced by the rule which associates a and b.

Example:

A = {2, 4, 5, 6, 8}, B = {4, 6, 8, 9}

Let R = {(2, 4), (4, 6), (6, 8), (8, 10)}

The set builder form of R = {(a, b):  ∈ A, b ∈ B a is 2 less than b}

Roster Form:

The roster form of the relation R from set A to set B is represented as the set of ordered pairs. In every ordered pair, 1st component is collected from set A, and 2nd component is collected from set B.

Example:

If A = {1, 8, 5}, B = {p, q, r}

then R = {(1, ), (8, p), (5, r)}

Hence, R ⊆ A × B

Arrow Diagram:

You need to draw two circles to represent two sets. Write elements in their corresponding sets. Draw arrows from set A to B which satisfy the relation and indicate the ordered pairs.

Example:

If A = {2, 4, 5, 6, 8} and B = {4, 6, 8, 9}

Then the relation from set A to set B is R = {(2, 4), (4, 6), (4, 8), (5, 8), (6, 9)}

Draw a diagram to represent the relation.

Types of Relations

There are 8 types of relations. They are along the lines.

1. Empty Relation:

It is also known as void relation. If there is no relation between any elements of a set, then it is an empty relation. The representation is R = Φ ⊂ A x A.

2. Transitive Relation:

In a transitive relation, if (a, b) ∈ R and (b, c) ∈ R, then (a, c) ∈ R. The representation is aRb and bRc ⇒ aRc such that a, b, c ∈ A.

3. Identity Relation:

Identity relation means every element of a set is related to itself only. The representation is i = {(a, a), a ∈ A}

4. Inverse Relation:

If a set has elements that are inverse pairs of another set, then it is called inverse relation. The representation is R^-1 = {(b, a): (a, b) ∈ R}

5. Universal Relation:

In universal relation, every element of a set is related to each other. The representation is R = A x A.

6. Reflexive Relation:

In reflexive relation, every element maps to itself. The representation is (a, a) ∈ R.

7. Symmetric Relation:

If a = b is true then b = a is also true in a symmetric relation. The representation is aRb ⇒ bRa, such that a, b ∈ A.

8. Equivalence Relation:

If a relation is reflexive, symmetric, and transitive at the same time then it called the equivalence relation.

Frequently Asked Questions on Relation in Math

1. What is a relation in math?

A relation between two sets is a collection of ordered pairs having one object from each set. If the element x is from the first set and element y is from the second set, then the elements are said to be related if the ordered pair (x, y) is in the relation.

2. What are the example of relation in math?

Let us take two sets as A = {a, b, c, d}, B = {x, y, z}. Then the relation from set A to set b is R = {(a, x), (b, y), (d, z), (c, y)}

3. What is a domain and range?

In any relation, the first set is called the domain and the second set is called the range.

Refer to Worksheet on Ratios while preparing for the exam. You can perform great by practicing various problems from Ratio Worksheets. Multiple models of questions along with the detailed solutions and explanations are given here. The Ratios Practice Worksheet is designed by the experts as per the latest syllabus.

So, students who wish to learn the ratio concepts can refer to these Word Problems on Ratios and score well in the examinations. All the concepts of ratios along with the basic concept of ratio, the ratio in the simplest form, conversion of ratios, comparison of ratios, and finding the equivalent ratios are given in this article.

Also, Read:

• Ratios
• Practice Test on Ratio and Proportion
• Worked Out Problems on Ratio and Proportion

Simplifying Ratios Worksheets

1. Express the following ratios in the simplest form.
(a) 60 : 90
(b) 500 : 200
(c) 3 : 15.3
(d) 4.2 : 14
(e) 16a : 24a
(f) 2 \(\frac { 1 }{ 6 } \) ∶ 1 \(\frac { 1 }{ 3 } \)
(g) \(\frac { 2 }{ 8 } \) ∶ \(\frac { 2 }{ 10 } \)
(h) \(\frac { 4 }{ 3 } \) ∶ \(\frac { 10 }{ 7 } \) : 2
(i) 4 \(\frac { 2 }{ 5 } \) ∶ 2 \(\frac { 1 }{ 2 } \) : 2 \(\frac { 2 }{ 5 } \)
(j) \(\frac { 4 }{ 5 } \) ∶ \(\frac { 6 }{ 10 } \)
(k) 1 ∶ \(\frac { 1 }{ 2 } \) ∶ \(\frac { 1 }{ 3 } \)
(l) 8m²n : 20mn²
(m) a dozen to a score
(n) 18 months : 5 years
(o) 10 m : 1200 cm

Solution:

(a) Given that 60: 90
List the factors of 60
The factors of 60 are 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30 and 60.
List the factors of 90.
The factors of 90 are 1, 2, 3, 5, 6, 9, 10, 15, 18, 30, 45, 90.
Find the greatest common factor of 60 and 90, GCF(60, 90)
GCF of 60 and 90 is 30.
Divide 60 and 90 each by the GCF.
\(\frac { 60 }{ 30 } \) = 2
\(\frac { 90 }{ 30 } \) = 3
Use the whole number results to rewrite the ratio in the simplest form.

Therefore, the final answer is 2:3

(b) Given that 500 : 200
List the factors of 500
The factors of 500 are 1, 2, 4, 5, 10, 20, 25, 50, 100, 125, 250, and 500.
List the factors of 200.
The factors of 200 are 1, 2, 4, 5, 8, 10, 20, 25, 40, 50, 100, and 200.
Find the greatest common factor of 500 and 200, GCF(500, 200)
GCF of 500 and 200 is 100.
Divide 500 and 200 each by the GCF.
\(\frac { 500 }{ 100 } \) = 5
\(\frac { 200 }{ 100 } \) = 2
Use the whole number results to rewrite the ratio in the simplest form.

Therefore, the final answer is 5: 2

(c) Given that 3: 15.3
Find the greatest common factor of 3 and 15.3, GCF(3, 15.3)
GCF of 3 and 15.3 is 3.
Divide 3 and 15.3 each by the GCF.
\(\frac { 3 }{ 3 } \) = 1
\(\frac { 15.3 }{ 3 } \) = 3.1
Use the whole number results to rewrite the ratio in the simplest form.

Therefore, the final answer is 1: 3.1

(d) Given that 4.2: 14
Divide 4.2 and 14 each by the 14.
\(\frac { 4.2 }{ 14 } \) = 0.3
\(\frac { 14 }{ 14 } \) = 1
Use the whole number results to rewrite the ratio in the simplest form.

Therefore, the final answer is 0.3: 1

(e) Given that 16a : 24a
Find the greatest common factor of 16a and 24a, GCF(16a, 24a)
GCF of 16a and 24a is 8a.
Divide 16a and 24a each by the GCF.
\(\frac { 16a }{ 8a } \) = 2
\(\frac { 24a }{ 8a } \) = 3
Use the whole number results to rewrite the ratio in the simplest form.

Therefore, the final answer is 2 : 3

(f) Given that 2 \(\frac { 1 }{ 6 } \) ∶ 1 \(\frac { 1 }{ 3 } \)
Find the greatest common factor of 2 \(\frac { 1 }{ 6 } \) and 1 \(\frac { 1 }{ 3 } \), GCF(2 \(\frac { 1 }{ 6 } \), 1 \(\frac { 1 }{ 3 } \))
GCF of 2 \(\frac { 1 }{ 6 } \) and 1 \(\frac { 1 }{ 3 } \) is \(\frac { 1 }{ 6 } \).
Divide 2 \(\frac { 1 }{ 6 } \) and 1 \(\frac { 1 }{ 3 } \) each by the GCF.
2 \(\frac { 1 }{ 6 } \) : \(\frac { 1 }{ 6 } \) = 13
1 \(\frac { 1 }{ 3 } \) :  \(\frac { 1 }{ 6 } \) = 8
Use the whole number results to rewrite the ratio in the simplest form.

Therefore, the final answer is 13: 8

(g) Given that \(\frac { 2 }{ 8 } \) ∶ \(\frac { 2 }{ 10 } \)
\(\frac { 2 }{ 8 } \) ∶ \(\frac { 2 }{ 10 } \) = \(\frac { 1 }{ 4 } \) ∶ \(\frac { 1 }{ 5 } \)
\(\frac { 1 }{ 4 } \) ∶ \(\frac { 1 }{ 5 } \) = 5 : 4
Use the whole number results to rewrite the ratio in the simplest form.

Therefore, the final answer is 5: 4

(h) Given that \(\frac { 4 }{ 3 } \) ∶ \(\frac { 10 }{ 7 } \) : 2
\(\frac { 4 }{ 3 } \) ∶ \(\frac { 10 }{ 7 } \) : 2
Divide the given numbers with 2.
\(\frac { 2 }{ 3 } \) ∶ \(\frac { 2 }{ 7 } \) : 1
Use the whole number results to rewrite the ratio in the simplest form.

Therefore, the final answer is 14: 15: 21

(i) Given that 4 \(\frac { 2 }{ 5 } \) ∶ 2 \(\frac { 1 }{ 2 } \) : 2 \(\frac { 2 }{ 5 } \)
Divide the given numbers with 2.
2 \(\frac { 1 }{ 5 } \) ∶ 1 \(\frac { 1 }{ 4 } \) : 1 \(\frac { 1 }{ 5 } \)
Use the whole number results to rewrite the ratio in the simplest form.

Therefore, the final answer is 44: 25: 24

(j) Given that \(\frac { 4 }{ 5 } \) ∶ \(\frac { 6 }{ 10 } \)
Divide the given numbers with 2.
\(\frac { 2 }{ 5 } \) ∶ \(\frac { 3 }{ 10 } \)
\(\frac { 20 }{ 15 } \)
Divide the above fraction with 5.

Therefore, the final answer is 4 : 3

(k) Given that 1 ∶ \(\frac { 1 }{ 2 } \) ∶ \(\frac { 1 }{ 3 } \)
1 ∶ \(\frac { 1 }{ 2 } \) ∶ \(\frac { 1 }{ 3 } \) = 6 : 3 : 2

Therefore, the final answer is 6 : 3 : 2

(l) Given that 8m²n : 20mn²
Find the greatest common factor of 8m²n and 20mn², GCF(8m²n, 20mn²)
GCF of 8m²n and 20mn² is 4mn.
Divide 8m²n and 20mn² each by the GCF.
8m²n : 4mn = 2m
20mn² :  4mn = 5n
Use the whole number results to rewrite the ratio in the simplest form.

Therefore, the final answer is 2m: 5n

(m) a dozen to a score
a dozen = 12
a score = 20
12: 20
Find the greatest common factor of 12 and 20, GCF(12, 20)
GCF of 12 and 20 is 4.
Divide 12 and 20 each by the GCF.
12 : 4 = 3
20 :  4 = 5
Use the whole number results to rewrite the ratio in the simplest form.

The answer for a dozen to a score in a simple ratio is 3: 5

(n) 18 months: 5 years
1 year = 12 months
5 years = 5 * 12 = 60
18 months: 60 months
Find the greatest common factor of 18 months and 60 months, GCF(18 months, 60 months)
GCF of 18 months and 60 months is 6 months.
Divide 18 months and 60 months each by the GCF.
18 months : 6 months = 3
60 months :  6 months = 10
Use the whole number results to rewrite the ratio in the simplest form.

The answer for a dozen to a score in a simple ratio is 3: 10

(o) 10 m: 1200 cm
1 m = 100 cm
10 m = 10 * 100 = 1000
1000 cm : 1200 cm
Find the greatest common factor of 1000 cm and 1200 cm, GCF(1000 cm, 1200 cm)
GCF of 1000 cm and 1200 cm is 200 cm.
Divide 1000 cm and 1200 cm each by the GCF.
1000 cm : 200 cm = 5
1200 cm :  200 cm = 6
Use the whole number results to rewrite the ratio in the simplest form.

The answer for a dozen to a score in a simple ratio is 5: 6

---

2. In a school library, the ratio of English books to that of Hindi books is 6: 7. If there are 156 English books, find the number of Hindi books in the library?

Solution:

Given that in a school library, the ratio of English books to that of Hindi books is 6: 7.
English : Hindi = 6 : 7
Substitute 156 in the place of English
156 : Hindi = 6 : 7
We know that product of extreme terms = product of mean terms
156 * 7 = Hindi * 6
Hindi = \(\frac { 1092 }{ 6 } \) = 182

Therefore, the number of Hindi books in the library are 182.

---

3. The ratio of the number of male and female teachers in a school is 4: 9. If the number of male teachers is 72, find the number of female teachers?

Solution:

Given that the ratio of the number of male and female teachers in a school is 4: 9.
male teachers: female teachers = 4: 9
Let the female teachers = x
Substitute 72 in the place of male teachers
72 : female teachers = 4 : 9
We know that product of extreme terms = product of mean terms
72 * 9 = Female teachers * 4
Female teachers = \(\frac { 648 }{ 4 } \) = 162

Therefore, the number of Female teachers are 162.

---

4. An alloy contains zinc and copper in the ratio 14: 18. Find the weight of copper if it has 63 kg of zinc?

Solution:

Given that an alloy contains zinc and copper in the ratio 14 : 18.
Let the weight of zinc be 14x
Let the weight of copper = 18x
As per the given information, 14x = 63 kg
x = \(\frac { 63 }{ 14 } \) = 4.5 kg
Find the weight of the copper by multiplying 4.5 kg with 18.
Substitute the x value in 18x.
The weight of the copper = 18 (4.5 kg) = 81 kg

Therefore, the weight of the copper is 81 kg.

---

5. Compare the following ratios.
(a) 4: 6 and 8: 10
(b) 22: 38 and 38: 42
(c ) 1 ∶ \(\frac { 2 }{ 3 } \) and \(\frac { 2 }{ 3 } \) ∶ \(\frac { 1 }{ 2 } \)
(d) \(\frac { 4 }{ 3 } \) : \(\frac { 5 }{ 9 } \) and \(\frac { 2 }{ 7 } \) ∶ \(\frac { 3 }{ 5 } \)

Solution:

(a) Given that 4 : 6 and 8 : 10.
4 : 6 can write as \(\frac { 4 }{ 6 } \)
8 : 10 can write as \(\frac { 8 }{ 10 } \)
Firstly, find the LCM of the 6 and 10.
The LCM of the 6 and 10 is 30.
\(\frac { 4 × 5 }{ 6 × 5 } \) = \(\frac { 20 }{ 30 } \)
\(\frac { 8 × 3 }{ 10 × 3 } \) = \(\frac { 24 }{ 30 } \)
Now, we can compare \(\frac { 20 }{ 30 } \) and \(\frac { 24 }{ 30 } \)
20 is less than 24.
\(\frac { 20 }{ 30 } \) < \(\frac { 24 }{ 30 } \)

Therefore, 4: 6 < 8 : 10

(b) Given that 22 : 38 and 38 : 42.
22 : 38 can write as \(\frac { 22 }{ 38 } \)
38 : 42 can write as \(\frac { 38 }{ 42 } \)
Firstly, find the LCM of the 38 and 42.
The LCM of the 38 and 42 is 798.
\(\frac { 22 × 21 }{ 38 × 21 } \) = \(\frac { 462 }{ 798 } \)
\(\frac { 38 × 19 }{ 42 × 19 } \) = \(\frac { 722 }{ 798 } \)
Now, we can compare \(\frac { 462 }{ 798 } \) and \(\frac { 722 }{ 798 } \)
462 is less than 722.
\(\frac { 462 }{ 798 } \) < \(\frac { 722 }{ 798 } \)

Therefore, 22 : 38 < 38 : 42.

(c) Given that 1 ∶ \(\frac { 2 }{ 3 } \) and \(\frac { 2 }{ 3 } \) ∶ \(\frac { 1 }{ 2 } \).
1 ∶ \(\frac { 2 }{ 3 } \) can write as \(\frac { 3 }{ 2 } \)
\(\frac { 2 }{ 3 } \) ∶ \(\frac { 1 }{ 2 } \) can write as \(\frac { 4 }{ 3 } \)
Firstly, find the LCM of the 2 and 3.
The LCM of the 2 and 3 is 6.
\(\frac { 3 × 3 }{ 2 × 3 } \) = \(\frac { 9 }{ 6 } \)
\(\frac { 4 × 2 }{ 3 × 2 } \) = \(\frac { 8 }{ 6 } \)
Now, we can compare \(\frac { 9 }{ 6 } \) and \(\frac { 8 }{ 6 } \)
9 is greater than 8.
\(\frac { 3 }{ 2 } \) > \(\frac { 4 }{ 3 } \)

Therefore, 1 ∶ \(\frac { 2 }{ 3 } \) > \(\frac { 2 }{ 3 } \) ∶ \(\frac { 1 }{ 2 } \).

(d) Given that \(\frac { 4 }{ 3 } \) : \(\frac { 5 }{ 9 } \) and \(\frac { 2 }{ 7 } \) ∶ \(\frac { 3 }{ 5 } \)
\(\frac { 4 }{ 3 } \) : \(\frac { 5 }{ 9 } \) can write as \(\frac { 36 }{ 15 } \)
\(\frac { 2 }{ 7 } \) ∶ \(\frac { 3 }{ 5 } \) can write as \(\frac { 10 }{ 21 } \)
Firstly, find the LCM of the 15 and 3.
The LCM of the 2 and 3 is 6.
\(\frac { 3 × 3 }{ 2 × 3 } \) = \(\frac { 9 }{ 6 } \)
\(\frac { 4 × 2 }{ 3 × 2 } \) = \(\frac { 8 }{ 6 } \)
Now, we can compare \(\frac { 9 }{ 6 } \) and \(\frac { 8 }{ 6 } \)
9 is greater than 8.
\(\frac { 3 }{ 2 } \) > \(\frac { 4 }{ 3 } \)

Therefore, 1 ∶ \(\frac { 2 }{ 3 } \) > \(\frac { 2 }{ 3 } \) ∶ \(\frac { 1 }{ 2 } \).

---

6. (a) If a : b = 12 : 10 and b : c = 20 : 18, find a : c.
(b) If x : y = \(\frac { 1 }{ 3 } \) ∶ \(\frac { 1 }{ 4 } \) and y : z = \(\frac { 1 }{ 4 } \) ∶ \(\frac { 1 }{ 5 } \), find x : z
(c) If p : q = 6 : 10 and q : r = 12 : 14 find p : q : r
(d) If a : b = 12 : 14 and b : c = 6 : 10, find a : b : c

Solution:

(a) Given that a : b = 12 : 10 and b : c = 20 : 18.
12 : 10 can write as \(\frac { 12 }{ 10 } \)
20 : 18 can write as \(\frac { 20 }{ 18 } \)
To get a : c, multiply a/b and b/c
a/b × b/c = a/c
\(\frac { 12 }{ 10 } \) × \(\frac { 20 }{ 18 } \)
a/c = \(\frac { 4 }{ 3 } \)

Therefore, the final answer is a : c = \(\frac { 4 }{ 3 } \).

(b) Given that x : y = \(\frac { 1 }{ 3 } \) ∶ \(\frac { 1 }{ 4 } \) and y : z = \(\frac { 1 }{ 4 } \) ∶ \(\frac { 1 }{ 5 } \).
x : y = \(\frac { 1 }{ 3 } \) ∶ \(\frac { 1 }{ 4 } \) can write as \(\frac { 4 }{ 3 } \)
y : z = \(\frac { 1 }{ 4 } \) ∶ \(\frac { 1 }{ 5 } \) can write as \(\frac { 5 }{ 4 } \)
To get x : z, multiply x/y and y/z
x/y × y/z = x/z
\(\frac { 4 }{ 3 } \) × \(\frac { 5 }{ 4 } \)
x/z = \(\frac { 5 }{ 3 } \)

Therefore, the final answer is x : z = \(\frac { 5 }{ 3 } \).

(c) Given that p : q = 6 : 10 and q : r = 12 : 14.
p : q = 6 : 10 can write as \(\frac { 6 }{ 10 } \) : 1
q : r = 12 : 14 can write as 1 : \(\frac { 14 }{ 12 } \)
Therefore, p : q : r = \(\frac { 6 }{ 10 } \) : 1 : \(\frac { 14 }{ 12 } \)
Taking the L.C.M. of 10 and 12
The L.C.M. of 10 and 12 is 60.
= \(\frac { 6 }{ 10 } \) × 60 : 1 × 60 : \(\frac { 14 }{ 12 } \) × 60
= 36 : 60 : 70

Therefore, the final answer is p: q: r = 36: 60: 70.

(d) Given that a : b = 12 : 14 and b : c = 6 : 10.
a : b = 12 : 14 can write as \(\frac { 12 }{ 14 } \) : 1
b : c = 6 : 10 can write as 1 : \(\frac { 10 }{ 6 } \)
Therefore, p : q : r = \(\frac { 12 }{ 14 } \) : 1 : \(\frac { 10 }{ 6 } \)
Taking the L.C.M. of 14 and 6
The L.C.M. of 14 and 6 is 42.
= \(\frac { 12 }{ 14 } \) × 42 : 1 × 42 : \(\frac { 10 }{ 6 } \) × 42
= 36 : 42 : 70 = 18 : 21 : 35

Therefore, the final answer is a: b: c = 18: 21: 35.

---

7. Are the following ratios equivalent?
(a) 6: 10 and 30: 50
(b) 2: 8 and 4 : 6
(c) 16: 6 and 48: 60
(d) 5: 7 and 10: 14

Solution:

(a) Given that 6: 10 and 30: 50.
From the given data, extreme terms are 6 and 50, mean terms are 10 and 30.
Find the Product of extreme terms and mean terms.
Product of extreme terms = 6 × 50 = 300
Product of mean terms = 10 × 30 = 300.
Compare the Product of extreme terms and the Product of mean terms.
If the product of means = product of extremes, the given ratios are equivalent.
300 = 300

Therefore, 6 : 10 and 30 : 50 ratios are equivalent.

(b) Given that 2: 8 and 4: 6.
From the given data, extreme terms are 2 and 6, mean terms are 8 and 4.
Find the Product of extreme terms and mean terms.
Product of extreme terms = 2 × 6 = 12
Product of mean terms = 8 × 4 = 32.
Compare the Product of extreme terms and the Product of mean terms.
If the product of means = product of extremes, the given ratios are equivalent.
12 is not equal to 32

Therefore, 2: 8 and 4: 6 ratios are not equivalent.

(c) Given that 16: 6 and 48: 60.
From the given data, extreme terms are 16 and 60, mean terms are 6 and 48.
Find the Product of extreme terms and mean terms.
Product of extreme terms = 16 × 60 = 960
Product of mean terms = 6 × 48 = 288.
Compare the Product of extreme terms and the Product of mean terms.
If the product of means = product of extremes, the given ratios are equivalent.
960 is not equal to 288.

Therefore, 16: 6 and 48: 60 ratios are not equivalent.

(d) Given that 5: 7 and 10: 14.
From the given data, extreme terms are 5 and 14, mean terms are 7 and 10.
Find the Product of extreme terms and mean terms.
Product of extreme terms = 5 × 14 = 70
Product of mean terms = 7 × 10 = 70.
Compare the Product of extreme terms and the Product of mean terms.
If the product of means = product of extremes, the given ratios are equivalent.
70 is equal to 70.

Therefore, 5: 7 and 10: 14 ratios are equivalent.

---

8. Two numbers are in the ratio 8 : 3. If their difference is 75, find the numbers.

Solution:

Given that two numbers are in the ratio 8 : 3 and the difference between them is 75.
From the given data, let the numbers are 8a and 3a.
Now, subtract the 3a from 8a.
8a – 3a = 75
5a = 75
a = \(\frac { 75 }{ 5 } \)
a = 15.
Now, substitute the a value to find the numbers.
The first number = 8a = 8 × 15 = 120
The second number = 3a = 3 × 15 = 45

Therefore, the two numbers are 120 and 45.

---

9. Two numbers are in the ratio 6: 4 and their sum is 120. Find the numbers?

Solution:

Given that two numbers are in the ratio 6 : 4 and their sum is 120.
From the given data, let the numbers are 6a and 4a.
Now, add 6a and 4a.
6a + 4a = 120
10a = 120
a = \(\frac { 120 }{ 10 } \)
a = 12.
Now, substitute the a value to find the numbers.
The first number = 6a = 6 × 12 = 72
The second number = 4a = 4 × 12 = 48

Therefore, the two numbers are 72 and 48.

---

10. The three angles of a scalene triangle are in the ratio 4: 6: 10. Find the measures of the angles.

Solution:

Given that the three angles of a scalene triangle are in the ratio 4 : 6 : 10.
From the given data, let the numbers are 4a, 6a, and 10a.
We know that the sum of three angles of a triangle is 180°.
Now, add 4a, 6a, and 10a.
4a + 6a + 10a = 180°
20a = 180°
a = \(\frac { 180° }{ 20 } \)
a = 9°.
Now, substitute the a value to find the numbers.
The first angle = 4a = 4 × 9° = 36°
The second angle = 6a = 6 × 9° = 54°
Also, the third angle = 10a = 10 × 9° = 90°

Therefore, the three angles are 36°, 54°, and 90°.

---

11. Divide $369 in the ratio 4: 5.

Solution:

Given that two numbers are in the ratio 4 : 5.
From the given data, let the numbers are 4a and 5a.
Now, add 4a and 5a.
4a + 5a = 9a
Given 9a = $369
9a = $369
a = \(\frac { $369 }{ 9 } \)
a = $41.
Now, substitute the a value to find the numbers.
The first number = 4a = 4 × $41 = $164
The second number = 5a = 5 × $41= $205

Therefore, the two numbers are $164 and $205.

---

12. Divide 72 chocolates in the ratio of 4: 6: 8.

Solution:

Given that two numbers are in the ratio 4 : 6 : 8.
From the given data, let the numbers are 4a, 6a, and 8a.
Now, add 4a, 6a, and 8a.
4a + 6a + 8a = 18a
Given 18a = 72
18a = 72
a = \(\frac { 72 }{ 18 } \)
a = 4.
Now, substitute the a value to find the numbers.
The first number = 4a = 4 × 4 = 16
The second number = 6a = 6 × 4 = 24
Also, the second number = 8a = 8 × 4 = 32

Therefore, the three numbers are 16, 24, and 32.

---

13. A-line segment of length 40 cm is divided into three parts in the ratio 3: 2: 5. Find the length of each part?

Solution:

Given that a line segment of length 40 cm is divided into three parts in the ratio 3 : 2 : 5.
From the given data, let the numbers are 3a, 2a, and 5a.
Now, add 3a, 2a, and 5a.
3a + 2a + 5a = 10a
Given 10a = 40 cm
10a = 40 cm
a = \(\frac { 40 }{ 10 } \)
a = 4.
Now, substitute the a value to find the numbers.
The first number = 3a = 3 × 4 = 12
The second number = 2a = 2 × 4 = 8
Also, the second number = 5a = 5 × 4 = 20

Therefore, the three numbers are 12, 8, and 20.

---

14. A certain amount is divided into two parts in the ratio of 8: 10. If the first part is 240, find the total?

Solution:

Given that a certain amount is divided into two parts in the ratio 8: 10. The first part is equal to 240.
From the given data, let the numbers are 8a and 10a.
Now, calculate a from 8a = 240.
a = \(\frac { 240 }{ 8 } \)
a = 30
Now, substitute the a value to find the second part.
The second part = 10a = 10 × 30 = 300.
Now, add the first part and second part to find out the total.
Total = 240 + 300 = 540

Therefore, the total amount is 540.

---

15. Divide $688 into three parts such that the first part is 4/3 of the second and the ratio between the second and third is 8: 10.

Solution:

Given that $688 divided into three parts such that the first part is 4/3 of the second and the ratio between the second and third is 8: 10
From the given data, let the common multiple of these ratio = a.
Then, the second and third ratio = 8a and 10a
Given that the first part is 4/3 of the second part
The first part is \(\frac { 4 }{ 3 } \) × 8a = \(\frac { 32a }{ 3 } \)
Given \(\frac { 32a }{ 3 } \) + 8a + 10a = $688
Given \(\frac { 86a }{ 3 } \) = $688
86a = $2064
Now, calculate a from 86a = $2064.
a = \(\frac { $2064 }{ 86 } \)
a = 24
Now, substitute the a value to find the answer.
The first part = \(\frac { 32a }{ 3 } \) = \(\frac { 32 }{ 3 } \) × 24 = 256.
The second part = 8a = 8 × 24 = 192.
The third part = 10a = 10 × 24 = 240.

Therefore, the final answer is 256, 192, 240.

---

16. Divide 216 bottles of juice between A and B in the ratio \(\frac { 2 }{ 5 } \) : \(\frac { 2 }{ 7 } \).

Solution:

Given that 216 bottles of juice divided between A and B in the ratio \(\frac { 2 }{ 5 } \) : \(\frac { 2 }{ 7 } \)
From the given data, let the common multiple of these ratio = a.
Then, the first and second numbers are = \(\frac { 2a }{ 5 } \) and \(\frac { 2a }{ 7 } \)
Now, add \(\frac { 2a }{ 5 } \) and \(\frac { 2a }{ 7 } \)
\(\frac { 2a }{ 5 } \) + \(\frac { 2a }{ 7 } \) = \(\frac { 24a }{ 35 } \)
Now, calculate a from \(\frac { 24a }{ 35 } \) = 216.
a = \(\frac { 7560 }{ 24 } \)
a = 315
Now, substitute the a value to find the answer.
The first part = \(\frac { 2 }{ 5 } \) a = \(\frac { 2 }{ 5 } \) × 315 = 126.
The second part = \(\frac { 2 }{ 7 } \) a = \(\frac { 2 }{ 7 } \) × 315 = 90.

Therefore, the final answer is 126, and 90.

---

17. The sides of the quadrilateral are in the ratio 2: 4: 6: 8 and the perimeter is 80 cm. Find the length of each side?

Solution:

Given that the sides of the quadrilateral are in the ratio 2 : 4 : 6 : 8 and the perimeter is 80 cm.
From the given data, let the common multiple of these ratio = a.
Add 2a, 4a, 6a, and 8a.
2a + 4a + 6a + 8a = 80.
20a = 80
a = 80/20 = 4
Now, substitute the a value to find the answer.
The first part = 2a = 2 × 4 = 8.
The second part = 4a = 4 × 4 = 16.
Also, the third part = 6a = 6 × 4 = 24.
The fourth part = 8a = 8 × 4 = 32.

Therefore, the length of each side is 8, 16, 24, and 32.

---

Are you facing any trouble in understanding the concept of Adding Integers? If so, don’t worry as we have covered everything on how we can add two integers. Go through the entire article to be well versed with details like Adding Integers Definition, Rules, Steps for Integers Addition. Furthermore, you can have an insight on the Addition of Integers Examples with Answers for a better understanding of the concept.

Also Read:

• Examples on Multiplication of Integers
• Examples on Division of Integers
• Properties of Division of Integers

What is an Integer?

An integer is a whole number (can have a positive or a negative sign) that completes itself. An integer can never be a fraction number.

Integers Addition Methods

There are various methods to find the addition of integers such as the Concrete Method, Number Line Method, Absolute Value Method. We have explained how to add integers using the Absolute Value Method in detail.

Absolute Value: Absolute value is the magnitude of a real number or a whole number irrespective of its sign or its relation with other values.

Addition of Integers Possibilities

In general, there are three possibilities while adding integers. They are as follows

• The addition between two positive numbers
• The addition between two negative numbers
• The addition between a positive number and a negative number

Rules for Adding Integers

Now that we know what absolute value is, let’s see how we can use this method to add integers. Here in this method, we have to keep two rules in our mind as mentioned below.

Rule 1: If the sign of both the integers is the same you have to add the absolute value and take the same sign for the result.

Rule 2: If the sign of both the integers is different you have to subtract the absolute value of the integers and take the sign of the integer having of larger absolute value as the result.

Addition of Integers Examples with Answers

Example 1: 

Add two positive integers (4) and (5)

Solution:

(4) + (5) = 9

Here in the above example, we took 2 integers having positive sign so we can just simply add both integers and the result will also be positive

Example 2: 

Add two negative integers (-4) & (-5)

Solution:

(-4) + (-5) = -9

Here in the above examples, we took 2 integers negative same sign so we can just simply add both integers and the result will also be negative.

Example 3: 

Add Integers (4) + (-7)?

Solution:

(4) + (-7) = -3

Here both integers are having different signs so we have to subtract the absolute value of the numbers. So here the absolute value of (4) is (4) and (-7) is (7). Now we have to subtract 7 from 4 which is 3.

According to our rule, the number with the largest absolute value is going to determine the sign of the result. So here (-7) is having the largest absolute value so the result is going to be negative.

Example 4: 

Add integers be (-4) + (7)?

Solution:

(-4) + (7) = 3

Here both integers are having different signs so we have to subtract the absolute value of the numbers. So here the absolute value of (-4) is (4) and (7) is (7). Now we have to subtract 7 from 4 which is 3.

According to our rule, the number with the largest absolute value is going to determine the sign of the result. So here (7) is having the largest absolute value so the result is going to be positive.

FAQs on Addition of Integers

1. When two positive integers are added together, then what is the sign of resulted value?

When two positive integers are added together, is the sum of two integers with a positive sign.

2. What are the rules for Adding Integers?

Rules for Addition of Integers is as follows

• When two integers with positive signs are added the result will also have a positive sign.
• When two integers with one positive and negative sign are added resultant sign is the value of integer having the largest value.
• When two integers with negative signs are added the result will also have a negative sign.

3. What is th result when integers 6 and -3 are added?

When integers 6 and -3 are added the sum is 6+(-3) i.e. 3

A decimal is a number whose decimal part and fractional part are separated using a decimal point. In this article, we have presented all about Non-Terminating Decimals such as Definition How to Identify a Non-Terminating or Recurring Decimal all in one place. Refer to Solved Examples on Non-Terminating Decimals so that you will better understand the concept.

Also, Check:

• Terminating Decimal
• Decimals

Non-Terminating Decimal – Definition

While converting a fraction to decimal we usually perform division operation and obtain a certain remainder. If the division procedure doesn’t end and we get a remainder other than zero, then such decimal is called a non-terminating decimal.

Example: 2.666 is a non-terminating repeating decimal and can be written as 2.\( \overline6\)

How to Determine if it is a Non-Terminating Decimal?

Follow the simple guidelines listed below to determine whether a given decimal number a non-terminating decimal or not. They are along the lines

• Whenever we perform a division we get a certain block of digits that do repeat in the decimal part.
• Such Decimals are the ones that don’t end and continue and are called as Non-Terminating Decimal or Recurring Decimal.
• You can represent these decimals by placing a bar on the repeated part.

Examples of Non-Terminating Decimals or Recurring Decimals

1. Express 7/3 in decimal form and determine whether it is a non-terminating decimal or not?

Solution:

Perform division operation and check if the decimal quotient has finite numbers or infinite numbers. If the digits after the decimal part don’t end and continue then they are called Non-Terminating or Recurring Decimals.

Since 7/3 has an infinite number of digits after the decimal point i.e. 2.3333333…. thus it is a non-terminating decimal.

2. Express 125/99 in decimal form and determine whether it is a non-terminating decimal or not?

Solution:

Perform division operation and check if the decimal quotient has finite numbers or infinite numbers. If the digits after the decimal part don’t end and continue then they are called Non-Terminating or Recurring Decimals.

Since 125/99 has an infinite number of digits after the decimal point i.e. 1.2626…… thus it is non-terminating.

FAQs on Non-Terminating Decimals

1. What is a Non-Terminating Decimal?

A Non-Terminating Decimal is a decimal number that continues endlessly.

2. How do you know if a decimal is Non-Terminating?

After performing division if you get a certain block of digits that repeat in the decimal part then the decimal part is called a Non-Terminating Decimal.

3. Is 0.544444….. a non-terminating decimal?

Yes, 0.54444…. is a non-terminating decimal as it has an infinite number of terms.

Seek help regarding the Terminating Decimals concept from this webpage. Get to know all about Terminating Decimals such as Definitions, How to Determine if it is a Terminating Decimal or not by going through this entire article. We have also listed the steps on how to convert a terminating decimal to a repeating decimal here. Find Solved Examples on Terminating Decimals available here so as to understand the concept clearly.

Also, See:

• Decimal and Fractional Expansion
• Simplify Decimals Involving Addition and Subtraction Decimals
• Rounding Decimals

Terminating Decimal – Definition

A Terminating Decimal is a decimal that ends. A Decimal Number that contains a finite number of digits next to the decimal point is called a Terminating Decimal. In fact, we can rewrite the terminating decimals as fractions.

Example: 0.52, 0.625, 0.78, etc.

How to know if it is a Terminating Decimal?

While expressing a fraction in decimal form, when we perform division we observe that the division is complete after few steps i.e. we get a remainder zero. The decimal quotient obtained is known as the terminating decimal. In fact, such decimals will have a finite number of digits after the decimal point.

Terminating Decimals can be expressed as repeating decimals by simply placing zeros that are never-ending. For Example, 12.32 can be written as 12.3200000000…….

Solved Examples on Terminating Decimals

1. Express 1/4 in decimal form and check if it is terminating decimal?

Solution:

Perform Division Operation and check if the decimal quotient has a finite number of digits or not. If it has a finite number of digits after the decimal point then it is a terminating decimal.

Since 0.25 has a finite number of digits next to the decimal point it is considered a terminating decimal.

2. Express 15/4 in Decimal Form and check if it is terminating decimal?

Solution:

Perform Division Operation and check if the decimal quotient has a finite number of digits or not. If it has a finite number of digits after the decimal point then it is a terminating decimal.

Since 3.75 has a finite number of digits next to the decimal point it is considered as a terminating decimal.

3. Express 23/5 in Decimal Form?

Solution:

Perform Division Operation and check if the decimal quotient has a finite number of digits or not. If it has a finite number of digits after the decimal point then it is a terminating decimal.

Since the decimal quotient, 4.6 has a finite number of decimal places next to the decimal point it is called a terminating decimal.

FAQs on Terminating Decimal

1. What is a terminating decimal?

Terminating Decimal is a Decimal Number that contains a finite number of digits next to the decimal point.

2. Is 2.3 a terminating decimal?

Yes, 2.3 is a terminating decimal as it has a finite number of digits after the decimal point.

3. How do you find terminating decimals without actual division?

Prime Factorization of the denominator should contain factor 2 or factor 5 or both factors 2 and 5 to tell if it’s a terminating decimal. Any factor other than these gives a non-terminating decimal.

Problems on frequency polygon are here. Check the practice material and solution of frequency polygons. Get the various steps to solve these problems in an easy manner. Follow the step-by-step procedure to solve frequency polygon problems. Know the various polygon concepts and examples. Check the below sections to know the polygon concepts, step-by-step procedure, example problems, etc.

Also, Read:

• Different Types of Polygons
• Convex and Concave Polygons
• Regular and Irregular Polygon

Polygon – Definition

Any closed 2-D shaped figure bounded by three or more sides is a polygon. If all the sides of the polygon are equal, then it is called the regular polygon. Before going to solve frequency polygon problems, check the formulae here.

Properties of Polygon

1. Sum of all the interior angles of a regular polygon: (n-2)180°
2. Each interior angle of a regular polygon: (n-2)180°/n
3. Number of Diagonals: n(n-3)/2
4. Sum of all exterior angles of a regular polygon: 360°

Problem 1:

The runs scored by two teams A and B on the first 60 balls in a cricket match are given below.

S. No	Number of Balls	Team A	Team B
1	1-6	2	5
2	7-12	1	6
3	13-18	8	2
4	19-24	9	10
5	25-30	4	5
6	31-36	5	6
7	37-42	6	3
8	43-48	10	4
9	49-54	6	8
10	55-60	2	10

Represent the data of both the teams on the same graphs by frequency polygons. [Hint: First make the class intervals continuous]

Solution:

As given in the question,

To make the class intervals continuous, subtract 0.5 from the lowest value and add 0.5 to the highest value

Therefore, the new intervals are

S. No	Number of Balls	Team A	Team B
1	0.5-6.5	2	5
2	6.5-12.5	1	6
3	12.5-18.5	8	2
4	19-24.5	9	10
5	24.5-30.5	4	5
6	30.5-36.5	5	6
7	36.5-42.5	6	3
8	42.5-48.5	10	4
9	48.5-54.5	6	8
10	54.5-60.5	2	10

To represent the data on the graph, we require the mean value of the interval i.e.,

1st interval mean value is 0.5 + 6.5 / 2 = 3.5

2nd interval mean value is  6.5+12.5 / 2 = 9.5

3rd interval mean value is  12.5+18.5 / 2 = 15.5

4th interval mean value is 19+24.5 / 2 = 21.75

5th interval mean value is 24.5+30.5 / 2 = 27.5

6th interval mean value is  30.5+36.5 / 2 = 33.5

7th interval mean value is 36.5+42.5 / 2 = 39.5

8th interval mean value is 42.5+48.5 / 2 = 45.5

9th interval mean value is 48.5+54.5 / 2 = 51.5

10th interval mean value is 54.5+60.5 / 2 = 57.5

The final graph is

Hence, the frequency polygons graph data is represented here.

Problem 2:

Represent the data on the same graphs by frequency polygons.

Class Interval (price of pen)	10-20	20-30	30-40	40-50	50-60
Frequency (Number of pens sold)	15	20	30	25	5

Solution:

As given in the above table,

The frequency has gradually increased and then decreased. Hence the graph is as follows.

Hence, the frequency polygons graph data is represented here.

Problem 3:

Draw a histogram, a frequency polygon and frequency curve of the following data:

Marks	0-10	10-20	20-30	30-40	40-50	50-60
Number of Students	5	12	15	22	14	4

 Solution:

By considering the above-given data

Hence, the histogram, frequency polygons graph, and curve are represented here.

Problem 4:

Each interior angle of a regular polygon is three times its exterior angle, then the number of sides of the regular polygon is?

Solution:

As given in the question,

Each interior angle of a regular polygon is three times its exterior angle

Suppose the exterior angle = 1

Then the interior angle = 3

The sum of interior and exterior angle = 4

Each side of a regular polygon = 180°

Therefore, the angle of one side = 180/4 = 45°

The complete angle of a polygon = 360°

No of sides of a polygon = 360º/N

= 360/45

= 8

Therefore, the regular polygon has 8 sides

Problem 5:

With the help of the frequency distribution for the calculus table, draw the frequency polygon graph?

Lower Bound	Upper Bound	Frequency	Cumulative Frequency
49.5	59.5	5	5
59.5	69.5	10	15
69.5	79.5	30	45
79.5	89.5	40	85
89.5	99.5	15	100

 Solution:

As given in the question,

We consider the scores and test scores of frequency distribution for the calculus

The frequency polygon distribution graph is

Hence, the frequency polygons graph data is represented here.

Problem 6:

With the help of the frequency distribution, draw the frequency polygon graph?

Age (in years)	0-5	5-10	10-20	20-40	40-50	50-80
Number of Persons	7	9	14	12	8	15

 Solution:

As we know,

Adjusted frequency of a class = Minimum class size / Class size * Frequency

The frequency polygon distribution graph is as follows

Hence, the frequency polygons graph data is represented here.

Problem 7:

With the help of the frequency distribution, draw the frequency polygon graph?

CB	Frequency
0.5 – 10.5	2
10.5 – 20.5	3
20.5 – 30.5	6
30.5 – 40.5	9
40.5 – 50.5	4

 Solution:

As we know,

The frequency polygon distribution graph is as follows

Hence, the frequency polygons graph data is represented here.

Problem 8:

With the help of the frequency distribution, draw the frequency polygon graph?

Upper Bound	Lower Bound	Midpoint	Frequency
10	19	14.5	4
20	29	24.5	5
30	39	34.5	7
40	49	44.5	5
50	59	54.5	5
60	69	64.5	4

 Solution:

As we know,

The frequency polygon distribution graph is as follows

Hence, the frequency polygons graph data is represented here.

Problem 9:

The following is the distribution of workers of a factory. On the basis of this information, construct a histogram and convert it into a frequency polygon.

Age (In Years)	20-30	30-40	40-50	50-60	60-70
No of workers	15	25	7	5	2

Solution:

As we know,

The frequency polygon distribution graph is as follows

Hence, the frequency polygons graph data is represented here.

Problem 10:

The following is the distribution of workers of a factory. On the basis of this information, construct a histogram and convert it into a frequency polygon.

Marks	Mid Value	Number of Students
10-20	15	10
20-30	25	15
30-40	35	20
40-50	45	22
50-60	55	15
60-70	65	10

Solution:

As we know,

The frequency polygon distribution graph is as follows

Hence, the frequency polygons graph data is represented here.