Engage NY Eureka Math Algebra 2 Module 2 Lesson 4 Answer Key
Eureka Math Algebra 2 Module 2 Lesson 4 Example Answer Key
Example 1.
Suppose that point P is the point on the unit circle obtained by rotating the initial ray through 30°. Find sin(30°) and cos(30°).
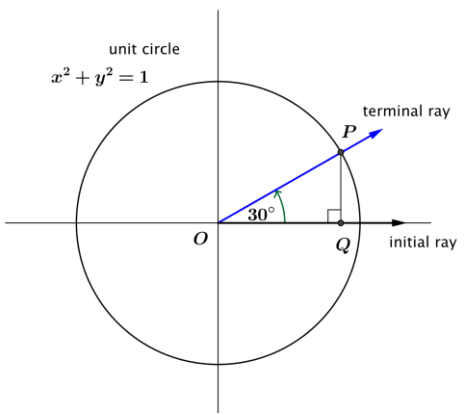
What is the length OQ of the horizontal leg of our triangle?
Answer:
By remembering the special triangles from geometry, we have OQ = \(\frac{\sqrt{3}}{2}\).
What is the length QP of the vertical leg of our triangle?
Answer:
Either by the Pythagorean theorem or by remembering the special triangles from Geometry, we have QP = \(\frac{1}{2}\)
What is sin(30°)?
Answer:
sin(30°) = \(\frac{1}{2}\)
What is cos(30°)?
Answer:
cos(30°) = \(\frac{\sqrt{3}}{2}\)
Example 2.
Suppose that P is the point on the unit circle obtained by rotating the initial ray through 150°. Find sin(150°) and cos(150°).
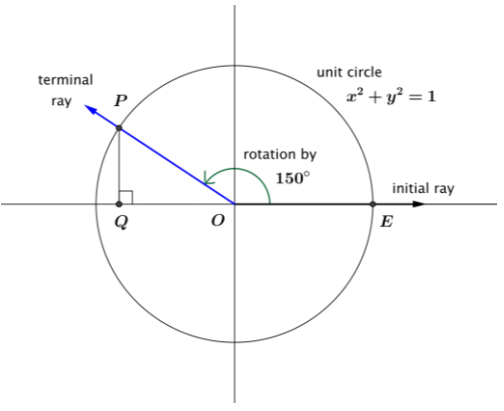
Answer:
Notice that the 150° angle formed by \(\overrightarrow{O P}\) and \(\overrightarrow{O E}\) is exterior to the right triangle ∆ POQ. Angle POQ is the reference angle for rotation by 150°. We can use symmetry and the fact that we know the sine and cosine ratios of 30° to find the values of the sine and cosine functions for 150 degrees of rotation.
→ What are the coordinates (xθ, yθ) of point P?
Using symmetry, we see that the y-coordinate of P is the same as it was for a 30° rotation but that the x-coordinate is the opposite sign as it was for a 30° rotation. Thus (xθ, yθ) = \(\left(-\frac{\sqrt{3}}{2}, \frac{1}{2}\right)\)
→ What is sin(150°)?
sin (150°) = \(\frac{1}{2}\)
→ What is cos(150°)?
cos (150°) = \(-\frac{\sqrt{3}}{2}\)
Eureka Math Algebra 2 Module 2 Lesson 4 Opening Exercise Answer Key
Exercise 1.
Find the lengths of the sides of the right triangles below, each of which has hypotenuse of length 1.
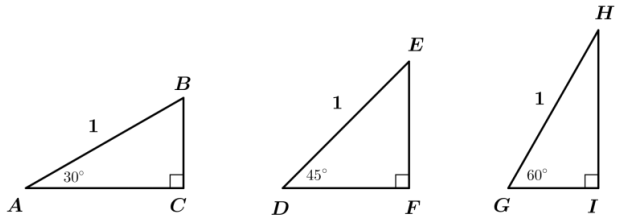
Answer:
Exercise 2.
Given the following right triangle ∆ ABC with m∠A = θ°, find sin(θ°) and cos(θ°).
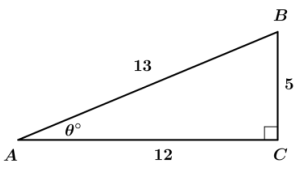
Answer:
Eureka Math Algebra 2 Module 2 Lesson 4 Exercise Answer Key
Exercise 1.
Suppose that P Is the point on the unit circle obtained by rotating the initial ray through 45°. Find sin(45°) and cos(45°).
Answer:
We have sin(45°) = \(\frac{\sqrt{3}}{2}\) and cos(45°) = \(\frac{\sqrt{2}}{2}\).
Exercise 2.
Suppose that P is the point on the unit circle obtained by rotating the initial ray through 60°. Find sin(60°) and cos (60°).
Answer:
We have sin(60°) = \(\frac{\sqrt{3}}{2}\) and cos(60°) = \(\frac{1}{2}\).
Discussion
Answer:
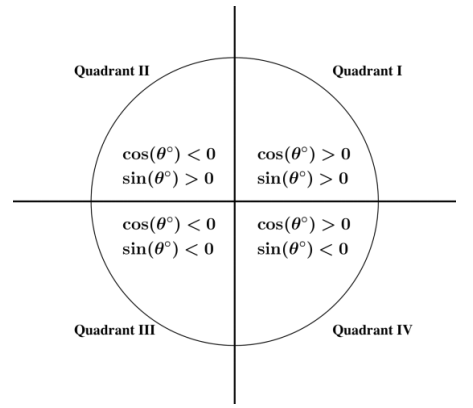
Exercise 3.
Suppose that P is the point on the unit circle obtained by rotating the initial ray counterclockwise through 120 degrees. Find the measure of the reference angle for 120°, and then find sin(120°) and cos(120°).
Answer:
The measure of the reference angle for 120° is 60°, and P is in Quadrant II. We have sin(120°) = \(\frac{\sqrt{3}}{2}\) and cos(120°) = –\(\frac{1}{2}\).
Exercise 4.
Suppose that P is the point on the unit circle obtained by rotating the initial ray counterclockwise through 240°. Find the measure of the reference angle for 240°, and then find sin(240°) and cos(240°).
Answer:
The measure of the reference angle for 240° is 60°, and P is in Quadrant Ill. We have sin(240°) = –\(\frac{\sqrt{3}}{2}\) and cos(240°) = –\(\frac{1}{2}\).
Exercise 5.
Suppose that P is the point on the unit circle obtained by rotating the initial ray counterclockwise through 330° degrees. Find the measure of the reference angle for 330°, and then find sin(330°) and cos(330°).
Answer:
The measure of the reference angle for 330° is 30°, and P is in Quadrant IV. We have sin(330°) = –\(\frac{1}{2}\) and cos(330°) = \(\frac{\sqrt{3}}{2}\).
Eureka Math Algebra 2 Module 2 Lesson 4 Problem Set Answer Key
Question 1.
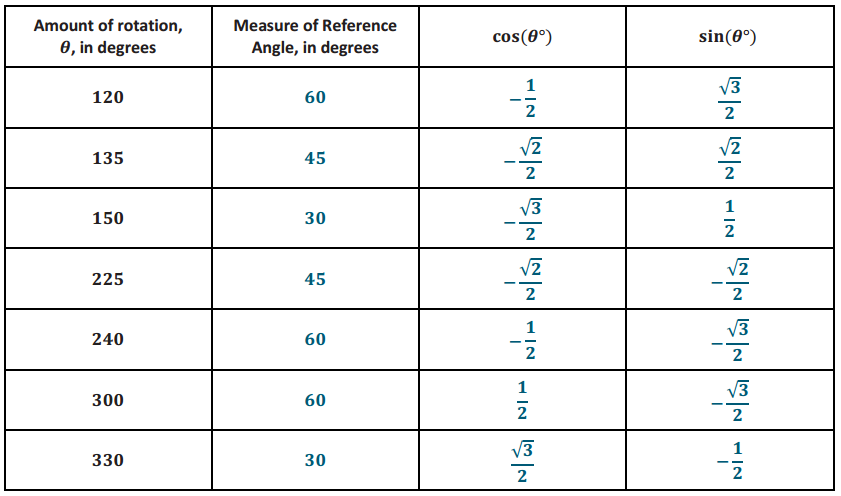
Fill in the chart. Write in the reference angles and the values of the sine and cosine functions for the indicated values of θ.
Answer:
Question 2.
Using geometry, Jennifer correctly calculated that sin(15°) = \(\frac{1}{2} \sqrt{2-\sqrt{3}}\) . Based on this information, fill in the chart.
Answer:
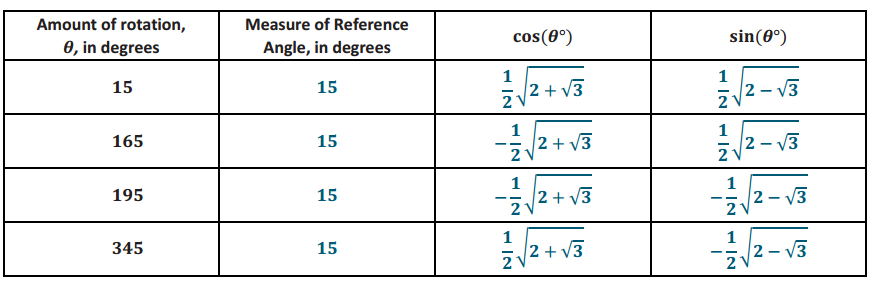
Question 3.
Suppose 0 < θ < 90 and sin(θ°) = \(\frac{1}{\sqrt{3}}\) What is the value of cos(θ°)?
Answer:
cos(θ°) = \(\frac{\sqrt{6}}{3}\)
Question 4.
Suppose 90 < θ < 180 and sin(θ°) = \(\frac{1}{\sqrt{3}}\). What is the value of cos(θ°)?
Answer:
cos(θ°) = – \(\frac{\sqrt{6}}{3}\)
Question 5.
If cos(θ°) = –\(\frac{1}{\sqrt{5}}\), what are two possible values of sin(θ°)?
Answer:
sin(θ°) = \(\frac{2}{\sqrt{5}}\) or sin(θ°) = –\(\frac{2}{\sqrt{5}}\)
Question 6.
Johnny rotated the initial ray through θ degrees, found the intersection of the terminal ray with the unit circle, and calculated that sin(θ°) = √2. Ernesto Insists that Johnny made a mistake in his calculation. Explain why Ernesto is correct.
Answer:
Johnny must have made a mistake since the sine of a number cannot be greater than 1.
Question 7.
If sin(θ°) = 0. 5, and we know that cos(θ°) < 0, then what is the smallest possible positive value of θ?
Answer:
150
Question 8.
The vertices of ∆ ABC have coordinates A(0, 0), B(12, 5), and C(12, 0).
a. Argue that ∆ ABC is a right triangle.
Answer:
Clearly \(\overline{\boldsymbol{A C}}\) is horizontal and \(\overline{\boldsymbol{B C}}\) is vertical, so ∆ ABC is a right triangle.
b. What are the coordinates where the hypotenuse of ∆ ABC intersects the unit circle X2 + y2 = 1?
Answer:
Using similar triangles, the hypotenuse intersects the unit circle at \(\left(\frac{12}{13}, \frac{5}{13}\right)\),
c. Let θ denote the number of degrees of rotation from \(\overrightarrow{A C}\) to \(\overrightarrow{B C}\). Calculate sin(θ°) and cos(θ°).
Answer:
By the answer to part (b), sin(θ°) = \(\frac{5}{13}\) and cos(θ°) = \(\frac{12}{13}\)
Question 9.
The vertices of ∆ ABC have coordinates A(0, 0), B(4, 3), and C(4, 0). The vertices of ∆ ADE are A(0, 0), D(3, 4), and E(3, 0).
a. Argue that ∆ ABC is a right triangle.
Answer:
Clearly \(\overline{\boldsymbol{A C}}\) is horizontal and \(\overline{\boldsymbol{B C}}\) is vertical, so ∆ ABC is a right triangle.
b. What are the coordinates where the hypotenuse of ∆ ABC intersects the unit circle x2 + y2 = 1?
Answer:
Using similar triangles, the hypotenuse intersects the unit circle at \(\left(\frac{4}{5}, \frac{3}{5}\right)\).
c. Let θ denote the number of degrees of rotation from \(\overrightarrow{A C}\) to \(\overrightarrow{B C}\). Calculate sin(θ°) and cos(θ°).
Answer:
By the answer to part (b), sin(θ°) = \(\frac{3}{5}\) and cos(θ°) = \(\frac{4}{5}\)
d. Argue that ∆ ADE is a right triangle.
Answer:
Since a2 + b2 = c2, the converse of the Pythagorean theorem guarantees that ∆ ADE is a right triangle.
e. What are the coordinates where the hypotenuse of ∆ ADE intersects the unit circle x2 + y2 = 1?
Answer:
Using similar triangles, the hypotenuse intersects the unit circle at (\(\left(\frac{3}{5}, \frac{4}{5}\right)\)).
f. Let Φ denote the number of degrees of rotation from \(\overrightarrow{A E}\) to \(\overrightarrow{A D}\). Calculate sin(Φ°) and cos(Φ°).
Answer:
By the answer to part (e), sin(Φ°) = \(\frac{4}{5}\), and cos(Φ°) = \(\frac{3}{5}\)
g. What is the relation between the sine and cosine of θ and the sine and cosine of Φ?
Answer:
We find that sin(Φ°) = \(\frac{4}{5}\) = cos(θ°), and cos(Φ°) = \(\frac{3}{5}\) = sin(θ°).
Question 10.
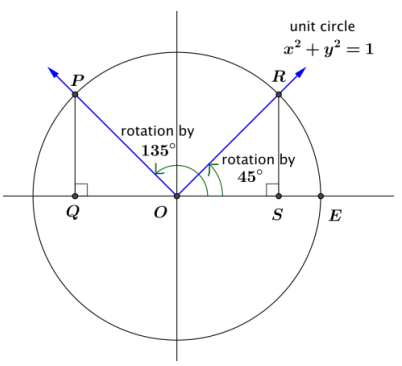
Use a diagram to explain why sin(135°) = sin(45°), but cos(135°) ≠ cos(45°).
Answer:
Let O be the center of the circle, let P and R be the points where the terminal rays of rotation by 135° and 45°, and let Q and S be the feet of the perpendicular lines from P and R to the x-axis, respectively. Then △ OPQ and △ ORS are both isosceles right triangles with hypotenuses of length 1, so they are congruent. Thus, PQ = RS, and OQ = OS. Let the coordinates of P and R be (xp, yp,) and (xR, yR). Then xp = – OQ = – OR = – xR, and yp = PQ = RS = yQ. Then we have cos(135°) = – cos(45°), and sin(135°) = sin(45°).
Eureka Math Algebra 2 Module 2 Lesson 4 Exit Ticket Answer Key
Question 1.
How did we define the sine function for a number of degrees of rotation 8, where 0 < θ < 360?
Answer:
First we rotate the initial ray counterclockwise through θ degrees and find the intersection of the terminal ray with the unit circle. This intersection is point P. The y-coordinate of point P is the value of sin(θ°).
Question 2.
Explain how to find the value of sin(210°) without using a calculator.
Answer:
The reference angle for and angle of measure 210° has measure 30°, and a rotation by 210° counterclockwise places the terminal ray in the 3rd quadrant, where both coordinates of the intersection point P are negative.
So, sin(210°) = – sin(30°) = – \(\frac{1}{2}\)